Optimal predator control policy and weak Allee effect in a delayed prey–predator system

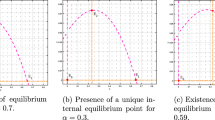
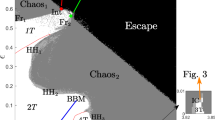
Abstract

In this article, a system of delay differential equations to represent the predator–prey dynamics with weak Allee effect in the growth of predator population is discussed. The delay parameter regarding the time lag corresponds to the predator gestation period. Mathematical features such as uniform persistence, permanence, stability, Hopf bifurcation at the interior equilibrium point of the system are analyzed and verified by numerical simulations. Bistability between different equilibrium points is properly discussed. The chaotic behaviors of the system are recognized through bifurcation diagram, Poincare section, and maximum Lyapunov exponent. By constructing a suitable Lyapunov functional for the time-delayed model, global asymptotic stability analysis of the positive equilibrium points has been performed separately. It can be observed that the Allee parameter $\theta$ can destabilize the non-delay system, whereas $\theta$ and the attack rate of predator can stabilize the time-delayed model and can control the chaotic oscillations through period-halving bifurcation. The optimal predator control policy with Allee parameter ($\theta$) as the control parameter is also discussed.

Appendix

First, consider the transformation $z_{1}(t) = S-S_{*}$, $z_{2}(t) = P-P_{*}$.

Let $\tau =\tau ^{*}+\mu$, $\mu \in \mathbf {R}$. Then $\mu =0$ is the Hopf bifurcation value of system (3). Equation (3) can be written in the form

$$\begin{aligned} \dot{z}(t)=L_{\mu }(z_{t})+F(\mu , z_{t}), \end{aligned}$$

(45)

where $z(t)=(z_{1}(t), z_{2}(t))^\mathrm{T}\in \mathbf {R^{2}}$. For $\psi =(\psi _{1}, \psi _{2})^\mathrm{T}\in \mathbf {C}([-1, 0], \mathbf {R^{2}_{+}})$, $L_{\mu }: \mathbf {C}\rightarrow \mathbf {R}$ and $F: \mathbf {R}\times \mathbf {C}\rightarrow \mathbf {R}$ are given by

$$\begin{aligned} L_{\mu }(\psi )= & {} (\tau ^{*}+\mu )A_3 \left( \begin{array}{c} \psi _{1}(0) \\ \psi _{2}(0) \\ \end{array} \right) \nonumber \\&+\,(\tau ^{*}+\mu )A_4 \left( \begin{array}{c} \psi _{1}(-1) \\ \psi _{2}(-1) \\ \end{array} \right) , \end{aligned}$$

(46)

and

$$\begin{aligned} F(\mu , \psi )=(\tau ^{*}+\mu ) A_5, \end{aligned}$$

(47)

where

$$\begin{aligned} A_3= & {} \left( \begin{array}{ccc} a_1 &{}\quad a_2 \\ 0 &{}\quad a_3\\ \end{array} \right) ,\\ A_4= & {} \left( \begin{array}{ccc} 0 &{}\quad 0 \\ a_4 &{}\quad a_5\\ \end{array} \right) ,\\ A_5= & {} \left( \begin{array}{l} b_1 \psi _{1}^{2}(0)+ b_2 \psi _{1}(0)\psi _{2}(0) \\ b_3 \psi _{1}(-1)\psi _{2}(-1) +b_4 \psi _{1}(-1)\psi _{2}(0)\\ \quad \quad +b_5 \psi _{2}(-1)\psi _{2}(0)+b_6 \psi _{2}^{2}(0) \\ \end{array} \right) ,\\ a_1= & {} -ab S_*;\quad a_2=-\beta S_*;\\ a_3= & {} -\alpha S_*P_*\frac{P_*}{(\theta +P_*)^2};\\ a_4= & {} \frac{ \alpha P_*^2}{\theta +P_*};\quad a_5=\frac{ \alpha S_*P_*}{\theta +P_*};\\ b_1= & {} -2ab;\quad b_2=-\beta ;\quad b_3=\frac{ \alpha P_*}{\theta +P_*};\\ b_4= & {} -\alpha \theta \frac{P_*}{(\theta +P_*)^2};\quad b_5=-\alpha \theta \frac{S_*}{(\theta +P_*)^2};\\ b_6= & {} -\alpha \theta \frac{P_*S_*}{(\theta +P_*)^2}. \end{aligned}$$

By the Riesz representation theorem, there exists a function $\eta (\theta , \mu )$ of bounded variation for $\theta \in [-1, 0]$ such that

$$\begin{aligned} L_{\mu }\psi =\int _{-1}^{0} d\eta (\theta , \mu )\psi (\theta ), \ \ \ \ \text{ for } \ \ \psi \in \mathbf {C}. \end{aligned}$$

(48)

In fact,

$$\begin{aligned} \eta (\theta , \mu )= & {} (\tau ^{*}+\mu )\left( \begin{array}{ccc} a_1 &{}\quad a_2 \\ 0 &{}\quad a_3\\ \end{array} \right) \delta (\theta )\\&-(\tau ^{*}+\mu ) \left( \begin{array}{ccc} 0 &{}\quad 0 \\ a_4 &{}\quad a_5 \\ \end{array} \right) \delta (\theta +1), \end{aligned}$$

where $\delta$ is defined by $\delta (\theta )=\Big \{^{1, \ \ \theta =0,}_{0, \ \ \theta \ne 0.}$

For $\psi \in \mathbf {C}^{1}\left( [-1, 0], \mathbf {R^{3}_{+}}\right)$, define

$$\begin{aligned} A(\mu )\psi =\left\{ \begin{array}{ll} \frac{d\psi (\theta )}{d\theta } &{}\quad \ \theta \in [-1, 0) \\ \\ \int _{-1}^{0} d\eta (\mu , s)\psi (s)&{} \quad \theta =0 \end{array} \right. \end{aligned}$$

and

$$\begin{aligned} R(\mu )\psi =\left\{ \begin{array}{c} 0, \quad \theta \in [-1, 0), \\ F(\mu , \psi ), \quad \theta =0. \end{array} \right. \end{aligned}$$

Then system (45) is of the form

$$\begin{aligned} \dot{z_{t}}=A(\mu )z_{t}+R(\mu )z_{t}, \end{aligned}$$

(49)

where $z_{t}(\theta )=z_{t}(t+\theta )$ for $\theta \in [-1, 0]$.

For $\phi \in \mathbf {C}^{1}([0, 1], (\mathbf {R^{3}_{+}})^{*})$, define

$$\begin{aligned} A^{*}\phi (s)=\left\{ \begin{array}{l} -\frac{d\phi (s)}{\mathrm{d}s}, \quad s \in (0, 1], \\ \int _{-1}^{0}d\eta ^\mathrm{T}(t, 0)\phi (-t), \quad s=0, \end{array} \right. \end{aligned}$$

and a bilinear inner product

$$\begin{aligned}&\langle \phi (s), \psi (\theta )\rangle =\overline{\phi }(0)\psi (0)-\int _{-1}^{0} \int _{\alpha =0}^{\theta }\nonumber \\&\quad \overline{\phi }(\alpha -\theta )\mathrm{d}\eta (\theta )\psi (\alpha )\mathrm{d}\alpha , \end{aligned}$$

(50)

where $\eta (\theta )=\eta (\theta , 0)$. Clearly, A(0) and $A^{*}$ are adjoint operators. We know that $\pm i\rho _{0}\tau ^{*}$ are eigenvalues of A(0). So, they are also eigenvalues of $A^{*}$. Now we search for the eigenvector of A(0) and $A^{*}$ corresponding to $i\rho _{0}\tau ^{*}$ and $-i\rho _{0}\tau ^{*}$, respectively.

The author assumes that $q(\theta )=(1, u)^\mathrm{T} e^{i\rho _{0}\tau ^{*}\theta }$ and $q^{*}(s)$ are the eigenvectors of A(0) and $A^{*}$ corresponding to $i\rho _{0}\tau ^{*}$ and $-i\rho _{0}\tau ^{*}$. Then, $A(0)q(\theta )=i\rho _{0}\tau ^{*}q(\theta )$. By the definition of A(0) and from (48), it follows that

$$\begin{aligned} \tau ^{*}\left( \begin{array}{ccc} a_1-i\rho _{0} &{}\quad a_2 \\ a_4 e^{-i\rho _{0}\tau ^{*}} &{}\quad a_5 e^{-i\rho _{0}\tau ^{*}} +a_3 -i\rho _{0}\\ \end{array} \right) q(0)=\left( \begin{array}{c} 0 \\ 0 \\ \end{array} \right) . \end{aligned}$$

Then, $q(0)=(1, u)^\mathrm{T}$,

and

$$\begin{aligned} q^{*}(s)= & {} D\left( 1, u^{*}\right) ^\mathrm{T} e^{i\rho _{0}\tau ^{*}s}, \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} u&= \frac{-a_1+i\rho _{0}}{a_2},\\ u^{*}&= -\frac{a_1+i\rho _{0}}{a_4 e^{i\rho _{0}\tau ^{*}}}. \end{aligned} \end{aligned}$$

(51)

D can be chosen in such a way that $\langle q^{*}(s), q(\theta ) \rangle =1$, $\langle q^{*}(s), \overline{q}(\theta ) \rangle =0$.

Hence,

$$\begin{aligned} \begin{aligned}&\langle q^{*}(s), q(\theta ) \rangle \\&\quad = \overline{D}(1, \overline{u^{*}})(1, u)^\mathrm{T}- \int _{-1}^{0}\int _{\zeta =0}^{\theta }\overline{D}(1, \overline{u^{*}})e^{-i\rho _{0}\tau ^{*}(\zeta -\theta )}\\&\qquad \quad d\eta (\theta )(1, u)^\mathrm{T}e^{i\rho _{0}\tau ^{*}\zeta } \mathrm{d}\zeta \\&\quad = \overline{D}\left[ 1+\overline{u^{*}}u-\int _{-1}^{0}(1, \overline{u^{*}} )\theta e^{i\rho _{0}\tau ^{*}\theta } d\eta (\theta )(1, u)^\mathrm{T}\right] \\&\quad = \overline{D}\left[ 1+\overline{u^{*}}u+ \tau ^{*} \overline{u^{*}}(a_4+a_5 u) e^{-i\rho _{0}\tau ^{*}} \right] . \end{aligned} \end{aligned}$$

Thus, $\overline{D}=\frac{1}{\left[ 1+\overline{u^{*}}u+ \tau ^{*} \overline{u^{*}}(a_4+a_5 u) e^{-i\rho _{0}\tau ^{*}} \right] }$.

To describe the center manifold $\mathbf {C}_{0}$ at $\mu =0$, we compute the coordinates by using the same notations and procedures as proposed by [63].

Let $z_{t}$ be the solution of Eq. (45) when $\mu =0$.

Define

$$\begin{aligned} \text {z}(t)=\langle q^{*}, z_{t}\rangle , \ \ \ W(t,\theta )=z_{t}(\theta )-2Re\{\text {z}(t)q(\theta ) \}.\nonumber \\ \end{aligned}$$

(52)

On the center manifold $\mathbf {C}_{0}$, we have

$$\begin{aligned} W(t, \theta )=W\Bigl (\text {z}(t), \overline{\text {z}}(t), \theta \Bigr ), \end{aligned}$$

where

$$\begin{aligned} W(\text {z}, \overline{\text {z}}, \theta )= & {} W_{20}(\theta )\frac{\text {z}^{2}}{2}+ W_{11}(\theta )\text {z}\overline{\text {z}}\\&+W_{02}(\theta )\frac{\overline{\text {z}}^{2}}{2} + W_{30}(\theta ) \frac{\text {z}^{3}}{6}+\cdot \cdot \cdot , \end{aligned}$$

$\text {z}$ and $\overline{\text {z}}$ are local coordinates for center manifold $\mathbf {C}_{0}$ in the direction of $q^{*}$ and $\overline{q}^{*}$. Here W is real when $z_{t}$ is real. For solution $z_{t} \in \mathbf {C}_{0}$ of Eq. (45), since $\mu =0$,

$$\begin{aligned} \begin{aligned}&\dot{\text {z}}(t) \\&\quad = i\rho _{0}\tau ^{*}\text {z}+ \Bigl \langle \overline{q}^{*}(\theta ), F\Bigl (0, W(\text {z}, \overline{\text {z}},\theta )+2Re\{\text {z}q(\theta )\} \Bigr ) \Bigr \rangle \\&\quad = i\rho _{0}\tau ^{*}\text {z}+\overline{q}^{*}(0)F\Bigl (0, W(\text {z}, \overline{\text {z}},0)+2Re\{\text {z}q(0)\} \Bigr )\\&\quad {\mathop {=}\limits ^{\text {def}}} i\rho _{0}\tau ^{*}\text {z}+\overline{q}^{*}(0)F_{0}(\text {z}, \overline{\text {z}}), \end{aligned} \end{aligned}$$

so $\dot{\text {z}}=i\rho _{0}\tau ^{*}\text {z}+g(\text {z}, \overline{\text {z}})$ with

$$\begin{aligned} g(\text {z}, \overline{\text {z}})= & {} \overline{q}^{*}(0)F_{0}(\text {z}, \overline{\text {z}})=g_{20}\frac{\text {z}^{2}}{2}+ g_{11}\text {z}\overline{\text {z}}\nonumber \\&+g_{02}\frac{\overline{\text {z}}^{2}}{2} + g_{21} \frac{\text {z}^{2}\overline{\text {z}}}{2}+\cdot \cdot \cdot . \end{aligned}$$

(53)

Then from Eq. (52),

$$\begin{aligned} \begin{aligned} z_{t}(\theta )&=(z_{1t}(\theta ), z_{2t}(\theta )) = W(t,\theta )+2Re\{\text {z}(t)q(\theta ) \} \\&= W_{20}(\theta )\frac{\text {z}^{2}}{2}+ W_{11}(\theta )\text {z}\overline{\text {z}} +W_{02}(\theta )\frac{\overline{\text {z}}^{2}}{2}\\&\quad +\,(1, u)^\mathrm{T} e^{i\rho _{0}\tau ^{*}\theta } \text {z}+ (1, \overline{u})^\mathrm{T} e^{-i\rho _{0}\tau ^{*}\theta } \overline{\text {z}}\\&\quad +\,O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}). \end{aligned} \end{aligned}$$

(54)

Thus, from Eq. (53),

$$\begin{aligned} g(\text {z}, \overline{\text {z}})&=\overline{q}^{*}(0)F_{0}(\text {z}, \overline{\text {z}}) \\&=\overline{D}(1, \overline{u^{*}})\tau ^{*}\nonumber \\&\quad \left( \begin{array}{l} b_1 z_{1t}^{2}(0)+b_2 z_{1t}(0)z_{2t}(0) \\ b_3 z_{1t}(-1)z_{2t}(-1)+b_4 z_{1t}(-1)z_{2t}(0)\\ \quad \quad +b_5 z_{2t}(-1)z_{2t}(0) +b_6 z_{2t}^{2}(0)\\ \end{array} \right) \\&= \overline{D}\tau ^{*} \Big [b_1 \Big \{ z+\overline{z}+\,W_{20}^{1}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{1}(0)\text {z}\overline{\text {z}}\\&\quad +W_{02}^{1}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3})\Big \}^{2} \\&\quad + b_2 \left\{ z+\overline{z}+W_{20}^{1}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{1}(0)\text {z}\overline{\text {z}} \right. \\&\quad \left. +W_{02}^{1}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}) \right\} \\&\quad \times \left\{ uz+ \overline{u} \ \overline{z}+W_{20}^{2}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{2}(0)\text {z}\overline{\text {z}}\right. \\&\quad \left. \left. +W_{02}^{2}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}) \right\} \right] \\&\quad +\overline{D}\tau ^{*} \Big [b_3 \Big \{ z e^{-i\rho _{0}\tau ^{*}}+ \overline{z} e^{i\rho _{0}\tau ^{*}}+W_{20}^{1}(-1)\frac{\text {z}^{2}}{2}\\&\quad + W_{11}^{1}(-1)\text {z}\overline{\text {z}} +W_{02}^{1}(-1)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3})\Big \}\\&\quad \times \left\{ uz e^{-i\rho _{0}\tau ^{*}}+ \overline{u} \ \overline{z} e^{i\rho _{0}\tau ^{*}}+W_{20}^{2}(-1)\frac{\text {z}^{2}}{2}\right. \\&\left. \quad + W_{11}^{2}(-1)\text {z}\overline{\text {z}} +W_{02}^{2}(-1)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3})\right\} \\&\quad + b_4\left\{ z e^{-i\rho _{0}\tau ^{*}}+ \overline{z} e^{i\rho _{0}\tau ^{*}}+W_{20}^{1}(-1)\frac{\text {z}^{2}}{2}\right. \\&\left. \quad + W_{11}^{1}(-1)\text {z}\overline{\text {z}} +W_{02}^{1}(-1)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3})\right\} \\&\quad \times \left\{ uz+ \overline{u} \ \overline{z}+W_{20}^{2}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{2}(0)\text {z}\overline{\text {z}}\right. \\&\quad \left. +W_{02}^{2}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}) \right\} \end{aligned}$$

$$\begin{aligned}&\quad \quad \quad \quad \quad + b_5 \{uz e^{-i\rho _{0}\tau ^{*}}+ \overline{u} \ \overline{z} e^{i\rho _{0}\tau ^{*}}+W_{20}^{2}(-1)\frac{\text {z}^{2}}{2}\nonumber \\&\quad \quad \quad \quad \quad + W_{11}^{2}(-1)\text {z}\overline{\text {z}} +W_{02}^{2}(-1)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3})\}\nonumber \\&\quad \quad \quad \quad \quad \times \left\{ uz+ \overline{u} \ \overline{z}+W_{20}^{2}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{2}(0)\text {z}\overline{\text {z}}\right. \nonumber \\&\quad \quad \quad \quad \quad \left. +W_{02}^{2}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}) \right\} \nonumber \\&\quad \quad \quad \quad \quad + b_6 \left\{ uz+ \overline{u} \ \overline{z}+W_{20}^{2}(0)\frac{\text {z}^{2}}{2}+ W_{11}^{2}(0)\text {z}\overline{\text {z}}\right. \nonumber \\&\quad \quad \quad \quad \quad \left. \left. +W_{02}^{2}(0)\frac{\overline{\text {z}}^{2}}{2}+O(\mid (\text {z}, \overline{\text {z}})\mid ^{3}) \right\} ^2\right] . \end{aligned}$$

(55)

Comparing the coefficients with (53) one can obtain that

$$\begin{aligned} g_{20}=\,&2\overline{D}\tau ^{*}\Big [b_1+b_2 u +b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}\nonumber \\&+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2\Big ],\nonumber \\ g_{11}=\,&2\overline{D}\tau ^{*}\Big [b_1+ b_2 \mathfrak {R}\{u\} +b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\}\nonumber \\&+b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2\Big ],\nonumber \\ g_{02}=\,&2\overline{D}\tau ^{*}\Big [b_1+b_2 \overline{u} +b_3 \overline{u} e^{2i\rho _{0}\tau ^{*}}+b_4 \overline{u} e^{i\rho _{0}\tau ^{*}}\nonumber \\&+b_5 \overline{u}^2 e^{i\rho _{0}\tau ^{*}} +b_6 \overline{u}^2\Big ],\nonumber \\ g_{21}=\,&\overline{D}\tau ^{*}\Big [ b_2( W_{20}^{2}(0)+ 2W_{11}^{2}(0)+\overline{u} W_{20}^{1}(0)\nonumber \\&+ 2uW_{11}^{1}(0))+2 b_1 ( W_{20}^{1}(0)+ 2W_{11}^{1}(0))\nonumber \\&+2 b_6( \overline{u}W_{20}^{2}(0)+ 2 u W_{11}^{2}(0))\nonumber \\&+b_3(2uW_{11}^{1}(-1)e^{-i\rho _{0}\tau ^{*}}+\overline{u}W_{20}^{1}(-1)e^{i\rho _{0}\tau ^{*}}\nonumber \\&+ W_{20}^{2}(-1)e^{i\rho _{0}\tau ^{*}}+ 2W_{11}^{2}(-1)e^{-i\rho _{0}\tau ^{*}})\nonumber \\&+ b_4 ( 2W_{11}^{2}(0)e^{-i\rho _{0}\tau ^{*}}+W_{20}^{2}(0)e^{i\rho _{0}\tau ^{*}}\nonumber \\&+ \overline{u}W_{20}^{1}(-1)+ 2u W_{11}^{1}(-1))\nonumber \\&+ b_5 ( 2uW_{11}^{2}(0)e^{-i\rho _{0}\tau ^{*}}+\overline{u}W_{20}^{2}(0)e^{i\rho _{0}\tau ^{*}}\nonumber \\&+ \overline{u}W_{20}^{2}(-1)+ 2u W_{11}^{2}(-1))\Big ]. \end{aligned}$$

(56)

To calculate the value of $g_{21}$, we need to compute the values of $W_{20}(\theta )$ and $W_{11}(\theta )$. From Eqs. (49) and (52),

(57)

where

$$\begin{aligned} H(\text {z}, \overline{\text {z}}, \theta )= & {} H_{20}(\theta )\frac{\text {z}^{2}}{2}+ H_{11}(\theta )\text {z}\overline{\text {z}}\nonumber \\&+\,H_{02}(\theta )\frac{\overline{\text {z}}^{2}}{2}+ \cdot \cdot \cdot . \end{aligned}$$

(58)

Expanding the above series and comparing the corresponding coefficients,

$$\begin{aligned}&(A-i2\rho _{0}\tau ^{*}I)W_{20}(\theta )=-H_{20}(\theta ), \nonumber \\&\quad AW_{11}(\theta )=-H_{11}(\theta ). \end{aligned}$$

(59)

From Eq. (57), for $\theta \in [-1, 0),$

$$\begin{aligned} H(\text {z}, \overline{\text {z}}, \theta )= & {} -\overline{q}^{*}(0)F_{0}q(\theta )-q^{*}(0)\overline{F}_{0}\overline{q}(\theta )\nonumber \\= & {} -g q(\theta )-\overline{g}\ \overline{q}(\theta ). \end{aligned}$$

(60)

Comparing the coefficients with (58) gives that

$$\begin{aligned} H_{20}( \theta )=-g_{20}q(\theta )-\overline{g}_{02} \overline{q}(\theta ) \end{aligned}$$

(61)

and

$$\begin{aligned} H_{11}( \theta )=-g_{11}q(\theta )-\overline{g}_{11} \overline{q}(\theta ). \end{aligned}$$

(62)

From (59) and (61),

$$\begin{aligned} \dot{W}_{20}(\theta )=i2\rho _{0}\tau ^{*}W_{20}(\theta )+g_{20}q(\theta )+\overline{g}_{02} \overline{q}(\theta ). \end{aligned}$$

Since $q(\theta )=(1, u)^\mathrm{T} e^{i\rho _{0}\tau ^{*}\theta }$,

$$\begin{aligned} W_{20}(\theta )&=\frac{ig^{}_{20}}{\rho _{0}\tau ^{*}} q(0)e^{i\rho _{0}\tau ^{*}\theta }+\frac{i\overline{g}^{}_{20}}{3\rho _{0}\tau ^{*}} \overline{q}(0)e^{-i\rho _{0}\tau ^{*}\theta }\nonumber \\&+E_{1}e^{i2\rho _{0}\tau ^{*}\theta }, \end{aligned}$$

(63)

where $E_{1}=(E_{1}^{(1)}, E_{1}^{(2)}) \in \mathbf {R}^{2}$ is a constant vector.

Similarly, from Eqs. (59) and (62),

$$\begin{aligned} W_{11}(\theta )&=-\frac{ig^{}_{11}}{\rho _{0}\tau ^{*}} q(0)e^{i\rho _{0}\tau ^{*}\theta }\nonumber \\&+\,\frac{i\overline{g}^{}_{11}}{\rho _{0}\tau ^{*}} \overline{q}(0)e^{-i\rho _{0}\tau ^{*}\theta }+E_{2}, \end{aligned}$$

(64)

where $E_{2}=(E_{2}^{(1)}, E_{2}^{(2)}) \in \mathbf {R}^{2}$ is a constant vector.

In what follows, $E_{1}$ and $E_{2}$ in (63) and (64), respectively, should be chosen appropriately. From the definition of A and (59),

$$\begin{aligned} \int _{-1}^{0}d\eta (\theta )W_{20}(\theta )=i2\rho _{0}\tau ^{*} W_{20}(0)-H_{20}(0) \end{aligned}$$

(65)

and

$$\begin{aligned} \int _{-1}^{0}d\eta (\theta )W_{11}(\theta )=-H_{11}(0), \end{aligned}$$

(66)

where $\eta (\theta )=\eta (0, \theta )$. From (59),

$$\begin{aligned}&H_{20}(0)=-g_{02}q(0)-\overline{g}_{02}\overline{q}(0)+2 \tau ^{*}\nonumber \\&\left( \begin{array}{c} b_1+u b_2 \\ b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2 \\ \end{array} \right) \nonumber \\ \end{aligned}$$

(67)

and

$$\begin{aligned}&H_{11}(0)=-g_{11}q(0)-\overline{g}_{11}\overline{q}(0)+2 \tau ^{*}\nonumber \\&\quad \left( \begin{array}{c} b_1+\mathfrak {R}\{u\}b_2 \\ b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\} +b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2 \\ \end{array} \right) .\nonumber \\ \end{aligned}$$

(68)

Noting that

$$\begin{aligned} \left( i\rho _{0}\tau ^{*}I-\int _{-1}^{0}e^{i\rho _{0}\tau ^{*}\theta }d\eta (\theta ) \right) q(0)=0, \end{aligned}$$

and

$$\begin{aligned} \left( -i\rho _{0}\tau ^{*}I-\int _{-1}^{0}e^{-i\rho _{0}\tau ^{*}\theta }d\eta (\theta ) \right) \overline{q}(0)=0, \end{aligned}$$

and putting (63) and (67) into (65),

$$\begin{aligned}&\left( i2\rho _{0}\tau ^{*}I-\int _{-1}^{0}e^{i2\rho _{0}\tau ^{*}\theta }d\eta (\theta )\right) E_{1}\\&\quad =2\tau ^{*} \left( \begin{array}{c} b_1+u b_2 \\ b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2 \\ \end{array} \right) , \end{aligned}$$

which implies that

$$\begin{aligned}&\left( \begin{array}{ccc} -a_1 +2i\rho _{0} &{}\quad -a_2\\ -a_4 e^{-2i\rho _{0}\tau ^{*}} &{}\quad -a_5 e^{-2i\rho _{0}\tau ^{*}} +a_3 +2i\rho _{0}\\ \end{array} \right) E_{1}\nonumber \\&\quad =2 \left( \begin{array}{c} b_1+u b_2 \\ b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2 \\ \end{array} \right) .\nonumber \\ \end{aligned}$$

(69)

It follows that

$$\begin{aligned} E_{1}^{(1)}=\frac{|\Delta _{11}|}{|\Delta _{1}|}, \ \ E_{1}^{(2)}=\frac{|\Delta _{12}|}{|\Delta _{1}|}, \nonumber \\ \end{aligned}$$

(70)

where

$$\begin{aligned} \Delta _{11}= & {} 2\left( \begin{array}{ccc} b_1+u b_2 &{}\quad -a_2\\ b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2 &{}\quad -a_5 e^{-2i\rho _{0}\tau ^{*}} +a_3 +2i\rho _{0}\\ \end{array} \right) ,\\ \Delta _{12}= & {} 2\left( \begin{array}{ccc} -a_1 +2i\rho _{0} &{}\quad b_1+u b_2 \\ -a_4 e^{-2i\rho _{0}\tau ^{*}} &{}\quad b_3 u e^{-2i\rho _{0}\tau ^{*}}+b_4 u e^{-i\rho _{0}\tau ^{*}}+b_5 u^2 e^{-i\rho _{0}\tau ^{*}} +b_6 u^2\\ \end{array} \right) ,\\ \Delta _{1}= & {} \left( \begin{array}{ccc} -a_1 +2i\rho _{0} &{}\quad -a_2\\ -a_4 e^{-2i\rho _{0}\tau ^{*}} &{}\quad -a_5 e^{-2i\rho _{0}\tau ^{*}} +a_3 +2i\rho _{0}\\ \end{array} \right) . \end{aligned}$$

Similarly, putting (64) and (68) into (66),

$$\begin{aligned} \left( \int _{-1}^{0}d\eta (\theta ) \right) E_{2}=2\tau ^{*} \left( \begin{array}{c} b_1+\mathfrak {R}\{u\}b_2 \\ b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\} +b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2 \\ \end{array} \right) , \end{aligned}$$

which implies that

$$\begin{aligned} \left( \begin{array}{ccc} a_1 &{}\quad a_2\\ a_4 &{}\quad a_5+a_3\\ \end{array} \right) E_{2}=2 \left( \begin{array}{c} b_1+\mathfrak {R}\{u\}b_2 \\ b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\} +b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2 \\ \end{array} \right) , \end{aligned}$$

and hence,

$$\begin{aligned} E_{2}^{(1)}=\frac{|\Delta _{21}|}{|\Delta _{2}|}, \ \ E_{2}^{(2)}=\frac{|\Delta _{22}|}{|\Delta _{2}|}, \end{aligned}$$

(71)

where

$$\begin{aligned} \Delta _{21}= & {} \left( \begin{array}{ccc} b_1+\mathfrak {R}\{u\}b_2 &{}\quad a_2\\ b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\} +b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2 &{}\quad a_5+a_3\\ \end{array} \right) ,\\ \Delta _{22}= & {} \left( \begin{array}{ccc} a_1 &{}\quad b_1+\mathfrak {R}\{u\}b_2\\ a_4 &{}\quad b_3 \mathfrak {R}\{u\} +b_4 \mathfrak {R}\{u e^{i\rho _{0}\tau ^{*}}\} +b_5 |u|^2 \mathfrak {R}\{e^{i\rho _{0}\tau ^{*}}\} +b_6 |u|^2 \\ \end{array} \right) ,\\ \Delta _{2}= & {} \left( \begin{array}{ccc} a_1 &{}\quad a_2 \\ a_4 &{}\quad a_5+a_3 \\ \end{array} \right) . \end{aligned}$$