The role of dynamic friction in the appearance of periodic oscillations in mechanical systems

Abstract

This article investigates the appearance of periodic mechanical oscillations associated with the transition between static and dynamic friction regimes. The study employs a mechanical system with one degree of freedom and a friction model recently proposed by Brown and McPhee, whose continuity and differentiability properties make it particularly appropriate for an analytical treatment of the equations. A bifurcation study of the system, including stability analysis, transformation to normal form and numerical continuation techniques, reveals that stable periodic orbits can be created either by a supercritical Hopf bifurcation or by a saddle-node bifurcation of limit cycles. The influence of all system parameters on the appearance of periodic oscillations is investigated in detail. In particular, the effect of the friction model parameters (static-to-dynamic friction ratio and transition speed between the static and dynamic regimes) on the bifurcation behavior of the system is addressed.

1 Introduction

Mechanical systems may exhibit self-sustained oscillations in certain conditions. Such oscillations are the so-called limit cycles and are also described as isolated closed orbits in the phase portrait of the system [1]. The stick-slip phenomenon resulting from dry friction between surfaces is one of the sources of oscillations that may occur in brakes [2], towed caster wheels [3], wheel-rail contact and bowed instruments such as violins [4, 5]. Limit cycles are also found in rotordynamic systems [6, 7]. In addition, when a mechanical system is controlled, the presence of backlash, dead zone or stick-slip may also result in limit cycles which require special means for efficiently controlling the system [8, 9], where the interaction between friction and friction compensation may be in fact the source of periodic oscillations [10] and may result in steady-state errors [11]. Furthermore, dissipative effects have been shown to produce limit cycle oscillations in an underactuated Chaplygin sleigh [12].

The literature devoted to the study of stick-slip vibrations in mechanical systems is tremendously extensive. Therefore, only some researches that have been inspirational for the authors are cited here. This kind of induced vibration is, for instance, responsible for the squeal noise in windscreen wipers [13]. The study of stick-slip vibrations with the aim of controlling noise or wear is of major interest for the industry [14]. Maybe, the most simple system showing this kind of vibrations is composed of a spring and mass system that may slide or stick on a belt when the driver moves it periodically or at a constant speed [15], while other authors have studied more sophisticated belt-driven systems [16]. Wang et al. [17] concluded that the thermo-mechanical instability and dynamical interaction between shear dissipation and nonlinear friction in metal cutting induce stick-slip friction oscillations. Electromagnetic dampers in combination with friction have been efficiently used to dissipate mechanical energy converted to electrical energy through a mechanical-magnetic-electric coupling [18]. Leine et al. [4] used a simplified friction model consisting of a set of ordinary non-stiff differential equations to simulate stick-slip vibrations. Amer et al. [5] analysed stick-slip vibrations in a system of two coupled bodies through the use of a smooth friction-velocity curve. Also using a non-linear smooth friction-velocity dependence, Kang et al. [19] analysed the stick-slip oscillation in two degrees of freedom systems and found two different forms of oscillation according to the frequency distance of the two modes. When self-excitation caused by negative damping is high enough (maximum friction damping), limit cycles lose stability via a fold bifurcation, what complicates further if there is any internal resonance conditions. According to Woiwode et al. [20], increasing self-excitation intensity leads to chaos via a cascade of period-doubling bifurcations. Numerous authors have used bifurcation theory to study the onset of chaotic motion in stick-slip vibrations [21,22,23].

Many studies have their focus on the characterization of transitions from different dynamic behaviours through bifurcation analysis [24]. A Hopf bifurcation occurs when an equilibrium point changes stability because a pair of conjugate complex eigenvalues of the linearised system around the fixed point crosses the imaginary axis (see, for example, [25,26,27]). It is the simplest form of occurrence of periodic orbits in mechanical systems, appearing, for instance, in flutter [28], centrifugal flywheel governors [29], self-exciting single-disk homopolar dynamos [30], etc. Putelat et al. [31] carried out a weakly nonlinear stability analysis of the spring-block system, as well as a numerical study using the continuation package AUTO, and observed a change of nature in the Hopf bifurcation from supercritical to subcritical, as previously observed in experiments by Heslot et al [32]. Saha et al. [33] experimentally characterized the bifurcation diagram of a friction induced vibrating spring block system showing the subcriticality of the Hopf bifurcation encountered. The existence of subcritical Hopf bifurcations associated with stick-slip vibrations was also demonstrated by Veraszto et al., who found a wide bistable region in the parameter space of a single-degree-of-freedom system using hardware-in-the-loop experiments. [34].

Contact events appear frequently in mechanical systems, being a major source of nonlinear effects. These events result in a discontinuous dynamics of non-linear, friction-induced mechanical systems. Therefore, some authors have focused on the investigation of the conditions that govern the motion switching mechanism in nonlinear oscillators [35,36,37]. The modelling of contact in such systems has attracted many researchers during past and recent years [38]. The different modelling techniques are classified as regularized, when contacting bodies are assumed to be flexible at the contact zone and the contact forces are expressed as a continuous function of the local deformation, and non-smooth, where the contacting bodies are assumed to be rigid, and the contact dynamics is included by adding unilateral constraints. Frictional contact events are frequently considered in state of the art mechanical system models, where the selection of an adequate contact modelling technique has a large influence on the numerical accuracy and efficiency of the numerical simulation [39,40,41]. The use of continuous dry friction models as LuGre model [42] eliminates the need for a stick-slip transition criterion as is required by static friction models [43]. In the context of multibody systems, Marques et al. [44] presented a comparison of the performance of well-known friction force models as the Dahl, Reset Integrator, LuGre and Gonthier models in terms of their constituting parameters. Also, Jaiswal at al. [45] presented a comparative study of the Bengisu-Akay model [46, 47], the recent Brown-McPhee’s model [48], the LuGre model and a modified LuGre model [49] in the modelling of friction of hydraulic cylinders in multibody systems applications. They concluded that Brown-McPhee’s model is numerically efficient and capable of describing usual friction features in dynamic simulation of hydraulically driven multibody systems. Nevertheless, the interest in developing friction models continues active and a new continuously differentiable, dynamic dry friction model has been recently proposed by Chaturvedi et al. [50]. This novel approach, which depends on normal contact force, slip velocity, and static and dynamic friction coefficients, aims at overcoming the fact that the Brown and McPhee friction model depends on a transitional velocity, which is an empirical parameter.

This research explores periodic mechanical oscillations during the transition from static to dynamic friction. Using a single-degree-of-freedom mechanical system and Brown-McPhee’s friction model, known for its analytical convenience, a bifurcation analysis is conducted. The study reveals stable periodic orbits generated through supercritical Hopf bifurcations or saddle-node bifurcations of limit cycles. The influence of all system parameters on the occurrence of periodic oscillations is closely examined, with special interest in those parameters associated with the friction model.

The structure of this document is as follows. The mechanical system to be studied as well as the continuous dry friction model are presented in Sect. 2. Section 3 includes an analytical study of the stability and the bifurcations of the system at hand. Some numerical results used to validate, complement and illustrate the mentioned analytical developments are discussed in Sect. 4. Finally, a summary and the main conclusions drawn from this analysis are included in Sect. 5.

2 Mechanical system of interest and friction model

We investigate the dynamics of a rigid body being dragged by a moving wall with constant speed $v_w$, as represented in Fig. 1. The rigid body has a mass m and is connected to the wall through a linear spring with stiffness k and a linear dashpot with damping coefficient c. The absolute displacement of the moving wall is denoted by $y(t)=v_wt$, while x(t) represents the absolute displacement of the mass (coordinate x is measured with respect to the position that the mass would occupy at $t=0$ if the spring was undeformed).

Fig. 1

Scheme of the mechanical system under study: a rigid body is dragged by a constant-speed moving wall through a spring-dashpot connection. The friction between the rigid body and the fixed ground is described by the Brown-McPhee model

The friction force between the rigid body and the fixed ground is modelled using the expression recently proposed by Brown and McPhee [48], which belongs to the general class of friction models known as friction-curve models or Stribeck models [51]. The friction force, for a given normal contact force, is expressed in these models as a function of the relative sliding speed between surfaces. The main drawback of this approach is that it does not take into account the influence of factors such as microslip, local temperature or wear debris [48]. The main benefit is that friction-curve models tend to be computationally efficient—which makes them very appropriate for multibody simulation—while being able to capture some key aspects of the friction phenomena, such as the Stribeck effect and viscous friction [48]. Among the available friction-curve models, the one proposed by Brown and McPhee has the advantage of being continuously differentiable in all its domain, which makes it both numerically efficient [52] and useful for analytical studies, as will be shown in this article.

According to the Brown-McPhee model, the relation between friction force, F, and relative speed between surfaces, v, is given by [48]

$$\begin{aligned} F(v) = F_d\tanh {\left( 4\frac{v}{v_t}\right) }+\left( F_s-F_d\right) \frac{\frac{v}{v_t}}{\left( \frac{1}{4}\left( \frac{v}{v_t}\right) ^2+\frac{3}{4}\right) ^2}\nonumber \\ \end{aligned}$$

(1)

where $F_d$ represents the dynamic friction force, $F_s$ is the static friction force and $v_t$ is the transition velocity. The physical meaning of the 3 dimensional parameters of the friction model ($F_d$, $F_s$ and $v_t$) is highlighted in Fig. 2a. We note that, although parameters $v_t$ and $F_s$ do not correspond with the exact maximum of the friction curve, they provide an approximation to the maximum that is sufficiently accurate for most practical purposes (see Fig. 2a), as discussed in Ref. [48].

It should also be mentioned that the expression proposed by Brown and McPhee includes an additional viscous term [48]. This has been omitted in Eq. (1) because it would not introduce any qualitative difference in the mechanical model of interest, which already incorporates the viscous damping of the dashpot (see Fig. 1).

Once the friction model has been chosen, the equation of motion of the system represented in Fig. 1 can be readily written as

$$\begin{aligned} m\ddot{x}+c(\dot{x}-\dot{y})+k(x-y)=-F(\dot{x}), \end{aligned}$$

(2)

where a dot represents differentiation with respect to time t. It is convenient to rewrite the equation using a new coordinate, $z=x-y$, which measures the position of the mass with respect to the moving wall:

$$\begin{aligned} m\ddot{z}+c\dot{z}+kz=-F(\dot{z}+v_w), \end{aligned}$$

(3)

where it has been used that $\ddot{y}=0$, since the wall moves at constant speed. In order to transform the equation of motion into dimensionless form, we define the following dimensionless parameters:

$$\begin{aligned} \begin{aligned} \beta&=\frac{c}{2m\omega _n}\\ \nu&=\frac{v_w}{v_t}\\ f_s&= \frac{F_s}{F_d}\\ u_t&= v_t\frac{k}{F_d\omega _n} \end{aligned} \end{aligned}$$

(4)

where $\omega _n=\sqrt{k/m}$. Consider also the following dimensionless versions of displacement z and time t:

$$\begin{aligned} \begin{aligned} s&=z\frac{\omega _n}{v_t}\\ \tau&=\omega _n t \end{aligned} \end{aligned}$$

(5)

By introducing Eqs. (4) and (5) into Eqs. (1) and (3), a dimensionless equation of motion is obtained:

$$\begin{aligned} & \ddot{s}+2\beta \dot{s}+s \nonumber \\ & \quad =-\frac{1}{u_t}\left( \tanh {\left( 4(\dot{s}+\nu )\right) }+(f_s-1)\frac{\dot{s}+\nu }{\left( \frac{\left( \dot{s}+\nu \right) ^2}{4}+\frac{3}{4}\right) ^2}\right) \nonumber \\ \end{aligned}$$

(6)

where a dot now denotes differentiation with respect to dimensionless time $\tau$ (this will be the case for the rest of the paper).

Fig. 2

Friction force versus relative sliding velocity according to the Brown-McPhee model. a Dimensional form of the friction law, given by Eq. (1). Parameters $F_s$, $F_d$ and $v_t$ represent, respectively, the static friction force, the dynamic friction force and the transition velocity. b Dimensionless form of the frition law, given by Eq. (7). Parameter $f_s$ represents the static-to-dynamic friction ratio

Equation (6) represents a two-dimensional nonlinear autonomous system with 4 parameters: the viscous damping ratio, $\beta$, the dimensionless wall speed, $\nu$, the dimensionless transition velocity for the friction model, $u_t$, and the static-to-dynamic friction ratio, $f_s$. The objective of this work is to analyse the bifurcations of this system, in order to understand how stable periodic oscillations are created and how they depend on the system parameters.

Before turning to the bifurcation analysis, it is useful to rewrite Eq. (6) in an abbreviated manner by defining

$$\begin{aligned} f(u)=\tanh {\left( 4u\right) }+(f_s-1)\frac{u}{\left( \frac{u^2}{4}+\frac{3}{4}\right) ^2} \end{aligned}$$

(7)

where f represents the dimensionless friction force, $F/F_d$, and u is a dimensionless sliding velocity, $v/v_t$. Observe that Eq. (7) is a dimensionless version of the Brown-Mc Phee original expression, given in Eq. (1) (compare Fig. 2a and b).

Combining Eqs. (6) and (7) yields

$$\begin{aligned} \ddot{s}+2\beta \dot{s}+s=-\frac{1}{u_t}f(\dot{s}+\nu ). \end{aligned}$$

(8)

3 Analytical study of stability and bifurcations

This section is organized in 3 subsections. Section 3.1 investigates the stability of the only fixed point in the system, finding that it can undergo a Hopf bifurcation. Section 3.2 studies the conditions under which this bifurcation is supercritical or subcritical. Finally, Sect.3.3 addresses the existence of a curve of saddle-node bifurcations (SN bifurcations) of limit cycles in the parameter space. A local approximation to this curve is analytically derived.

3.1 Fixed point, stability and Hopf bifurcation

We begin the analysis of the system given by Eqs. (7) and (8) by investigating its fixed points. In this case, it is straightforward to find that there is only one fixed point, given by

$$\begin{aligned} \dot{s}=\ddot{s}=0 \Rightarrow s=s_0=-\frac{f(\nu )}{u_t}. \end{aligned}$$

(9)

This corresponds to an equilibrium condition in which the mass moves at the same speed as the wall, with the friction force being balanced by the elastic force of the stretched spring. The stability of this equilibrium is investigated in the next paragraphs.

Using a new coordinate, $q=s-s_0$, which measures the dimensionless displacement with respect to the equilibrium position, Eq. (8) becomes

$$\begin{aligned} \ddot{q}+2\beta \dot{q}+q=-\frac{1}{u_t}g\left( \dot{q}\right) \end{aligned}$$

(10)

where function $g(\dot{q})$ is defined as

$$\begin{aligned} g(\dot{q})=f(\dot{q}+\nu )-f(\nu ). \end{aligned}$$

(11)

Eq. (10) can be written as a first-order system by defining a new variable $p=\dot{q}$:

$$\begin{aligned} \begin{aligned} \dot{q}&=p\\ \dot{p}&=-q-2\beta p-\frac{1}{u_t}g(p) \end{aligned} \end{aligned}$$

(12)

It is now convenient to separate the linear and nonlinear terms in Eq. (12) through a Taylor expansion of function g(p):

$$\begin{aligned} \begin{aligned} \dot{q}&=p\\ \dot{p}&=-q+\gamma p-\frac{H(p)}{u_t} \end{aligned} \end{aligned}$$

(13)

where $\gamma =-2\beta -f'(\nu )/u_t$ and H(p) is given by

$$\begin{aligned} H(p)=\sum _{k\ge 2}\frac{h_k}{k!}p^k, \quad \textrm{with}\;\; h_k=g^{(k)}(0)=f^{(k)}(\nu )\nonumber \\ \end{aligned}$$

(14)

The fixed point of interest, $s=s_0$, is given in the new coordinates by $(q,p)=(0,0)$. We can obtain the stability properties of this equilibrium through the eigenvalues of the jacobian matrix of system (12), evaluated at the fixed point:

$$\begin{aligned} \varvec{J}_\text {eq}= \begin{bmatrix} 0 & 1 \\ -1 & \gamma \end{bmatrix} \end{aligned}$$

(15)

It is straightforward to find that, as long as condition $|\gamma |<2$ holds, $\varvec{J}_\text {eq}$ has two complex conjugate eigenvalues, $(\lambda ,\bar{\lambda })$, where

$$\begin{aligned} \lambda =\mu + i \omega , \quad \textrm{with} \; \left\{ \begin{matrix} \mu =\frac{\gamma }{2}=-\beta -\frac{f'(\nu )}{2u_t} \\ \\ \omega =\sqrt{1-\frac{\gamma ^2}{4}}=\sqrt{1-\mu ^2} \end{matrix}\right\} \nonumber \\ \end{aligned}$$

(16)

Equation (16) shows that the pair of eigenvalues crosses the imaginary axis for $\gamma =0$, which means that the stability of the fixed point changes at $\gamma =0$ through a Hopf bifurcation:

$$\begin{aligned} \begin{aligned} \textrm{If}&\quad \beta<-\frac{f'(\nu )}{2u_t} \qquad \rightarrow \qquad \gamma>0 \qquad \\&\quad \rightarrow \qquad \text {Unstable Equilibrium}\\ \textrm{If}&\quad \beta >-\frac{f'(\nu )}{2u_t} \qquad \rightarrow \qquad \gamma <0 \qquad \\&\quad \rightarrow \qquad \text {Stable Equilibrium} \end{aligned} \end{aligned}$$

(17)

Whether this bifurcation is subcritical, supercritical or degenerate is investigated in Sect.3.2.

3.2 Characterization of the Hopf bifurcation

At the Hopf Bifurcation point (i.e. for $\gamma =0$), system (13) can be transformed into normal form as follows:

$$\begin{aligned} \begin{aligned} \dot{\tilde{r}}&=\alpha _1\tilde{r}^3+\alpha _2\tilde{r}^5+\mathcal {O}(\tilde{r}^7)\\ \dot{\tilde{\theta }}&=1+\mathcal {O}(\tilde{r}^2) \end{aligned}\nonumber \\ \end{aligned}$$

(18)

where $\tilde{r}$ and $\tilde{\theta }$ are polar coordinates (see [27, 53] for details). Coefficients $\alpha _1$ and $\alpha _2$ can in turn be computed as [54]

$$\begin{aligned} \begin{aligned}&\alpha _1=-\frac{h_3}{16u_t}=-\frac{f'''(\nu )}{16u_t}.\\&\alpha _2=-\frac{h_5}{384u_t}-\frac{13}{1152}\\&\cdot \frac{h_2^2h_3}{u_t^3}=-\frac{f^{\textrm{v}}(\nu )}{384u_t}-\frac{13}{1152}\cdot \frac{f''(\nu )^2f'''(\nu )}{u_t^3} \end{aligned} \end{aligned}$$

(19)

The sign of coefficient $\alpha _1$ determines whether the bifurcation is subcritical or supercritical [27, 53]:

$$\begin{aligned} \begin{aligned} \textrm{If}&\,\, f'''(\nu )<0 \quad \rightarrow \quad \alpha _1>0\quad \\&\rightarrow \quad \text {Subcritical Hopf Bifurcation}\\ \textrm{If}&\,\, f'''(\nu )>0 \quad \rightarrow \quad \alpha _1<0\quad \\&\rightarrow \quad \text {Supercritical Hopf Bifurcation} \end{aligned} \end{aligned}$$

(20)

It is convenient at this point to graphically represent the obtained Hopf bifurcation in parameter space. Recall that the system is characterized by 4 parameters: $\beta$, $\nu$, $f_s$ and $u_t$. A useful representation is obtained by considering the plane spanned by the dimensionless wall speed, $\nu$, and the damping ratio, $\beta$, for some fixed values of parameters $(f_s,u_t)$. The Hopf bifurcation given by condition $\beta =-f'(\nu )/(2u_t)$ provides a curve in the $(\nu ,\beta )$ plane such as represented in Fig. 3. Note that, with the Brown-McPhee model, all derivatives of the friction force with respect to the sliding velocity can be analytically obtained. The expressions for the first five derivatives are provided in Appendix A.

Fig. 3

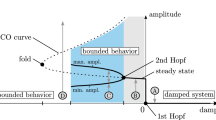
Two-parameter bifurcation diagram. The Hopf bifurcation curve given by condition $\beta =-f'(\nu )/(2u_t)$ separates the parameter plane into two regions, depending on whether the fixed point is stable or unstable. The inflexion point of the bifurcation curve, $P_3$, corresponds to the degenerate case in which $f'''(\nu )=0$ and, therefore, $\alpha _1=0$. Fixed points and limit cycles have been sketched at both sides of the bifurcation curve: a solid (open) dot represents a stable (unstable) fixed point, while a solid (dotted) ellipse represents a stable (unstable) limit cycle

As expressed in Eq. (20), the characterization of the Hopf bifurcation as subcritical or supercritical depends on the sign of $f'''(\nu )$. This means that it depends on the curvature of the Hopf bifurcation curve represented in Fig. 3 and given by relation $\beta =-f'(\nu )/(2u_t)$: the bifurcation is supercritical (subcritical) where the bifurcation curve is concave (convex). These two portions of the curve are connected at the inflexion point where $f'''(\nu )=0$, which is denoted as $P_3$ ($\nu =\nu _3$, $\beta =\beta _3$) in Fig. 3. At this point, the Hopf bifurcation is neither supercritical nor subcritical, but degenerate.

Figure 3 also includes a schematic representation of the fixed points and limit cycles of the system around the bifurcation curve. Where the bifurcation is supercritical, we expect to have a stable fixed point above the curve and a unstable fixed point, surrounded by a stable limit cycle, below the curve. Likewise, where the bifurcation is subcritical, we expect to have a stable fixed point surrounded by an unstable limit cycle above the curve and an unstable fixed point below the curve (see Fig. 3). Note that Fig. 3 includes additional stable limit cycles above and below the subcritical Hopf bifurcation curve, which is not predicted by the Hopf bifurcation analysis. The reason is that, from physical intuition on this particular mechanical system, we expect some bounded attractor to exist around any unstable limit cycle or unstable fixed point, because otherwise there would be trajectories escaping to infinity. The existence of these additional stable limit cycles will be confirmed by numerical simulations in Sect. 4.

The scenario of fixed points and periodic orbits depicted in Fig. 3 suggests the existence of a curve of saddle-node bifurcations of limit cycles, stemming from point $P_3$, which creates the stable-unstable limit cycle pair above the subcritical Hopf curve. This new bifurcation curve is analytically investigated in Sect.3.3.

3.3 Saddle-node bifurcation of limit cycles

Let us conduct a local analysis of periodic orbits in the vicinity of point $P_3$ (see Fig. 3). This requires transforming system (13) into normal form. However, the normal form given in Eq. (18) is not suitable for this purpose, since it is only valid at the Hopf bifurcation point, while now we are interested in analysing a vicinity of the Hopf bifurcation in parameter space. Hence, a more general normal form needs to be used. Our derivation of this normal form, which will be presented in this subsection, is mainly based on Chapters 3 and 8 of Ref. [53].

The first step in the required transformation is the change of coordinates

$$\begin{aligned} \begin{aligned}&q=-\omega \tilde{x}+\mu \tilde{y}\\&p=\tilde{y}, \end{aligned} \end{aligned}$$

(21)

which transforms system (13) into

(22)

Note that, thanks to the applied coordinate change, the jacobian matrix of the system now appears in real modal form [55, 56]. The second step consists in rewriting system (22) using a complex variable:

$$\begin{aligned} z=\tilde{x}+i\tilde{y} \end{aligned}$$

(23)

Taking the time-derivative of Eq. (23) and introducing Eq. (22) yields

$$\begin{aligned} \dot{z}=\lambda z +G(z,\bar{z}) \end{aligned}$$

(24)

where $\lambda =\mu +i\omega$ (see Eq. (16)), $\bar{z}$ is the complex conjugate of z and function $G(z,\bar{z})$ is given by

$$\begin{aligned} G(z,\bar{z})=-\frac{\lambda }{\omega u_t}H\left( \frac{z-\bar{z}}{2i}\right) \end{aligned}$$

(25)

It is useful to write function $G(z,\bar{z})$ in Taylor series form:

$$\begin{aligned} G(z,\bar{z})=\sum _{k+l\ge 2}\frac{g_{kl}}{k!l!}z^k\bar{z}^l \end{aligned}$$

(26)

The coefficients $g_{kl}$ can be obtained by equating expressions (25) and (26) and expanding function H as in Eq. (14). This yields the following result (only the coefficients that will be needed for the normal form are provided):

$$\begin{aligned} \begin{aligned}&g_{20}=\frac{\lambda }{4\omega u_t}h_2\\&g_{02}=\frac{\lambda }{4\omega u_t}h_2\\&g_{11}=-\frac{\lambda }{4\omega u_t}h_2\\&g_{21}=i\frac{\lambda }{8\omega u_t}h_3 \end{aligned} \end{aligned}$$

(27)

The final steps in the derivation of the normal form are a quasi-identity transformation and a transformation to polar coordinates. This procedure, whose details can be found in Ref. [53], allows writing system (24) as

$$\begin{aligned} & \dot{r}=\mu (\varvec{\delta }) r+\textrm{Re}\left( c_1(\varvec{\delta })\right) r^3+\textrm{Re}\left( c_2(\varvec{\delta })\right) r^5+\mathcal {O}(r^7)\nonumber \\ & \dot{\theta }=\omega (\varvec{\delta })+\mathcal {O}(r^2) \end{aligned}$$

(28)

where r and $\theta$ are polar coordinates and $\varvec{\delta }$ is a vector that contains the varying parameters. Recall that, from the 4 parameters of the system under study, $(f_s,u_t,\beta ,\nu )$, we consider $(f_s,u_t)$ to be fixed for the bifurcation analysis, while $(\beta ,\nu )$ can vary (see the two-parameter bifurcation diagram in Fig. 3). Hence, vector $\varvec{\delta }$ should in principle include parameters $(\beta ,\nu )$. It is, however, more convenient to define

$$\begin{aligned} \varvec{\delta }= \begin{bmatrix} \mu&\tilde{\nu } \end{bmatrix}, \end{aligned}$$

(29)

with $\tilde{\nu }=\nu -\nu _3$. Note that there is a direct correspondence between the pairs of parameters $(\beta ,\nu )$ and $(\mu ,\tilde{\nu })$, since $\mu =-\beta -f'(\nu )/(2u_t)$ (see Eq. (16)). Note also that $\varvec{\delta }=\varvec{0}$ at point $P_3$ (see Fig. 3).

Complex coefficient $c_1$ in Eq. (28) is given by the following expression (see Ref. [53])

$$\begin{aligned} c_1=\frac{g_{21}}{2}+\frac{g_{20}g_{11}(2\lambda +\bar{\lambda })}{2\vert \lambda \vert ^2}+\frac{\vert g_{11}\vert ^2}{\lambda }+\frac{\vert g_{02}\vert ^2}{2(2\lambda -\bar{\lambda })}\nonumber \\ \end{aligned}$$

(30)

where dependence on $\varvec{\delta }$ has been omitted for convenience.

By introducing expressions (27) into Eq. (30), and taking the real part, the following result is obtained:

$$\begin{aligned} \textrm{Re}\left( c_1\right)= & -\frac{f'''(\nu )}{16u_t}+\frac{\mu }{32u_t^2\omega ^2}f''(\nu )^2 \nonumber \\ & \quad \left( 7-8\mu ^2+\frac{1}{9-8\mu ^2}\right) \end{aligned}$$

(31)

Since we are interested in performing a local analysis in the vicinity of point $P_3$, where $\varvec{\delta }=\varvec{0}$, it is convenient to expand Eq. (31) in powers of $(\mu ,\tilde{\nu })$:

$$\begin{aligned} \textrm{Re}\left( c_1\right) =-\frac{f^{\textrm{iv}}(\nu _3)}{16u_t}\tilde{\nu }+\frac{2}{9u_t^2}f''(\nu _3)^2\mu +\mathcal {O}(\Vert \varvec{\delta }\Vert ^2).\nonumber \\ \end{aligned}$$

(32)

The general expression for coefficient $c_2$ in Eq. (28) is rather complex and can be found in Ref. [53]. Fortunately, as will be seen later on, it is sufficient for the desired level of approximation to expand $\textrm{Re}\left( c_2(\varvec{\delta })\right)$ in powers of $(\mu ,\tilde{\nu })$ and retain only the constant term:

$$\begin{aligned} \textrm{Re}\left( c_2(\varvec{\delta })\right) =\textrm{Re}\left( c_2(\varvec{0})\right) +\mathcal {O}(\Vert \varvec{\delta }\Vert ). \end{aligned}$$

(33)

Notice that $\varvec{\delta }=\varvec{0}$ corresponds to the Hopf bifurcation condition. This means that, for $\varvec{\delta }=\varvec{0}$, the normal form given in Eq. (28) has to be the same as the one given in Eq. (18), which implies

$$\begin{aligned} \textrm{Re}\left( c_2(\varvec{0})\right) =\alpha _2(\nu _3)=-\frac{f^{\textrm{v}}(\nu _3)}{384u_t} \end{aligned}$$

(34)

Thus, we can write

$$\begin{aligned} \textrm{Re}\left( c_2\right) =-\frac{f^{\textrm{v}}(\nu _3)}{384u_t}+\mathcal {O}(\Vert \varvec{\delta }\Vert ). \end{aligned}$$

(35)

Now we can use the normal form given in Eq. (28) to find small limit cycles ($r\approx 0$) in the vicinity of point $P_3$ ($\varvec{\delta }\approx \varvec{0}$). These limit cycles correspond to the solutions $r>0$ that make $\dot{r}=0$ in Eq. (28):

$$\begin{aligned} \mu (\varvec{\delta }) +\textrm{Re}\left( c_1(\varvec{\delta })\right) r^2+\textrm{Re}\left( c_2(\varvec{\delta })\right) r^4+\mathcal {O}(r^6)=0\nonumber \\ \end{aligned}$$

(36)

Let us now look for solutions of Eq. (36) with the following scaling:

$$\begin{aligned} \begin{aligned} r&=\varepsilon r_0+\mathcal {O}(\varepsilon ^2)\\ \tilde{\nu }&=\varepsilon ^2 \tilde{\nu }_0+\mathcal {O}(\varepsilon ^3)\\ \mu&=\varepsilon ^4\mu _0+\mathcal {O}(\varepsilon ^5) \end{aligned} \end{aligned}$$

(37)

where $\varepsilon$ is a sufficiently small positive parameter ($0<\varepsilon \ll 1$). If Eq. (37) is introduced into Eq. (36), using relations (32) and (35), the following result is obtained for the lowest order terms:

$$\begin{aligned} \mu _0-\frac{f^{\textrm{iv}}(\nu _3)}{16u_t}\tilde{\nu }_0r_0^2-\frac{f^{\textrm{v}}(\nu _3)}{384u_t}r_0^4=0. \end{aligned}$$

(38)

Note that solving Eq. (38) amounts to finding the roots of a second-order polynomial in $r_0^2$. The folding of the limit cycles (SN bifurcation of limit cycles) occurs when this polynomial has a double root, hence when the discriminant is zero:

$$\begin{aligned} \left( \frac{f^{\textrm{iv}}(\nu _3)}{16u_t}\tilde{\nu }_0\right) ^2+4\mu _0\frac{f^{\textrm{v}}(\nu _3)}{384u_t}=0 \end{aligned}$$

(39)

From Eq. (39), the follwing condition for the SN bifurcation of limit cycles is obtained:

$$\begin{aligned} \mu _0=-\frac{3}{8u_t}\frac{f^{\textrm{iv}}(\nu _3)^2}{f^{\textrm{v}}(\nu _3)}\tilde{\nu }_0^2 \end{aligned}$$

(40)

On the other hand, the double root of the polynomial in Eq. (38) is given by

$$\begin{aligned} r_0^2=-12\frac{f^{\textrm{iv}}(\nu _3)}{f^{\textrm{v}}(\nu _3)}\tilde{\nu }_0 \end{aligned}$$

(41)

Since $r_0^2$ must be positive, and taking into account that $f^{\textrm{iv}}(\nu _3)<0$, $f^{\textrm{v}}(\nu _3)>0$ (see Appendix B), it is clear that the obtained solution can only exist if $\tilde{\nu }_0>0$ (i.e. for $\nu >\nu _3$).

The last remaining step consists in transforming relation (40) back into the original parameters $(\beta ,\nu )$ by using Eqs. (16) and(37), which yields

$$\begin{aligned} & \beta ^{sn}=-\frac{f'(\nu _3)}{2u_t}-\frac{f''(\nu _3)}{2u_t}(\nu -\nu _3)\nonumber \\ & +\frac{3}{8u_t}\frac{f^{\textrm{iv}}(\nu _3)^2}{f^{\textrm{v}}(\nu _3)}(\nu -\nu _3)^2+\mathcal {O}((\nu -\nu _3)^3) \end{aligned}$$

(42)

where superscript sn is used to indicate that the equation corresponds to the SN bifurcation condition.

By discarding the higher order terms in Eq. (42), we obtain

$$\begin{aligned} & \beta ^{sn}_2=-\frac{f'(\nu _3)}{2u_t}-\frac{f''(\nu _3)}{2u_t}(\nu -\nu _3)\nonumber \\ & +\frac{3}{8u_t}\frac{f^{\textrm{iv}}(\nu _3)^2}{f^{\textrm{v}}(\nu _3)}(\nu -\nu _3)^2 \end{aligned}$$

(43)

where subscript 2 indicates that the equation provides a second-order local approximation to the actual SN bifurcation curve.

Fig. 4

Two-parameter bifurcation diagram including the Hopf bifurcation curve, given by condition $\beta =-f'(\nu )/(2u_t)$, and the analytical approximation to the SN bifurcation curve, given by Eq. (43). The parameter plane gets divided into three regions, depending on the number and type of attractors in the system. Fixed points and limit cycles are represented as follows: a solid (open) dot represents a stable (unstable) fixed point, while a solid (dotted) ellipse represents a stable (unstable) limit cycle

Now the two-parameter bifurcation diagram of Fig. 3 can be completed by adding the curve given in Eq. (43), as displayed in Fig. 4. This graph shows how the bifurcation curves divide the $(\nu ,\beta )$ parameter plane into three regions, depending on the number and type of system attractors. Below the Hopf bifurcation curve, the equilibrium is unstable and all trajectories tend towards a stable limit cycle. The region above the Hopf bifurcation curve, where the equilibrium is stable, is in turn split into two areas by the SN bifurcation curve, which defines the appearance of a stable-unstable limit cycle pair.

Although Fig. 4 seems to provide a full picture of the system bifurcations and stability, it is important to recall that the depicted SN bifurcation curve only constitutes a second-order Taylor approximation around point $P_3$ of the exact curve. In order to overcome this limitation, numerical continuation techniques will be used in Sect. 4 to obtain a SN bifurcation curve that is accurate well beyond point $P_3$. This will also serve as a confirmation of the local validity of Eq. (43).

4 Numerical results and discussion

This section comprises two subsections. Section 4.1 investigates the bifurcation behaviour of the system of interest through a detailed exploration of the 4D parameter space. Numerical continuation is used for the SN bifurcation curve, in order to overcome the limitation (local validity) of the analytical approximation obtained in Sect. 3.3. For a better understanding of the system dynamics in different scenarios, Sect.4.2 presents and discusses some illustrative phase portraits and time histories obtained through numerical integration of the equation of motion.

4.1 Bifurcation diagrams using numerical continuation

The analysis presented in Sect. 3 has provided the analytical expressions for a Hopf bifurcation curve (see Eq. (17)) and a curve of SN bifurcations of limit cycles (see Eq. (43)), which are jointly represented in Fig. 4. This bifurcation analysis has been conducted by varying parameters $(\nu ,\beta )$, while considering $(f_s,u_t)$ to be fixed, which means that the obtained bifurcation curves will depend on the chosen values of $(f_s,u_t)$.

For a thorough exploration of the 4D parameter space, the bifurcation curves will now be represented for different pairs of values $(f_s,u_t)$. The static-to-dynamic friction ratio, $f_s$, varies substantially depending on the materials in contact, surface roughness, lubrication and other factors. According to the consulted literature, $f_s$ tends to vary between 1 and 3 in most applications [57,58,59,60,61], although higher values can also be found in some cases (for example, Joven et al. report $f_s\approx 5.3$ for a contact between aluminum and a carbon fiber composite [62], while Papangelo et al. report $f_s\approx 7.3$ for cast iron-to-cast iron contact [63]). In order to cover a sufficiently wide range of variation for the friction ratio, the following values have been chosen: $f_s=1.5,\;3,\;8$. For the dimensionless transition speed, the values $u_t=0.001$, 0.01, 0.1, 1, 10 have been considered (the reason for this specific choice, as will be seen, is that such range of values for $u_t$ seems to cover the different bifurcation scenarios that the system can exhibit). This yields a total of 15 combinations of parameters $(f_s,u_t)$, for which the bifurcation curves have been graphed in Fig. 5.

Fig. 5

Two-parameter bifurcation diagrams obtained for different combinations of parameters ($f_s$, $u_t$). In each of the graphs, the solid black line represents the Hopf bifurcation curve, the solid red line is the analytical local approximation to the SN bifurcation curve and the dashed black line is the SN bifurcation curve obtained by numerical continuation. A white dot represents the inflexion point of the Hopf bifurcation curve, $P_3$. Each graph is divided into regions of different colors, depending on the number and type of attractors in the system: Green area: the only attractor is a stable fixed point. White area: the only attractor is a stable limit cycle. Grey area: bistable region (a stable fixed point coexists with a stable limit cycle)

Fig. 6

Comparison between friction models. a Brown-McPhee model (three curves are plotted for decreasing values of the transition speed, $v_t$). b Coulomb’s model with stiction. Both graphs represent friction force, F, against sliding speed, v. It is shown that the Brown-McPhee model approaches Coulomb’s model as the transition speed tends to zero

Fig. 7

Numerical solutions of system (8) for different initial conditions, represented on the phase plane. Each initial condition is marked with an ‘x’. Stable and unstable limit cycles are shown as solid and dashed red lines, respectively. The grey area represents the sticking region of the phase plane, given by condition $-1<\dot{s}+\nu <1$. a $f_s=3,\;u_t=0.1,\;\nu =5,\;\beta =0.9$ b $f_s=3,\;u_t=0.1,\;\nu =5,\;\beta =0.7$ c $f_s=3,\;u_t=0.1,\;\nu =5,\;\beta =0.5$

As was stressed in Sect. 3.3, the analytical expression given in Eq. (43) for the SN bifurcation curve (represented in red in Fig. 5) is only valid locally, around point $P_3$. Thus, in order to complete the global picture of bifurcations, numerical continuation has been used for the SN curve, using the software XPPAUT. The result of this numerical continuation procedure is represented in Fig. 5 as a dashed black line on each of the graphs. Note the good accordance between the analytical and numerical SN curves in the vicinity of point $P_3$, which is marked with a white dot.

Using the representation shown in Fig. 5, the mechanisms whereby periodic orbits are created in the system can be described as follows. Assume that parameters $(f_s,u_t,\nu )$ are fixed and that the damping ratio $\beta$ decreases from some large initial value. There are two possible scenarios:

• Supercritical case ($\nu <\nu _3$). Initially, the fixed point of the system is stable and all trajectories are attracted towards it. If $\beta$ decreases up to the Hopf bifurcation point, the fixed point loses its stability and a small stable limit cycle is generated around it.

• Subcritical case ($\nu >\nu _3$). Like in the previous case, the stable fixed point initially attracts all trajectories. If $\beta$ reduces until reaching the SN bifurcation point, a stable-unstable limit cycle pair is generated around the fixed point. As $\beta$ decreases further, the unstable limit cycle shrinks until it disappears at the Hopf bifurcation point, rendering the equilibrum unstable. For lower values of $\beta$, all trajectories tend towards the only remaining attractor in the system, which is the stable limit cycle.

According to the above description, the Hopf bifurcation and SN bifurcation curves divide the parameter plane into three distinct regions, marked with different colors in Fig. 5, depending on the number and type of attractors in the system. In the green area, all trajectories tend towards a stable fixed point. Conversely, in the white region, this fixed point is unstable and all trajectories are attracted towards a stable limit cycle. The grey area is the bistable region of the parameter plane, where stable fixed point coexists with a stable limit cycle.

Note that, in agreement with physical intuition, the system equilibrium is stable and attracts all trajectories when viscous damping is the predominant factor (large values of $\beta$). As damping decreases, more complex dynamic phenomena can be found.

The collection of ordered graphs in Fig. 5 can be used to assess the effect of parameters ($f_s$,$u_t$) on the bifurcation behavior of the system. Let us examine the influence of the transition speed, $u_t$, by comparing the graphs within the same column in Fig. 5. While the shape of the Hopf bifurcation curve is not observed to change with $u_t$ (apart from a rescaling of the vertical axis), the shape of the SN curve does show a dependence on this parameter (of course, we focus now on the numerical version of the SN curve, since the analytically obtained curve has only local validity). It is found that, as $u_t\downarrow 0$, the SN curve becomes indistinguishable from the Hopf curve, which makes the bistable region of the parameter plane (grey area in Fig. 5) vanishingly small. Conversely, for sufficiently large values of $u_t$ (see the last two rows of graphs in Fig. 5), the SN curve adopts a fixed shape above the Hopf curve, as does the bistable region of the parameter plane. For intermediate values of parameter $u_t$, a smooth transition between these two kinds of behaviour is observed (see the graphs corresponding to $u_t=0.01$, 0.1). Notice that the range of values of $u_t$ for which this transition occurs depends on the specific value of the friction ratio, $f_s$.

Since a strong dependence has been found between the bifurcation behaviour of the system and the order of magnitude of $u_t$, a brief discussion on the physical meaning of this parameter is appropriate. Recall from Eq. (4) that $u_t$ is defined as a dimensionless version of the transition speed of the friction model, $v_t$: $u_t=v_tk/(F_d\omega _n)$. Hence, a reduction in $u_t$ can be understood as a reduction in $v_t$, while keeping $(k,F_d,\omega _n)$ constant. It is interesting to see that decreasing parameter $v_t$ can in turn be interpreted as making the Brown-McPhee model approach the classical Coulomb model with stiction, as shown in Fig. 6. This means that the effect of $u_t$ in the system bifurcations and stability may be stated as follows: For sufficiently large values of $u_t$, the shape of the two-parameter bifurcation diagram in the $(\nu ,\beta )$ plane is fixed, including a bistable region. As $u_t$ decreases, i.e. as the Brown-McPhee model approaches Coulomb’s model, a transition is found towards a new scenario where the bistable region is vanishingly small.

4.2 Numerical integration of the equation of motion

In order illustrate the three possible scenarios discussed in Sect. 4.1 (green, grey and white regions in Fig. 5), it is useful to make some representation of the system trajectories on the phase plane. As an example, we consider the specific case $f_s=3$, $u_t=0.1$, $\nu =5$, with three different values for the damping ratio: $\beta =0.9,\;0.7,\;0.5$. It can be observed in Fig. 5 that these three combinations of parameters correspond to the green, grey and white regions of the parameter plane, respectively. For each of these three cases, system (8) has been numerically solved with different initial conditions, and the obtained trajectories are represented in Fig. 7.

Figure 7a shows that, for $\beta =0.9$, the fixed point is a stable focus that attracts the whole system dynamics, while no limit cycles exist. On the other hand, it is observed in Fig. 7c that, for $\beta =0.5$, the equilibrium is an unstable focus and all trajectories tend towards a stable limit cycle. For an intermediate damping value $(\beta =0.7)$, Fig. 7b exhibits the coexistence of a stable focus and a stable limit cycle. Note also the existence of an unstable limit cycle, which acts as a separatrix between the two system attractors. For the scenario displayed in Fig. 7b, variable $\dot{s}$ (dimensionless speed of the mass relative to the moving wall) has also been plotted against time in Fig. 8 for two sets of initial conditions, showing how the system can evolve towards an equilibrium point or a stick-slip periodic oscillation, depending on its initial state.

Fig. 8

Numerical solutions (velocity vs time) of system (8) with parameters $f_s=3$, $u_t=0.1$, $\nu =5$, $\beta =0.7$, for two different sets of initial conditions. The graph shows how the system evolves towards an equilibrium point or a stick-slip periodic oscillation depending on the initial state

Fig. 9

Phase portrait of the system represented by Eq. (8) for parameters $f_s=3$, $u_t=0.1$, $\nu =5$, $\beta =0.7$. The direction and speed of the flow is represented with arrows. Trajectories starting from different initial conditions, obtained by numerical integration of Eq. (8), have been plotted in black. The straight and curved red lines are the nullclines of system (8), corresponding, respectively, to conditions $\dot{s}=0$ and $\ddot{s}=0$

It is interesting to see how trajectories in Fig. 7 tend to flatten in the area that has been coloured in grey, which represents the region of the phase plane where there is sticking between the mass and the ground (as shown in Fig. 2, the sticking behaviour is modelled by the Brown-McPhee equation as a slow creep between the surfaces, given by condition $-v_t<v<v_t$ or, equivalently, by inequality $-1<\dot{s}+\nu <1$). For a better understanding of the system behaviour within this sticking band, the phase portrait in Fig. 7b (bistable scenario) has been replotted with additional information in Fig. 9. In this graph, the direction and speed of the flow is represented with arrows (each arrow is a scaled version of vector $\begin{bmatrix} \dot{s}&\ddot{s} \end{bmatrix}$, evaluated at the origin of the arrow). In addition, the nullclines of the system, given by conditions $\dot{s}=0$ and $\ddot{s}=0$, are represented as straight and curved red lines, respectively. Interestingly, the flattening of trajectories in the sticking region is observed to be associated with an evolution of the system along the nearly horizontal portion of the nullcline $\ddot{s}=0$. In fact, trajectories around this part of the nullcline seem to display the typical behaviour of a fast-slow system around a slow manifold [64,65,66]. An asymptotic analysis of this type of fast-slow dynamics in the system under study will be conducted in the future.

Fig. 10

Representation of the first five derivatives of the Brown-McPhee equation for $f_s=1.5$

Fig. 11

Fourth and fifth Brown-Mc Phee derivatives evaluated at $\nu _3$ (point of degeneracy of the Hopf bifurcation)

Finally, it is worth making a brief remark on the generality of our results. Although the analysis presented in this article is specifically based on the Brown-McPhee model [48], it is reasonable to expect that similar results would be obtained with other friction models, as long as the F(v) graph (friction force as a function of the sliding speed) was qualitatively similar to that shown in Fig. 2. The reason is that, independently of the particular mathematical expression for F(v), a smooth curve such as shown in Fig. 2 will always have a first derivative $F'(v)$ with the qualitative shape displayed as $f'$ in Fig. 10 from Appendix A. This in turn means that the first derivative curve will exhibit an inflexion point, acting as a separation between a supercritical Hopf bifurcation and a subcritical one.

5 Summary and conclusions

This paper uses a 1-degree-of-freedom model to investigate how the transition between static and dynamic friction regimes can give rise to periodic oscillations in mechanical systems. The model consists in a mass that is dragged by a constant-speed moving wall through a spring-dashpot connection. The friction force between the mass and the ground is modelled using an expression recently proposed by Brown and McPhee, which is particularly suited for an analytical bifurcation study due to its continuity and differentiability properties.

It was found that stable periodic oscillations can be generated in this system through either a supercritical Hopf bifurcation or a SN bifurcation of limit cycles. Using stability analysis, transformation to normal form and numerical continuation techniques, both bifurcation curves were obtained. These curves divide the parameter plane into three distinct regions: two of them are monostable (the system has only one attractor), while the other one is bistable (the system tends towards an equilibrium or a periodic oscillation depending on its initial state).

Four dimensionless parameters characterize the system of interest: damping ratio, dimensionless wall speed, static-to-dynamic friction ratio and dimensionless transition speed between static and dynamic friction. The 4D parameter space was explored in detail, showing how each of the parameters affects the bifurcation behaviour of the system. Particularly interesting is the fact that two qualitatively different bifurcation configurations were found depending on the order of magnitude of the transition speed. For sufficiently large values of this parameter, the shape of the bifurcation diagram, with two monostable regions and one bistable region, is fixed. However, as the transition speed decreases (i.e. as the Brown-McPhee model approaches Coulomb’s model), a transition is found towards a new scenario where the SN bifurcation curve almost overlaps with the Hopf bifurcation curve, making the bistable region of the parameter plane practically disappear.

Appendices

Appendix A: Derivatives of the Brown-McPhee equation

The analytical expressions of the first five derivatives of the dimensionless Brown-McPhee function, f(u), given in Eq. (7), are provided below.

$$\begin{aligned} & f'(u)=\dfrac{48(1-u^2)(f_s-1)}{\left( 3+u^2\right) ^3}+4{{\,\textrm{sech}\,}}{\left( 4u\right) }^2 \end{aligned}$$

(A.1)

$$\begin{aligned} & f''(u)=32\left( \dfrac{6u(f_s-1)(u^2-3)}{\left( u^2+3\right) ^4}-{{\,\textrm{sech}\,}}{\left( 4u\right) }^2\tanh {\left( 4u\right) }\right) \end{aligned}$$

(A.2)

$$\begin{aligned} & \begin{aligned}&f'''(u)=\dfrac{-192(f_s-1)\left( 5u^4-30u^2+9\right) }{\left( u^2+3\right) ^5}\\&\qquad +128{{\,\textrm{sech}\,}}{\left( 4u\right) }^4\left( \cosh {\left( 8u\right) }-2\right) \end{aligned} \end{aligned}$$

(A.3)

$$\begin{aligned} & \begin{aligned}&f^{\textrm{iv}}(u)=\dfrac{5760u\left( f_s-1\right) \left( u^4-10u^2+9\right) }{\left( u^2+3\right) ^6}\\&\qquad -1024{{\,\textrm{sech}\,}}{\left( 4u\right) }^4\tanh {\left( 4u\right) }\left( \cosh {\left( 8u\right) }-5\right) \end{aligned} \end{aligned}$$

(A.4)

$$\begin{aligned} & \begin{aligned}&f^{\textrm{v}}(u)=\dfrac{5760\left( f_s-1\right) \left( -7u^6+105u^4-189u^2+27\right) }{\left( u^2+3\right) ^7}\\&\qquad +2048{{\,\textrm{sech}\,}}{\left( 4u\right) }^6\left( 33-26\cosh {\left( 8u\right) }+\cosh {\left( 16u\right) }\right) \end{aligned} \end{aligned}$$

(A.5)

Appendix B: On the signs of $f^{\textrm{iv}}(\nu _3)$ and $f^{\textrm{v}}(\nu _3)$

This appendix is intended to justify the assumption $f^{\textrm{iv}}(\nu _3)<0$, $f^{\textrm{v}}(\nu _3)>0$, which was used for the analytical derivation of the SN bifurcation curve (see Eq. (41) and the text below it).

It should be recalled that the dimensionless Brown-McPhee function, given in Eq. (7), and its derivatives, given in Appendix A, depend on the dimensionless sliding speed, u, and on the static-to-dynamic friction ratio, $f_s$. This double dependence will be made explicit here by writing $f(u,f_s)$, $f'(u,f_s)$,..., $f^{\textrm{v}}(u,f_s)$.

Since parameter $\nu _3$ is defined by condition $f'''(\nu _3,f_s)=0$, we can find $\nu _3=\nu _3(f_s)$ by solving $f'''=0$ in Eq. (A.3). By introducing this result into Eqs. (A.4) and (A.5), we obtain $f^{\textrm{iv}}(\nu _3(f_s),f_s)$ and $f^{\textrm{v}}(\nu _3(f_s),f_s)$, which are represented in Fig. 11. This graph shows that, for $1<f_s<8$, conditions $f^{\textrm{iv}}(\nu _3(f_s),f_s)<0$ and $f^{\textrm{v}}(\nu _3(f_s),f_s)>0$ are met (the reason for choosing this specific range of values for parameter $f_s$ is discussed in Sect. 4.1).