A partially debonded circular inhomogeneity in nonlinear thermoelectricity

Abstract

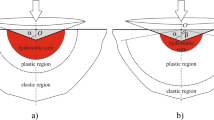
We study the two-dimensional thermoelectric problem associated with a circular inhomogeneity partially bonded to an infinite matrix subjected to uniform remote electric current density and energy flux. Both the inhomogeneity and the matrix are composed of nonlinearly coupled thermoelectric materials. The four analytic functions characterizing the thermoelectric fields in the two-phase composite are derived rigorously, in closed-form, by solving two Riemann–Hilbert problems with discontinuous coefficients. We obtain elementary expressions for the normal electric current density and normal energy flux along the bonded portion of the circular interface as well as the thermoelectric potential and temperature jumps across the remaining debonded section.

Appendix: detailed derivations of Eqs. (8) and (20)

Let n be the unit outward normal to L. We have

$$\begin{aligned} n_{1} =\frac{dx_{2} }{ds},{\, \, }n_{2} =-\frac{dx_{1} }{ds},{\, \, }n_{3} =0. \end{aligned}$$

(A1)

Using Eqs. (5), (6) and (A1), differentiating $\Phi$ and $\Theta$ along the boundary L, we obtain

$$\begin{aligned} \frac{d\Phi }{ds}= & {} \Phi _{,1} \frac{dx_{1} }{ds}+\Phi _{,2} \frac{dx_{2} }{ds}=-J_{1} n_{1} -J_{2} n_{2} =-J_{j} n_{j} =-J_{n} , \nonumber \\ \frac{d\Theta }{ds}= & {} \Theta _{,1} \frac{dx_{1} }{ds}+\Theta _{,2} \frac{dx_{2} }{ds}=-J_{u1} n_{1} -J_{u2} n_{2} =-J_{uj} n_{j} =-J_{un} . \end{aligned}$$

(A2)

This proves Eq. (8).

The solution to the the Riemann–Hilbert problem in Eqs. (17) and (19) can be written in the form

(A3)

This proves Eq. (20).