Abstract
In this paper, we study the existence of positive solutions for the nonlinear fractional boundary value problem with a p-Laplacian operator
where , , , are the standard Riemann-Liouville fractional derivatives, , , , , and . By the properties of Green’s function, the Guo-Krasnosel’skii fixed-point theorem, the Leggett-Williams fixed-point theorem, and the upper and lower solutions method, some new results on the existence of positive solutions are obtained. As applications, examples are presented to illustrate the main results.
MSC:34A08, 34B18, 35J05.
Similar content being viewed by others
1 Introduction
Recently, fractional differential equations have been of great interest. The motivation for those works stems from both the intensive development of the theory of fractional calculus itself and the applications such as economics, engineering and other fields [1–7]. Many people pay attention to the existence and multiplicity of solutions or positive solutions for boundary value problems of nonlinear fractional differential equations by means of some fixed-point theorems [8–24] (such as the Schauder fixed-point theorem, the Guo-Krasnosel’skii fixed-point theorem, the Leggett-Williams fixed-point theorem) and the upper and lower solutions method [25–27].
To the best of our knowledge, there are few papers devoted to the study of fractional differential equations with a p-Laplacian operator [22–24, 26–29]. Its theories and applications seem to be just being initiated.
Wang et al.[26] considered the following p-Laplacian fractional differential equations boundary value problems:
where , , , and is the standard Riemann-Liouville fractional derivative. , , , . They obtained the existence of at least one positive solution by means of the upper and lower solutions method.
Wang et al.[24] investigated the existence and multiplicity of concave positive solutions of a boundary value problem of a fractional differential equation with a p-Laplacian operator as follows:
where , , , is the Caputo derivative. By using a fixed-point theorem, some results for multiplicity of concave positive solutions are obtained.
Chen et al.[28] considered the boundary value problem for a fractional differential equation with a p-Laplacian operator at resonance
where , , and is the Caputo fractional derivative. By using the coincidence degree theory, a new result on the existence of solutions is obtained.
Guoqing Chai [29] investigated the existence and multiplicity of positive solutions for a class of boundary value problems of fractional differential equations with a p-Laplacian operator
where , , , , σ is a positive constant number, , , are the standard Riemann-Liouville derivatives. By means of the fixed-point theorem on cones, some existence and multiplicity results of positive solutions are obtained.
Motivated by all the works above, in this paper, we deal with the following p-Laplacian fractional differential equation boundary value problem:


where , , , are the standard Riemann-Liouville fractional derivatives and . By the properties of Green’s function, the Guo-Krasnosel’skii fixed-point theorem, the Leggett-Williams fixed-point theorem, and the upper and lower solutions method, some new results on the existence of positive solutions are obtained for the fractional differential equation boundary value problem (1.1) and (1.2).
The rest of this paper is organized as follows. In Section 2.7, we introduce some definitions and lemmas to prove our main results. In Section 3, we investigate the existence of a single positive solution for boundary value problems (1.1) and (1.2) by the upper and lower solutions method. In Section 4, we establish the existence of single and multiple positive solutions for boundary value problems (1.1) and (1.2) by fixed-point theorems. As applications, examples are presented to illustrate our main results in Section 3 and Section 4, respectively.
2 Preliminaries and lemmas
For the convenience of the reader, we give some background material from fractional calculus theory to facilitate the analysis of problem (1.1) and (1.2). These materials can be found in the recent literature, see [7, 8, 26, 27, 30–33].
Definition 2.1[7]
The fractional integral of order of a function is given by
provided the right-hand side is pointwise defined on .
Definition 2.2[7]
The fractional derivative of order of a continuous function is given by
where n is the smallest integer greater than or equal to α, provided that the right-hand side is pointwise defined on .
Lemma 2.1[7]
Let. If we assume, then the fractional differential equation
has
where n is the smallest integer greater than or equal to α.
Lemma 2.2[7]
Assume thatwith a fractional derivative of order. Then
for some, , where n is the smallest integer greater than or equal to α.
Lemma 2.3[27]
Letand. Then fractional differential equation boundary value problem


has a unique solution
where
Lemma 2.4 Letand, . Then the fractional differential equation boundary value problem


has a unique solution
where
is defined as (2.3).
Proof From Lemma 2.2 and , we have
In view of (2.4), we obtain
Therefore,
that is,
By the boundary conditions , we have
Therefore, the solution of fractional differential equation boundary value problem (2.4) and (2.5) satisfies
Consequently, . Thus, fractional differential equation boundary value problem (2.4) and (2.5) is equivalent to the following problem:
Lemma 2.3 implies that fractional differential equation boundary value problem (2.4) and (2.5) has a unique solution
The proof is complete. □
Lemma 2.5 Let, . The functionsanddefined by (2.3) and (2.6), respectively, are continuous onand satisfy
-
(1)
, for ;
-
(2)
, for ;
-
(3)
for ;
-
(4)
there exist two positive functions such that
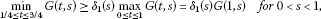 (2.7)
(2.7) (2.8)
(2.8)
Proof Observing the expression of and , it is easy to see that and for .
From Lemma 3.1 in [27] and Lemma 2.4 in [8], we obtain (2) and (3).
In the following, we consider the existence of and . Firstly, for given , is increasing with respect to t for . Consequently, setting
we have
Secondly, with the use of the monotonicity of , we have
Thus, setting
then (2.7) holds.
Similar to Lemma 2.4 in [8], we choose
The proof is complete. □
Lemma 2.6 Let. Ifand, then fractional differential equation boundary value problem (2.1) and (2.2) has a unique solution, .
Proof From Lemma 2.3, the fractional differential equation boundary value problem (2.1) and (2.2) has a unique solution
In view of Lemma 2.5, we know is continuous on and for . If and , we obtain . The proof is complete. □
Let . Now, we introduce definitions about the upper and lower solutions of fractional differential equation boundary value problem (1.1) and (1.2).
Definition 2.3[26]
A function is called an upper solution of fractional differential equation boundary value problem (1.1) and (1.2) if and satisfies
Definition 2.4[26]
A function is called a lower solution of fractional differential equation boundary value problem (1.1) and (1.2) if and satisfies
Definition 2.5[8]
The map θ is said to be a nonnegative continuous concave functional on a cone P of a real Banach space E provided that is continuous and
for all and .
Lemma 2.7[8]
Let E be a Banach space, be a cone, and, be two bounded open balls of E centered at the origin with. Suppose thatis a completely continuous operator such that either
-
(i)
, and , or
-
(ii)
, and ,
holds. Thenhas a fixed point in.
Let be constants, , .
Lemma 2.8[8]
Let P be a cone in a real Banach space E, , θ be a nonnegative continuous concave functional on P such thatfor all, and. Supposeis completely continuous and there exist constantssuch that
(C1) andfor;
(C2) for;
(C3) forwith.
Then ℬ has at least three fixed points, , andwith
Let be endowed with . Define the cone by
Let the nonnegative continuous concave functional θ on the cone P be defined by
Lemma 2.9 Let be the operator defined by
Thenis completely continuous.
Proof Let , in view of the nonnegativeness and continuity of , , and , we have is continuous.
Let be bounded, i.e., there exists a positive constant such that for all . Let , then for , we have
Hence, is uniformly bounded.
On the other hand, since is continuous on , it is uniformly continuous on . Thus, for fixed and for any , there exists a constant such that any and ,
Then, for all ,
that is to say, is equicontinuous. By the Arzela-Ascoli theorem, we have is completely continuous. The proof is complete. □
3 Existence of a single positive solution
In this section, for the sake of simplicity, we assume that
(H1) is nonincreasing to u;
(H2) There exists a continuous function , such that


Theorem 3.1 Assume that (H1) and (H2) hold. Then the fractional differential equation boundary value problem (1.1) and (1.2) has at least one positive solution.
Proof From Lemma 2.9, we obtain . By direct computations, we have


Now, we prove that the functions , are upper and lower solutions of fractional differential equation boundary value problem (1.1) and (1.2), respectively.
From (H1) and (H2), we have
Hence, . By , we know . From (3.3)-(3.5) we have


that is, and are upper and lower solutions of fractional differential equation boundary value problem (1.1) and (1.2), respectively.
Next, we show that the fractional differential equation boundary value problem


has a positive solution, where
Thus, we consider the operator defined as follows:
where and are defined as (2.3) and (2.6), respectively. It is clear that for all and a fixed point of the operator B is a solution of the fractional differential equation boundary value problem (3.8) and (3.9).
Similar to Lemma 2.9, we know that B is a compact operator. By the Schauder fixed-point theorem, the operator B has a fixed point, that is, the fractional differential equation boundary value problem (3.8) and (3.9) has a positive solution.
Finally, we will prove that fractional differential equation boundary value problem (1.1) and (1.2) has at least one positive solution.
Suppose that is a solution of (3.8) and (3.9). Now, to complete the proof, it suffices to show that , .
Let be a solution of (3.8) and (3.9). We have
From (H1), we have
By (H2) and (3.5), we obtain
By and (3.3), we can get
Combining (3.4), (3.11)-(3.14), we have


Let . By (3.16), we obtain .
By Lemma 2.6, we know , , which implies that
Since is monotone increasing, we obtain , that is, . By Lemma 2.6, (3.15) and (3.16), we have . Therefore, , .
In a similar way, we can prove that , . Consequently, is a positive solution of fractional differential equation boundary value problem (1.1) and (1.2). This completes the proof. □
Example 3.1
We consider the following fractional differential equation boundary value problem:


Clearly, is nonincreasing relative to u. This shows that (H1) holds.
Let . From Lemma 2.5, we have
and , there exist positive numbers and such that and .
Choosing a positive number and combining the monotonicity of T, we have
Taking , then we have

That is, the condition (H2) holds. By Theorem 3.1, the fractional differential equation boundary value problem (3.17) and (3.18) has at least one positive solution.
4 Existence of single and multiple positive solutions
In this section, for convenience, we denote
Theorem 4.1 Letbe continuous on. Assume that there exist two positive constantssuch that
(A1) for;
(A2) for.
Then the fractional differential equation boundary value problem (1.1) and (1.2) has at least one positive solution u such that.
Proof From Lemmas 2.3, 2.4, and 2.9, we get that is completely continuous and fractional differential equation boundary value problem (1.1) and (1.2) has a solution if and only if u solves the operator equation . In order to apply Lemma 2.7, we divide our proof into two steps.
Step 1. Let . For , we have for all . It follows from (A1) that for ,
So,
Step 2. Let . For , we have for all . It follows from (A2) that for ,
Therefore,
Then, by (ii) of Lemma 2.7, we complete the proof. □
Example 4.1
We consider the following fractional differential equation boundary value problem:


Let . By a simple computation, we obtain , . Choosing , , therefore
With the use of Theorem 4.1, the fractional differential equation boundary value problem (4.1) and (4.2) has at least one solution u such that .
Theorem 4.2 Letbe continuous on. Assume that there exist constantssuch that the following assumptions hold:
(B1) for;
(B2) for;
(B3) for.
Then the fractional differential equation boundary value problem (1.1) and (1.2) has at least three positive solutions, , andwith
Proof From Lemmas 2.3, 2.4, and 2.9, we have is completely continuous and fractional differential equation boundary value problem (1.1) and (1.2) has a solution if and only if u satisfies the operator equation .
We show that all the conditions of Lemma 2.8 are satisfied. If , then . By (B3), we have
Hence, . In the same way, if , then assumption (B1) yields . Therefore, condition (C2) of Lemma 2.8 is satisfied.
To check condition (C1) of Lemma 2.6, we choose , . It is easy to see that , ; consequently, . Hence, if , then for . From assumption (B2), we have for . So,
i.e., for all . Choosing , this shows that condition (C1) of Lemma 2.8 is also satisfied.
In the same way, if and , we also obtain . Then condition (C3) of Lemma 2.8 is also satisfied.
By Lemma 2.8, the fractional differential equation boundary value problem (1.1) and (1.2) has at least three positive solutions , , and , satisfying
The proof is complete. □
Example 4.2
We consider the following fractional differential equation boundary value problem:


where
Let . We obtain , . Choosing , , , therefore

With the use of Theorem 4.2, the fractional differential equation boundary value problem (4.3) and (4.4) has at least three positive solutions , , and with
References
Oldham KB, Spanier J: The Fractional Calculus. Academic Press, New York; 1974.
Podlubny I Mathematics in Science and Engineering. In Fractional Differential Equations. Academic Press, New York; 1999.
Agrawal OP: Formulation of Euler-Lagrange equations for fractional variational problems. J. Math. Anal. Appl. 2002, 272(1):368-379. 10.1016/S0022-247X(02)00180-4
Weitzner H, Zaslavsky GM: Some applications of fractional equations. Commun. Nonlinear Sci. Numer. Simul. 2003, 8(3-4):273-281. 10.1016/S1007-5704(03)00049-2
Meral FC, Royston TJ, Magin R: Fractional calculus in viscoelasticity: an experimental study. Commun. Nonlinear Sci. Numer. Simul. 2010, 15(4):939-945. 10.1016/j.cnsns.2009.05.004
Machado JT, Kiryakova V, Mainardi F: Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16(3):1140-1153. 10.1016/j.cnsns.2010.05.027
Kilbas AA, Srivastava HH, Trujillo JJ: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam; 2006.
Bai Z, Lü H: Positive solutions for boundary value problem of nonlinear fractional differential equation. J. Math. Anal. Appl. 2005, 311(2):495-505. 10.1016/j.jmaa.2005.02.052
Wang G, Zhang L, Ntouyas SK: Existence of multiple positive solutions of a nonlinear arbitrary order boundary value problem with advanced arguments. Electron. J. Qual. Theory Differ. Equ. 2012., 2012: Article ID 15
Zhou Y, Jiao F, Li J: Existence and uniqueness for p -type fractional neutral differential equations. Nonlinear Anal. 2009, 71(7-8):2724-2733. 10.1016/j.na.2009.01.105
Zhao Y, Sun S, Han Z, Zhang M: Positive solutions for boundary value problems of nonlinear fractional differential equations. Appl. Math. Comput. 2011, 217(16):6950-6958. 10.1016/j.amc.2011.01.103
Zhao Y, Sun S, Han Z, Li Q: Positive solutions to boundary value problems of nonlinear fractional differential equations. Abstr. Appl. Anal. 2011., 2011: Article ID 390543. doi:10.1155/2011/390543
Feng W, Sun S, Han Z, Zhao Y: Existence of solutions for a singular system of nonlinear fractional differential equations. Comput. Math. Appl. 2011, 62(3):1370-1378. 10.1016/j.camwa.2011.03.076
Zhao Y, Sun S, Han Z, Li Q: The existence of multiple positive solutions for boundary value problems of nonlinear fractional differential equations. Commun. Nonlinear Sci. Numer. Simul. 2011, 16(4):2086-2097. 10.1016/j.cnsns.2010.08.017
Yang X, Wei Z, Dong W: Existence of positive solutions for the boundary value problem of nonlinear fractional differential equations. Commun. Nonlinear Sci. Numer. Simul. 2012, 17(1):85-92. 10.1016/j.cnsns.2011.05.007
Xu X, Jiang D, Yuan C: Multiple positive solutions for the boundary value problem of a nonlinear fractional differential equation. Nonlinear Anal. 2009, 71(10):4676-4688. 10.1016/j.na.2009.03.030
Nyamoradi N, Bashiri T: Multiple positive solutions for nonlinear fractional differential systems. Fract. Differ. Calc. 2012, 2(2):119-128.
Ahmad B, Nieto JJ: Sequential fractional differential equations with three-point boundary conditions. Comput. Math. Appl. 2012, 64(10):3046-3052. 10.1016/j.camwa.2012.02.036
Nyamoradi N, Bashiri T: Existence of positive solutions for fractional differential systems with multi point boundary conditions. Ann. Univ. Ferrara 2012. doi:10.1007/s11565-012-0160-x
Nyamoradi N, Javidi M: Existence of multiple positive solutions for fractional differential inclusions with m -point boundary conditions and two fractional orders. Electron. J. Differ. Equ. 2012., 2012: Article ID 187
Ahmad B, Nieto JJ, Alsaedi A, El-Shahed M: A study of nonlinear Langevin equation involving two fractional orders in different intervals. Nonlinear Anal., Real World Appl. 2012, 13(2):599-606. 10.1016/j.nonrwa.2011.07.052
Han Z, Lu H, Sun S, Yang D: Positive solutions to boundary value problems of p -Laplacian fractional differential equations with a parameter in the boundary conditions. Electron. J. Differ. Equ. 2012., 2012: Article ID 213
Chen T, Liu W: An anti-periodic boundary value problem for fractional differential equation with p -Laplacian operator. Appl. Math. Lett. 2012, 25(11):1671-1675. 10.1016/j.aml.2012.01.035
Wang J, Xiang H, Liu Z: Existence of concave positive solutions for boundary value problem of nonlinear fractional differential equation with p -Laplacian operator. Int. J. Math. Math. Sci. 2010., 2010: Article ID 495138. doi:10.1155/2010/495138
Liang S, Zhang J: Positive solutions for boundary value problems of nonlinear fractional differential equation. Nonlinear Anal. 2009, 71(11):5545-5550. 10.1016/j.na.2009.04.045
Wang J, Xiang H: Upper and lower solutions method for a class of singular fractional boundary value problems with p -Laplacian operator. Abstr. Appl. Anal. 2010., 2010: Article ID 971824. doi:10.1155/2010/971824
Lu H, Han Z: Existence of positive solutions for boundary value problem of fractional differential equation with p -Laplacian operator. Proceedings of the 2012 International Conference on Computer Science and Information Processing (CSIP) 2012, 582-585.
Chen T, Liu W, Hu Z: A boundary value problem for fractional differential equation with p -Laplacian operator at resonance. Nonlinear Anal. 2012, 75(6):3210-3217. 10.1016/j.na.2011.12.020
Chai G: Positive solutions for boundary value problem of fractional differential equation with p -Laplacian operator. Bound. Value Probl. 2012., 2012: Article ID 18
El-Shahed M: Positive solutions for boundary value problems of nonlinear fractional differential equation. Abstr. Appl. Anal. 2007., 2007: Article ID 10368. doi:10.1155/2007/10368
Leggett RW, Williams LR: Multiple positive fixed points of nonlinear operators on ordered Banach spaces. Indiana Univ. Math. J. 1979, 28(4):673-688. 10.1512/iumj.1979.28.28046
Krasnosel’skii MA: Positive Solution of Operator Equation. Noordhoff, Groningen; 1964.
Isac G: Leray-Schauder Type Alternatives, Complementarity Problems and Variational Inequalities. Springer, New York; 2006.
Acknowledgements
The authors sincerely thank the reviewers for their valuable suggestions and useful comments that have led to the present improved version of the original manuscript. This research is supported by the Natural Science Foundation of China (11071143, 60904024, 61174217), Natural Science Outstanding Youth Foundation of Shandong Province (JQ201119) and supported by Shandong Provincial Natural Science Foundation (ZR2012AM009, ZR2010AL002, ZR2011AL007), also supported by Natural Science Foundation of Educational Department of Shandong Province (J11LA01).
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
The authors declare that the study was realized in collaboration with the same responsibility. All authors read and approved the final manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Lu, H., Han, Z., Sun, S. et al. Existence on positive solutions for boundary value problems of nonlinear fractional differential equations with p-Laplacian. Adv Differ Equ 2013, 30 (2013). https://doi.org/10.1186/1687-1847-2013-30
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/1687-1847-2013-30