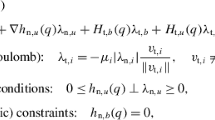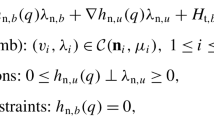Abstract
This article deals with the analysis of the contact complementarity problem for Lagrangian systems subjected to unilateral constraints, and with a singular mass matrix and redundant constraints. Previous results by the authors on existence and uniqueness of solutions of some classes of variational inequalities are used to characterize the well-posedness of the contact problem. Criteria involving conditions on the tangent cone and the constraints gradient are given. It is shown that the proposed criteria easily extend to the case where the system is also subjected to a set of bilateral holonomic constraints, in addition to the unilateral ones. In the second part, it is shown how basic convex analysis may be used to show the equivalence between the Lagrangian and the Hamiltonian formalisms when the mass matrix is singular.






Similar content being viewed by others
Notes
Another constraint qualification guaranteeing nonemptyness of \(\tilde{K}\), hence of Φ, is range \((\nabla h(q)^{\mathrm{T}})- \mathbb {R}^{m}_{+}=\mathbb {R}^{m}\), which does not imply that ∇h(q)T has rank m [17].
The minus sign is added here just to be coherent with the unilateral case.
References
Acary, V., Brogliato, B.: Numerical Methods for Nonsmooth Dynamical Systems. Lecture Notes in Applied and Computational Mechanics, vol. 35. Springer, Berlin (2008)
Addi, K., Brogliato, B., Goeleven, D.: A qualitative mathematical analysis of a class of linear variational inequalities via semi-complementarity problems. Applications in electronics. Math. Program., Ser. A 126(1), 31–67 (2011)
Agrawal, S.K.: Inertia matrix singularity of series-chain spatial manipulators with point masses. J. Dyn. Syst. Meas. Control 115(4), 723–725 (1993). doi:10.1115/1.2899204
Agrawal, S.K.: Inertia matrix singularity of planar series-chain manipulators. In: Proceedings of the IEEE Int. Conference on Robotics and Automation, Sacramento, California, USA, April 1991, pp. 102–107 (1991)
Agrawal, S.K.: Series-chain planar manipulators: inertial singularities. J. Mech. Des. 115(4), 941–945 (1993). doi:10.1115/1.2919291
Arnold, V.I.: Mathematical Methods of Classical Mechanics. Graduate Texts in Mathematics, vol. 60. Springer, Berlin (1989)
Aubin, J.P.: Applied Functional Analysis. Wiley, New York (1979)
Balakrishnan, A.V.: Vibrating systems with singular mass-inertia matrices. In: Proceedings of First International Conference on Nonlinear Problems in Aviation and Aerospace, Daytona Beach, Florida, USA, 9–11 May 1996 (1996)
Batlle, C., Gomis, J., Pons, J.M., Roman-Roy, N.: Equivalence between the Lagrangian and Hamiltonian formalism for constrained systems. J. Math. Phys. 27(12), 2953–2962 (1986)
Bernstein, D.S.: Matrix, Mathematics. Theory, Facts, and Formulas with Application to Linear Systems Theory. Princeton University Press, Princeton (2005)
Bertsekas, D.P.: Convex Optimization Theory. Athena Scientific, Belmont (2009)
Bhat, S.P., Bernstein, D.S.: Second-order systems with singular mass matrix and an extension of Guyan reduction. SIAM J. Matrix Anal. Appl. 17(3), 649–657 (1996)
Brezis, H.: Analyse Fonctionnelle. Théorie et Applications, 4th edn. Masson, Paris (1983)
Brogliato, B.: Nonsmooth Mechanics. Models, Dynamics and Control, 2nd edn. Springer, London (1999)
Brogliato, B.: Inertial couplings between unilateral and bilateral holonomic constraints in frictionless Lagrangian systems. Multibody Syst. Dyn. 29(3), 289–325 (2013). doi:10.1007/s11044-012-9317-8
Brogliato, B.: Kinetic quasi-velocities in unilaterally constrained Lagrangian mechanics with impacts and friction. Multibody Syst. Dyn. 32(2), 175–216 (2014). doi:10.1007/s11044-013-9392-5
Brogliato, B., Thibault, L.: Well-posedness results for non-autonomous complementarity systems. J. Convex Anal. 17(3–4), 961–990 (2010)
Cariñena, J.F.: Theory of singular Lagrangians. Fortschr. Phys. 38(9), 641–679 (1990)
Facchinei, F., Pang, J.S.: Finite-Dimensional Variational Inequalities and Complementarity Problems. Volume I. Springer Series in Operations Research. Springer, New York (2003)
Fraczek, J., Wojtyra, M.: On the unique solvability of a direct dynamics problem for mechanisms with redundant constraints and Coulomb friction in joints. Mech. Mach. Theory 46, 312–334 (2011)
Garcia de Jalon, J., Gutierrez-Lopez, M.D.: Multibody dynamics with redundant constraints and singular mass matrix: existence, uniqueness, and determination of solutions for accelerations and constraints forces. Multibody Syst. Dyn. 30(3), 311–341 (2013). doi:10.1007/s11044-013-9358-7
Garcia de Jalon, J., Callejo, A., Hidalgo, A.F.: Efficient solution of Maggi’s equations. J. Comput. Nonlinear Dyn. 7, 021003 (2012)
Havelkova, M.: A geometric analysis of dynamical systems with singular Lagrangians. Commun. Math. 19, 169–178 (2011)
Hiriart-Urruty, J.B., Lemaréchal, C.: Fundamentals of Convex Analysis. Grundlehren Text Editions. Springer, Berlin (2001)
Hurtado, J.E., Sinclair, A.J.: Lagrangian mechanics of overparameterized systems. Nonlinear Dyn. 66, 201–212 (2011)
Kamimura, K.: Singular Lagrangian and constrained Hamiltonian systems, generalized canonical formalism. Nuovo Cimento B 68(1), 33–54 (1982)
Laulusa, A., Bauchau, O.A.: Review of classical approaches for constraint enforcement in multibody systems. J. Comput. Nonlinear Dyn. 3, 011004 (2008)
Lötstedt, P.: Mechanical systems of rigid bodies subject to unilateral constraints. SIAM J. Appl. Math. 42(2), 281–296 (1982)
McClamroch, N.H., Wang, D.: Feedback stabilization and tracking of constrained robots. IEEE Trans. Autom. Control 33(5), 419–426 (1988)
Moreau, J.J.: Les liaisons unilatérales et le principe de Gauss. C. R. Acad. Sci., Paris, 1er Sem. 256(4), 871–874 (1963)
Moreau, J.J.: Quadratic programming in mechanics: dynamics of one-sided constraints. SIAM J. Control 4(1), 153–158 (1966)
Moreau, J.J.: Unilateral contact and dry friction in finite freedom dynamics. In: Moreau, J.J., Panagiotopoulos, P.D. (eds.) Nonsmooth Mechanics and Applications. Int. Centre for Mechanical Sciences, Courses and Lectures, vol. 302, pp. 1–82. Springer, Berlin (1988)
Pons, J.M.: On Dirac’s incomplete analysis of gauge transformations. Stud. Hist. Philos. Mod. Phys. 36, 491–518 (2005)
Rockafellar, R.T., Wets, R.J.B.: Variational Analysis. Grundlehren der Mathematischen Wissenschaften, vol. 317. Springer, Berlin (1998)
Rockafellar, R.T.: Convex Analysis. Princeton Landmarks in Mathematics (1970)
Scheck, F.: Mechanics. From Newton’s Laws to Deterministic Chaos, 2nd edn. Springer, Berlin (1994)
Shampine, L.F., Reichelt, M.W., Kierzenka, J.A.: Solving index-I DAEs in Matlab and Simulink. SIAM Rev. 41(3), 538–552 (1999)
Schutte, A., Udwadia, F.: New approach to the modeling of complex multibody dynamical systems. J. Appl. Mech. 78, 021018 (2011)
Simeon, B.: Computational Flexible Multibody Dynamics. A Differential-Algebraic Approach. Springer, Berlin (2013)
Udwadia, F.E., Phohomsiri, P.: Explicit equations of motion for constrained mechanical systems with singular mass matrices and applications to multi-body dynamics. Proc. R. Soc. A, Math. Phys. Eng. Sci. 462, 2097–2117 (2006)
Udwadia, F.E., Schutte, A.D.: Equations of motion for general constrained systems in Lagrangian mechanics. Acta Mech. 213(1–2), 111–129 (2010)
Wojtyra, M.: Joint reaction in rigid body mechanisms with dependent constraints. Mech. Mach. Theory 44, 2265–2278 (2009)
Yen, J.: Constrained equations of motion in multibody dynamics as ODEs on manifolds. SIAM J. Numer. Anal. 30(2), 553–568 (1993)
Zimmer, G., Meier, J.: On observing nonlinear descriptor systems. Syst. Control Lett. 32, 43–48 (1997)
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix A: The Mangasarian–Fromovitz Constraint Qualifications (MFCQ) [19, pp. 17, 252]
Let \(K=\{x \in \mathbb {R}^{n} : h(x) \geq0\}\), where \(h: \mathbb {R}^{n} \rightarrow \mathbb {R}^{m}\) is continuously differentiable, and K is not necessarily convex. Suppose that there exists a vector \(v \in \mathbb {R}^{n}\) such that ∇h i (x)T v>0 for all \(i \in\mathcal{ I}(x)=\{i : h_{i}(x) =0\}\). Then the tangent cone to K at x, defined as the dual of the normal cone in (14) is equal to the linearization cone \(\{z \in \mathbb {R}^{n} : z^{\mathrm{T}}\nabla h_{i}(x) \geq0,\ \mbox{for all} i \in\mathcal{I}(x)\}\).
Appendix B: The chain rule of convex analysis
Theorem 1
[35, Theorem 23.9]
Let f(x)=h(Ax) where h(⋅) is a proper convex function on \(\mathbb {R}^{m}\) and A is a linear transformation from \(\mathbb {R}^{n}\) to \(\mathbb {R}^{m}\). Then if h(⋅) is polyhedral and Im(A) contains a point of dom(h), one has ∂f(x)=A T ∂h(Ax), for all x.
Rights and permissions
About this article
Cite this article
Brogliato, B., Goeleven, D. Singular mass matrix and redundant constraints in unilaterally constrained Lagrangian and Hamiltonian systems. Multibody Syst Dyn 35, 39–61 (2015). https://doi.org/10.1007/s11044-014-9437-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11044-014-9437-4