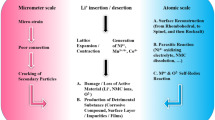
Abstract
The main purpose of these studies is to compare the diffusion coefficient and ionic conductivity of carbon-based anode materials (graphene and corn starch carbon, graphene nanoplatelets) at different temperatures. The tests were carried out using electrochemical impedance spectroscopy (EIS) in a temperature chamber, which allows the assessment of important parameters during the cell’s operation and optimization, as well as the thermodynamic and kinetic response of the system to various conditions, which are important parameters taking into account the cell’s safety. An interesting method was proposed to determine thermodynamic and kinetic parameters for the development of two models of lithium ion diffusion during half-cell charging. It has been shown that the lithiation process is thermodynamically endothermic and forced. Various carbon materials were used, including corn starch-based carbon as an anode material that equates to the slogans of biodegradability, lower cost and ecology.
Graphical Abstract

Similar content being viewed by others
Avoid common mistakes on your manuscript.
Statement of Novelty
The work is a scientific novelty, because the obtained carbonized starch material has a similar structure to that of graphene nanoplatelets, which are recently frequently considered anode active material for LiBs. The paper presents the main mechanisms accompanying the processes of electrochemical intercalation of lithium ions, in which diffusion dominates. The diffusion coefficients at different operating temperatures of the half-cell were determined. A scientific innovation is the use of starch in lithium-ion cells as an anode material compared to GNP (graphene nanoplates) with different particle sizes and graphene. In addition, the work presents a thermodynamic and kinetic analysis of temperature-dependent behavior using electrochemical impedance spectroscopy. This finding was made possible by the use of two innovative copyright models. The kinetic and thermodynamic parameters enable comparison of different carbon materials due to their thermal stability and electrochemical reactions speed and mechanism. It plays an important role f.e. in case of roll-press process while the electrode material is being pressed under high temperature. If the stability of material is quite low there is possibility of defects formation on the coated area. This parameter also influences electrode mechanical properties such as tensile strength and elongation, which, if proper, ensure higher battery life and voltage regimes optimalization. Ultimately, economic modeling of electrode manufacturing was used to investigate whether the application of these materials could be scalable or whether other solutions were sought.
Introduction
Getting to know the unique properties of new materials allows you to expand your knowledge with new, often very subtle physicochemical parameters describing our environment. As a consequence, it makes it possible to improve the already existing solutions and introduce new research methods. The increasing demand for portable electricity receivers and the development of the automotive, aviation, military, and even space industries forces high efficiency and miniaturization of power devices used in them. They are not without significant safety and ecology considerations, which are largely guaranteed by modern materials. Important parameters describing electrochemical current sources are energy, power, and specific capacity. Achieving high values of these parameters is associated with the effective interaction of all system components, which include: electrolyte, electrodes and the electrode/electrolyte phase boundary, and electrochemically inactive parts of the device (current collector, separator, housing).
Carbon is also used in the production of protective mask inserts and the production of water and air filters. It is also used for the clarification of protein hydrolysates, musts, wines, and juices, for the production of spirit, and pure sugar, for the separation of gas mixtures, and the purification of vapors and gases. On the other hand, very black varieties of activated carbon are used for coloring.
The interest in carbon as an electrochemically active material dates back to the beginning of the nineteenth century when in the years 1807–1808 Sir Humphrey Davy used graphite electrodes for the electro-winning of alkali metals, potassium, and magnesium [1]. Then, in 1866, Leclanché used carbon nanoparticles, the so-called carbon black (CB) as an anode in cells of the first type, in the form of a rod surrounded by powdered manganese dioxide (MnO2) [2]. In later years, CB was replaced with porous glassy carbon, graphite, or carbon fibers [2, 3]. Due to its high conductivity and good oxygen absorption, graphite has also found application in [4] alkaline batteries, and CB and other carbon varieties in [5] zinc-oxygen batteries and [6, 7] fuel cells. This allowed for the construction of cells or sensors free from toxic heavy metals [8, 9]. The advantage of carbon materials is their high affinity for biologically active molecules. Bio-cells [10] or enzymatic biosensors [11] or sensors are sensitive to biomolecules [12], which are easily adsorbed on developed surfaces of carbon materials [13]. The use of carbon for the above-mentioned purposes has its justification in unusual electrical and structural properties. Its uniqueness is associated with the occurrence of many permanent forms and allotropic varieties (Fig. 1).
Depending on the type of hybridization and the atomic structure, carbon can form layers of semiconductor graphite, non-conductive, very hard diamond, or amorphous carbon with a highly developed surface. In addition, it creates many other nanostructures, such as nanotubes, nanobubbles, graphene [14], or the recently proposed penta-graphene [15]. A controversial allotropic form is carbon, in which carbon atoms are alternately linked by a triple and a single bond. Despite its interesting potential properties, such as high mechanical strength, due to its low stability and complex synthesis, remains in the realm of research. Such a huge variety of carbon forms gives many possibilities for design solutions and applications in modern technology and electrochemistry.
In electrochemistry, structures based on carbon with sp2 hybridization, such as graphite, amorphous carbon, carbon nanotubes (CNTs), fullerenes, and graphene, are most often used. The least ordered amorphous carbon is made of graphene layers arranged like houses of cards, with many defects and distortions. The graphene layer is composed of planar carbon atoms connected by strong covalent bonds to form a hexagonal structure. It occurs in these layers sp2 hybridized carbon, unlike sp3 in diamond, a material with completely different properties. In the case of amorphous carbon in addition to sp2 hybridization, there are also carbon fractions with sp3 hybridization.
The aim of the research was to determine the kinetic and thermodynamic parameters based on the analysis of impedance spectroscopy. Various carbon materials were compared, from the point of potential use as an electrode material.
Experimental
Materials Used
During the research, commercial starches, graphene nanoplatelets, and graphene were applied. Corn starch (CS) was purchased from Sigma-Aldrich. Also the graphene nanoplatelets (GNPs) were purchased from Sigma-Aldrich:
-
Graphene nanoplatelets (5 μm particle size, BET specific surface area (SSA) 120–150 m2 g−1) (5GNPs);
-
Graphene nanoplatelets (25 μm particle size, BET SSA 50–80 m2 g−1) (25GNPs).
However, graphene (G) was ordered from ACS Material:
-
Single-layer graphene (Graphene Factory) (specific BET surface area 650–750 m2 g−1) synthesized via thermal exfoliation reduction and hydrogen reduction.
To prepare electrode paste the following materials were applied:
-
1 M Lithium hexafluorophosphate in ethylene carbonate (EC) and dimethyl carbonate (DMC) (1:1 v/v) (purity ≥ 99.9%) from Sigma-Aldrich;
-
Acetylene Black (AB) from Sigma-Aldrich;
-
Poly (vinylidene fluoride) (PVdF) from Fluka;
-
1-methyl-2-pyrrolidone (NMP) (purity ≥ 98%) from Fluka.
Starch-based carbon material was prepared by carbonizing the polymer under nitrogen (inert gas) in a Nabertherm tube furnace. The carbonization process is the thermal treatment of a sample in an inert atmosphere to obtain carbon-based material. The whole process lasted 6 h. The temperature rate was equal to 300 °C h−1, while the whole carbonization process lasted 4 h. Before this process, the heating of the furnace was carried out for 2 h. The volumetric nitrogen flow rate (gas flow rate) was 50 l h−1. The conditioning of the system in inert gas before starting carbonization took 1 h to the process (600 °C). The purity of technical gas was 99.998%. Figure 2a, b shows the actual image of the structure of the obtained carbon after carbonization, using the CSC (corn starch after carbonization) example, and the appearance of the pipe after the process. It is believed that the yellow oily liquid may be phenolic derivatives and needs further investigation using chromatographic methods.
Figure 2c shows the starch carbonization process used to obtain carbon with specific properties, and the intercalation of lithium ions during charging-discharging of the half-cell into the carbon structure.
Physicochemical Properties
Particle Diameter
A Malvern Instruments Zetasizer was used to analyze the particle diameter. Its operation is based on dynamic light scattering. Using this technique, Brownian motion is analyzed and converted to size and size distribution using the Stokes–Einstein relationship. The non-invasive backscatter technology works over a wide range of concentrations. A heterogeneous solution was prepared by weighing 0.01 g of the sample and dispersing it in 25 mL of isopropyl alcohol.
Brunauer–Emmett–Teller Analysis (BET)
The analysis of sorption properties of carbon materials was performed using the ASAP (Accelerated Surface Area and Porosimetry System) 2020 apparatus with the Micrometritics Instrument Corporation (USA) software with low-temperature nitrogen adsorption. Before the measurement, the samples were degassed for 4 h at the temperature of 120 °C. During the analysis of data, it was assumed according to ISO procedure that micropores have diameters lower than 2 nm, mesopores higher than 2 nm, and lower than 50 nm while macropores have diameters higher than 50 nm.
Scanning Electron Microscope (SEM)
A scanning electron microscope with electron acceleration from 0.2 to 30 kV EVO40 (Zeiss, Jena, Germany) was used to show the morphological structure of carbon samples. The technique is optical and focuses the electrons beam to produce high magnification pictures of surface topography.
X-Ray Diffraction (XRD)
To determine the crystallinity, X-ray diffraction and interference analysis were performed. The analysis was carried out by placing the sample in the D8 Advance diffractometer (Bruker, Germany). Here, Cu Kα (α = 1.5418 Å) as X-ray energy was used. Moreover, the filter was Ni (nickel), while the scan was performed in the angular range of 5–50° (Δ2θ = 0.05°).
Electrochemical Impedance Spectroscopy (EIS)
For conducting electrochemical measurements Gamry GTM750 Potentiostat/Galvanostat/ZRA was used. Impedance was measured in the frequency range from 10 mHz to 100 kHz, with a voltage amplitude of 10 mV.
The process of heating was carried out in the thermal chamber Wamed KBC (18 W)—Fig. 3. The temperature is evenly distributed throughout the device. The device is equipped with double, independent systems protecting against uncontrolled temperature increases.
Inspected lithium-ion cells were created as follows. First, the electrode slurry was prepared. It consisted of 80% active anode material (which was GNPS, CS, and G), 10% acetylene black, and 10% polyvinylidene fluoride. All ingredients were mixed with the addition of N-Methyl-2-pyrrolidone. In the next step, the prepared slurry was spread on copper foil with industrial-type apparatus, Dr. Blade. The covered sheet was later put into a dryer to evaporate the solvent and stick nicely to the current collector. Anodes were dried for 24 h at 120 °C. Later, the circular electrodes were cut from the sheet and weight in. Finally, the lithium-ion cells were prepared in a Glovebox under an argon atmosphere.
The analysis was carried out after half-cell charging process at scan rate of 20 mA g−1 in the temperature range of 25–50 °C thermal stability of the electrolyte. Working electrode consisted of active anode material, binder, conductivity improving substance and filler. The electrodes were separated by the glass microfiber membrane (Whatmann, 0.4 mm thick). These were placed in Swagelok® as the connecting tube. The mass of electrode ingredients was as follows: carbon material: 3.5 mg, lithium plate: 45 mg. The thickness of the electrode was equal to 0.55 mm.
Every cell contained: investigating anode, counter electrode made from metallic lithium, 1 M solution of LiPF6 dissolved in a mixture of organic solvents EC and DMC with a mass ration 1:1 between them, Whatman® glass fiber separator.
Method—Mathematical Models Adopted to Obtain Thermodynamic and Kinetic Diffusion Mechanism of Lithium
In order to compare and understand the behavior of lithium ions at different temperatures during the charging of anode materials, Nyquist plots are presented and interpreted after data deconvolution (Fig. 4). In the next stage, activation energies of kinetic control processes were determined. Then, the diffusion coefficient of lithium ions and ionic conductivity were determined. On this basis, thermodynamic parameters (such as Gibbs function, entropy, enthalpy, equilibrium and kinetic constant (reaction rate constant, activation energy) for the anodes were determined.
The Arrhenius equation was used to obtain the rate constant k (Eq. 1) To designate the Gibbs free enthalpy (ΔG) of the diffusion the general Eyring-Polanyi equation was used (Eq. 2), where h is determined as the Planck constant.
Further, to estimate enthalpy (ΔH) in isobaric conditions (p = const), the combination of (1) and (2) Eqs was used, given by formula (3).
Finally, to determine entropy (ΔS), the Gibbs function (ΔG) is used (Eq. 4).
To calculate the equilibrium constant (K), the isotherm of van’t Hoff was adopted (Eq. 5).
For all samples after the charging process, diffusion coefficient DLi+ (cm2 s−1) due to Warbung curves in Nyquist plots was appointed. In literature [16, 17] researchers used Eqs. 6–7 (Table 1).
In the Eq. (6) R is gas constant equal to 8.314 J mol−1 K−1, T- standard temperature (298 K),
A—effective surface area of the electrode (1.54 cm2), n—electronic transport ratio during oxidation, F- Faraday’s constant (96,500 C mol−1), c—molar density of Li-ion in an electrode (0.001 mol cm−3), б—diffusion constant from Warbung line in Ω s−1/2.
To obtain constant б, the plots Z’, Z” versus ω−1/2 for diffusion resistances range were created and the slope was equal to б (Eq. 7). The calculation is only possible for solid-solution behavior. After that, the modified Arrhenius (Eq. 8) (model 1) is used to obtain a diffusion activation energy \({E}_{D}^{\#}\).
To obtain kinetic parameters a plot of linear expression of ln(Aw T−1) = f(T−1) has been done, which slope indicates the activation energy \({E}_{D}^{\#}\) of the diffusion process. In the Eq. (3) β is a constant determined by D0 (the pre-exponential factor), A, and c values. This model was used in the literature data [18]. Zhian Yu et al. [19] presented a different equation (model 2) to obtain the activation energy of the diffusion process: (Eq. 9).
Using Eq. (9), from the linear relationship between ln(DLi+) versus T−1 the activation energy can be obtained from the slope of the linear fitting.
During the charge and discharge, Li+ transfers from one side to another thus Boroujeni et al. [21] proposed, that there are five main regions, where lithium ions move (Fig. 5).
Figure 5 shows a diagram of the intercalation of lithium ions in a carbon material. So-called areas of solid active material in the anode and cathode can be observed. Additionally, solid particles separated from the electrolyte interface on the anode and cathode side are highlighted. It is also worth noting that the electrolyte may be in the separator, anode or cathode [20]. During charging, Li ions diffuse from the fixed cathode, overcoming the energy barrier between the mass and the electrolyte, picking up one electron and forming a thin layer at the interface. Then they go to the electrolyte (Butler-Volmer reaction), due to the concentration and electric field gradient. The last stage is the passage of ions through the SEI layer of the anode, obtaining an electron, getting inside the particles and diffusing.
At low salt concentrations, the ionic conductivity of the electrolyte with a ratio of 1:1 can be described by the Nernst-Einstein equation (N-E)—ionic conductivity of lithium ions (σLi+) in Warbung area [20], in which the ionic conductivity is related only to the self-diffusion of ions. However, this dependence may be approximated at high salt concentrations, where the ion-ion interaction effect begins to manifest [21,22,23,24,25,26,27,28,29] (Eq. 10)—Table 1.
Further, to obtain the pre-expotential factor A, the Eq. (11) was used. Where kb is the Boltzmann constant, and the plot ln (σLi+) = f(T−1) was created. Here, the intercept is the lnA.
Results and Discussion
Table 2 shows a comparison of the different sorption properties of starch after carbonization compared to GNPs and G. The thermal modification was carried out to improve the surface properties and electrical conductivity and reduce the thermal conductivity of the material. The carbonized starch have much larger specific surface area (SSA) than GNPs. G, unlike other materials, has a larger SSA, which in a way proves that there are more pores in the structure with a smaller diameter. Thus, CSCs and G could be applied as adsorbents that can also act as process catalysts. Compared to GNPs, starch after inert calcination has a similar pore volume, 2 times larger pore diameter and 3.8 times greater specific surface than 5GNPs, and 9.6 times greater than 25GNPs. The obtained pore diameter will be evidence of the mesopores’ presence. However, this diameter is at the limit of the micropores, which may therefore lower the diffusion coefficient of lithium ions (Table 2).
It should be noted that increasing the development of the SSA does not have to be associated with the improvement of the electronic conductivity and the intensified migration of the charge. The most important aspect is the pore size concerning the size of the electrolyte ions without and with a solvation shell. When the value is right, the SSA value begins to play an increased role in charge and ion transport. The larger the pore volume, the more lithium ions can enter the porous surface, which further increases the specific capacity. If, on the other hand, too many ions get into certain pores, it may result in the necessity to use higher current regimes for the deintercalation process.
It should be noted that the lithium-ion has an ion radius of about 0.60 nm. It means that the pore diameter must be larger than the ion diameter for the intercalation process to occur. Thus, if the pore diameter is greater than 1.2 nm, a positive insertion occurs. Because the pore size is much larger, it allows more ions to enter, while the problem is the high irreversibility of the process. This is due to the build-up of an SEI layer each time, which increases the overall resistance of the system and lowers the capacity and stability due to the presence of solvated shell ions. Assuming that the structures possess open-through pores the character of liquid movement in these is subcapillary. It is assumed based on the pore radius (lower than 10−4 mm). It means that if water was there it would be completely bounded and immobilized by molecular forces.
Also, it should be noticed that porosity is not influenced by grain size but strictly depends on grain sphericity and grain uniformity. Moreover, in the porous body different types of flows can occur (based on the so-called Knudsen number). These could be Poiseuille, Knudsen, or Volmer flow. The kind of flow depends mainly on resistance, viscosity, intermolecular interactions and collisions, and diffusion process.
The obtained morphological structures after starch carbonization are morphologically significantly similar to GNPs. GNPs are plate-shaped and almost identical to those found in the walls of carbon nanotubes (CNTs) but in a flat form. A similar shape of 25GNPs particles was found in work [30]. Although, in the presented here results the carbonization process was performed without any other modifications, such as sol–gel processes. Thus, it makes here presented modification less controlling and costly. Indeed, the particles form wrinkled and ultra-thin sheets upon carbonization. Because the main aim was to obtain graphene-based materials the as-obtained carbon sample was compared to these materials. From literature reports [31, 32], GNS can be morphologically compared with SCs. However, there is a great similarity in SCs to highly graphitized hard carbon more than to pyrolytic disordered carbon samples. Moreover, graphite contains parallel planted graphene layers in its structure and this may be the reason why the structures appear morphologically similar to both graphite and graphene. According to the literature, GNPs are thin and have flat particles that consist of single- and multi-layer graphene mixed with coarser graphite. Therefore, they have an intermediate form between graphene and graphite [33]. In the work [34], similar microscopic images were obtained for GNPs, synthesized in the process of mechanochemical synthesis from expanded graphite. In this work, the resulting carbon has a dense lamellar structure and the surface is visibly smoother. It influences the mechanical structure which is more durable, thicker and possesses a lower number of structural defects than GNPs. However, it is at the expense of reduced reactivity. For the G sample, the obtained SEM picture is similar to 25GNPs but G possesses a lower amount of defects.
In work [35], the graphene structure has been divided into single graphene layers and multilayers. Based on this visualization, it can be concluded in the submitted work that mainly multilayers for the structure of 25GNPs, G is observed. The most developed monolayer structure can be observed for CSC. This means that they have a lower tendency to aggregate particles. The disadvantage of the materials obtained is their less “ironed” structure, which may lead to lower mechanical strength.
Based on the data, each material is determined to be monodisperse due to its predominant intensities and volumes for one particle diameter range. Due to sedimentation, some materials exhibit polydisperse properties, which is characteristic of carbon materials. This determines their relatively complicated analysis (semicrystalline materials).
It should be noted that a very important rule in the selection of electrode materials for lithium-ion cells is the appropriate crystallographic structure. This means that during lithium-ion intercalation, the ions occupy free spaces within the lattice without disturbing the lattice.
Thus, there is no change in the crystallographic structure and thus complete reversibility of the insertion process is achieved. This has a significant impact on the value of the irreversible specific capacity. A material whose properties change during insertion exhibits inhomogeneous behavior and is therefore industrially unpredictable. The simplest example is graphite, the greatest advantage of which is its stable specific capacity. This is possible thanks to the six-member ordered layers of cyclic systems. The incorporation of ions into the material structure depends on the properties of the cathode material and the electrolyte. Ions solvated by the solvent must get rid of the solvation mantle while being incorporated into the structure of the anode material. Thus, the efficiency of building an SEI layer is dependent on the properties of the solvent, the interaction between the solvent molecule and the material, and the compatibility of the solvent with the lithium salt. After thermal treatment, as expected, the carbon obtained from starch contains most of the disorder (amorphous) area [36].
After carbonization (Fig. 6), the CSC were completely amorphous, which may be related to the destruction of the amylose structure. None of the obtained structures showed structural similarity to that before the process, which means that the resulting carbon structures have completely new crystallographic properties.
Since the obtained structure was hard carbon, peaks that would indicate an ordered structure were unnoticeable. The flow of electric charges is possible and is not limited by the size of the unit cell. A similar result was obtained for carbon fibers obtained from lignin [37]. The increase in the carbonization temperature did not contribute to the ordering of the system even close to the graphitization temperature. Moreover, defects present in the structure cause the peaks to broaden. Increasing the carbonization temperature causes the interlayer spacing to decrease and the crystallite size to increase [38, 39]. In work [40] authors obtained and examined hard carbon from corn straw piths and tried to apply this material successfully in sodium-ion batteries. The hard carbon can be used in LIB as well but the efficiency is lower.
Furthermore, considering higher lithium than sodium electrochemical equivalent the energetic results could be similar. For this material authors observed two broad peaks (23 ° and 43 °) that can correspond to the crystallographic planes: (002) and (100). They are characteristic of amorphous carbon. Also in this work, it was justified that an increase in the carbonization temperature reduces the interlayer distances between graphite-like layers. Thus, it can be concluded that a higher carbonization temperature would create in examined in this work case the peak at 43 °, which would be broad.Moreover, reducing (increasing) the number of diffraction peaks may be related to the disappearance (appearance) of functional groups that decrease (increase) the distances between graphene layers. It was confirmed for GNS (graphene nanosheets) in [41] and allows to confirm the supposition that SCs and GNPs have similar structural properties as GNS. For GNPs in both cases, the 2θ angles are seen at around 27 ° and indicate pure crystalline structure. On the other side, G possesses a semi-crystalline structure but is mostly disordered. It is confirmed in some literature reviews on graphene [42, 43]. The amorphous G consists of 5-6-7 polygons with a kind of predominant bonding creating a 2D structure [44].
Optimizing battery operating conditions has become extremely important with the advancement of electric vehicles. The most important problems are the economic issue and too long charging. The fast charging process is highly needed, while excessively high currents significantly affect the condition of the battery. It should be noted that the condition of the battery depends on the chemistry of the battery (including electrode materials, electrolyte, and separator). In addition, charging cycles, duty cycles, temperature conditions as well as SoC (state of charge) conditions have a great influence.
The model of a lithium-ion cell with 3 parts: electrochemical, thermal, and aging is described in the literature [45, 46]. Electrochemical modeling is closely related to the electrochemical reactions that take place during the operation of the cell. The advantage of this model is the observation of the present processes taking place in the cell. The disadvantage is the need to use Computational Fluid Dynamics (CFD) frameworks. For LIB, models such as SP (single particle), extended SP, P2D (Pseudo-Two-Dimensional) are used [45, 46].
Other methods can be found in the literature, using other systems to determine the resistances in the cell. Figure 7 shows the electrothermal aging model. Rct can be determined from the linearization of the Butler-Volmer equation. SoCpow is the lithium concentration at the surface of the particles. The electrical system model captures the low-frequency diffusion dynamics (BMS operating frequency range) with high accuracy. The aging model shows the dependence of the degradation mechanism as a function of SoC, temperature, and the C factor (charging or discharging current normalized to the cell's capacity). This makes it possible to distinguish between the different aging mechanisms and assess the contribution of each to the overall capacity decay. This useful information can be used to develop optimized charging processes and for this purpose validation tests are used. The authors of cited research intend to use this model to optimize the fast charging profile and model-based intelligent charging control [46, 47].
The designed thermal model with electric coupling, based on [45]
Kinetics of the process of charge transfer through the interface electrolyte/electrode, both for the lithium electrode and the remaining electrodes, can be characterized based on the resistance of the transition reaction (Rct) and the exchange current density, and the value of the rate constant of this process.
The impedance spectra for the material are presented in Fig. 8. The spectra are different, however, they have a common feature in the presence of a semicircle at high frequencies and a straight line at low frequencies.
The change in the angle of inclination increases and slightly resembles a curve similar to a capacitor cell. Diffusion was determined on the basis of the Warburg curve fit and it limits the charge exchange (Fig. 8).
The line impedance area occurs at low frequencies. The transition reaction and the formation of the SEI layer had almost no effect on the kinetics of the lithium ion intercalation reaction. It can also be assumed that the area of real resistance close to 80 Ω may be related to the electrode reactions and to the separator. They lead to diffusion and accumulation of lithium ions after crossing the diffusion boundary area. Thus, the diffusion and accumulation of Li+ ions have a significant influence on the kinetics of the reaction, while the resistance of the electrolyte is neglected. When comparing the GNPS impedance spectra (Fig. 7c, d), it should be noted that the actual resistance values are higher than the impedance, which may indicate a slower kinetics of the lithium ion intercalation reaction. Comparing the graphs for the anode made of graphene and starch after carbonization (Fig. 8a, b), we can see that the clear influence of temperature on the decreasing charge transfer resistance in the case of the anode made of biomaterial. All ranges clearly decrease with increasing temperature.
Larger grain size allows for a significant extension of the diffusion path and incomplete overcoming of the charge transfer resistance, at the expense of increasing conductivity. The curves obtained are characteristic of electrodes consisting of irregular particles. Different impedance waveforms can be related to surface adsorption, film formation, reduction–oxidation processes.
The mid-frequency area (semicircle in the Nyquist plot) is related to the charge transfer across the electrolyte/electrode interface. A straight line at low frequencies may be responsible for the diffusion processes taking place in the material [48]. In this case, the Warburg impedance has been replaced with the Warburg impedance finite-length element—GFW) [49, 50]. A significant decrease in the resistance can be observed with increasing temperature (Table 3).
The aim of improving the properties of electrode materials used in lithium batteries is to obtain materials. Reducing the particle size shortens the lithium diffusion path and facilitates the transport of lithium ions and electrons inside them (increased ion and electron conductivity). Therefore, it was decided to compare these values for different carbon materials (Table 4). The fragmentation of the material to the nanometric scale increases its specific surface, and thus the contact surface between the material electrode and the electrolyte. The number of active sites and the transport of reagents increases, which increases the speed and efficiency of the electrode reaction (maximum power of the cells). The electrical contact between adjacent grains and with the current collector increases, which reduces the electrical resistance of the cell. The mechanical properties of the material also improve, making it more resistant to changes in volume. In some cases, there is an increase in the redox potential and the emergence of new lithium storage mechanisms in the pores or at the interfaces.
These results mean that the electrode processes in such systems will occur almost instantaneously since all of the depolarizers are located in the diffusion layer. These types of systems are increasingly used in the construction of modern supercapacitors, batteries, and accumulators because one of the significant problems in energy sources is the ability to obtain as much energy as possible per unit of time. while in analytical applications, micro-and ultra-microelectrodes and similar systems operating under diffusion conditions from a limited area, give very high and narrow voltammetric peaks in measurements. For each electrode material, we see a clear increase in the diffusion coefficient as the temperature increases.
Surface diffusion—occurs when there is a concentration gradient of adsorbed molecules along the surface of a solid. Migration along the surface of a solid is possible due to the energetic heterogeneity of the surface. Particle transport can most likely be depicted in this case as a jump of molecules from one adsorption site to another, without breaking away from the surface.
Ionic conductivity is a measure of the ability of ions and other charged components of an electrolyte to move under the influence of an applied electric field and is derived from the mobility of the ions. The mobility of ions, and hence the conductivity of the electrolyte, is related to the concentration of the components and the temperature, as well as to the physical properties of the solvent such as viscosity and dielectric constant. The dielectric constant largely affects the thickness of the solvation layer formed by the solvent molecules surrounding the cation of the dissociated conductive salt. It prevents the cation and anion from reassociating into ion pairs and larger neutral or charged agglomerates. The formation of such a layer takes place in the case of completely dissociated salts, i.e. for dilute solutions and/or systems with a high dielectric constant. As the salt concentration increases, the proportion of associations increases. On the other hand, the anion structure of a lithium salt can affect the association affinity by evenly distributing the negative charge over the atoms in its structure. The viscosity of the solution also affects the mobility of the ions by slowing them down due to intermolecular friction. A common method of measuring conductivity uses electrochemical impedance spectroscopy (EIS) with an electrolyte between the blocking electrodes. The ionic conductivity influences parameters such as the maximum current density. There is it is an important parameter for all portable devices or electric vehicles that require high-power one-time consumption. Current density is expressed as a ratio. The current to the electrode surface is a derivative of the internal resistance of the cell, which includes the resistance of the electrodes, the electrolyte, and the interfacial layer. The element with the smallest conductivity (the highest resistance), which is a cathode with a conductivity of 10−5 S cm−1, limits the maximum current density. However, the conductivity of liquid electrolytes reaching 10−3 S cm−1 and the resistance of the growing passive layer also significantly affect the internal resistance of the cell and depend on the salt, solvent and functional additives used.
In typical liquid organic electrolytes, an SEI layer is formed on the surface of a graphite electrode, which hinders the diffusion of lithium ions. This leads to Li build-up on this layer and the formation of dangerous dendrites, but a lesser extent than with metallic lithium electrodes. To improve the properties of electrode materials used in lithium batteries, efforts are being made to obtain nanocrystalline materials. Particle size reduction shortens the lithium diffusion path and facilitates the transport of lithium ions and electrons inside them (increased ion and electronic conductivity). The fragmentation of the material to the nanometric scale increases its specific surface and thus the contact surface between the electrode material and the electrolyte. The number of active sites and the transport of reagents increases, which increases the speed and efficiency of the electrode reaction (the maximum power of the cell increases). The electrical contact between adjacent grains and with the current collector increases, which reduces the electrical resistance of the cell. The mechanical properties of the material also improve, making it more resistant to changes in volume. In some cases, an increase is observed redox reaction potential and the emergence of new lithium storage mechanisms in pores or at phase boundaries.
Graphene is currently an extensively researched anode material. In recent years, various types of this material have been experimented with, both with graphene itself or with composite materials. There is a lot of research into nanoplates graphene (GNS), graphene sheets (GP), graphene sponge (GF), reduced graphene oxide (RGO), graphene nanoflakes, graphene + carbon nanotubes (CNTs), and many other graphenes [51]. GNS as a material with excellent transport capacity, large area, and excellent thermal and mechanical stability is a frequently tested material for composite electrodes. The addition of graphene can promote electron transport as well as the diffusion of lithium ions anchored in the material structure, and thus improve the efficiency of electrochemical storage of lithium. The composite of GNS with nanocrystalline Fe3O4 results in the improvement of cyclic stability [52]. J. Zhu and colleagues examined the Sn-Co-GNS composite anode [53]. Cell parameters turned out to be satisfactory; the reversible value of the product of current and time for the 1000 mA g−1 current was 726 mAh g−1 and 483 mAh g−1 for the 800 mA g−1 current. On the other hand, the addition of silicon increases the theoretical capacity of the anode even to the value of 4000 mAh g−1, therefore, much attention is paid to this material [54, 55]. Reduced graphene oxide together with porous silicon nanoparticles also show good parameters, for a current of 160 mA g−1 it was obtained of about 900 mAh g−1 [56]. An interesting idea is also a composite of silicon and graphene sheets, which show good stability during the charging/discharging of the cell [57]. Many studies have been devoted to composites of various carbon materials, for example, a composite of graphene, porous carbon, and tin oxide SnO2 [58].
Tables 5 and 6 also include the determined thermodynamic parameters, such as the Gibbs function, the change of entropy and the enthalpy of the thermal reaction of degradation of the carbonized carbon materials. The value of the free enthalpy (> 0) indicates a forced, not spontaneous, enthalpy reaction with an endothermic transformation, i.e. with energy absorption. Entropy values lower than zero also indicate an undesirable nature of the transformation, i.e. with an increase in the number of degrees of freedom. This significantly lowers the entropy of the reaction. A positive enthalpy value indicates that an increase in temperature will shift the equilibrium (according to the law of controversy) of the reaction towards the formation of products.
Chemical thermodynamics with a quantitative description of the energy effects accompanying the changes and the prediction of the possibility of the spontaneous course of any conceived changes, as well as a quantitative description of the systems which, as a result of such changes, reached the state of equilibrium.
Carbon nanotubes (CNTs), thanks to their electrical, physical, and chemical properties, such as good electrical conductivity, and good thermal and mechanical stability [59], can be used in modern Li-ion batteries [60]. The maximum theoretical current density for single-walled CNTs was estimated at 1116 mAh g−1 in LiC2 stoichiometry, which gives the highest density value for carbon materials [59]. The authors report that for the produced single-wall carbon nanotubes, the value of the charge density was Qpractical 1050 mAh g−1 [61]. It is possible to couple CNTs with metals or metal oxides on a nanometric scale, e.g. Sn, Ge, nickel oxide, and copper oxide, which increases the number of charge/discharge cycles, an increase in electrical conductivity, and a reduction in material delamination during the charge/discharge cycles. An example is a composite based on multi-wall carbon nanotubes and MoS2, for which a charge density of 1030 mAh g−1 was obtained in the 60 cycle [59,60,61,62,63,64]. Despite good parameters, it was not possible to introduce CNT-containing anode materials into mass production. It is specially related to the production costs of CNTs themselves [59]. There are several attempts to use graphene to produce anodes, for example [65, 66]. Graphene is characterized by good electrical conductivity, mechanical strength, and low charge transfer resistance [63, 66]. When using a larger number of graphene sheets, it is possible to obtain a density value of 780 mAh g−1 for Li2C6 stoichiometry (adsorption on both sides of the graphene sheet) or 1116 mAh g−1 for LiC2 stoichiometry [66, 67]. The charge density range for the produced graphene in the cited works was 790–1050 mAh g−1.
LIBs utilize reversible electrochemical reactions to convert and store electrochemical energy. To achieve high-rate LIBs performance, it is important to understand the Li-ion and electron transport pathways in the whole battery systems and to increase the diffusion kinetics in the rate-limiting step. Taking discharging process as an example, Li-ion and electron transportation can be identified in the following steps: Li-ion and electron disassociate from the anode material simultaneously and move toward opposite directions via solid-state diffusion; Li-ion approach the electrode/electrolyte interface, and diffuse through the electrolyte; the electron, driven by the higher potential of cathode side, pass through anode particles and their interfaces toward the current collector instead of riding into the electrolyte, and migrate via an external circuit to power a device; electron and Li-ion enter the cathode materials concurrently via solid-state diffusion.
The thermal stability of the cell is limited by the thermal stability of the electrolyte. The electrodes, current collectors, and the housing have a wide temperature range in their applications. Batteries in ordinary portable devices operate at room or ambient temperature, i.e. from approx. −20 to 50 °C for most inhabited climate zones. The lower end of the cell's useful life is determined by the freezing point in the case of a liquid electrolyte, and the upper saturated vapor pressure of the solvent, which cannot cause leakage of the housing. The upper limit is also limited by the irreversible destruction of the passive layer at elevated temperatures. Exposing the cell to elevated temperatures may lead to thermal decomposition of the substances used in its construction. Essential the temperature at which decomposition takes place is as high as possible and the decomposition products are harmless, which is important in extreme situations such as fire. The use of functional additives for electrolytes is also intended to increase the temperature range of electrolyte stability in both directions. The improvement of the thermal stability of the cell is achieved not only by lowering the freezing point or reducing the vapor pressure, but also by shifting the temperature limit upwards, in which the passive layer is irreversibly damaged.
Conclusions
The Nyquist plots consisted of a semicircle resulting from the load-carrying resistance at a high average frequency area and an inclined line assigned to the diffusion of ions in the low-frequency area. It is seen that the diameter of the first semicircle was much smaller than that of the second, suggesting that the GNPS electrode would have lower contact and charge transfer resistances which can be mainly attributed to the higher carbon content in the submicron sphere. Here, the graphitized carbon in cs can play the role of an efficient charge carrier and promote charge transfer at the interface, which can make the electrode have better electron conductivity and lower resistance.
CSC shows a lower diffusion activation energy barrier (37.37–39.95 kJ mol−1) compared to G(43.80–46.38 kJ mol−1). 5GNPs and 25GNPs exhibited greater differences in the activation energies (30.73–51.43 kJ mol−1 and 29.78–42.52 kJ mol−1 for 5GNPs and 25GNPs, respectively). Diffusion coefficients decrease with increasing intercalation temperature and reach values of 7.73‧10−9–2.38‧10−8 cm2 s−1 for CSC and 4.53‧10−11–2.16‧10−10 cm2 s−1, respectively (towards higher temperatures) for G. Moreover these values for 5GNPs and 25GNPs were in the range of 2.19‧10−17–5.54‧10−17 cm2 s−1and 8.14‧10−16–2.86‧10−15 cm2 s−1, respectively. Thus, the temperature has a significant influence on the diffusion process which is relatively complex. As the temperature rises, the SEI layer resistance decreases. Moreover, kinetic control due to SEI, electrolyte, and charge transfer resistance is a more restrictive process for CSC than for G anode. It is due to the higher energy barrier for these processes compared to satisfactory diffusion kinetics. Thermodynamic parameters indicate that the process is forced and endothermic. Consequently, CSC shows better cyclic performance at elevated temperatures compared to the G anode.
Data Availability
The research data was performed at the Poznań University of Technology. Please contact the corresponding author for details. They are not posted on any page.
References
McCreery, R.L.: Chem. Rev. 108, 2646–2687 (2008)
Frycz, C.A., Shui, X., Chung, D.D.L.: J. Power Sources 58, 41–45 (1996)
Czerwinski, A.: Akumulatory baterie ogniwa; Wydawnictwo Komunikacji i Łączności, ISBN: 978-83-206-1564-7, 1–188 (2005)
Sayilgan, E., Kukrer, T., Civelekoglu, G., Ferella, F., Akcil, A., Veglio, F., Kitis, M.: Hydrometallurgy 97, 158–166 (2009)
Neburchilov, V., Wang, H., Martin, J.J., Qu, W.: J. Power Sources 195, 1271–1291 (2010)
Cao, D., Sun, Y., Wang, G.: J. Power Sources 167, 250–257 (2007)
Dicks, A.L.J.: J. Power Sources 156, 128–141 (2006)
Li, W., Dahn, J.R., Wainwrigbt, D.S.: Science 264, 1115–1118 (1994)
Gilmartin, M.A.T., Hart, J.P.: Sensing with chemically and biologically modified carbon electrodes. A review, Analyst, 120, 1029–1044 (1995)
Willner, I., Yan, Y.M., Willner, B., TelVered, R.: Fuel Cells 9, 7–24 (2009)
Besteman, K., Lee, J.O., Wiertz, F.G.M., Heering, H.A., Dekker, C.: Nano Lett. (2003). https://doi.org/10.1021/nl034139u
Jacobs, C.B., Peairs, M.J., Venton, B.J.: Anal. Chim. Acta 662, 105–127 (2010)
Gasser, M., Rothen-Rutishauser, B., Krug, H.F., Gehr, P., Nelle, M., Yan, B., Wiek, P.J.: NanoBiotechnology 8, 31 (2010)
Falcao, E.H.L.: Wudl F. 531, 524–531 (2007)
Zhang, S., Zhou, J., Wang, Q., Chen, X., Kawazoe, Y., Jena, P.: Proc. Natl. Acad. Sci. 112, 2372–2377 (2015)
Lin, P.C., Wu, J.Y., Liu, W.R.: Green and facile synthesis of few-layer graphene via liquid exfoliation process for lithium-ion batteries. Sci. Rep. 8(1), 9766 (2018)
Xiao, P., Lv, T., Chen, X., Chang, Ch.: LiNi0.8Co0.15Al0.05O2: enhanced electrochemical performance from reduced cationic disordering in li slab. Sci. Rep. 7(1), 1408 (2017)
Lewandowski, A., Biegun, M., Galinski, M., et al.: Kinetic analysis of Li|Li+ interphase in an ionic liquid electrolyte. J Appl Electrochem 43, 367–374 (2013). https://doi.org/10.1007/s10800-012-0515-0
Yu, Z., Yiang, H., Gu, D., Li, J., Wang, L., Shen, L.: A new way to prepare MoO3/C as anode of lithium ion battery for enhancing the electrochemical performance at room temperature. J. Electrochem. Sci. Technol. 7(2), 170–178 (2016)
Boroujeni, S.M., Birke, K.P.: Study of a li-ion cell kinetics in five regions to predict li plating using a pseudo two-dimensional model. Sustainability 11, 6392 (2019). https://doi.org/10.3390/su11226392
Harris, K.R.: Relations between the fractional stokes-Einstein and Nernst-Einstein equations and velocity correlation coefficients in ionic liquids and molten salts. J. Phys. Chem. B 114, 9572–9577 (2010). https://doi.org/10.1021/jp102687r
Hansen, J.P., McDonald, I.R.: Statistical mechanics of dense ionized matter. IV. density and charge fluctuations in a simple molten salt. Phys. Rev. A 11, 2111–2123 (1975). https://doi.org/10.1103/PhysRevA.11.2111
Nitzan, A.: Chemical dynamics in condensed phases: relaxation, transfer and reactions in condensed molecular systems; Oxford graduate texts. Oxford University Press, Oxford, New York (2006)
Altenberger, A.R., Friedman, H.L.: Theory of conductance and related isothermal transport coefficients in electrolytes. J. Chem. Phys. 78, 4162–4173 (1983). https://doi.org/10.1063/1.445093
Zhong, E.C., Friedman, H.L.: Self-diffusion and distinct diffusion of ions in solution. J. Phys. Chem. A 92, 1685–1692 (1988). https://doi.org/10.1021/j100317a059
Padró, J., Trullàs, J., Sesé, G.: Computer simulation study of the dynamic cross-correlations in liquids. Mol. Phys. 72, 1035–1049 (1991). https://doi.org/10.1080/00268979100100761
Chowdhuri, S., Chandra, A.: Molecular dynamics simulations of aqueous NaCl and KCl solutions: effects of ion concentration on the single-particle, pair, and collective dynamical properties of ions and water molecules. J. Chem. Phys. 115, 3732–3741 (2001). https://doi.org/10.1063/1.1387447
Kashyap, H.K., Annapureddy, H.V.R., Raineri, F.O., Margulis, C.J.: How is charge transport different in ionic liquids and electrolyte solutions? J. Phys. Chem. B 115, 13212–13221 (2011). https://doi.org/10.1021/jp204182c
Hansen, J.P., McDonald, I.R.: Theory of simple liquids, pp. 403–454. Elsevier, Oxford, U.K (2013)
Gong, J., Zhou, Z., Sumathy, K., Yang, H., Qiao, Q.: Activated graphene nanoplatelets as a counter electrode for dye-sensitized solar cells. J. Appl. Phys. 119(13), 135501 (2016)
Bakierska, M., Chojnacka, A., Świętosławski, M., Natkański, P., et al.: Multifunctional carbon aerogels derived by sol-gel process of natural polysaccharides of different botanical origin. Materials 10(11), 1336 (2017)
Zhang, J., Weng, W., Yang, J., Yang, L., et al.: Lithium-ion battery fiber constructed by diverse-dimensional carbon nanomaterials. J. Mater. Sci. 54(1), 582 (2019)
Tian, Y., Yuan, P., Yang, F., Gu, J., Chen, M., Tang, J., Su, Y., et al.: Research on the principle of a new flexible screw conveyor and its power consumption. Appl. Sci. 8, 1038 (2018)
Melezhik, A.V., Pershin, V., Memetov, N., Tkachev, A.G.: Mechanochemical synthesis of graphene nanoplatelets from expanded graphite compound. Nanotechnol. Russ. 11, 421 (2016)
Kurniawan, R., Utomo, J., Fibriyanti, A.A., et al.: Formation of graphene island on si (100) substrate prepared by simple-spray method: morphological and optical analyses. IOP Conf. Series Mat. Sci. Eng. 515, 012019 (2019)
Xue, Z., Chen, P., He, L., et al.: Preparation of potato starch-based carbon particles by low-temperature carbonization in oil. Int. J. Mater. Res. 106(11), 1196 (2015)
Nowak, A., Hagberg, J., Leijonmarck, S., Schweinebarth, H., et al.: Lignin-based carbon fibers for renewable and multifunctional lithium-ion battery electrodes. Holzforschung 72(2), 81 (2018)
Warren, B.E.: X-ray diffraction. Addison-Wesley Publishing Co, Boston, MA, USA (1969)
Tenhaeff, W.E., Rios, O., More, K., McGuire, M.A.: Highly robust lithium-ion battery anodes from lignin: an abundant, renewable, and low-cost material. Adv. Funct. Mater. 24, 86 (2014)
Zhu, Y.-E., Gu, H., Chen, Y.-N., et al.: Hard carbon derived from corn straw piths as anode materials for sodium-ion batteries. Ionics 24(4), 1075 (2017)
Simon, M., Benitez, A., Caballero, A., Morales, J., Vargas, O.: Untreated natural graphite as a graphene source for high-performance li-ion batteries. Batteries 4, 13 (2018)
Lee, HCh., Liu, W.W., Chai, S.-P., et al.: Review of the synthesis, transfer, characterization and growth mechanisms of single and multilayer graphene. RSC Adv. 7(26), 15644 (2017)
Liu, W.W., Chai, S.P., Mohamed, A.R., Hashim, U.: Synthesis and characterization of graphene and carbon nanotubes: a review on the past and recent developments. J. Ind. Eng. Chem. 20(4), 1171 (2014)
A. Antidormi, L. Colombo, S. Roche. (2021). Emerging properties of non-crystalline phases of graphene and boron nitride based materials Nano Materials Science (In Press)
Mohajer, S., Sabatier, J., Lanusse, P., Cois, O.: Electro-thermal and aging lithium-ion cell modelling with application to optimal battery charging. Appl. Sci. 10, 4038 (2020)
Mohajer, S., Sabatier, J., Lanusse, P., Cois, O.: A fractional-order electro-thermal aging model for lifetime enhancement of lithium-ion batteries. IFAC-PapersOnLine 51(2), 220–225 (2018)
Zhou, Ch., Manzie, Ch., Nesić, D.: Model predictive control for lithium-ion battery optimal charging. IEEE/ASME Trans. Mechatron. 23(2), 947–957 (2018)
Funabiki, A.: J. Electrochem. Soc. 145, 172 (1998)
MacDonald, J.R., Johnson, M.B.: Impedance spectroscopy: emphasizing solid materials and systems. Wiley-Vch, New York (1987)
Wang, C., Appleby, A.J., Little, F.E.: Electrochim. Acta 46, 1793 (2001)
Lewandowski, A., Swiderska-Mocek, A., Rudnicka, E., Jakobczyk, P.: J. Solid State Electrochem. 18, 2781 (2014)
Ren, M., Yang, M., Liu, W., Li, M., Su, L., Qiao, C., Wu, X., Ma, H.: Electrochim. Acta 194, 219 (2016)
Zhu, J., Wang, D., Liu, T., Guo, C.: Electrochim. Acta 125, 347 (2014)
Kim, S., Yook, S., Kannan, A.G., Kyung, S., Park, C., Kim, D.: Electrochim. Acta 209, 278 (2016)
Sun, W., Hu, R., Zhang, M., Liu, J., Zhu, M.: J. Power Sources 318, 113 (2016)
Jiao, L.S., Liu, J.Y., Li, H.Y., Wu, T.S., Li, F., Wang, H.Y., Niu, L.: J. Power Sources 315, 9 (2016)
Jiang, H., Zhou, X., Liu, G., Zhou, Y., Ye, H., Liu, Y., Han, K.: Electrochim. Acta 188, 777 (2016)
Miao, C., Liu, M., He, Y.-B., Qin, X., Tang, L., Huang, B., Li, R., Li, B., Kang, F.: Energy Storage Mater. 3, 98 (2016)
Goriparti, S., Miele, E., De Angelis, F., Di Fabrizio, E., Proietti Zaccaria, R., Capiglia, C.: J. Power Sources 257, 421–443 (2014)
Kang, C., Patel, M., Rangasamy, B., Jung, K.-N., Xia, C., Shi, S., Choi, W.: J. Power Sources 299, 465 (2015)
Dileo, R.A., Castiglia, A., Ganter, M.J., Rogers, R.E., Cress, C.D., Raffaelle, R.P., Landi, B.J.: ACS Nano 4, 6121 (2010)
Wang, W., Gu, L., Qian, H., Zhao, M., Ding, X., Peng, X., Sha, J., Wang, Y.: J. Power Sources 307, 410 (2016)
Stankovich, S., Dikin, D.A., Dommett, G.H.B., Kohlhaas, K.M., Zimney, E.J., Stach, E.A., Piner, R.D., Nguyen, S.T., Ruoff, R.S.: Nature 442, 282 (2006)
Simon, P., Gogotsi, Y.: Nat. Mater. 7, 845 (2008)
Chen, S., Chen, P., Wu, M., Pan, D., Wang, Y.: Electrochem. Commun. 12, 1302 (2010)
Ren, Y., Wei, H., Huang, X., Ding, J.: Int. J. Electrochem. Sci. 9, 7784 (2014)
Yin, S., Zhang, Y., Kong, J., Zou, C., Li, C.M., Lu, X., Ma, J., Boey, F.Y.C., Chen, X.: ACS Nano 5, 3831 (2011)
Funding
This work was supported by the Polish Ministry of Science and Higher Education, Grant No. 0911/SBAD/2202.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Pigłowska, M., Kurc, B. & Rymaniak, Ł. Modeling of Diffusion of Metal Ions in Carbon Electrodes for Lithium-Ion Cells. Waste Biomass Valor 14, 2401–2417 (2023). https://doi.org/10.1007/s12649-022-01974-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12649-022-01974-9