Compassion and envy in distributional comparisons

Abstract

Normative-based distributional comparisons across countries and over time build upon the assumption that individuals are selfish. However, there is a consolidated evidence that individuals also care about what others have. In this paper, we propose a framework for comparing and ranking distributions that includes non-individualistic possibilities. Specifically, we consider ranking criteria that account, in one case, for the feeling of compassion and, in the other case, for the feeling of envy. These feelings are generated respectively by those having lower resources and those having higher resources. We illustrate our framework using CNEF data for Australia, Korea, Germany, Switzerland, and the US and show that accounting for the presence of compassion and envy might lead to different welfare rankings.

1 Introduction

Since the pioneering works of Kolm (1969) and Atkinson (1970), the derivation of normative-based partial ranking criteria for distributional evaluations has become a prominent topic in welfare economics. Most of the contributions in this field develop sets of dominance conditions to rank distributions of income according to given classes of social welfare functions and investigate the implications of different assumptions on the form of these functions (see, among others, Atkinson & Bourguignon, 1987; Lambert & Ramos, 2002; Aaberge et al., 2013). Such criteria are widely adopted to evaluate the performances of societies. For instance, they have been used to study the normative and distributive effects on the population of periods or sustained growth or recession, as in the case of the 2007 financial crisis followed by the crisis of the sovereign debts in 2011. These models have also been implemented to evaluate the effects of the introduction of public policies and tax reforms (see, among others, Bourguignon, 2011b; Jenkins & Van Kerm, 2016).

A common feature to these models is the ‘self-regarding’ property postulating that a society is composed by agents who only care about the amount of income that they can enjoy. However, there is consolidated evidence, based on experimental and survey data, that the magnitude of the income received by an individual constitutes only part of the relevant information for the assessment of her well-being (see Deaton, 2003; Alpizar et al., 2005; Luttmer, 2005; Ferrer-i Carbonell, 2005; Senik, 2009; Quintana-Domeque & Wohlfart, 2016; Clark et al., 2017). Individuals are not living in isolated islands, in most cases individuals’ well-being is unavoidably related to the (complex) society in which they live and the way they evaluate their own achievements strictly depends on the achievement of others (see also Corazzini et al., 2012). Hence, individuals have other-regarding preferences, that is, they care about their own income and the income levels enjoyed by other individuals in the same society.

The literature has provided theoretical foundations for the presence of relative concerns and their impact on individuals’ well-being (see, among others, Cole et al., 1992; Fehr & Schmidt, 1998). The essential idea of relative concerns is that individual economic welfare depends (at least in part) on how the individual is doing relative to a set of comparators in society. Several models of other-regarding preferences have been proposed (see for a survey Dhami, 2016). Some theories of relative concerns predict negative welfare effects when friends and neighbours become better-off. For instance models of ‘pride’ assume that any improvement for others, either richer or poorer, has a negative effect on own utility (Friedman, 2005). By contrast, other theories predict a negative welfare effect only when richer individuals get richer: these are the models of ‘envy’; whereas, a welfare improvement experienced by more disadvantaged individuals has a positive effect on own utility: these are the models of ‘compassion’ (Bolton & Ockenfels, 2000; Fehr & Schmidt, 1998). It is on these two classes of models that we focus on in this paper.

Specifically, we address the issue of how different distributions of income should be compared from the perspective of a social planner knowing that individuals are characterized by feelings of envy or compassion. The first step in our analysis consists in building a social welfare function expressing these ethical concerns, namely aversion to the feeling of compassion and envy. The concept of compassion can be formulated by considering those individuals that fall in the bottom part of the distribution—the disadvantaged part of the population toward which the richer feel compassionate. In a specular way, the concept of envy will be formulated by considering those individuals that sit in the upper part of the distribution—the advantaged part of the population that represents a source of envy for the rest of the population. We will exploit these basic intuitions and we will translate them into formal properties that will be imposed on the social evaluation functions. Analytically, we will characterize partial orderings defined over income distributions that will be coherent with social preferences endorsing aversion either to the feeling of envy or to the feeling of compassion. In our framework, the sources of compassion and envy would disappear with a perfectly equal distribution. A social planner overlooking such feelings would not make use of the full-set of information available in the design of welfare-maximizing policies.

In addition to the literature on game theory and experimental economics mentioned above. Our work is related to other two branches of the literature. From one side, it is related to that part of the literature that deals with the issue of aggregating preferences when envy and compassion impact individual utilities (see, among others, Kolm, 1995; Schmidt & Wichardt, 2019; Weinzierl, 2017). The implications of using utility functions with such characteristics have also been investigated in the context of designing optimal taxation models (see, for instance, Bruce & Peng, 2018; Kanbur & Tuomala, 2013; Støstad & Cowell, 2019). Our work presents a distinctive feature with respect to this related literature: while these models are either based on aggregated indices of social welfare or framed into the optimal income tax model à la Mirlees, we use a partial dominance approach, which is more in line with the social choice tradition and allows for a more robust ethical assessment (see Atkinson & Bourguignon, 1987; Zoli & Lambert, 2012; Palmisano & Petrillo, 2021).

Most importantly, this work is related to that part of the literature in normative economics that proposes indices of relative deprivation and satisfaction that can be considered direct proxies of the extent of envy and compassion in a society (see, for instance, Runciman, 1966; Chakravarty, 1997; Moyes, 2007; Magdalou & Moyes, 2009; Esposito, 2018). Now, while all these contributions develop different methodologies to assess either relative deprivation or satisfaction, we instead provide welfare ranking criteria that account for aversion either toward the feeling of compassion or toward the feeling of envy in the society; hence, we assume that this information is normatively relevant.

Specifically, in our framework, compassion will correspond to a welfare loss imposed on the society and generated by the sufferance that arises in the presence of individuals that are deemed to be disadvantaged, since they are not as well-off as others in the same society—the advantaged part of the population that feels the pain of compassion. This loss will be higher the lower is the level of income that goes to the disadvantaged recipients. Thus, this notion of compassion considers altruistic individuals, whose utility is increasing not only in their own level of income but also in the level of income enjoyed by the disadvantaged segment of the population. The feeling of compassion is then quantified by focusing on a measure of cumulated incomes. It can then be inferred that the existence of disadvantaged individuals in a society might bring about to a sentiment of compassion in the rest of the community and might, therefore, constitute the basis of social judgement (see also Chakravarty & Moyes, 2003; D’Ambrosio, 2018).

By contrast, envy will refer to the welfare loss imposed on the society because of the presence of individuals that are judged to be the advantaged, as they are better-off than others in the same society—the disadvantaged part of the population that bears the cost of envy. This implies that the society is composed by envious individuals, whose utility increases with their own level of income but decreases with the level of income of those that are already better-off. The feeling of envy is then quantified by focusing on the distance between the income of each worse-off individual and the average income benefited by the advantaged segment of the society.

In our model, compassion and envy are symmetrical notions. They are both generated from the frustration the individual experiences by comparing her own economic resources with those owned by others in the same society. However, in the case of compassion, the relevant comparators are the individuals classified as disadvantaged; in the case of envy, the relevant comparators are those classified as advantaged. Both of them arise to be normatively relevant and, therefore, should be accounted for in normative evaluations. In fact, these feelings generate negative externalities being potential sources of conflicts between individuals, and are likely to exacerbate tensions in the society. A society realizing perfect equality would eliminate the sources of compassion and envy. But the choice of the path to undertake in order to achieve such equality will depend on whether the social planner is more prone to reduce compassion or envy (see also Cowell & Flachaire, 2022; Chakravarty & Mukherjee, 1999).

We adopt our theoretical framework to make pairwise welfare comparisons considering five different countries—namely Australia, Germany, Korea, Switzerland, and the U.S.—and to evaluate the evolution of each of these countries between 2001 and 2015. We find that Switzerland, followed by US, surfaces as the best-performing country, that is, its distribution turns out to be the dominating one in the largest number of pairwise comparisons. On the other hand, Korea, followed by Germany and Australia, turns out to be the worst-performing, that is, its distribution turns out to be the dominated one in most of the pairwise comparisons considered. We also show that implementing evaluation criteria that include aversion toward the feeling of envy or compassion may lead to a different assessment of the distributions under analysis. Thus, the empirical analysis gives further support to the relevance of adopting our framework for distributional comparisons.

The rest of the paper is structured as follows. Section 2 presents the theoretical framework and provides a set of criteria that can be adopted for making distributional comparisons. Section 3 provides an empirical illustration. Section 4 concludes.

2 The framework

2.1 The setup

Let a distribution of individual incomes, y, be represented by its cumulative distribution function (cdf) denoted by F(y) and let $\mathcal {F}$ be the set of all such cumulative distributions. We are interested in judging F from a normative perspective. We assume that a social planner is endowed with preferences over such income distribution denoted by W(F). To represent such preferences, we resort to the rank-dependent model proposed by Yaari (1987).¹ It assumes that the welfare derived from a risky distribution can be written as a weighted average of all possible realizations, where the weights are a function of the rank of the realization in the distribution.² Thus, let $p\in$ $\left[ 0,1\right]$ denote the rank in the income distribution F(y), so that $F^{-1}(p)=\inf \left\{ y: F(y) \ge p \right\}$ represents the inverse of the cdf F, that is, the level of income benefited by the individual ranked p in F. The social preference over such income distribution can be defined as follows:

$$\begin{aligned} W(F)=\int _0^1\omega (p) F^{-1}(p)dp \end{aligned}$$

(1)

where $\omega (p) \ge 0 \ \forall p \in [0,1]$ is a differentiable function, such that $\omega (p)=f'(p) \ \forall p \in [0,1]$ with f continuous and increasing so that $f(0)=0$ and $f(1)=1$. The function $\omega (p)$ can be interpreted as a preference function of a social planner that assigns weights to the incomes of each agent in the distribution according with her relative position in that distribution. By analysing the functional form of $\omega (p)$, it is possible to infer the distributional attitudes of a social planner adopting W(F) to compare states of the world.

Then, the evaluation of a given society will be the result of a weighted aggregation across individuals, where the weighting scheme is constructed upon the relative position of individuals in the income distribution. Restrictions on weights will define different classes of social welfare functions, characterized by different normative implications. Hence concerns for compassion and envy, separately, will be expressed throughout such restrictions.

Now, if the functional form of $\omega (p)$ was known, we could directly check welfare dominance between the different income distributions compared. In practice, this is not possible. Hence, we need to reformulate the dominance expressed in terms of W in a dominance expressed in terms of a restriction that involves only the observables, i.e. the income distributions under alternative states of the world. This can only be realized by imposing restrictions on the class of social welfare functions denoted by Z, defined as follows:

$$\begin{aligned} \begin{aligned} Z&=\{ W: \omega (p)=f'(p) \ \text {continuous and differentiable on} \ [0,1], \\&\quad f'(p) >0 \ \forall p \in [0,1], f(0)=0 \ \text {and} \ f(1)=1 \}. \end{aligned} \end{aligned}$$

Hence, our departing point is a class of rank-dependent social evaluation functions, which are explicitly sensitive to information about the relative position of individuals. This is consistent with the increasing interest given in the economic literature on the role of individual economic positions as indicator of social status, and complements the growing discussion about the formal integration of other-regarding preferences into a normative measurement model (see Decerf & Van der Linden, 2016; Treibich, 2019). Other-regarding preferences, in fact, acting as externalities could lead to substantive welfare gains or losses. It is, therefore, essential to understand how they should be incorporated into normative evaluations. Indeed, the rank of each agent in the distributions of income (or of other general economic resources) in her society, represents a standard proxy of her social status and plays an important role in her appraisal of her own welfare and the welfare of the whole society. The relevance of the rank suggests that attitudes of envy and compassion are important components of individual judgements and cannot be overlooked when assessing alternative states of the world (Weiss & Fershtman, 1998).

Before illustrating the results, it is useful to clarify the notion of compassion and envy endorsed in this model. Compassion considers altruistic individuals that evaluate their own welfare on the basis of two components: their income and the income of the disadvantaged individuals, that is, those individuals whose relative position in the income distribution falls below a given threshold. Both components act by increasing the utility of altruistic individuals who, thus, feel compassionate with respect to the disadvantaged agents. The sentiment of compassion that arises overall in the society constitutes the basis of social judgement. Because both components positively affect the utility of altruistic individuals, compassion can be quantified on the basis of the cumulative effect of the income of the disadvantaged individuals on the income of the advantaged altruistic individual.

Also envious individuals evaluate their welfare on the basis of two components. The first is identical to the case of altruistic individuals, that is, their own income. The second corresponds to the income of the advantaged individuals, that is, those individuals whose relative position in the income distribution is found above a given threshold. The utility of an envious individual is increasing in the first component but decreasing in the second. Envy can then be quantified on the basis of the distance between the income of each worse-off individual and the average income benefited by the advantaged segment of the society.

Hence, envy has to do with the frustration an agent experiences because some other individuals are in a better position than she is, while compassion arises from becoming aware of the existence of individuals in worse situations.

2.2 Ranking criteria

The first ranking criterion we propose is formalized in the following Proposition 1 and provides a robust method to compare alternative states of the world when the feeling of compassion is accounted for in the normative assessment.³

Proposition 1

Given two income distributions F and $G\in \mathcal {F}$ and a threshold percentile $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_1=\{W: W \in Z, f''(p) \le 0 \ \forall \; p \in [0,\bar{p}], f''(s) = 0 \ \forall \; s \in [\bar{p}, 1]\}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Phi (p) \ge 0 \ \forall \; p \in [0,\bar{p}]\\{} & {} (ii) \;\Delta \mu \ge 0 \end{aligned}$$

where $\Phi (p)=\int _0^p [F^{-1}(q) - G^{-1} (q)] dq$ and $\Delta \mu =\int _0^1 [F^{-1}(p) - G^{-1} (p)] dp$.

According to Proposition 1, in order to judge one distribution as superior to another distribution two conditions need to be satisfied. The first is a second order inverse stochastic dominance condition that must hold for every percentile of the distribution up to $\bar{p}$. This dominance means that the cumulated income in the dominant distribution must be nowhere lower than the cumulated income in the dominated distribution up to the threshold level $\bar{p}$. The second condition requires checking that the average income of the dominant distribution is not lower than the average income of the dominated distribution. This proposition is based on the ideal that the higher the income of the more disadvantaged part of the society the lower will be the feeling of compassion suffered by individuals the more socially desirable will be a distribution, by safeguarding at the same time efficiency through condition (ii). The distinction between the two parts of the distribution depends on the value of the threshold $\bar{p}$ that we assume it is defined exogenously.⁴

This Proposition formalizes the ranking criterion that a social planner with particular preferences should adopt. These preferences encompass a common monotonicity (or efficiency) property represented by $\omega (p)=f'(p) \ge 0$ for all $p \in [0,1]$, according to which an increase in individual income never reduces social welfare, independently on where the individual sits in the distribution, and a property that we define as aversion to the feeling of compassion and that is obtained by imposing $f^{''}(p) \le 0$ $\forall \; p \in [0,\bar{p}]$, $f^{''}(s) = 0$ $\forall \; s \in [\bar{p}, 1]$, which implies that $f'(p) \ge f'(s) \ge 0$ $\forall \; p \in [0, \bar{p}]$ and $\forall \; s \in [\bar{p},1]$ such that $0\le p \le \bar{p} \le s \le 1$. This property, focusing on the part of the distribution encompassed between 0 and $\bar{p}$, introduces a concern for the disutility generated by the compassion suffered by those individuals ranked above $\bar{p}$. Technically this property says that the marginal effect of a reduction of income on social welfare is higher the poorer is the individual and hence the higher the feeling of compassion that is generated among those richer than him. Whereas, the marginal effect of a reduction in income is constant for those that are not considered as disadvantaged and hence that do not represent a source of compassion. Hence, a progressive transfer between individuals ranked above $\bar{p}$ does not have any effect on social welfare, whereas a progressive transfer from an individuals ranked above $\bar{p}$ to an individual ranked below, as well as a progressive transfer between individuals both ranked below $\bar{p}$, reduces the feeling of compassion and may increase social welfare. This is the case of designing a policy intervention that must account for the potential creation of negative externalities in the society, through acting especially on alleviating the economic hardship of the most disadvantaged individuals.

Special cases of Proposition 1 are obtained by selecting two particular values for the threshold $\bar{p}$. That is, for $\bar{p}=1$ Proposition 1 boils down to a standard second order inverse stochastic dominance condition, corresponding to a situation of pervasive compassion in the distribution. Whereas, for $\bar{p}=0$ Proposition 1 boils down to a dominance test applied on the average income of the two distributions, corresponding to a situation of absence of compassion. A third special case worth emphasizing is obtained by assuming $\bar{p} \rightarrow 0$ and $\omega (s) = f(s)'=0 \ \forall \; s \in [\bar{p}, 1]$ and is formalized in Remark 1.

Remark 1

Given two income distributions F and $G\in \mathcal {F}$ and a threshold percentile $\bar{p} \rightarrow 0$, $W(F)\ge W(G)$ $\forall \; W \in Z_1^*=\{W: W \in Z, f''(p) \le 0 \ \forall \; p \in [0,\bar{p}], f'(p) \ge f'(s) = 0 \ \forall \; p \in [0, \bar{p}], \forall s \in [\bar{p},1]\}$ if and only if

$$\begin{aligned} \; \Phi (p) \ge 0 \ \forall \; p \in [0,\bar{p}]. \end{aligned}$$

The class of social welfare functions considered in Remark 1 is consistent with the preferences of a social planner valuing an income distribution socially desirable if and only if the most disadvantaged individuals experiences an increase in income, which echoes back the maximin criterion à la Rawls.

It is interesting to note that the SWF referred to in Proposition 1 is consistent with individual utility functions that are separable on individual income and the income of all other individuals with lower income, as expressed below:

$$\begin{aligned} U(q)=\alpha (q) y(q)+\int _{0}^{q}\beta (p)y(p)dp \; \forall \; q \in [0,1] \end{aligned}$$

(2)

with $\alpha (q), \beta (p) \ge 0$, $\frac{\delta \alpha (q)}{\delta p}, \frac{\delta \beta (p)}{\delta p} \le 0$, and $\omega (p)=\alpha (p)+ (1-p) \beta (p)dp$. This complements well-known results in Lambert (2003) and Schmidt and Wichardt (2019) outlining the relationship between relative concerns and social welfare in the case of utilitarian social welfare functions.⁵

The second ranking criterion we propose is formalized in the following Proposition 2 and provides a robust method to compare alternative states of the world when the feeling of envy is accounted for in the normative assessment.

Proposition 2

Given two income distributions F and $G \in \mathcal {F}$ and a threshold percentile $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_2=\{W:W \in Z, f''(s) \le 0 \ \forall \; s \in [\bar{p}, 1], f''(p) = 0 \ \forall \; p \in [0,\bar{p}]\}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Xi (s) \ge 0 \ \forall \; s \in [\bar{p}, 1]\\{} & {} (ii) \; \Phi (\bar{p}) \ge 0 \end{aligned}$$

where $\Xi (s)=\int _{\bar{p}}^s \left[ F^{-1}(t) - G^{-1} (t) \right] dt$ and $\Phi (\bar{p})= \int _0^{\bar{p}} \left[ F^{-1}(q) - G^{-1}(q)\right] dq$.

Proposition 2 formalizes a ranking criterion that the social planner should apply in case of aversion to the feeling of envy generated by an income distribution. This criterion is composed of two conditions. As in Proposition 1, the first is a second order inverse stochastic dominance condition, but differently from Proposition 1 it must hold for every percentile of the distribution between $\bar{p}$ and 1. The second condition imposes that the average income in the lower part of the distribution, specifically from 0 to $\bar{p}$, cannot be lower in the dominant distribution. The principle underlying this proposition is that the higher the income of the richest individuals the higher will be the feeling of envy in a society. The condition $\omega (p) = f'(p) \ge 0 \forall \; p \in [0,1]$ ensures that an increase in income of one individual, ceteris paribus, does not decrease social welfare. At the same time, the envy aversion endorsed by the social planner is reflected in the structure of the weighting scheme: $f''(s) \le 0$ $\forall \; s \in [\bar{p}, 1]$, $f''(p) = 0$ $\forall \; p \in [0,\bar{p}]$. Such weights, by focusing on the part of the distribution encompassed between $\bar{p}$ and 1 introduce a concern for the feeling of envy that is felt by those individuals ranked below $\bar{p}$. According to these conditions, the marginal effect of an increase of income on social welfare is lower the richer is the individual and hence the lower the feeling of envy that is generated among those poorer than him. Whereas, the marginal effect of a reduction in income is constant for those that are not considered as advantaged and hence that do not represent a source of envy, but it safeguards efficiency in the lower part of the distribution through condition (ii). Hence a progressive transfer between individuals ranked below $\bar{p}$ does not have any effect on social welfare, whereas a progressive transfer between individuals both ranked above $\bar{p}$ reduces the feeling of envy and may increase social welfare. Clearly, for $\bar{p}=1$ and $\bar{p}=0$, Propositions 1 and  2 will coincide. In its role of defining the ideal policies, the social planner must be aware that the economic gains generated in favour of the more advantaged individuals also act as negative externalities imposed on the society. They must be taken into account in elaborating a policy intervention, for instance in case of potential utilitarian gain when that gain is due to a negative welfare externality imposed by envy. Similarly to the argument made above, the SWF referred to in Proposition 2 can be obtained as an aggregation of individual utility functions that are separable in individual income and the income of all other individuals with higher income:

$$\begin{aligned} U(p)=\gamma (p)y(p)-\int _{p}^1\sigma (q)y(q)dq \; \forall \; p \in [0,1] \; \end{aligned}$$

(3)

with $\gamma (p), \sigma (q) \ge 0$, $\frac{\delta \gamma (p)}{\delta p}, \frac{\delta \sigma (q)}{\delta q} \le 0$ and $\omega (p)=\gamma (p)-p \sigma (p)$.

Note that the social preferences considered in Propositions 1 and  2 are reminiscent of the approach to inequality measurement based on the notion of complaints about income distribution, whose philosophical foundations can be traced back to Temkin (1986, 1993). This approach is based on the ideal that each individual may express a complaint when comparing her welfare to that of a reference person or a group in a given society. This is exactly the situation taking place in our framework, where both envy and compassion are oriented towards a group (those below $\bar{p}$ for compassion and those above $\bar{p}$ for envy). Therefore, compassion and envy can be regarded as the weighted sum of these complaints, with weights increasing with the size of the complaint (see also Cowell & Ebert, 2004). Although it has the features of an individualistic approach, the aggregations that are generated are also normatively relevant since—as also argued by Temkin (1986)—the overall complaint in the community represents a form of social bad.

In case of intersection in condition (i) of either Propositions 1 or  2, or if condition (i) and (ii) of either Propositions 1 or  2 result in a conflicting dominance, the two distributions cannot be ranked. Hence, we need to identify the minimal refinement on the set of admissible preferences that allows unambiguous assessments of distributions. This is done in the following two propositions. In particular, one can resort to the test proposed in Proposition 3 if Proposition 1 results in a clash of the distributions compared. Similarly, the test proposed in Proposition 4 can be implemented if it is Proposition 2 that generates incomplete rankings.

Proposition 3

Given two income distributions F and $G\in \mathcal {F}$ and a threshold percentile $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_3=\{W:W \in Z_1, f'''(p) \ge 0 \ \forall \; p \in [0, \bar{p}] \}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Psi (p) \ge 0 \ \forall \; p \in [0,\bar{p}]\\{} & {} (ii) \; \Phi (\bar{p}) \ge 0; \Phi (\bar{p})+\Xi (1) \ge 0 \end{aligned}$$

where $\Psi (p)=\int _0^p \Phi (q)dq$ and $\Xi (1)=\int _{\bar{p}}^1 \left[ F^{-1}(q)-G^{-1}(q)\right] dq$.

Proposition 3 characterizes a ranking criterion based on two conditions. Condition (i) is a (upward) third order inverse stochastic dominance condition up to $\bar{p}$. Condition (ii) is a sequential test composed of two steps. The first step consists in checking the dominance between the average income of the bottom part of the distribution up to $\bar{p}$; it coincides with the last step of condition (i) in Proposition 1. In the second step, the average income of the upper part of the distribution is added, coinciding with condition (ii) of Proposition 1. Differently from Proposition 1, we are restricting the set of preferences for which the dominance must hold to those satisfying $\omega ^{''}(p)=f'''(p) \ge 0$ $\forall \; p \in [0, \bar{p}]$ asking that one should prefer progressive transfers taking place at the very bottom part of the distribution to progressive transfer taking place somewhere above. If the transfer takes place from one individual ranked above $\bar{p}$ to one individual ranked below $\bar{p}$, then one should prefer transfers that benefit individuals that are the poorest among those ranked below $\bar{p}$. Both kinds of transfer that one should prefer are those that focus on individuals whose economic conditions generate the highest feeling of compassion.

Proposition 4

Given two income distributions F and $G\in \mathcal {F}$ and a threshold percentile $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_4=\{W:W \in Z_2, f'''(s) \le 0 \ \forall \; s \in [\bar{p}, 1] \}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Omega (s) \ge 0 \ \forall \; s \in [\bar{p},1]\\{} & {} (ii) \; \Phi (\bar{p}) \ge 0\\{} & {} (iii) \; \Xi (1) \ge 0 \end{aligned}$$

where $\Omega (s)=\int _s^1 \Xi (t)dt$.

Proposition 4 characterizes a ranking criterion based on: (i) downward third order inverse stochastic dominance condition between $\bar{p}$ and 1; (ii) a test on the difference in the magnitude of the mean income of the bottom part of the distribution as for condition (ii) of Proposition 3; (iii) a test on the difference in the magnitude of the mean income of the upper part of the distribution. Differently from Proposition 2, we are restricting the set of preferences for which the dominance must hold to those satisfying $f'''(s) \le 0 \; \forall \; s \in [\bar{p}, 1]$, asking that among all possible progressive transfers that could be implemented in the group of advantaged individuals, one should prefer progressive transfers taking place between individuals ranked very close to 1 to progressive transfer taking place between individuals ranked very close to $\bar{p}$. Such kinds of transfer are those that focus on individuals whose economic conditions generate the highest feeling of envy.

From a technical point of view, it is interesting to notice that while condition (i) of all previous Propositions is based on an upward dominance test, condition (i) of Proposition 4 introduces a dominance test to be implemented through a ‘downward’ procedure, which means that one has to start from the highest percentile, add sequentially the cumulated income corresponding to lower percentiles, and check that the dominance holds at every step of the downward cumulative process.⁶

Last, we propose a generalization of the dominance conditions characterized so far, which considers the advantaged and disadvantage groups as pushed far apart from each other. This implies two thresholds need to be introduced: a lower threshold for identifying the disadvantaged group, denoted by $\underline{p}$, and an upper threshold for identifying the advantaged group, denoted by $\bar{p}$.⁷

Proposition 5

Given two income distributions F and $G\in \mathcal {F}$ and two threshold percentiles $\underline{p}$ and $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_5=\{W:W \in Z, f''(p) \le 0 \ \forall \; p \in [0,\underline{p}], f''(q) =f''(s)= 0 \ \forall \; q \in [\underline{p}, \bar{p}] \ \forall \; s \in [\bar{p}, 1], f'(p) \ge f'(q) \ge f'(s) \}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Phi (p) \ge 0 \ \forall \; p \in [0,\underline{p}]\\{} & {} (ii) \; \Phi (\bar{p}) \ge 0; \Phi (\bar{p}) + \Xi (1) \ge 0 \end{aligned}$$

where $\Phi (p)=\int _0^p [ F^{-1}(q) - G^{-1} (q)] dq$, $\Phi (\bar{p})=\int _0^{\bar{p}}[F^{-1}(p) - G^{-1} (p)] dp$ and $\Xi (1)=\int _{\bar{p}}^1 [F^{-1}(s) - G^{-1} (s) ]ds$.

Proposition 5 derives two conditions that must be satisfied in order to identify the dominant distribution and establish a rank across distributions. The first condition is a second order inverse stochastic dominance condition that must hold for every percentile of the distribution up to the lower threshold $\underline{p}$. It corresponds to condition (i) of Proposition 1 except for the selection of the threshold percentile. The second condition is a sequential test composed of two steps: the first step requires checking that the average income of the individuals ranked up to $\bar{p}$ of the dominant distribution is not lower than the average income of individuals ranked in the same position in the dominated distribution; the second step requires checking the dominance of the overall average income between the two distributions compared.

Proposition 6

Given two income distributions F and $G\in \mathcal {F}$ and two threshold percentiles $\underline{p}$ and $\bar{p}$, $W(F)\ge W(G)$ $\forall \; W \in Z_6=\{W:W \in Z, f''(s) \le 0 \ \forall \; s \in [\bar{p},1], f''(p) =f''(q)= 0 \ \forall \; p \in [0, \underline{p}], \forall q \in [\underline{p}, \bar{p}]\}$ if and only if

$$\begin{aligned}{} & {} (i) \; \Xi (s) \ge 0 \ \forall \; s \in [\bar{p},1 ]\\{} & {} (ii) \; \Phi (\underline{p}) \ge 0\\{} & {} (iii) \; \Pi (\bar{p}) \ge 0 \end{aligned}$$

where $\Phi (\underline{p})=\int _0^{\underline{p}} [F^{-1}(p) - G^{-1} (p)] dp$ and $\Pi (\bar{p})=\int _{\underline{p}}^{\bar{p}} \left[ F^{-1}(q) - G^{-1} (q)\right] dq$.

The first condition of Proposition 6, as above, is equivalent to condition (i) of Proposition 2, except for the threshold percentile: it is a dominance of the cumulated sum of incomes ranked between $\bar{p}$ and 1. The second condition requires checking that the average income of the individuals ranked up to $\underline{p}$ of the dominant distribution is not lower than the average income of the dominated distribution. The third condition, instead, concerns the dominance between the average income of individuals ranked in the middle, more precisely between $\underline{p}$ and $\bar{p}$.

As discussed in the introduction, the theoretical results developed in this section contribute to the literature on game theory, happiness economics, and normative economics. However, they are also related to the literature in the filed of applied mathematics and insurance that devotes particular attention to the properties of the Yaari preference functionals and their implications for inverse stochastic dominance.⁸ Most prominent contributions include, among others, Muliere and Scarsini (1989), Wang and Young (1998), Dentcheva and Ruszczyński (2006). Muliere and Scarsini (1989) are concerned with the implications of resorting to second-degree Lorenz dominance as an additional criterion to be implemented for the construction of Lorenz curves orderings. They also argue that there may be a closer relationship between Lorenz dominance of first or higher degrees and rank-dependent measures of inequality than between Lorenz dominance and utilitarian measures of inequality, as the former are explicitly defined in terms of the Lorenz curve, whereas the utilitarian measures are not.⁹ Similarly, Wang and Young (1998) characterize partial orders to be applied for intersecting distribution functions. Dentcheva and Ruszczyński (2006) introduce sequences of orders that are based on iterated integrals. They also present optimality and duality conditions for convex optimization problems that satisfy the stochastic dominance constraints. With respect to this literature, this paper contributes by proposing dominance conditions that are consistent with a Yaari functional that is expression at the same time of different attitudes towards the income benefited by different parts of the distribution. This is realized by imposing different constraints on social preferences that depend on the introduction of a threshold identifying the different partition of the distributions toward which social preferences do not coincide. In some specific cases, our dominance results can be interpreted as generalization of existing inverse stochastic dominance orderings. Because of the different attitudes incorporated in the same social preferences, our results also consider situations of downward dominance that were not contemplated in the above mentioned literature. In fact, the existing frameworks impose an arbitrary focus on compassion by focusing on the bottom of the distribution. This paper contributes to the existing literature by providing new normative criteria, within a self-contained and unifying framework, that will not suffer from these restrictions.

3 Empirical illustration

3.1 Data

Our empirical illustration is based on the Cross National Equivalent File (CNEF), designed at Cornell University to provide harmonized data for a set of country-specific representative surveys of their resident population. In particular, we consider Australia (CNEF version of the Household Income and Labour Dynamics in Australia—HILDA), Germany (CNEF version of the German Socio-Economic Panel—SOEP), Korea (CNEF version of the Korea Labor and Income Panel Study—KLIPS), Switzerland (CNEF version of the Swiss Household Panel—SHP), and the US (CNEF version of the US panel Study of Income Dynamics—PSID). The focus on this specific group of countries is motivated by the possibility of benefiting from microdata from different countries that are already harmonized. This makes easier the operationalization of our work by comparing countries with different economic background as well as different social norms. We use the 2001 and the 2015 waves, respectively the first and last year for which we have harmonized data for more than two countries, so that we cover the longest period possible.

The unit of observation is the individual. The measure of living standards is equivalized disposable household income, which includes income after transfers and the deduction of income tax and social security contributions. One of the main objectives of public policy in most countries is to make sure that their own citizens may have access to acceptable living standards. This ability can be assessed by the degree of their command over goods and services, which they consume to support their standard of living. Household income summarizes this command over resources, including the resources needed to improve health and education. Moreover, the use of income at household level as proxy of the living standards of an individual allows to account for the possibility of benefiting from resources that are pooled within the household, so that an individual’s well-being will be better represented by the amount of household resources than by the amount of his own resources. While a household’s command over goods and services may in part be affected by issues of access, it is most often a question of families having the financial resources to acquire goods and services in the market. And for most people, the most important economic resource available to support their standard of living is regular income received, independently of its source.

Incomes are expressed in constant 2010 prices, using country and year-specific price indices and are adjusted for differences in household size by dividing incomes by the square root of the household size. They are then expressed in 2010 Purchasing Power Parity. Individuals with zero sampling weights are excluded since our measures are calculated using sample weights designed to make the samples nationally representative.

Some descriptive statistics are reported in Table 3 in Appendix B. We can see that the countries in our sample differ in terms of mean incomes and Gini coefficient. In both waves considered, mean income is highest in US, the country that also has the highest Gini coefficient. Mean income is the lowest in Korea in 2001—country with the lowest Gini index in the same year—and in Germany in 2015; inequality is the lowest in Switzerland in 2015.

3.2 Results

We now apply the dominance tests presented in Sect. 2 to rank countries and check whether the ranking obtained is stable over time. The results are obtained through pairwise comparisons of the countries analyzed. That is, for every Proposition we proceed by establishing a rank between pairs of countries in each of the two years considered. Thus, overall we will perform 48 tests. To implement our framework, however, we need to select the threshold $\bar{p}$ that we fix at the 40th percentile.¹⁰

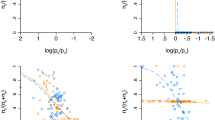
We start from Proposition 1, where efficiency and compassion matter. Table 1 shows that although the conditions imposed in this Proposition are quite strong—it requires second-order dominance of the income of each percentile up to $p=0.40$ (Fig. 1) and dominance of average income of the distribution (Table 2)—some of the distributions can already be ranked. In particular, for 2001 we find that Korea is dominated by all the other countries and there is no county that dominates all the others. We also find that Switzerland and US dominates Australia and Korea. The impossibility to establish a ranking for some of the comparisons mostly depends on the inconsistent ranking generated by conditions (i) and (ii) of the proposition (this is the case of Australia and Germany, Germany and US, and Switzerland and US).

Table 1 Test of Propositions 1, 2, 3 and 4, cross-country comparison in 2001 and 2015

After 14 years, there are changes to this country ranking. Korea is no more dominated by Germany, while, Germany turns out to be dominated by Switzerland and Australia, this latter country being no more dominated by Switzerland and US.

Fig. 1

Source: Author’s Elaboration based on CNEF

Test of Proposition 1 condition (i), cross-country comparison in 2001 and 2015.

Table 2 Test of Propositions 1 and 2, condition (ii), cross-country comparison in 2001 and 2015

We now consider the test proposed in Proposition 2, promoting efficiency and expressing concerns with respect to the feeling of envy. The summary results are reported in Table 1 and show that the ranking of countries might change when the social planner is concerned with envy rather then compassion. This is the case, in our empirical illustration, of the comparison between Australia and Germany in 2001 that can be ranked when one considers aversion to envy (with the former country dominating the latter) but cannot be ranked when one considers aversion to compassion. A similar conclusion holds for Switzerland and Germany and Switzerland and US. In 2015, these changes concern Australia and Korea with the former dominating the latter when compassion matters but not when envy does, similarly for Switzerland and Korea. The last change concerns Germany that dominates Korea when envy matters; this is not true when one accounts for aversion to the feeling of compassion (see Fig. 2).

Fig. 2

Source: Author’s Elaboration based on CNEF

Test of Proposition 2 condition (i), cross-country comparison in 2001 and 2015.

When the application of the tests proposed in the first two Propositions cannot help to establish a clear dominance between countries, one can resort to the test proposed respectively in Proposition 3 when Proposition 1 fails and in Proposition 4 when Proposition 2 fails. Indeed, Propositions 3 helps in solving two instances of ambiguous ranking. It is the case of the comparison between Germany and Switzerland in 2001, with Switzerland dominating Germany, and between Switzerland and US in 2015, with Switzerland dominating US. Proposition 4 establishes four additional rankings with respect to Proposition 2, all concentrated in 2015. With Proposition 4 we are, in fact, able to rank Australia above Korea, Switzerland above Australia and Korea, and US above Australia (see Table 1).¹¹

For completeness, the tests proposed in Propositions 5 and 6 are implemented by following the literature on inequality at the extreme tails of the distribution (see Atkinson et al., 2011; Roine et al., 2009) and fixing $\underline{p}=0.10$ and $\bar{p}=0.90$ (see Table 1 and Appendix D). Although Proposition 5 endorses the same concern for compassion as Proposition 1, the country rankings that they generate do not necessarily need to coincide as under Proposition 5 the feeling of compassion is supposed to arise with respect to those that are positioned at the very extreme ends of the distribution (the social outcasts). For instance, in our illustration, in 2001 Germany and Switzerland cannot be ranked according to Proposition 1 but they can according to Proposition 5. The other changes in the countries ranking concern the 2015 (Australia vs. Korea, Switzerland vs. Korea; US vs. Germany; US vs. Korea). In a similar vein, Proposition 6 rests upon aversion to envy but differently from Proposition 2, the feeling of aversion is caused by those individuals at the top of the income ladder (the super rich). In our analysis this is the case for the comparisons concerning Korea vs. Australia and Germany, US vs. Korea and Switzerland.

The above results have been obtained by comparing in pairs the countries under analysis, in order to judge which of each country is more socially desirable according to different social preferences. Because the dominance conditions characterized in this paper belong to the class of partial ranking criteria, and given the data employed in this empirical illustration, in neither of the years it is possible to establish a complete ranking across the five countries considered. Therefore, to summarize the results and get insights in terms of overall distributional performance, we propose to rely to the pairwise comparisons—allowing for complete rankings in most of the cases—and count the number of these comparisons for which a given country turns out to dominate or to be dominated. Accordingly, Switzerland is the dominant country in the largest number of pairwise comparisons, 31 times out of 48 pairwise comparisons (24 in 2001 and 24 in 2015), and it is never dominated. It is followed by US, which turns out to be the dominant country 31 times and it is dominated four times. Korea ranks at the bottom: it is dominated 34 times and it dominates twice. Australia and Germany are in between: Australia is the dominated country in 17 instances of comparison and it dominates 14 times; Germany is dominated 23 times and it is the dominant country in 9 instances of comparison.

In order to better grasp the relevance of adopting the framework proposed when making distributional comparisons, we report the results of the dominance test that would be implemented if the preferences of a social planner would be represented by the standard property of inequality aversion. This is done in Fig. 3 and in the bottom part of Table 1 reporting a standard second order inverse stochastic dominance. Formally we implement the following test: $\int _0^p F^{-1}(q) dq \ge \int _0^p G^{-1}(q) dq \forall p \in \left[ 0,1 \right]$.

Fig. 3

Source: Author’s Elaboration based on CNEF

Standard dominance test: cross-country comparison in 2001 and 2015.

These results show that accounting either for compassion or for envy might generate different conclusions concerning each pairwise distributional comparison performed. When we account for envy there are three pairwise comparisons that generate a different result, all found in 2015 (this is the case of Australia vs Switzerland and US vs. Korea and Switzerland). When we account for compassion, there are many more instances of non-coincident results (this is the case in 2001 of Australia vs. Germany and Switzerland vs. Germany and US, in 2015 of Australia vs Korea and Switzerland, Germany vs. Korea and US, US vs. Korea).

4 Conclusions

A large number of contributions propose alternative models to evaluate and compare distributions of income from a normative perspective, most of them build upon the assumption that the society is made of individuals with self-regarding preferences. At the same time, behavioural economists have shown, through empirical and experimental analyses, that individuals do have social preferences and their well-being depends not only on their own specific level of income but also on the level of income owned by those that are richer or poorer. In this paper, we have argued that a social planner that is willing to account for the feelings of compassion—that is generated by those having access to relatively lower income—and envy—that is generated by those having access to relatively higher income—should adopt criteria that differ from those that have already been proposed by the literature in the evaluation of alternative states of the world.

Hence, we have used the rank dependent model proposed by Yaari (1987) and we have imposed restrictions on the weights that incorporate either aversion to the feeling of compassion or aversion to the feeling of envy. Such restrictions have allowed us to obtain new dominance criteria that can be used to rank countries for a given period or for a given country to make comparisons across time. These criteria enrich the arsenal of tools available in normative economics to perform distributional comparisons, by providing new normative criteria, within a self-contained and unifying framework that can be easily adapted for the evaluation of public policies. These criteria could be employed by governmental policy analysts as well as by analysts interested in studying the social welfare implications of the different redistribution schemes that are generated under a broad class of decision procedures.

We have shown the usefulness of our framework to make cross-country comparisons, by focusing on a set of five developed countries: Australia, Germany, Korea, Switzerland, and the US. Our results show that Switzerland proves to be the best-performing country followed by US. While Korea, Germany, and Australia ranks among the least desirable. Our results also make light on the meaningfulness of including concerns for compassion and envy in carrying out distributional comparisons, as they might result into different country rankings with respect to those arising by implementing standard evaluation methods that only include inequality aversion but neglect that inequality may give rise to two different feelings, namely, compassion and envy.

The results derived in our paper can be extended in a number of directions both theoretically and empirically. From a theoretical point of view, our framework can be extended in two different directions. One possible extension concerns the evaluation of distributional changes, whose existing evaluation methods could be modified to account for compassion and envy. To do this, one could depart from the contributions of Bourguignon (2011a) and Jenkins and Van Kerm (2016). Both contributions propose evaluations of distributional changes based on the construction of mobility curves, whose dominance is shown to imply normative dominance for specific sets of social welfare functions. Thus it would be interesting to check whether incorporating in such frameworks concerns for compassion and envy enables obtaining easily implementable dominance conditions for the evaluation of mobility processes across countries and over time. In the same vein, the framework proposed could be extended to endorse an intertemporal perspective and consider aversion to compassion and envy by implementing a backward looking perspective. The second possible extension concerns the evaluation and comparison of multidimensional distributions of well-being, for instance, departing from the models proposed by Zoli (1999). From an empirical point of view, the distributional comparison scheme introduced in this paper could be applied to consider alternative outcomes, either monetary, such as wealth, or non-monetary outcomes, such as health status. They could also be applied to assess the impact on the society of exogenous events as for the case of natural disasters.

Appendices

Appendix A

Proof of Proposition 1

We want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta {W}= \int _0^1 \omega (p) \left[ F^{-1}(p) - G^{-1}(p)\right] dp \ge 0 \end{aligned}$$

(4)

with $W \in Z_1$ so that $\omega ^{'}(p) \le 0$ $\forall p \in [0,\bar{p}]$, $\omega ^{'}(s) = 0 \forall s \in [\bar{p}, 1]$ and $\omega (p) \ge \omega (s)\ge 0 \forall p \in [0, \bar{p}]$ and $s \in [\bar{p},1]$.

Let $\Delta (p)=F^{-1}(p) - G^{-1}(p)$. Given that $\omega ^{'}(s) = 0$ $\forall s \in [\bar{p}, 1]$, we can denote $\omega (s) = \beta$, so that for a fixed $\bar{p}$ we rewrite Eq. 4 as follows:

$$\begin{aligned} \Delta {W}=\int _0^{\bar{p}} \omega (p) \Delta (p)dp + \beta \int _{\bar{p}}^1 \Delta (s)ds \ge 0 \end{aligned}$$

(5)

For the sufficiency part, we integrate by parts the first part of Eq. 5:

$$\begin{aligned} \Delta W=\omega (\bar{p}) \int _0^{\bar{p}} \Delta (p) dp - \int _0^{\bar{p}} \omega ^{\prime }(p) \int _0^{p} \Delta (q)dq dp + \beta \int _{\bar{p}}^1 \Delta (s)ds \end{aligned}$$

(6)

Using the following notation $\Phi (p)=\int _0^p \Delta (q)dq$, $\Phi (\bar{p})=\int _0^{\bar{p}} \Delta (p)dp$ and $\Xi (1)=\int _{\bar{p}}^1\Delta (s)ds$, the above equation becomes:

$$\begin{aligned} \Delta W=\omega (\bar{p}) \Phi (\bar{p}) - \int _0^{\bar{p}} \omega ^{\prime }(p) \Phi (p)dp + \beta \Xi (1) \end{aligned}$$

(7)

Since $\omega ^{\prime }(p) \le 0 \forall p \in [0, \bar{p}]$, $\Phi (p) \ge 0$ for all $p \in [0,\bar{p}]$ implies $-\int _0^{\bar{p}} \omega ^{\prime }(p) \Phi (p)dp \ge 0$, and since $\omega (\bar{p}) \ge 0$, it also implies that $\omega (\bar{p})\Phi (\bar{p}) \ge 0$. Now, given that $\omega (p) \ge \beta \ge 0$, $\Phi (p) \ge 0$ for all $p \in [0,\bar{p}]$ and $\Phi (\bar{p})+\Xi (1)= \int _0^1 [F^{-1}(p) - G^{-1}(p)]dp \ge 0$ are sufficient for $\Delta {W} \ge 0$.

For the necessity part, assume that, given F, $G \in \mathcal {F}$ and $\bar{p} \in [0,1], W_{\omega }(F) \ge W_{\omega }(G)$ for all $\omega \in Z_1$. Consider the function $\omega \in Z_1$ such that $\omega (p)=g'(p)$ where $g(p)=p$ for all $p \in [0,1]$. It follows that $g'(p)=1$, for all $p \in [0,1]$. Thus from (4):

$$\begin{aligned} W(F)-W(G) \ge 0 \iff \int _0^1[F^{-1}(p)-G^{-1}(p)] dp \ge 0. \end{aligned}$$

(8)

Hence statement (ii) is true.

Now, take arbitrarily one $q \in (0, \bar{p}]$ and consider the function $\rho _q(p) \in Z_1$ where $\rho _q(p)= g'_q(p)$, with $g_q(p)$ being a smooth approximation of the function $f_q(p)$ defined by $f_q(p)=p/q$, for all $p \in [0,q]$ and $f_q(p)=1$ for all $p \in (q,1]$. By definition, $g_q'(p)=1/q >0$ for all $p \in [0,q]$ and $g'_q(p)=0$ for all $p \in (q,1]$. Thus from (5):

$$\begin{aligned} W_{\rho _q}(F)- & {} W_{\rho q}(G)\ge 0 \iff q\left[ W_{\rho q}(F)-W_{\rho q}(G)\right] \ge 0 \iff \int _0^q\left[ F^{-1}(p)\right. \nonumber \\{} & {} \left. -G^{-1}(p)\right] dp \ge 0. \end{aligned}$$

(9)

Recall that we have taken one arbitrary $q \in (0,\bar{p}]$. Since the previous relation is true for any q in this interval, the statement (i) is true.

Proof of Proposition 2

We want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta {W}= \int _0^1 \omega (p) \left[ F^{-1}(p)- G^{-1}(p)\right] dp \ge 0 \end{aligned}$$

(10)

with $W \in Z_2$ so that $\omega ^{'}(s) \le 0$ $\forall s \in [\bar{p}, 1]$, $\omega ^{'}(p) = 0$ $\forall p \in [0,\bar{p}]$.

Let $\Delta (p)=F^{-1}(p) - G^{-1}(p)$. Rewrite Eq. (10) as follows:

$$\begin{aligned} \Delta {W}= \int _0^{\bar{p}} \omega (p) \left[ F^{-1}(p)- G^{-1}(p)\right] dp+\int _{\bar{p}}^1 \omega (p) \left[ F^{-1}(p)- G^{-1}(p)\right] dp \ge 0 \end{aligned}$$

(11)

For $\omega ^{\prime }(p)=0 \; \forall p \in [0; \bar{p}]$ we can denote $\omega (p)=\alpha$ and rewrite Eq. (11) as:

$$\begin{aligned} \Delta W=\alpha \int _0^{\bar{p}} \Delta (p)dp + \int _{\bar{p}}^1 \omega (q)\Delta (q)dq\ge 0 \end{aligned}$$

(12)

Then using the following notation, $\Xi (s)=\int _{\bar{p}}^s \Delta (t)dt$, $\Phi (\bar{p})=\int _0^{\bar{p}}\Delta (p)dp$, and $\Xi (1)=\int _{\bar{p}}^1\Delta (s)ds$, the second component of Eq. (12) can be integrated by parts to obtain:

$$\begin{aligned} \Delta W=\alpha \Phi (\bar{p}) + \omega (1) \Xi (1) - \int _{\bar{p}}^1 \omega ^{\prime }(s) \Xi (s)ds \end{aligned}$$

(13)

Since $\omega ^{\prime }(s) \le 0 \; \forall s \in [\bar{p}; 1]$, $\Xi (s) \ge 0$ for all $s \in [\bar{p}; 1]$ implies $-\int _{\bar{p}}^1 \omega ^{\prime }(s) \Xi (s)ds\ge 0$, and since $\omega (1) \ge 0$, it also implies that $\omega (1)\Xi (1)\ge 0$. Hence, given $\alpha \ge 0$, $\Xi (s) \ge 0$ for all $s \in [\bar{p}; 1]$ and $\Phi (\bar{p})$ are sufficient for $\Delta W \ge 0$.

For the necessity part, assume that, given F, $G \in \mathcal {F}$ and $\bar{p} \in [0,1], W_{\omega }(F) \ge W_{\omega }(G)$ for all $\omega \in Z_2$. Consider the function $\omega \in Z_2$ such that $\omega (p)=g'(p)$ where $g(p)=p$ for all $p \in [0,\bar{p}]$ and $g(p)=1$ for all $p \in (\bar{p},1]$. It follows from (11) that:

$$\begin{aligned} \Delta W_{\omega } \ge 0 \iff \int ^{\bar{p}}_0 \Delta (p) dp \ge 0. \end{aligned}$$

(14)

Hence, statement (ii) is true.

Now, take arbitrarily any $q \in [\bar{p},1]$ and consider the function $\rho _q(p) \in Z_2$, with $\rho _q(p)=g_q'(p)$ where $g_q(p)$ is a smooth approximation of the function $f_q(p)$ defined by $f_q(p)= p$ for all $p \in [q,1]$, $f_q(p)=1/q$ for all $p \in [0, q)$. By definition, $g_q'(p)=0$ for all $p \in [0, q)$ and $g'(p)=1 > 0$ for all $p \in [q,1]$. Thus from (11):

$$\begin{aligned} \Delta W_{\rho _q} \ge 0 \iff \int _{\bar{p}}^q \Delta (s)ds. \end{aligned}$$

(15)

Recall that we have taken one arbitrary $q \in [\bar{p},1]$. Since the previous relation is true for any q in this interval, statement (i) is true.

Proof of Proposition 3

Let $\Delta (p)=F^{-1}(p) - G^{-1}(p)$, we want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta W=\int _0^1 \omega (p) \Delta (p)dp \ge 0 \end{aligned}$$

(16)

with $W \in Z_3$ so that $\omega ^{'}(p) \le 0$ and $\omega ^{''}(p) \ge 0$ $\forall p \in [0, \bar{p}]$ and $\omega ^{'}(s) = 0$ $\forall s \in [\bar{p}, 1]$ and $\omega (p) \ge \omega (s) \ge 0$ $\forall p \in [0, \bar{p}]$ and $s \in [\bar{p},1]$.

For the sufficiency proof, consider Eq. (7), using the following notation $\Psi (p)=\int _0^p\Phi (q)dq$, $\Psi (\bar{p})=\int _0^{\bar{p}}\Phi (q)dq$ and integrating by parts the second component:

$$\begin{aligned} \Delta W = \omega (\bar{p}) \Phi (\bar{p}) - \omega ^{\prime }(\bar{p}) \Psi (\bar{p}) + \int _0^{\bar{p}} \omega ^{\prime \prime }(p) \Psi (p) dp + \beta \Xi (1) \end{aligned}$$

(17)

Since $\omega ^{\prime \prime }(p) \ge 0 \forall p \in [0, \bar{p}]$, $\Psi (p) \ge 0$ for all $p \in [0,\bar{p}]$ implies $\int _0^{\bar{p}} \omega ^{\prime \prime }(p) \Psi (p) \ge 0$, and since $\omega (\bar{p})^{\prime } \le 0$, it also implies that $-\omega (\bar{p})^{\prime }\Psi (\bar{p}) \ge 0$. Hence, given $\omega (\bar{p}) \ge \beta \ge 0$ we can apply Abel lemma so that $\Psi (p) \ge 0$ for all $p \in [0,1]$, $\Phi (\bar{p}) \ge 0$ and $\Phi (\bar{p}) + \Xi (1) \ge 0$ are sufficient for $\Delta W \ge 0$.

For the necessity part, assume that, given F, $G \in \mathcal {F}$ and $\bar{p} \in [0,1], W_{\omega }(F) \ge W_{\omega }(G)$ for all $\omega \in Z_3$. Consider the function $\omega \in Z_3$ such that $\omega (p)=g'(p)$ where $g(p)=k p$ for all $p \in [0,\bar{p}]$ and $g(p)= r p$ for all $p \in (\bar{p}, 1]$ with $1>k>r>0$. It follows that $\omega (p)=k$, for all $p \in [0,\bar{p}]$ and $\omega (p)=r$, for all $p \in (\bar{p}, 1]$. Thus equation (11) becomes:

$$\begin{aligned} \Delta W_{\omega }=k \int _0^{\bar{p}} \Delta (p) dp + r \int _{\bar{p}}^1 \Delta (p) dp \end{aligned}$$

(18)

So that, because $k>r>0$:

$$\begin{aligned} \Delta W_{\omega } \ge 0 \iff \int _0^{\bar{p}}[F^{-1}(p)-G^{-1}(p)] dq \ge 0 \; \text {and} \int _0^{1}[F^{-1}(p)-G^{-1}(p)] dq\ge 0. \end{aligned}$$

(19)

Hence statement (ii) is true.

Now, since $\omega (p)$ is constant over the interval $[\bar{p}, 1]$ rewrite Eq. (4) as follows:

$$\begin{aligned} \Delta W= \int _0^{\bar{p}} \omega (p) \Delta (p) dp + \omega (\bar{p}) \int _{\bar{p}}^1 \Delta (p) dp. \end{aligned}$$

(20)

Integrate by parts the first component to obtain:

$$\begin{aligned} \Delta W= \omega (\bar{p}) \int _0^{\bar{p}} \Delta (p) dp - \int _0^{\bar{p}} \omega '(p) \int _0^p \Delta (q) dq dp +\omega (\bar{p}) \int _{\bar{p}}^1 \Delta (p) dq. \end{aligned}$$

(21)

After simplification one obtains:

$$\begin{aligned} \Delta W= \omega (\bar{p}) \int _0^1 \Delta (p) dp - \int _0^{\bar{p}} \omega '(p) \int _0^p \Delta (q) dq dp. \end{aligned}$$

(22)

Now, take arbitrarily one $q \in (0, \bar{p}]$ and consider the function $\rho _q(p) \in Z_3$, such that $\rho _q(p)=g'_q(p)$, where $g_q(p)$ is a smooth approximation of the function $f_q(p)$ defined by $f_q(p)=\alpha p - p^2$ with $\alpha \ge 2$ for all $p \in [0,q]$ and $f_q(p)=1$ for all $p \in (q,1]$ and for $p=\bar{p}$. By definition, $g_q''(p)=-2 <0$ for all $p \in [0,q]$ and $g'_q(p)=0$ for all $p \in (q,1]$. Thus from (22):

$$\begin{aligned} \Delta W_{\rho _q} \ge 0 \iff \int _0^q\phi (p) dp \ge 0. \end{aligned}$$

(23)

Recall that we have taken one arbitrary $q \in (0,\bar{p}]$. Since the previous relation is true for any q in this interval, statement (i) is true.

Proof of Proposition 4

Let $\Delta (p)=F^{-1}(p) - G^{-1}(p)$, we want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta W=\int _0^1 \omega (p) \Delta (p)dp \ge 0 \end{aligned}$$

(24)

with $W \in Z_4$ so that $\omega ^{'}(s) \le 0$, $\omega ^{''}(s) \le 0$ $\forall s \in [\bar{p}, 1]$, $\omega ^{'}(p) = 0$ $\forall p \in [0,\bar{p}]$.

For the sufficiency proof, consider Eq. (13), denote $\Theta (s)=\int _{\bar{p}}^s\Xi (t)dt$ and $\Theta (1)=\int _{\bar{p}}^1\Xi (t)dt$ and integrate by parts the third component:

$$\begin{aligned} \Delta W = \alpha \Phi (\bar{p}) + \omega (1) \Xi (1) - \omega ^{\prime }(1) \Theta (1) + \int _{\bar{p}}^1 \omega ^{\prime \prime }(s)\Theta (s) ds \end{aligned}$$

(25)

The last component of the above equation can be rewritten as follows:

$$\begin{aligned}{} & {} \int _{\bar{p}}^1 \omega ^{\prime \prime }(s)\left[ \int _{\bar{p}}^{1}\Xi (t)-\int _{s}^{1}\Xi (t)\right] dtds=\int _{\bar{p}}^1 \omega ^{\prime \prime }(s)\int _{\bar{p}}^{1}\Xi (t)dtds\\{} & {} \quad -\int _{\bar{p}}^1 \omega ^{\prime \prime }(s)\int _{s}^{1}\Xi (t)dtds. \end{aligned}$$

Noting that $\int _{\bar{p}}^1 \omega ^{\prime \prime }=\omega ^{\prime }(1)-\omega ^{\prime }(\bar{p})$, for $\omega ^{\prime }(1)=0$ we have

$$\begin{aligned} -\omega ^{\prime }(\bar{p})\int _{\bar{p}}^{1}\Xi (s)-\int _{\bar{p}}^{1}\omega ^{\prime \prime }(s)\int ^1_s \Xi (t)dsdt. \end{aligned}$$

Denoting $\Omega (s)=\int _s^1 \Xi (t)dt$, $\Delta W$ can now be rewritten as follows:

$$\begin{aligned} \Delta W = \alpha \Phi (\bar{p}) + \omega (1) \Xi (1) -\omega ^{\prime }(\bar{p})\Theta (1)-\int _{\bar{p}}^{1}\omega ^{\prime \prime }(s)\Omega (s)ds. \end{aligned}$$

(26)

Since $\omega ^{\prime \prime }(s) \le 0 \forall s \in [\bar{p}, 1]$, $\Omega (s) \ge 0$ for all $s \in [\bar{p}, 1]$ implies $-\int _{\bar{p}}^1 \omega ^{\prime \prime }(s) \Omega (s) ds \ge 0$, and since $\omega (\bar{p})^{\prime } \le 0$, it also implies that $-\omega (\bar{p})^{\prime }\Theta (1) \ge 0$. Hence, given $\alpha , \omega (1) \ge 0$, $\Omega (s) \ge 0$ for all $s \in [\bar{p}, 1]$, $\Phi (\bar{p}) \ge 0$ and $\Xi (1) \ge 0$ are sufficient for $\Delta W \ge 0$.

For the necessity part, assume that, given F, $G \in \mathcal {F}$ and $\bar{p} \in [0,1], W_{\omega }(F) \ge W_{\omega }(G)$ for all $\omega \in Z_4$. Now, take arbitrarily any $q \in [\bar{p},1]$ and consider the function $\omega _q(p) \in Z_4$, with $\omega _q(p)=g_q'(p)$ where $g_q(p)$ is a smooth approximation of the function $f_q(p)$ defined by $f_q(p)=1$ for all $p \in [0, \bar{p}]$ and $f_q(p)=\frac{\alpha }{2}p^2-\frac{p^3}{3}$ for all $p \in [\bar{p}, 1)$ with $1<\alpha <2$, and $f_q(p)=1$ for $p=1$ so that $g_q'(p)=0$ for all $p \in [0, \bar{p}]$, $g''_q(p)=0$ for $p=1$ $g'''(p)=-2$ for all $p \in [\bar{p}, 1)$. Thus from (26):

$$\begin{aligned} \Delta W_{\omega _q} \ge 0 \iff \int _q^1 \Omega (s)ds \ge 0. \end{aligned}$$

(27)

Recall that we have taken one arbitrary $q \in [\bar{p},1]$. Since the previous relation is true for any q in this interval, statement (i) is true.

Now consider Eq. (25) and the function $\omega (p) \in Z_4$ such that $\omega (p)=g'(p)$ where $g(p)=p$ for all $p \in [0, \bar{p}]$, $g(p)=2p-p^2$ for all $p \in [\bar{p},1)$, and $g(p)=1$ for $p=1$. This implies that $g'(p)=1$ for all $p \in [0, \bar{p}]$, $g'(p)=2-2p>0$, $g''(p)=-2<0$, and $g'''(p)=0$ for all $p \in [\bar{p},1)$, and $g'(p)=0$ for $p=1$ thus:

$$\begin{aligned} \Delta W_{\omega _q} \ge 0 \iff \int _0^{\bar{p}} \Delta (p) dp \ge 0. \end{aligned}$$

(28)

Hence, statement (ii) is true.

Take again Eq. (25), consider the function $\omega (p) \in Z_4$ such that $\omega (p)=g'(p)$ where $g(p)=1/k$ with $k>1$ for all $p \in [0, \bar{p}]$, $g(p)=\alpha -1/2p^2$ for all $p \in [\bar{p},1]$, and $g(p)=p$ for $p=1$. This implies that $g'(p)=0$ for all $p \in [0, \bar{p}]$, $g'(p)=2-2p>0$, $g''(p)=-2<0$, and $g'''(p)=0$ for all $p \in [\bar{p},1)$, $g'(p)=1$ and $g''(p)=0$ for $p=1$, thus:

$$\begin{aligned} \Delta W_{\omega _q} \ge 0 \iff \int _{\bar{p}}^1 \Delta (s) ds \ge 0. \end{aligned}$$

(29)

Hence, statement (iii) is true.

Proof of Proposition 5

We want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta {W} = \int _0^1 \omega (p) \left[ F^{-1}(p) - G^{-1}(p)\right] dp \ge 0 \end{aligned}$$

(30)

with $W \in Z_5$ so that $\omega ^{'}(p) \le 0$ $\forall p \in [0,\underline{p}]$, $\omega ^{'}(q) =\omega ^{'}(s)= 0$ $\forall q \in [\underline{p}, \bar{p}]$ and $\forall s \in [\bar{p}, 1]$, and $\omega (p) \ge \omega (q) \ge \omega (s)$.

Letting $\Delta (p)=F^{-1}(p) - G^{-1}(p)$, rewrite Eq. (30) as follows:

$$\begin{aligned} \Delta {W}=\int _0^{\underline{p}} \omega (p) \Delta (p)dp + \int _{\underline{p}}^{\bar{p}} \omega (q) \Delta (q)dq + \int _{\bar{p}}^1 \omega (s) \Delta (s)ds \ge 0 \end{aligned}$$

(31)

For the sufficiency part, denote $\omega (q)=\gamma$ and $\omega (s)=\pi$, integrate by parts the first component of Eq. (31):

$$\begin{aligned} \Delta W= & {} \omega (\underline{p}) \int _0^{\underline{p}} \Delta (p) dp - \int _0^{\underline{p}} \omega ^{\prime }(p) \int _0^{p} \Delta (q)dq dp \nonumber \\{} & {} + \gamma \int _{\underline{p}}^{\bar{p}} \Delta (q)dq+\pi \int _{\bar{p}}^1 \Delta (s)ds \end{aligned}$$

(32)

Use the following notation $\Phi (p)=\int _0^p \Delta (q)dq$, $\Phi (\underline{p})=\int _0^{\underline{p}} \Delta (p)dp$, $\Pi (\bar{p})=\int ^{\bar{p}}_{\underline{p}}\Delta (q)dq$, and $\Xi (1)=\int _{\bar{p}}^1\Delta (s)ds$ to obtain:

$$\begin{aligned} \Delta W= \omega (\underline{p}) \Phi (\underline{p}) - \int _0^{\underline{p}} \omega ^{\prime }(p) \Phi (p)dp + \gamma \Pi (\bar{p})+\pi \Xi (1) \end{aligned}$$

(33)

Since $\omega ^{\prime }(p) \le 0 \forall p \in [0, \underline{p}]$, $\Phi (p) \ge 0$ for all $p \in [0,\underline{p}]$ implies $-\int _0^{\underline{p}} \omega ^{\prime }(p) \Phi (p)dp \ge 0$, and since $\omega (\underline{p}) \ge 0$, it also implies that $\omega (\underline{p})\Phi (\underline{p}) \ge 0$. Hence, given $\omega (\underline{p}) \ge \gamma \ge \pi \ge 0$, applying Abel Lemma, $\Phi (p) \ge 0$ for all $p \in [0,\underline{p}]$, $\int _0^{\bar{p}} F^{-1}(q) - G^{-1} (q) dq \ge 0$ and $\int _0^1F^{-1}(p) - G^{-1} (p) dp \ge 0$, are sufficient for $\Delta {W} \ge 0$.

For the necessity part, assume that given $F, G \in \mathcal {F}$ and $\bar{p}, \underline{p} \in [0,1]$, $W_{\omega }(F)>W_{\omega }(G)$ for all $\omega \in Z_5$. Consider the function $\omega \in Z_5$ such that $\omega (p)=g'(p)$ and $g(p)=2p$ for all $p \in [0, \bar{p}]$ and $g(p)=p$ for all $p \in [\bar{p}, 1]$. It follows that $g'(p)=2$ for all $p \in [0, \bar{p}]$ and $g'(p)=1$ for all $p \in [\bar{p}, 1]$. Thus, from Eq. (31):

$$\begin{aligned} \Delta W_{\omega } \ge 0 \iff \int _0^{\bar{p}} \Delta (p) dp \ge 0 \; \text {and} \int _0^1 \Delta (p)dp \ge 0. \end{aligned}$$

(34)

Hence statement (ii) is true.

The second part of the necessity proof follows exactly as in Proposition 1. Thus, take arbitrarily one $q \in (0, \underline{p}]$ and consider the function $\rho _q(p) \in Z_5$ where $\rho _q(p)= g'_q(p)$, with $g_q(p)$ being a smooth approximation of the function $f_q(p)$ defined by $f_q(p)=p/q$, for all $p \in [0,q]$ and $f_q(p)=1$ for all $p \in (q,1]$. By definition, $g_q'(p)=1/q >0$ for all $p \in [0,q]$ and $g'_q(p)=0$ for all $p \in (q,1]$. Thus, from Eq. (31):

$$\begin{aligned} \Delta W_{\omega _q}\ge 0 \iff \int _0^q[F^{-1}(p)-G^{-1}(p)] dp \ge 0. \end{aligned}$$

(35)

Recall that we have taken one arbitrary $q \in (0,\underline{p}]$. Since the previous relation is true for any q in this interval, statement (i) is true.

Proof of Proposition 6

We want to find sufficient and necessary conditions for

$$\begin{aligned} \Delta {W} = \int _0^1 \omega (p) \left[ F^{-1}(p) - G^{-1}(p)\right] dp \ge 0 \end{aligned}$$

(36)

with $W \in Z_6$ so that $\omega ^{'}(s) \le 0$ $\forall s \in [\bar{p},1]$, $\omega ^{'}(p) =\omega ^{'}(q)= 0$ $\forall p \in [0, \underline{p}]$ and $\forall q \in [\underline{p}, \bar{p}]$, and $\omega (p) \ge \omega (q) \ge \omega (s)$.

For the sufficiency part, knowing that $\omega (p)$ is constant over the interval $[0, \underline{p}]$, so that we can denote it as $\omega (p)=\alpha$, and $\omega (q)$ is constant over the interval $[ \underline{p}, \bar{p}]$, so that we can denote it $\omega (q)=\gamma$. Denoting $\Xi (s)=\int _{\bar{p}}^s \Delta (t)dt$, $\Phi (\bar{p})=\int _0^{\bar{p}}\Delta (p)dp$, and $\Xi (1)=\int _{\bar{p}}^1\Delta (s)ds$, the equation can be rewritten as:

$$\begin{aligned} \Delta w= \alpha \Phi (\underline{p}) + \gamma \Pi (\bar{p}) +\int _{\bar{p}}^1 \omega (s) \Delta (s)ds. \end{aligned}$$

(37)

Integrate by parts the last component:

$$\begin{aligned} \Delta W= \alpha \Phi (\underline{p}) + \gamma \Pi (\bar{p}) + \omega (1) Xi(1)-\int _{\bar{p}}^1 \omega '(s)\Xi (s)ds. \end{aligned}$$

(38)

Since $\omega ^{\prime }(s) \le 0 \; \forall p \in [\bar{p}; 1]$, $\Xi (s) \ge 0$ for all $s \in [\bar{p}; 1]$ implies $-\int _{\bar{p}}^1 \omega ^{\prime }(s) \Xi (s)ds\ge 0$, and since $\omega (1) \ge 0$, it also implies that $\omega (1)\Xi (1)\ge 0$. Hence, given $\alpha , \gamma \ge 0$, $\Xi (s) \ge 0$ for all $s \in [\bar{p}; 1]$, $\Phi ( {p}) \ge 0, \Pi (\bar{p}) \ge 0$ are sufficient for $\Delta W \ge 0$.

For the necessity part, the proof follows the same steps as in Proposition 2. Assume that, given F, $G \in \mathcal {F}$ and $\bar{p} \in [0,1], W(F) \ge W(G)$ for all $\omega \in Z_6$. Take arbitrarily any $q \in [\bar{p},1]$ and consider the function $\rho _q(p) \in Z_6$, with $\rho _q(p)=g_q'(p)$ where $g_q(p)$ is a smooth approximation of the function $f_q(p)$ defined by $f_q(p)=1/q$ for all $p \in [0, q)$ and $f_q(p)=p$ for all $p \in [q, 1)$ and $f_q(p)=1$ for $p=1$ so that $g_q'(p)=0$ for all $p \in [0, q)$, $g_q'(p)=1>0$ for all $p \in [q, 1)$, and $g_q'(p)=0$ for $p=1$. Thus from (37):

$$\begin{aligned} \Delta W_{\rho _q} \ge 0 \iff \int _{\bar{p}}^q \Delta (s)ds. \end{aligned}$$

(39)

Recall that we have taken one arbitrary $q \in [\bar{p},1]$. Since the previous relation is true for any q in this interval, statement (i) is true.

Now consider again Eq. (37) and the function $\omega (p) \in Z_6$ such that $\omega (p)=g'(p)$ and $g(p)=2p$ for all $p \in [0, \underline{p}]$, $g(p)=2$ for all $p \in [\underline{p},1]$. This implies that $g'(p)=2$ for all $p \in [0, \underline{p}]$ and $g'(p)=0$ for all $p \in [\underline{p},1]$, thus we have that:

$$\begin{aligned} \Delta W_{\omega }\ge 0 \iff \int _0^{\underline{p}} \Delta (p) dp \ge 0. \end{aligned}$$

(40)

Hence, statement (ii) is true.

Consider again Eq. (37) but assume the function $\pi (p) \in Z_6$ such that $\pi (p)=g'(p)$, with $g(p)=1$ for all $p \in [0, \underline{p}]$, $g(p)=p$ for all $p \in [\underline{p}, \bar{p}]$, $g(p)=1/k$ with $k>0$ for all $p \in [\bar{p},1]$. This implies that $g'(p)=0$ for all $p \in [0, \underline{p}]$ and for all $p \in [\bar{p},1]$ and $g'(p)=1$ for all $p \in [\bar{p},1]$, thus we have that:

$$\begin{aligned} \Delta W_{\pi }\ge 0 \iff \int _{\underline{p}}^{\bar{p}} \Delta (s) ds \ge 0. \end{aligned}$$

(41)

Hence, statement (iii) is true.

Appendix B

1.1 Descriptive statistics

See Table 3.

Table 3 Descriptive statistics by country and year

Appendix C

1.1 Test of Proposition 3 and 4, condition (i) and (ii)

See Figs. 4, 5 and Table 4.

Fig. 4

Source: Author’s Elaboration based on CNEF

Test of Proposition 3 condition (i), cross-country comparison in 2001 and 2015.

Fig. 5

Source: Author’s Elaboration based on CNEF

Test of Proposition 4 condition (i), cross-country comparison in 2001 and 2015.

Table 4 Test of Propositions 3 and 4, condition (ii), cross-country comparison in 2001 and 2015

Appendix D

1.1 Propositions 5 and 6

See Figs. 6, 7 and Table 5.

Fig. 6

Source: Author’s Elaboration based on CNEF

Test of Proposition 5 condition (i), cross-country comparison in 2001 and 2015.

Fig. 7

Source: Author’s Elaboration based on CNEF

Test of Proposition 6 condition (i), cross-country comparison in 2001 and 2015.

Table 5 Proposition 5 and 6, condition (ii) and (iii) cross-country comparison in 2001 and 2010