Suppressing homoclinic chaos for a class of vibro-impact oscillators by non-harmonic periodic excitations

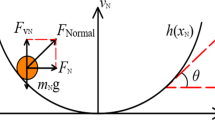
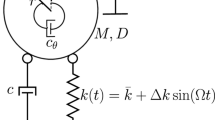Abstract

This paper proposes a theoretical framework and carries out numerical verification for suppressing homoclinic chaos of a class of vibro-impact oscillators by adding non-harmonic periodic excitations. Based on the Melnikov method of non-smooth systems, the theoretical sufficient conditions for suppressing homoclinic chaos are obtained by eliminating the simple zeros of the corresponding Melnikov function while retaining the infinite terms of the Fourier expansion of the non-harmonic periodic excitations. Furthermore, the effects of waveforms, amplitudes, initial phases, and impulse of the non-harmonic periodic excitations on chaos suppression are studied, and the optimal parameters for suppressing chaos are analytically obtained. Finally, the effectiveness of theories is verified by the vibro-impact Duffing oscillator. Numerical results show that chaos induced by the transversal intersection of homoclinic orbits can be weakened or even suppressed by adding the non-harmonic periodic excitations, and when the impulse transmitted by the non-harmonic periodic excitations is maximum, the effective amplitude for suppressing chaos is minimal. Moreover, there may be some phenomena that do not have too good a quantitative agreement between theoretical predictions and numerical results.

Appendix A

The Hamiltonian equation of system (12) is

$$\begin{aligned} H\left( x,y\right) =\frac{1}{2}y^{2}+V\left( x\right) . \end{aligned}$$

(A.1)

Let $H\left( x,y\right)$=$H\left( 0,0\right) =0$, and get

$$\begin{aligned} y^{2}=-2V\left( x\right) , \end{aligned}$$

which is equivalent to

$$\begin{aligned} y=\pm \sqrt{-2V\left( x\right) }. \end{aligned}$$

(A.2)

The calculation process of the Melnikov function Eq. (14) is as follows:

$$\begin{aligned}{} & {} M\left( \tau _{0}\right) \nonumber \\{} & {} \quad =-2\,\rho \left[ V\left( 0\right) -V\left( \alpha \right) \right] \nonumber \\{} & {} \qquad +\int _{-\infty }^{-T}\left[ JDH\left( \gamma ^{u}\left( s\right) \right) \wedge g_{1}\left( \gamma ^{u}\left( s\right) ,s+\tau _{0}+T\right) \right] ds\nonumber \\{} & {} \qquad +\int _{T}^{+\infty }\left[ JDH\left( \gamma ^{s}\left( s\right) \right) \wedge g_{1}\left( \gamma ^{s}\left( s\right) ,s+\tau _{0}-T\right) \right] ds\nonumber \\{} & {} \qquad +\int _{-\infty }^{-T}\left[ JDH\left( \gamma ^{u}\left( s\right) \right) \wedge g_{2}\left( \gamma ^{u}\left( s\right) ,s+\tau _{0}+T\right) \right] ds\nonumber \\{} & {} \qquad +\int _{T}^{+\infty }\left[ JDH\left( \gamma ^{s}\left( s\right) \right) \wedge g_{2}\left( \gamma ^{s}\left( s\right) ,s+\tau _{0}-T\right) \right] ds,\nonumber \\{} & {} \quad =-2\,\rho \left[ V\left( 0\right) -V\left( \alpha \right) \right] \nonumber \\{} & {} \qquad +\int _{-\infty }^{-T}-\delta y^{2}\left( \tau \right) +F y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}+T\right) \right) d\tau \nonumber \\{} & {} \qquad +\int _{T}^{+\infty }-\delta y^{2}\left( \tau \right) +F y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}-T\right) \right) d\tau \nonumber \\{} & {} \qquad -\int _{-\infty }^{-T}y\left( \tau \right) \eta f\left( \tau +\tau _{0}+T\right) d\tau \nonumber \\{} & {} \qquad -\int _{T}^{+\infty }y\left( \tau \right) \eta f\left( \tau +\tau _{0}-T\right) d\tau ,\nonumber \\{} & {} \quad =-2\,\rho \left[ V\left( 0\right) -V\left( \alpha \right) \right] -\delta \left[ \int _{-\infty }^{-T}y^{2}\left( \tau \right) d\tau \right. \nonumber \\{} & {} \qquad \left. +\int _{T}^{+\infty }y^{2}\left( \tau \right) d\tau \right] \nonumber \\{} & {} \qquad +F\left[ \int _{-\infty }^{-T}y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}+T\right) \right) d\tau \right. \nonumber \\{} & {} \qquad \left. +\int _{T}^{+\infty }y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}-T\right) \right) d\tau \right] \nonumber \\{} & {} \qquad -\int _{-\infty }^{-T}y\left( \tau \right) \eta \sum _{n=0}^{\infty }a_{n}\left( m\right) \sin \left( \Omega _{n}\left( \tau +\tau _{0}+T\right) \right. \nonumber \\{} & {} \qquad \left. +\left( 2n+1\right) \varphi \right) d\tau \nonumber \\{} & {} \qquad -\int _{T}^{+\infty }y\left( \tau \right) \eta \sum _{n=0}^{\infty }a_{n}\left( m\right) \sin \left( \Omega _{n}\left( \tau +\tau _{0}-T\right) \right. \nonumber \\{} & {} \qquad \left. +\left( 2n+1\right) \varphi \right) d\tau , \end{aligned}$$

(A.3)

where $\Omega _{n}=\left( 2n+1\right) \Omega$.

Mark that

$$\begin{aligned} \begin{aligned}&I_{1}=\int _{-\infty }^{-T}y^{2}\left( \tau \right) d\tau +\int _{T}^{+\infty }y^{2}\left( \tau \right) d\tau ,\\&I_{2}\left( \Omega \right) =\underbrace{\int _{-\infty }^{-T}y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}+T\right) \right) d\tau }_{\textit{I}_{21}\left( \Omega \right) }\\&\quad +\underbrace{\int _{T}^{+\infty }y\left( \tau \right) \cos \left( \Omega \left( \tau +\tau _{0}-T\right) \right) d\tau }_{\textit{I}_{22}\left( \Omega \right) },\\&I_{4}\left( \Omega _{n}\right) =\underbrace{\int _{-\infty }^{-T}y\left( \tau \right) \sin \left( \Omega _{n} \left( \tau +\tau _{0}+T\right) +\left( 2n+1\right) \varphi \right) d\tau }_{\textit{I}_{41}\left( \Omega _{n}\right) }\\&\quad +\underbrace{\int _{T}^{+\infty }y\left( \tau \right) \sin \left( \Omega _{n}\left( \tau +\tau _{0}-T\right) + \left( 2n+1\right) \varphi \right) d\tau }_{\textit{I}_{42}\left( \Omega _{n}\right) }.\\ \end{aligned} \end{aligned}$$

It is easy to obtain

$$\begin{aligned} \begin{aligned} I_{1}&=\int _{-\infty }^{-T}y\left( \tau \right) \dot{x}\left( \tau \right) d\tau +\int _{T} ^{+\infty }y\left( \tau \right) \dot{x}\left( \tau \right) d\tau \\&=\int _{0}^{\alpha }ydx+\int _{\alpha }^{0}ydx\\&=2\int _{0}^{\alpha }\sqrt{-2V\left( x\right) }dx. \end{aligned} \end{aligned}$$

(A.4)

Since

$$\begin{aligned} \begin{aligned} \cos \left( \Omega \left( \tau +\tau _{0}+T\right) \right) =&\cos \left( \Omega \tau _{0}\right) \cos \left( \Omega \left( \tau +T\right) \right) \\&-\sin \left( \Omega \tau _{0}\right) \sin \left( \Omega \left( \tau +T\right) \right) ,\\ \cos \left( \Omega \left( \tau +\tau _{0}-T\right) \right) =&\cos \left( \Omega \tau _{0}\right) \cos \left( \Omega \left( \tau -T\right) \right) \\&-\sin \left( \Omega \tau _{0}\right) \sin \left( \Omega \left( \tau -T\right) \right) , \end{aligned} \end{aligned}$$

$I_{21}\left( \Omega \right)$ can be reformed as

$$\begin{aligned} I_{21}\left( \Omega \right)= & {} \int _{-\infty }^{-T}y\left( \tau \right) \left[ \cos \left( \Omega \tau _{0}\right) \cos \left( \Omega \left( \tau +T\right) \right) \right. \nonumber \\{} & {} \left. -\sin \left( \Omega \tau _{0}\right) \sin \left( \Omega \left( \tau +T\right) \right) \right] d\tau \nonumber \\= & {} \cos \left( \Omega \tau _{0}\right) \int _{-\infty }^{-T}y\left( \tau \right) \cos \left( \Omega (\tau +T)\right) d\tau \nonumber \\{} & {} -\sin \left( \Omega \tau _{0}\right) \int _{-\infty }^{-T}y\left( \tau \right) \sin \left( \Omega (\tau +T)\right) d\tau \nonumber \\= & {} \cos \left( \Omega \tau _{0}\right) \int _{-\infty }^{-T}\cos \left( \Omega \left( \tau +T\right) \right) dx \left( \tau \right) \nonumber \\{} & {} -\sin \left( \Omega \tau _{0}\right) \int _{-\infty }^{-T}\sin \left( \Omega \left( \tau +T\right) \right) dx\left( \tau \right) \nonumber \\= & {} \cos \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\cos \left( \Omega \left( \tau +T\right) \right) dx\nonumber \\{} & {} -\sin \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\sin \left( \Omega \left( \tau +T\right) \right) dx. \end{aligned}$$

(A.5)

Note that

$$\begin{aligned} y=\frac{dx}{d\tau }=\sqrt{-2V\left( x\right) },\quad y>0, \end{aligned}$$

i.e.

$$\begin{aligned} d\tau =\frac{1}{\sqrt{-2V\left( x\right) }}dx, \end{aligned}$$

which means

$$\begin{aligned} \int _{-T}^{\tau }d\tau= & {} \tau +T=\int _{\alpha }^{x}\frac{1}{\sqrt{-2V\left( x\right) }}dx,\nonumber \\ \tau= & {} -T+\int _{\alpha }^{x}\frac{1}{\sqrt{-2V\left( x\right) }}dx. \end{aligned}$$

(A.6)

Substituting Eq. (A.6) into Eq. (A.5), yields

$$\begin{aligned} \begin{aligned} I_{21}\left( \Omega \right) =&\cos \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\cos \bigg (\Omega \int _{\alpha }^{x}\frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx\\&-\sin \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\sin \bigg (\Omega \int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx. \end{aligned} \end{aligned}$$

(A.7)

Similarly, get

$$\begin{aligned} \begin{aligned} I_{22}\left( \Omega \right) =&-\cos \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\cos \bigg (\Omega \int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx\\&-\sin \left( \Omega \tau _{0}\right) \int _{0}^{\alpha } \sin \bigg (\Omega \int _{\alpha }^{x}\frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx. \end{aligned} \end{aligned}$$

(A.8)

Then the expression of $I_{2}\left( \Omega \right)$ can be obtained as follows:

$$\begin{aligned} I_{2}\left( \Omega \right) =-2\sin \left( \Omega \tau _{0}\right) \int _{0}^{\alpha }\sin \bigg (\Omega \int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx. \nonumber \\ \end{aligned}$$

(A.9)

Let

$$\begin{aligned} I_{3}\left( \Omega \right) =-2\int _{0}^{\alpha }\sin \bigg (\Omega \int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx, \nonumber \\ \end{aligned}$$

(A.10)

so $I_{2}\left( \Omega \right) =I_{3}\left( \Omega \right) \sin \left( \Omega \tau _{0}\right)$.

Since

$$\begin{aligned} \begin{aligned} \sin&\left( \Omega _{n}\left( \tau +\tau _{0}+T\right) +\left( 2n+1\right) \varphi \right) \\&\quad =\sin \left( \Omega _{n}\left( \tau +T\right) \right) \cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad +\cos \left( \Omega _{n}\left( \tau +T\right) \right) \sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) ,\\&\sin \left( \Omega _{n}\left( \tau +\tau _{0}-T\right) +\left( 2n+1\right) \varphi \right) \\&\quad =\sin \left( \Omega _{n}\left( \tau -T\right) \right) \cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad +\cos \left( \Omega _{n}\left( \tau -T\right) \right) \sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) ,\\ \end{aligned} \end{aligned}$$

$I_{41}\left( \Omega _{n}\right)$ can be reformed as

$$\begin{aligned} \begin{aligned}&I_{41}\left( \Omega _{n}\right) \\&\quad =\int _{-\infty }^{-T} y\left( \tau \right) \left[ \sin \left( \Omega _{n}\left( \tau +T\right) \right) \cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \right. \\&\quad \left. +\cos \left( \Omega _{n}\left( \tau +T\right) \right) \sin \left( \Omega _{n}\tau _{0} +\left( 2n+1\right) \varphi \right) \right] d\tau \\&\quad =\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{-\infty }^{-T}y\left( \tau \right) \sin \left( \Omega _{n}\left( \tau +T\right) \right) d\tau + \sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{-\infty }^{-T}y\left( \tau \right) \cos \left( \Omega _{n}\left( \tau +T\right) \right) d\tau =\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{-\infty }^{-T}\sin \left( \Omega _{n}\left( \tau +T\right) \right) dx \left( \tau \right) +\sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{-\infty }^{-T}\cos \left( \Omega _{n}\left( \tau +T\right) \right) dx \left( \tau \right) =\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{0}^{\alpha }\sin \left( \Omega _{n}\left( \tau +T\right) \right) dx +\sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{0}^{\alpha }\cos \left( \Omega _{n}\left( \tau +T\right) \right) dx=\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{0}^{\alpha }\sin \bigg (\Omega _{n}\int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx +\sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\quad \int _{0}^{\alpha }\cos \bigg (\Omega _{n}\int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx. \end{aligned} \end{aligned}$$

(A.11)

Similarly, get

$$\begin{aligned} \begin{aligned} I_{42}\left( \Omega _{n}\right) =&\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\int _{0}^{\alpha }\sin \bigg (\Omega _{n}\int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx\\&-\sin \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\int _{0}^{\alpha }\cos \bigg (\Omega _{n}\int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx. \end{aligned} \end{aligned}$$

(A.12)

Then the expression of $I_{4}\left( \Omega _{n}\right)$ can be obtained as follows:

$$\begin{aligned} \begin{aligned} I_{4}\left( \Omega _{n}\right)&=2\cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) \\&\int _{0}^{\alpha }\sin \bigg (\Omega _{n}\int _{\alpha }^{x}\frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg ) dx. \end{aligned} \end{aligned}$$

(A.13)

Let

$$\begin{aligned} I_{5}\left( \Omega _{n}\right) =2\int _{0}^{\alpha }\sin \bigg (\Omega _{n}\int _{\alpha }^{x} \frac{1}{\sqrt{-2V\left( s\right) }}ds\bigg )dx, \nonumber \\ \end{aligned}$$

(A.14)

so $I_{4}\left( \Omega _{n}\right) =I_{5}\left( \Omega _{n}\right) \cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right)$.

Thus we obtain the Melnikov function Eq. (14) is as follows:

$$\begin{aligned}{} & {} M\left( \tau _{0}\right) \nonumber \\{} & {} \quad =-2\,\rho \left[ V(0)-V(\alpha )\right] -\delta I_{1} +FI_{3}\left( \Omega \right) \sin \left( \Omega \tau _{0}\right) \nonumber \\{} & {} \qquad -\eta \sum _{n=0}^{\infty }a_{n}\left( m\right) I_{5}\left( \Omega _{n}\right) \cos \left( \Omega _{n}\tau _{0}+\left( 2n+1\right) \varphi \right) .\nonumber \\ \end{aligned}$$

(A.15)