1 Introduction

Fractional calculus has mesmerizing features due to its pragmatic applications in various areas of science, social science, finance, and engineering to mention a few. Owing to this, a lot of meaningful definitions that have to do with fractional derivatives have been proposed by different authors in order to fully explain the memory effect [1–4]. Among the existing derivatives, we mention Grunwald–Letnikov, Marchaud, Riemann–Liouville, Hadamard, modified Riemann–Liouville, and Caputo [5–10].

Recently, a new definition of derivative has been introduced, and it is called the conformable derivative. The newly introduced conformable derivative satisfies a lot of characteristics such as product and quotient formulas, and it is used to model some physical problems [11]. Several authors have utilized this definition in the real world problems [12–16].

Cls have originated from the pragmatic phenomena such as energy, mass, and momentum [17]. The Cls have been utilized for developing numerical techniques, proving the existence and uniqueness of solutions [18], analysis of the internal characteristics like recursion operators, bi-Hamiltonian structures [19]. It should be noted that there have been numerous generalizations of Noether’s theorem and Euler–Lagrange’s [20] associating to several definitions of fractional derivative to establish Cls for fractional nonlinear PDEs possessing fractional Lagrangians [21–23]. Furthermore, nonlinear physical phenomena may be explained through establishing exact solutions. This has brought a strong motivation to authors to obtain exact solutions using different schemes [24–51].

In the present paper, we investigate the Cls and soliton-like solutions of the time fractional DLWS with RL and conformable derivatives, respectively. Moreover, we compute the Cls via the multiplier technique and the stability analysis via the concept of linear stability of the integer order DLWS.

2 Basic tools

In this section, some preliminaries pertaining to symmetry analysis for time fractional PDEs will be highlighted. Let us assume that we have a system of time fractional PDEs given as

$$ \begin{gathered} \frac{\partial^{\alpha}U}{\partial T}=F(X, T, U, V, U_{XX}, U_{XXX},\ldots), \\ \frac{\partial^{\alpha}V}{\partial T}=F(X, T, U, V, U_{XX}, U_{XXX},\ldots). \end{gathered} $$
(1)

Suppose also that a one-parameter Lie group of transformations is given as follows:

$$ \begin{gathered} \bar{X}=X+\epsilon\xi_{1}(X,T,U,V)+O\bigl( \epsilon^{2}\bigr), \\ \bar{T}=T+\epsilon\xi_{2}(X,T,U,V)+O\bigl(\epsilon^{2} \bigr), \\ \bar{U}=U+\epsilon\eta_{1}(X,T,U,V)+O\bigl(\epsilon^{2} \bigr), \\ \bar{V}=V+\epsilon\eta_{2}(X,T,U,V)+O\bigl(\epsilon^{2} \bigr), \\ \frac{\partial^{\alpha} \bar{U}}{\partial \bar{T}^{\alpha}}=\frac{\partial^{\alpha}U}{\partial T^{\alpha}}+\epsilon \eta_{1}^{\alpha,T}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial^{\alpha} \bar{v}}{\partial \bar{t}^{\alpha}}=\frac{\partial^{\alpha}v}{\partial t^{\alpha}}+\epsilon \eta_{2}^{\alpha,t}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial\bar{U}}{\partial\bar{X}}=\frac{\partial U}{\partial X}+\epsilon\eta_{1}^{X}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial\bar{V}}{\partial\bar{X}}=\frac{\partial V}{\partial X}+\epsilon\eta_{2}^{X}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial^{2} \bar{U}}{\partial \bar{X}^{2}}=\frac{\partial^{2} U}{\partial X^{2}}+\epsilon \eta_{1}^{XX}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial^{2} \bar{V}}{\partial\bar{X}^{2}}=\frac{\partial^{2} V}{\partial X^{2}}+\epsilon\eta_{2}^{XX}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial^{3} \bar{U}}{\partial \bar{X}^{3}}=\frac{\partial^{3} U}{\partial X^{3}}+\epsilon \eta_{1}^{XXX}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \\ \frac{\partial^{3} \bar{V}}{\partial\bar{X}^{3}}=\frac{\partial^{3} U}{\partial X^{3}}+\epsilon\eta_{2}^{XXX}(X,T,U,V)+O \bigl(\epsilon^{2}\bigr), \end{gathered} $$
(2)

where \(\xi_{1}\), \(\xi_{2}\), \(\eta_{1}\), \(\eta_{2}\) are the infinitesimal operators, \(\eta_{1}^{\alpha,t}\), \(\eta_{2}^{\alpha,t}\) are the extended infinitesimals of order α, and \(\eta_{1}^{X}\), \(\eta_{2}^{X}\), \(\eta_{1}^{XX}\), \(\eta_{2}^{XX}\), \(\eta_{1}^{XXX}\), \(\eta_{1}^{XXX}\) are the integer order extended infinitesimals. Surmise that we have vector fields as follows:

$$ \textbf{X}=\xi_{1}\frac{\partial}{\partial X}+\xi_{2} \frac{\partial}{\partial T}+\eta_{1}\frac{\partial}{\partial U}+\eta_{2} \frac{\partial}{\partial V}. $$
(3)

Equation (3) exhibits a point symmetry of Eq. (1) such that

$$ P^{\alpha, i}r \textbf{X}(\bigtriangleup)\big|_{\bigtriangleup=0}=0, $$
(4)

i exhibits an order Eq. (1). Also, for the invariance condition, we have

$$ \xi_{2}(X, T, U, V)\big|_{T=0}=0. $$
(5)

The αth extended infinitesimal related to the RL fractional time derivative with Eq. (7) is given as in [38, 40, 41].

3 The models

The time fractional dispersive long-wave system is given by

$$ \begin{gathered} \frac{\partial^{\alpha}U}{\partial T^{\alpha}}=\bigl(U^{2} -U_{X} +2V \bigr)_{X}, \\ \frac{\partial^{\alpha}V}{\partial T^{\alpha}}=(2UV+V_{X})_{X},\end{gathered} $$
(6)

where \(0<\alpha\le1\). If \(\alpha=1\), Eq. (6) becomes

$$ \begin{gathered} \frac{\partial U}{\partial T}=\bigl(U^{2} -U_{X} +2V \bigr)_{X}, \\ \frac{\partial V}{\partial T}=(2UV+V_{X})_{X}.\end{gathered} $$
(7)

Equation (7) has well been known as DLWE [52]. In the concept of the spectral transform, Eq. (7) is analyzed in [52] and thereafter in [53], where it was related to the Schrödinger equation with linear spectral dependence in the potential. In hydrodynamics it exhibits the evolution of the horizontal velocity component of water waves propagating in both directions in an infinite narrow channel of constant depth and could be generated from the water wave equations by including one more order of nonlinearity than is done in deriving the Boussinesq equation. The integrability and derivation for Eq. (7) was presented in [54]. Furthermore, in [55] the authors related it directly to the spectral problem

$$ \bigl(\partial_{X}+\alpha+\beta\partial^{-1}_{X} \bigr)\phi=\lambda\phi. $$

Considering the invariance of Eq. (6) under the group of transformations Eq. (2), we get the following:

$$\begin{aligned}& \eta^{\alpha,T}_{1}-2U\eta^{X}_{1}+2 \eta^{X}_{2}+\eta^{XX}_{1}=0, \end{aligned}$$
(8)
$$\begin{aligned}& \eta^{\alpha,T}_{1}-2U\eta^{X}_{1}+2V \eta^{X}_{2}+\eta^{XX}_{2}=0. \end{aligned}$$
(9)

Inserting the prolongations, we obtain determining equations. Solving the obtained determining equations, we acquire

$$ \xi_{1}=c_{3}\alpha X +c_{4},\qquad \xi_{2}=C_{1} +2Tc_{3}, \qquad\eta_{1}=-Uc_{3} \alpha, \qquad\eta_{2}=-2Vc_{3} \alpha, $$
(10)

and the corresponding Lie algebra is generated by the vector field below:

$$ X_{1}=\frac{\partial}{\partial X},\qquad X_{2}=\frac{\partial}{\partial T},\qquad X_{3}=\alpha X \frac{\partial}{\partial X}+2T\frac{\partial}{\partial T}-U\alpha \frac{\partial}{\partial U}-2V\alpha\frac{\partial}{\partial V}. $$
(11)

The solutions of Eq. (11) lead to the following theorem.

Theorem 1

By using the similarity transformations \(U=T^{-\frac {\alpha}{2}}f(\xi)\) and \(V=T^{-\alpha}g(\xi)\) with the similarity variable \(\xi=XT^{-\frac{\alpha}{2}}\), Eq. (6) attains to

$$ \begin{gathered} \bigl(P^{1-\alpha-\frac{\alpha}{2}, \alpha}_{\frac{2}{\alpha}}f \bigr) (\xi)- \bigl(f^{2}-f_{\xi}+2g\bigr)_{\xi}=0, \\ \bigl(P^{1-2\alpha, \alpha}_{\frac{2}{\alpha}}g \bigr) (\xi)-(2fg+g_{\xi})_{\xi}=0.\end{gathered} $$
(12)

Proof

Similar steps can be found in [41]. □

3.1 Conservation laws for Eq. (6)

Several works involving the procedures for computing Cls of fractional PDEs were presented in numerous research works [56–60]. In this work, we apply the procedures presented in [56, 57] to establish Cls for Eq. (6). Surmise that the formal Lagrangian for Eq. (6) is given as

$$ \begin{aligned}[b] \mathcal{L}={}&p(X,T) \biggl(\frac{\partial^{\alpha} U}{\partial T^{\alpha}}-2UU_{X}+U_{XX}-2V_{X} \biggr)\\ &+q(X,T) \biggl(\frac{\partial^{\alpha}V}{\partial T^{\alpha}}-2VU_{X}-2UV_{X}-V_{XX} \biggr).\end{aligned} $$
(13)

The adjoint equations can be presented as follows:

$$ \begin{gathered} \frac{\delta\mathcal{L}}{\delta U}=F^{*}_{1}=\bigl(D^{\alpha}_{T}\bigr)^{*}p-2up_{X}-p_{XX}+2Vq_{x}=0, \\ \frac{\delta\mathcal{L}}{\delta V}=F^{*}_{2}=\bigl(D^{\alpha}_{T} \bigr)^{*}q-2Uq_{X}-2p_{X}+q_{XX}=0.\end{gathered} $$
(14)

For Eq. (6) to be nonlinearly self-adjoint, Eq. (14) must hold for all solutions of Eq. (6) upon the following substitution:

$$ p=\Phi_{1}(X,T,U,V),\qquad q=\Phi_{2}(X,T,U,V), $$
(15)

such that \(\Phi_{i}\ne0\) for at least one i (\(i=1,2\)). The conditions for the nonlinear self-adjointness can be presented as follows:

$$ \frac{\delta\mathcal{L}}{\delta U}\bigg|_{\text{Eq. (15)}}=\lambda _{1}\biggl( \frac{\partial^{\alpha} U}{\partial T^{\alpha}}+\bigl(U^{2} -U_{X} +2V\bigr)_{X} \biggr)+\lambda_{2}\biggl(\frac{\partial V}{\partial T}+(2UV+V_{X})_{X} \biggr) $$
(16)

and

$$ \frac{\delta\mathcal{L}}{\delta V}\bigg|_{\text{Eq. (15)}}=\lambda _{3}\biggl( \frac{\partial^{\alpha} U}{\partial T^{\alpha}}+\bigl(U^{2} -U_{X} +2V\bigr)_{X} \biggr)+\lambda_{4}\biggl(\frac{\partial V}{\partial T}+(2UV+V_{X})_{X} \biggr). $$
(17)

Hence, applying Eq. (15) along with its associated derivatives in Eqs. (16) and (17) thereby solving the resulting expressions, the following solution is obtained:

$$ \begin{gathered} \lambda_{1}=\lambda_{2}= \lambda_{3}=\lambda_{4}=0, \\ \Phi_{1}(X,T,U,V)=0, \qquad\Phi_{2} (X,T,U,V)=A_{2}, \end{gathered} $$
(18)

where \(A_{2}\) is an arbitrary constant. Thus, Eq. (15) is nonlinearly self-adjoint.

The characteristic functions are given as follows:

$$ \begin{gathered} W_{1} =-U\alpha-2TU_{T}-\alpha X U_{X},\qquad W_{2} =-V\alpha-2TV_{T}-\alpha X V_{X}, \\ W_{3} =-U_{X},\qquad W_{4} =-V_{X} W_{5}=-U_{T},\qquad W_{5}=-V_{T}. \end{gathered} $$
(19)

Using Eq. (19) and setting \(A_{2} =1\), the conserved vectors are:

The X-components \(C^{X}_{i}\) associated with Eq. (19) are as follows:

$$\begin{gathered}\begin{aligned} C^{X}_{1} ={}&2(U+V) (-U\alpha-2TU_{T}-\alpha X U_{X}-V\alpha-2TV_{T}-\alpha X V_{X}) \\ & - \bigl((U_{X}+V_{X})+\alpha X(U_{XX}+V_{XX})+2T(U_{XT}+V_{XT}) \bigr),\end{aligned} \\ C^{X}_{2} =2(U+V) (U_{X} +UV_{X})+U_{XX}+V_{XX}, \\ C^{X}_{3} =2(U+V) (U_{T} +UV_{T})+U_{XX}+V_{XX}.\end{gathered} $$

The t-components \(C^{t}_{i}\) are given as follows:

Case 1. When \(\alpha\in(0,1)\), the conserved vectors are

$$\begin{gathered}\begin{aligned} C^{T}_{1} ={}&{-}\alpha\bigl(I^{1-\alpha}_{T}(U)+I^{1-\alpha}_{T}(V) \bigr) \\ & -\alpha X \bigl(I^{1-\alpha}_{T}(U_{X})+I^{1-\alpha}_{T}(V_{X}) \bigr)-2\bigl(I^{1-\alpha }_{T}(TU_{T})+I^{1-\alpha}_{T}(TV_{T}) \bigr),\end{aligned} \\ C^{T}_{2} =-I^{1-\alpha}_{T}(V_{X}).\end{gathered} $$

Case 2. When \(\alpha\in(1,2)\), the conserved vectors are

$$\begin{gathered}\begin{aligned} C^{T}_{1} ={}&{-}\alpha\bigl(D^{1-\alpha}_{T}(U)+D^{1-\alpha}_{T}(V) \bigr) \\ & -\alpha X \bigl(D^{1-\alpha}_{T}(U_{X})+D^{1-\alpha}_{T}(V_{X}) \bigr)-2\bigl(D^{1-\alpha }_{T}(TU_{T})+D^{1-\alpha}_{T}(TV_{T}) \bigr),\end{aligned} \\ C^{T}_{2} =-D^{1-\alpha}_{T}(V_{X}).\end{gathered} $$

4 Soliton-like solutions

In this section, by means of the conformable derivative [11, 12] and the GPR method [61], some soliton-like solutions will be presented for Eq. (6). Applying the conformable derivative and plugging the transformation \(U(X,T))=U(\eta)\), \(V(X,T)=V(\eta)\), \(\eta=k(X-\Lambda\frac{T^{\alpha}}{\alpha})\) in Eq. (6) yields

$$ \begin{gathered} k^{2} U''-k \Lambda U'-2 k U U'-2 k V'=0, \\ k^{2} V''+2 k V U'+2 k U V'+k \Lambda V'=0. \end{gathered} $$
(20)

According to the GPR method [61], applying homogeneous principles in Eq. (20), we can have the following solutions:

$$ \begin{gathered}U(\eta)=A_{0}+A_{1} \sigma( \eta)+B_{1} \tau(\eta), \\ V(\eta)=F_{0}+F_{1} \sigma(\eta)+G_{1} \tau ( \eta), \end{gathered} $$
(21)

where \(A_{0}\), \(A_{1}\), \(B_{1}\), \(F_{0}\), \(F_{1}\), \(G_{1}\) are constants and will be found later. The functions \(\sigma(\eta)\) and \(\tau(\eta)\) satisfy the ODE

$$\begin{aligned}& \sigma'(\eta)=\epsilon\sigma(\eta)\tau(\eta), \end{aligned}$$
(22)
$$\begin{aligned}& \tau'(\eta)=R+\epsilon\tau^{2}(\eta)-\mu\sigma(\eta),\quad \epsilon=\pm 1, \end{aligned}$$
(23)

where

$$ \tau^{2}(\eta)=-\epsilon \biggl(R-2\mu\sigma(\eta)+\frac {\mu^{2}-1}{R} \sigma^{2}(\eta) \biggr), $$
(24)

where R and μ are nonzero constants. Plugging Eq. (21) along with Eqs. (22) and (23) into Eq. (20), we obtain large algebraic expressions. Collecting terms in \((\sigma(\eta))^{3}\), \((\sigma(\eta))^{2}\), \((\sigma(\eta))^{1}\), \((\sigma(\eta))^{0}\), \((\sigma(\eta))^{2}\tau(\eta)\), \((\sigma(\eta))\tau(\eta)\), \(\tau (\eta)\) from the obtained algebraic expressions gives systems of algebraic expressions. Solving the obtained systems yields the following.

Results: \(\epsilon=-1\), \(A_{0}=A_{0}\), \(\Lambda=-2 A_{0}\), \(A_{1}=0\), \(B_{1}=B_{1}\), \(F_{0}=0\), \(F_{1}=\frac{1}{2} B_{1} \mu (2 B_{1}+k )\), \(G_{1}=0\), \(\mu R\neq0\). These results give the following soliton-like solutions: For \(\epsilon=-1\), \(R\ne0\), we acquire the soliton-like solution

$$ \begin{gathered}U(X,T)=A_{0}+B_{1} \frac{R \operatorname{tanh}(\sqrt{R}\eta)}{\mu \operatorname{sech}(\sqrt{R}\eta)+1}, \\ V(X,T)=\frac{1}{2} B_{1} \mu (2 B_{1}+k ) \frac{R \operatorname{sech}(\sqrt{R}\eta)}{\mu \operatorname{sech}(\sqrt{R}\eta)+1}, \end{gathered} $$
(25)

and

$$ \begin{gathered}U(X,T)=A_{0}+B_{1} \frac{R \operatorname{coth}(\sqrt{R}\eta)}{\mu \operatorname{csch}(\sqrt{R}\eta)+1}, \\ V(X,T)=\frac{1}{2} B_{1} \mu (2 B_{1}+k ) \frac{R \operatorname{csch}(\sqrt{R}\eta)}{\mu \operatorname{csch}(\sqrt{R}\eta)+1}, \end{gathered} $$
(26)

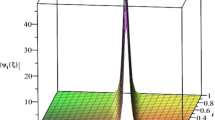
where \(\eta=k(X-\Lambda\frac{T^{\alpha}}{\alpha})\). Some physical features of the obtained soliton-like are illustrated in Figs. 1 and 2.

Figure 1
figure 1

3D plot of (25), with \(\alpha=0.75\), \(\mu=1.5\), \(k=2\), \(A_{0}=B_{1}=1\), respectively

Full size image
Figure 2
figure 2

3D plot of (26), with \(\alpha=0.75\), \(\mu=1.5\), \(k=2\), \(A_{0}=B_{1}=1\), respectively

Full size image

5 Conservation laws for Eq. (7) by multiplier

The description for Cls via the multiplier technique was presented in [62]. Here, we apply the same process to obtain Cls for the integer order of DLWE reported in Eq. (7) by using a first order multiplier that is \(\Lambda^{1}(X,T,U,V,U_{X},V_{X},U_{T},V_{T})\), \(\Lambda^{2}(X,T,U,V,U_{X},V_{X},U_{T},V_{T})\). We obtain the first order multiplier for Eq. (7) given by

$$ \begin{gathered}\Lambda^{1}=uc_{2}, \\ \Lambda^{2}=-2c_{1}UV+c_{2}U^{2}-c_{1}V_{X}-c_{2}U_{X}+2c_{2}V, \end{gathered} $$
(27)

where \(c_{1}\), \(c_{2}\) are arbitrary constants. Therefore, the multipliers for the non trivial local Cls involving the cases isolated by free constants can be obtained as follows:

1.

$$ \begin{gathered} \Lambda^{1}=0, \\ \Lambda^{2}=1, \end{gathered} $$
(28)

which gives the following fluxes:

$$ \begin{gathered} \textbf{C}^{T}=0,\\ \textbf{C}^{X}=-2UV-V_{X}. \end{gathered} $$
(29)

2.

$$ \begin{gathered} \Lambda^{1}=1, \\ \Lambda^{2}=0, \end{gathered} $$
(30)

which yields the following fluxes:

$$ \begin{gathered} \textbf{C}^{T}=U,\\ \textbf{C}^{X}=U^{2}-U_{X}+2V. \end{gathered} $$
(31)

5.1 Stability analysis to Eq. (7)

In this subsection, the concept of linear stability analysis [63–65] will be applied to investigate the stability analysis for the governing equation. Consider the integer order for DLWE as in Eq. (7). Then, by considering the perturbed solution of the form

$$ \begin{gathered} U(X,T)=P_{1}+\epsilon w(X,T), \\ V(X,T)=P_{2}+\tau r(X,T), \end{gathered} $$
(32)

it is easy to see that any constants \(P_{1}\) and \(P_{2}\) are a steady state solution of Eq. (7). Plugging Eq. (32) to Eq. (7), we obtain

$$ \begin{gathered} -\epsilon w_{T}+2 \tau r_{X}+2 \epsilon P_{1} w_{X}+2 \epsilon^{2} w w_{X}-\epsilon w_{XX}=0, \\ -\tau r_{T}+2 \tau P_{1} r_{X}+2 \epsilon \tau w r_{X}+2 \epsilon\tau r w_{X}+2 \epsilon P_{2} w_{X}+\epsilon w_{XX}=0. \end{gathered} $$
(33)

Linearizing (33) in ϵ and τ gives

$$ \begin{gathered} k^{2} \epsilon \alpha_{1}-i \epsilon \omega \alpha_{1}+2 i k \epsilon P_{1} \alpha_{1}+2 i k \tau \alpha_{2}=0, \\ k^{2} \epsilon \alpha_{1}-2 i k \epsilon P_{2} \alpha_{1}+i \tau \omega \alpha_{2}-2 i k \tau P_{1} \alpha_{2}=0. \end{gathered} $$
(34)

Surmise that Eq. (34) has solutions given by

$$ \begin{gathered} w(X,T)=\alpha_{1} e^{(ikX+i\omega T)}, \\ r(X,T)=\alpha_{2} e^{(ikX+i\omega T)}, \end{gathered} $$
(35)

k denotes a normalized wave number. Plugging Eq. (35) into Eq. (34) yields

$$ \begin{gathered} ik^{2} \epsilon\beta\alpha_{1}+ \epsilon\omega\alpha_{1}+k\epsilon P_{1}\alpha_{1}+k \tau\alpha_{2}=0, \\ -ik^{3}\alpha\epsilon\alpha_{1}+ik\epsilon P_{2} \alpha_{1}+k^{2}\beta\tau\alpha_{2}+i\tau\omega \alpha_{2}+ik\tau P_{1}\alpha_{2}=0. \end{gathered} $$
(36)

Collecting terms with \(\alpha_{1}\), \(\alpha_{2}\) gives

$$ \left ( \textstyle\begin{array}{c@{\quad}c} \epsilon(k^{2}-i\omega+2ikP_{1})& 2ik\tau\\ k\epsilon(k-2iP_{2}) & i\tau(\omega-2kP_{1}) \end{array}\displaystyle \right )\left ( \textstyle\begin{array}{c} \alpha_{1}\\ \alpha_{2} \end{array}\displaystyle \right )=\left ( \textstyle\begin{array}{c} 0\\ 0 \end{array}\displaystyle \right ), $$
(37)

and taking the determinant of the above yields

$$ -2 i \alpha_{1} k^{3} \tau \epsilon-2 i k^{3} P_{1} \tau \epsilon-4 \alpha_{1} k^{2} P_{2} \tau \epsilon+4 k^{2} P_{1}^{2} \tau \epsilon+i k^{2} \tau \omega \epsilon-4 k P_{1} \tau \omega \epsilon+\tau \omega^{2} \epsilon=0. $$
(38)

Solving for ω yields

$$ \omega(k)=\frac{1}{2} \bigl(4kP_{1}-i \bigl(k^{2}- \sqrt {k^{4}+8ik^{3}-16k^{2}P_{2}} \bigr) \bigr). $$
(39)

The relations for the dispersion in Eq. (39) will be investigated. The sign of the real part (Re) of ω suggests that either the solution will become bigger or vanish in a given period of time. When the sign of Re for \(\omega(k)\) is negative for all k, thus any superposition of \(e^{(i\omega t + ikx)}\) will come to vanished. Moreover, if the Re is positive for some k, then with time some components of a superposition will become much bigger. The former case is said to be stable, otherwise unstable. If the maximum of the Re is exactly zero, then it is said to be marginally stable. It is more difficult to assess the long term behavior in this case. Thus, from Eq. (39) one can observe that the Re is always negative for \(k<0\), \(P_{1}>0\) and \(k>0\), \(P_{1}<0\), which implies that the dispersion relation is stable. If \(k<0\), \(P_{1}<0\) and \(k>0\), \(P_{1}>0\), the Re will be positive, hence in this case the dispersion is unstable. When \(k=0\), the Re will be zero, which suggests that the dispersion is marginally stable in this case. In order to see the mechanism of Eq. (39), we plot Fig. 3.

Figure 3
figure 3

Frequency of the perturbation against the wave number with different parameter values

Full size image

6 Conclusion

We investigated time fractional DLWE and its corresponding integer order. The symmetry properties and reductions were derived. We constructed the Cls with RL for the time fractional DLWE via new conservation theorem. The conformable derivative was employed to establish soliton-like solutions for the time fractional DLWE by using the generalized projective method (GPM). Moreover, the Cls via the multiplier technique and the stability analysis via the concept of linear stability analysis for the integer order DLWE were established. Some graphical features for the obtained results were also presented.