Payments from Households to Distant Polluting Firms

Abstract

We investigate a novel way to encourage separation between firms, causing local pollution, and their victims (households): payments from households to distant polluting firms. These payments do not require monitoring of firms’ emissions or their abatement costs. In our model, households and firms can choose from two locations (A and B, with A larger than B). Households incur environmental damage from firms in the same location. Under laissez faire, payments from households in one location (say A) to firms in the other location (say B) will prompt firms to move from A to B and to stay there, thus reducing damage to households in A. The maximum that households are willing to pay temporarily is the amount that currently makes them indifferent between A and B. The payments make A less attractive to firms as well as to households. The unique positive-payment equilibrium implements the global welfare optimum where laissez faire does not. We examine from which starting points this payment equilibrium can be reached.

1 Introduction

Many environmental problems concern local pollution which can be alleviated or even resolved by moving polluters and victims away from each other. In a world where environmental regulation is difficult to accept politically, can payments between polluters and victims give the right incentives for location choice? This could be an option not just for pollution in the form of substances, but also in other forms, such as visual (e.g. wind turbines [Gibbons 2015; Dröes and Koster 2016], notwithstanding their supply of renewable energy), noise (Klaiber and Morawetz 2021) and light pollution.

Environmental buyouts, where polluters pay victims to move away (or vice versa), have emerged in the US as a way of separating polluters and victims (Guttel and Leshem 2013a, b). In the most spectacular case, in 2002 the electricity company AEP effectively paid for everyone in the small town of Cheshire, Ohio, to move and not to sue the company for any pollution damage from its nearby power plant (Buckley et al. 2005; Martin 2014).

The buying out of polluters by victims happens relatively rarely,¹ due to the free rider problem among victims and the transaction costs of coordinating a large number of victims. However, there are ways in which the victims can organize themselves. As more and more houses were being built around the Williams feedlot in Santa Maria (CA), complaints grew about its odour, dust and flies (Kolstad 2011, p. 259). At a public meeting in June 1996, local residents supported a proposal to pay Mr Williams $600,000 over ten years to close the feedlot (Bedell 1996a). A Benefit Assessment District would be drawn up of all properties affected by the feedlot. They would pay an extra $1.82 per month in property tax for 10 years. The city council sent all property owners an information leaflet with a cutout coupon they could return to register their response (Bedell 1996b). If more than half of the property owners disagreed in this way, the plan would not go ahead. In the event, only 14% of the approximately 2,086 property owners registered their disagreement (Bedell 1996c). Thus the city council approved the plan and bought out the feedlot. Although this kind of buyout by pollution victims is rare, it may become more prevalent in a world where right-wing governments are looking to soften environmental regulation and enforcement (Popovich et al. 2021; Schaeffer and Pelton 2019; Kaiser 2019).

To our knowledge, this paper is the first to analyze a particular variation to environmental buyouts where victims, possibly with the aid of their local government, pay polluters to stay away from them. Does this kind of payment lead to a better outcome for society or perhaps even the welfare optimum? We analyze this question in Dijkstra and de Vries' (2006) model with two locations, A and B, where A is larger than B. Households suffer from the pollution of firms in the same location.² There is nothing that firms or households can do to reduce pollution, except for move away from each other. There is no environmental policy. There may be two stable equilibria and two local welfare optima, one with households mostly in A and one with firms mostly in A. The former is the global welfare optimum, because it has the largest population in the largest area.

In this world, households in A (for instance) might come up with the idea of paying firms to move to B and to stay in B. We shall examine these payments in an evolutionary setting. This means that a payoff difference between A and B does not cause an immediate rush to the higher-payoff region, instantly restoring payoff equality. Rather, there will be a stream of migration over time to the higher-payoff location. This evolutionary setting, which we share with Dijkstra and de Vries (2006), allows us to determine whether payments help an economy to move to the good equilibrium rather than the bad equilibrium.

In an evolutionary setting, we also have to examine how payments from households to firms came about. We shall assume that households in A will only pay firms in B if households prefer A to B, given the current locations of households and firms. Then households feel attached to A and they would like to make it a better place to live. The evolutionary setting implies that households may not be able to predict exactly how payments will affect future migration streams. There may thus be some experimenting with payments, and payments might be set at a level that was wrong in hindsight.

We shall see that in the positive-payment equilibrium, all households are in A, setting the payment level that maximizes their payoff, given that firms are indifferent between A and B. Since the households own all the land and the firms, they take all effects of their payments into account such as to maximize welfare. They do not impose an externality on households in B, because there are no households there. The Coase theorem (Coase 1960; Medema 2020) holds in this sense, under the assumptions of perfect information, no free riding and no transaction costs.³ However, even under these assumptions, the welfare optimum cannot be reached from everywhere.

As discussed above, households may not have perfect knowledge of the effect of their payments all the time. However, we can expect that households learn the effect over time, so that eventually they can determine the payment that maximizes their payoff. Imperfect information is thus not an impediment to reaching the social optimum.

With a large number of households in A, free riding is a serious problem (Dixit and Olson 2000). Why should a household in A pay firms in B when the effect on its own payoff is minimal (and long-term), instead of free riding on other households’ payments? We shall assume that households have an effective way of dealing with free riding. As with the Santa Maria feedlot, there might be a local authority that can put the decision to pay firms in B to a referendum among all households in A. If the vote is in favour, the authority can collect the payments from all households.⁴

A key question we shall analyze is whether payments from households to firms can set the economy on a path to a different (hopefully better) equilibrium. Consider the case where households as well as firms are moving from B to A. Households in A might then pay firms in B. This will slow down firms’ movement from B to A. If the payment is high enough, it might even induce firms to revert from A to B. However, the payment reduces the payoff advantage of A for households as well and will thus also slow down households’ movement from B to A. In order to determine how payments affect the path of the economy, we have to analyze their effect on the relative migration speeds of firms and households. We also need to consider different scenarios of how the imperfectly informed households set the payment level.

The rest of this paper is organized as follows. We review the literature in Sect. 2. The model is set out in Sect. 3. Sect. 4 presents the welfare optima and the laissez-faire equilibria. In Sect. 5 we introduce payments from households to firms and derive the equilibrium. In Sect. 6 we analyze how the payments affect the relative relocation speeds of households and firms. Sect. 7 combines the dynamics of payments and location choice to examine an economy’s path to equilibrium. In Sect. 8 we consider extensions to our model. Finally, Sect. 9 concludes.

2 Literature Review

In this literature review we shall focus on welfare maximization, laissez faire and payments between polluters and victims in a spatial context with externalities.

Migrating to a cleaner or quieter location (Klaiber and Morawetz 2021) is a defensive activity (also called avoidance or averting behaviour) by pollution victims. Other examples include spending more time indoors using air conditioning (Sheldon and Sankara 2019) and wearing facemasks outdoors in response to air pollution alerts (Ward and Beatty 2016; Kim 2021), and drinking bottled water (Zivin et al. 2011). Baumol (1972) and Starrett (1972) were the first to note that these defensive activities introduce nonconvexities into the production set and/or consumer preferences, which may result in multiple welfare optima.⁵ Shibata and Winrich (1983) show that when it is (locally) optimal to have only victim defence measures and no abatement by firms, laissez faire implements the optimum.⁶

Parker (2007) examines the occupation of a line by three users: conventional and organic agriculture, and an alternative use. Proximity to conventional agriculture is damaging for organic agriculture. Under laissez faire, the conventional and organic farms will be on opposite ends of the line, but too close to each other: the buffer zone with the alternative use is too small. Parker (2007) presents three mechanisms for reaching the social optimum: a Pigouvian tax on land, making the conventional farmer liable for the damage to the organic farmer, and Coasean bargaining between the three parties where the conventional farmer holds the right to pollute. Unlike Parker (2007), our model is dynamic and spatially discrete, featuring two land uses instead of three and a continuum of agents rather than one agent for each land use.

Copeland and Taylor (1999) consider a two-country two-industry world with free trade and without environmental policy. The "Smokestack" industry adds to the national stock of pollution and the "Farming" industry suffers from pollution.⁷ Analogous to Miyao (1978), Copeland and Taylor (1999) find that the diversified autarky equilibrium is unstable and there may be two stable equilibria with at least partial specialization. When one country needs to be diversified, this should be the country with the lowest labour endowment or the largest regenerative capacity. However, Copeland and Taylor (1999) show that the world does not necessarily evolve to the desired specialization pattern.

Van Marrewijk (2005), Lange and Quaas (2007) and Wu and Reimer (2016) add local pollution to Forslid and Ottaviano's (2003) core-periphery economic geography model in order to study stable equilibria under laissez faire. Van Marrewijk (2005) assumes both manufacturing and agriculture are polluting (affecting household utility) and derives conditions under which complete agglomeration (the core-periphery outcome) and perfect spreading are stable equilibria. Lange and Quaas (2007) assume only manufacturing is polluting. They show that partial agglomeration can also be a stable equilibrium. Both papers find conditions under which there are two stable equilibria. Wu and Reimer (2016) assume a clean and a dirty industry and show that the dirty industry may be too dispersed in the spatial equilibrium.

While the core-periphery model is also a two-location model, there are several differences with our model. In our model, for simplicity there are no agglomeration forces, so that the laissez-faire stable equilibria are always corner solutions. Secondly, there is an ex ante difference between the two locations in our model (A is larger than B).⁸ Thirdly, we have two slow-moving populations (firms and households) while the core-periphery model, apart from Wu and Reimer’s (2016) application, only has one (human capital). Thirdly, we model the land rental market.

Chen et al. (2012) model agents’ location choice between two cities: The dirty city where all the manufacturing takes place and the clean city. In equilibrium the agents with the highest ability to work live in the clean city, trading off a cleaner environment against higher commuting costs. The authors find that there can be multiple equilibria, differing in the relative size of the two cities. While Chen et al. (2012) assume only households are mobile, we also include firm mobility, which allows us to analyze the location choice effects of payments from households in one area to firms in the other area. Unlike Chen et al. (2012), we model the land rental market, but we abstract from household heterogeneity and commuting costs.

Our paper builds on Dijkstra and de Vries (2006), who set up a model with two locations and two mobile populations. Firms cause environmental damage to households in the same location. The authors analyze the policy regimes of laissez faire, taxation and compensation. They find that each stable equilibrium features at least partial separation, with at least one area hosting one group only. Taxation always implements a (local) welfare optimum. However, laissez faire and compensation can also implement a welfare optimum, as long as there is complete separation, or the population that is in both regions takes environmental damage into account. Finally, Dijkstra and de Vries (2006) find there are starting points from which the economy evolves to only a local wefare optimum under taxation, but to the global optimum under compensation. The authors mention in the Conclusion that under laissez faire, households could pay firms to stay away from them. In this paper, we take the model and the laissez faire scenario from Dijkstra and de Vries (2006), and we add these payments.

Pitchford and Snyder (2003) and Innes (2008) analyze Coasean bargaining in a sequential location decision game of "coming to the nuisance". In both papers, party B considers moving close to party A, setting off a negative externality between the two parties. The property rights consist of injunction, damage or exclusion rights for the first or the second party. Pitchford and Snyder (2003) show that only second-party damage rights lead to the optimal outcome if A can make an ex-ante non-contractible investment.⁹ Innes (2008) shows that only first-party damage rights lead to the optimal outcome in case relocation by A would result in another negative externality.

Guttel and Leshem (2013a) show how welfare can fall when a polluter buys out some of his victims.¹⁰ While our paper only considers payments from victims to polluters, payments in the opposite direction can be analyzed in the same way. However, there are important differences between Guttel and Leshem (2013a) and our paper. Guttel and Leshem (2013a) provide a static partial-equilibrium model of a single polluter and many victims, where the polluter is required to take abatement measures such that total welfare is maximized. The present paper provides a dynamic general-equilibrium model of many polluters and many victims, where the polluters cannot take abatement measures and there is no environmental policy. In Guttel and Leshem’s (2013a) model, the polluter can strategically reduce the amount of abatement required by buying out some victims, thereby reducing the harm from his pollution. Buying out some victims imposes a negative externality on the other victims. In the present paper, all the victims of the same location act as one, mirroring Guttel and Leshem’s (2013a) single polluter. In our general equilibrium setting, the victims are also the consumers, the shareholders and the land owners. Moreover, in the positive-payment equilibrium, all households are in the same location. Thus the households take all the effects of their payments into account, thereby implementing the social optimum, given their own location.

3 The Model

Our model builds on Dijkstra and de Vries (2006). There are two populations k: Households h and firms f. There is a continuum of households of mass 1. There is a continuum of firms of mass $n_{f}<1.$ Households and firms can locate in two regions $z,\ z=A,B$. Denote by $s_{k}^{z}$ the share of population k located in region z. There is a fixed total area of land in either region that can be occupied.¹¹ Each household owns an equal share of land in A and in B. Region A’s size is normalized to one. Region B is of size $\beta <1,$ with $b\equiv 1/\beta >1.$

The firms f in region z produce a homogeneous good q with land $g_{f}^{z}$ and a firm-specific indivisible factor $F_{f}$, with $F_{f}=1.$ Each household owns an equal share in each firm’s $F_{f}.$ The production $q_{f}^{z}$ of q by a firm in region z is given by:¹²

$$\begin{aligned} q_{f}^{z}=q_{f}(F_{f},g_{f}^{z})=F_{f}\left( \phi -\frac{\theta F_{f}}{ 2g_{f}^{z}}\right) \end{aligned}$$

(1)

with $\phi ,\theta >0$. The production technology features constant returns to scale and thus diminishing marginal returns to land. $\phi$ is the maximum amount that a firm can produce with $F_{f}=1$ as $g_{f}^{z}$ goes to infinity.

The land rental market is perfectly competitive,  clearing instantaneously, with $p^{z}$ the rental price of land in area z. Firm f in z maximizes its operating profits $\Pi _{f}^{z}$ (revenue minus cost of renting land), which from (1) with $F_{f}=1$ and the choice of q as the numeraire are given by:

$$\begin{aligned} \Pi _{f}^{z}=\left\{ \max _{g_{f}^{z}}\ \phi -\frac{\theta }{2g_{f}^{z}} -p^{z}g_{f}^{z}\right\} \end{aligned}$$

(2)

Net utility from consumption and pollution for household h in region z is given by:

$$\begin{aligned} w_{h}^{z}=u(g_{h}^{z})+q_{h}^{z}-s_{f}^{z}n_{f} \end{aligned}$$

(3)

where $q_{h}^{z}$ is the consumption of q by a household in z and

$$\begin{aligned} u(g_{h}^{z})=\phi -\frac{\theta }{2g_{h}^{z}} \end{aligned}$$

(4)

is its utility from land. To simplify the analysis, we have set $u(g^{z})=q_{f}^{z}(1,g^{z}),$ as in Dijkstra and de Vries (2006).

The final term on the RHS of (3) is the environmental damage that the household incurs. Environmental damage from each firm to each household in the same region is constant and normalized to one. A household does not incur any damage from a firm located in the other region.¹³

A household h in region z maximizes its utility (3) under the budget constraint $p^{z}g_{h}^{z}+q_{h}^{z}=Y_{h}^{z},$ with $Y_{h}^{z}$ household h’s income in z. $Y_{h}^{z}$ is endogenous, but since utility ( 3) is quasilinear, household demand for land only depends on the land rental price and not on $Y_{h}^{z}$ (i.e. there is no income effect) or on environmental damage. The consumption good q is a residual on which the household spends all its remaining income.¹⁴ From (4), the household maximizes its consumer surplus $\Pi _{h}^{z}$ from land, given by:

$$\begin{aligned} \Pi _{h}^{z}=\left\{ \max _{g_{h}^{z}}\ \phi -\frac{\theta }{2g_{h}^{z}} -p^{z}g_{h}^{z}\right\} \end{aligned}$$

(5)

The solution to both firm (2) and household (5) maximization problems yields:

$$\begin{aligned} p^{z}=\frac{\theta }{2\left( g^{z}\right) ^{2}} \end{aligned}$$

(6)

as the inverse rental demand function for land with $g_{f}^{z}=g_{h}^{z}=g^{z}$, i.e. a firm and a household occupy the same amount of land in region z. Recall that land suppy is fixed at 1 in A and $\beta$ in B, so that in equilibrium the total area in each region is distributed equally among all occupants (firms plus households):

$$\begin{aligned} g^{A}=\frac{1}{n_{f}s_{f}^{A}+s_{h}^{A}},\ \ \ \ \ \ g^{B}=\frac{\beta }{ n_{f}(1-s_{f}^{A})+(1-s_{h}^{A})} \end{aligned}$$

(7)

Note that plot size $g^{z}$ in z is the inverse of density, i.e. the number of occupants on a given area of land in z. Substituting (7) into (6) gives $p^{A}$ as a function of the number of occupants:

$$\begin{aligned} p^{A}=\frac{\theta }{2}\left[ n_{f}s_{f}^{A}+s_{h}^{A}\right] ^{2},\ \ \ \ \ p^{B}=\frac{\theta b^{2}}{2}\left[ n_{f}(1-s_{f}^{A})+(1-s_{h}^{A})\right] ^{2} \end{aligned}$$

(8)

Substituting (6) into (2) and (5), the net payoff from land in region z is:

$$\begin{aligned} \Pi _{f}^{z}=\Pi _{h}^{z}=\Pi ^{z}=\phi -\frac{\theta }{g^{z}}=\phi -\sqrt{ 2\theta p^{z}} \end{aligned}$$

(9)

For the purpose of analyzing the model it is more convenient to solve for the endogenous $g^{z}$ and $p^{z}$ in (9) in order to express $\Pi ^{A}$ and $\Pi ^{B}$ as functions of $s_{f}^{A}$ and $s_{h}^{A}$. This is because the other elements of an agent’s payoff (pollution and payments) are also functions of $s_{f}^{A}$ and $s_{h}^{A}.$ Substituting (7) into the third equality of (9) yields:

$$\begin{aligned} \Pi ^{A}(s_{f}^{A},s_{h}^{A})=\phi -\theta n_{f}s_{f}^{A}-\theta s_{h}^{A},\ \ \ \ \ \Pi ^{B}(s_{f}^{A},s_{h}^{A})=\phi -\theta b\left[ n_{f}(1-s_{f}^{A})+1-s_{h}^{A}\right] \end{aligned}$$

(10)

We see that $\Pi ^{A}$ and $\Pi ^{B}$ are linear functions of $s_{f}^{A}$ and $s_{h}^{A}.$ From (2) and (5) to (10), the following relations between the variables are clear: The larger the number of occupants in a region, the higher is the density. The higher the density, the higher is the land rental price $p^{z},$ the smaller is the plot size $g^{z}$ and the lower is the net payoff $\Pi ^{z}$ from land in z.

Let $y\ (x)$ be the payment from each household in A (B) to each firm in B (A). A firm’s payoff $V_{f}^{z}$ in z equals its payoff $U_{f}^{z}=\Pi ^{z}$ from land plus any payment from households in the other region. From ( 10):

$$\begin{aligned} V_{f}^{A}= & {} U_{f}^{A}+x(1-s_{h}^{A}),\ \ \ \ \ \ U_{f}^{A}=\phi -\theta (n_{f}s_{f}^{A}+s_{h}^{A}) \end{aligned}$$

(11)

$$\begin{aligned} V_{f}^{B}= & {} U_{f}^{B}+ys_{h}^{A},\ \ \ \ \ \ \ \ \ \ \ \ \ U_{f}^{B}=\phi -b\theta n_{f}(1-s_{f}^{A})-b\theta (1-s_{h}^{A}) \end{aligned}$$

(12)

A household’s payoff $V_{h}^{z}$ in z equals its payoff $U_{h}^{z}$ from land and pollution (the former given by $\Pi ^{z}$ and the latter by the final term on the RHS of (3)) minus its payments to firms in the other region. From (10):

$$\begin{aligned} V_{h}^{A}= & {} U_{h}^{A}-yn_{f}(1-s_{f}^{A}),\ \ \ \ \ \ \ \ U_{h}^{A}=\phi -(\theta +1)n_{f}s_{f}^{A}-\theta s_{h}^{A} \end{aligned}$$

(13)

$$\begin{aligned} V_{h}^{B}= & {} U_{h}^{B}-xn_{f}s_{f}^{A},\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ U_{h}^{B}=\phi -(b\theta +1)n_{f}(1-s_{f}^{A})-b\theta (1-s_{h}^{A}) \end{aligned}$$

(14)

We see that the presence of a firm affects a household’s gross payoff linearly and negatively in two ways: The firm takes up space (reducing household payoff by $\theta$ in A and by $b\theta$ in B) and it pollutes (reducing household payoff by 1). Thus we can interpret $\theta$ in (4) as the crowding effect of a marginal increase in the number of firms in A,  relative to its pollution effect which is normalized to 1. We shall assume that $b\theta <1,$ so that the crowding effect is less important than the pollution effect, even in the smaller area B.

When payoff is higher in one location than in the other, agents do not move immediately to the higher-payoff region. Instead there will be a stream of migration over time until payoffs are equalized (or until everyone has moved to the higher-payoff region). Households and firms only evaluate their current location choice occasionally. Households may be attached to their current location because they are familiar with it, and they have friends and family there. Firms may have made location-specific investments such as factories.

We assume that the rate ${\dot{s}}_{k}^{A}\equiv ds_{k}^{A}/dt$ at which population k relocates to A is proportionate to its payoff difference $\Delta V_{k}\equiv V_{k}^{A}-V_{k}^{B}$:

$$\begin{aligned} {\dot{s}}_{k}^{A}=\left\{ \begin{array}{ll} \max \ (0,\rho _{k}\Delta V_{k}) &{} \text {for }s_{k}^{A}=0 \\ \rho _{k}\Delta V_{k} &{} \text {for }0<s_{k}^{A}<1 \\ \min \ (0,\rho _{k}\Delta V_{k}) &{} \text {for }s_{k}^{A}=1 \end{array} \right. \ \ \ \ \ \ \ \ k=f,h;\ \rho _{h}=1;\rho _{f}>0 \end{aligned}$$

(15)

This is the projection dynamic (Friedman 1991;¹⁵ Lakhar and Sandholm 2008; Sandholm et al. 2008).¹⁶ The projection dynamic has been applied to spatial pricing by Nagurney and Zhang (1996), to traffic flows by Nagurney and Zhang (1998), and to urban growth by Fujishima (2013).

The projection dynamic is the only deterministic dynamic described in Sandholm (2010, pp. 150-153) where the boundary of the state space is reached in finite time. This is essential for our application. Suppose all households are in A, paying firms in B, and firms are moving from A to B. With replicator dynamics and many other dynamics, the firms’ relocation speed is given by the product of $s_{f}^{A}$ and other factors. Thus as $s_{f}^{A}$ declines, the firms’ move to B slows down so much that there will always be some firms left in A at any time. At some point, households will realize that although they are paying firms in B, there are hardly any firms moving from A to B anymore. They will then stop trying to move firms to B. The result is an equilibrium that cannot be pinned down precisely, with all households in A and "almost" all firms in B.

Total welfare is:

$$\begin{aligned} W(s_{h}^{A},s_{f}^{A})\equiv & {} s_{h}^{A}w_{h}^{A}+(1-s_{h}^{A})w_{h}^{B} \end{aligned}$$

(16)

$$\begin{aligned}= & {} s_{h}^{A}u(g^{A})+(1-s_{h}^{A})u(g^{B})+n_{f}s_{f}^{A}u(g^{A})+n_{f}(1-s_{f}^{A})u(g^{B}) \nonumber \\&-n_{f}s_{f}^{A}s_{h}^{A}-n_{f}(1-s_{f}^{A})(1-s_{h}^{A}) \end{aligned}$$

(17)

$$\begin{aligned}= & {} \left( 1+n_{f}\right) \phi -\frac{\theta }{2}\left[ s_{h}^{A}+n_{f}s_{f}^{A}\right] ^{2}-\frac{\theta b}{2}\left[ 1-s_{h}^{A}+n_{f}\left( 1-s_{f}^{A}\right) \right] ^{2} \nonumber \\&-n_{f}s_{f}^{A}s_{h}^{A}-n_{f}(1-s_{f}^{A})(1-s_{h}^{A}) \end{aligned}$$

(18)

In (16), we define welfare as the population-weighted average of household utilities $w_{h}^{A}$ and $w_{h}^{B}$ in A and B respectively, defined by (3). From (3), the first two terms on the RHS of (17) are the household utility from land in either location. The next two terms are utility from the consumption good, which from (3) equals total production with $q_{z}^{f}\left( 1,g^{z}\right) =u\left( g^{z}\right) ,z=A,B,$ in (1) and (4). The final two terms are environmental damage from (3). Substituting (4) and (7) into (17) yields (18).

Note that the households own all the land and all the fixed production factors. Moreover, as noted above, household utility (3) is quasilinear in the consumption good and land. Thus households spend any extra revenue from land and fixed factor ownership on the consumption good. This means that the rental payments for land, the firms’ profits which accrue to the fixed factor as well as any payments from households to polluting firms drop out of the welfare equation.

4 Welfare Optima and Laissez Faire Equilibria

In this section we elaborate on Dijkstra and de Vries (2006) to derive and compare the welfare optima and the laissez faire equilibria. The FOCs for an interior welfare optimum are, from (18):

$$\begin{aligned} \frac{\partial W}{\partial s_{h}^{A}}= & {} -\theta \left[ s_{h}^{A}+n_{f}s_{f}^{A}\right] +\theta b\left[ 1-s_{h}^{A}+n_{f}\left( 1-s_{f}^{A}\right) \right] -n_{f}s_{f}^{A}+n_{f}(1-s_{f}^{A})=0\ \ \ \ \ \ \ \ \end{aligned}$$

(19)

$$\begin{aligned} \frac{\partial W}{\partial s_{f}^{A}}= & {} n_{f}\left\{ -\theta \left[ s_{h}^{A}+n_{f}s_{f}^{A}\right] +\theta b\left[ 1-s_{h}^{A}+n_{f}\left( 1-s_{f}^{A}\right) \right] -s_{h}^{A}+(1-s_{h}^{A})\right\} =0 \end{aligned}$$

(20)

However, since damage occurs when firms and households are together, there should be at least one region with only firms or only households. The optimum can then be either of type HF (households in A, firms in B, and a mix of firms and households in at most one region) or FH (defined analogously). The global welfare optimum is HF. Intuitively, since there are more households than firms and A is the largest region, it is better to have households predominantly in A and firms in B.

We denote the different locational patterns under partial separation by notation such as (h, fh),  which means: only households in A, and firms and households in B.

Formally we find:¹⁷

Lemma 1

• 1. There is always an HF welfare optimum. This is also the global optimum. The HF optimum is (h, fh) when:

  $$\begin{aligned} bn_{f}<1-\frac{n_{f}}{\theta } \end{aligned}$$

  (21)

  and (h, f) when (21) does not hold.

• 2. There is a local FH welfare optimum (fh, h) if and only if:

  $$\begin{aligned} (\theta +1)n_{f}<\theta b<\theta (1+bn_{f})+(3\theta +2)n_{f} \end{aligned}$$

  (22)

  If the first condition does not hold, the FH welfare optimum is (f, h). If the second condition does not hold, there is no FH welfare optimum.

• 3. If there is an interior solution to (19) and (20), this is a saddle point.

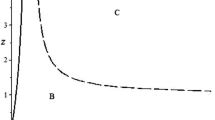
Let us now derive the laissez faire equilibria. Under laissez faire, there is no environmental policy and there are no payments from households to firms, i.e. $x=y=0$ and $V_{k}^{z}=U_{k}^{z}$ in (11) to (14). In the equilibrium, nobody wants to move. Figure 1 illustrates our findings.

On the locus $hh^{\prime }$, illustrated in Figure 1, households are indifferent between A and B. From (13) and (14):

$$\begin{aligned} \Delta U_{h}\equiv U_{h}^{A}-U_{h}^{B}=0:\ \ \ \left( \theta +1\right) n_{f}s_{f}^{A}+\theta s_{h}^{A}=(b\theta +1)n_{f}(1-s_{f}^{A})+b\theta (1-s_{h}^{A}) \end{aligned}$$

(23)

This is the same condition as (19) which maximizes welfare with respect to $s_{h}^{A}.$ Thus households make the location decision that maximizes welfare, because they take the pollution from firms into account.

On the locus $dd^{\prime }$, also illustrated in Figure 1, firms are indifferent between A and B. From (11) and (12):

$$\begin{aligned} \Delta U_{f}\equiv U_{f}^{A}-U_{f}^{B}=0:\ \ \ \ n_{f}s_{f}^{A}+s_{h}^{A}=bn_{f}(1-s_{f}^{A})+b(1-s_{h}^{A}) \end{aligned}$$

(24)

This condition is different from (20) which maximizes welfare with respect to $s_{f}^{A}.$ Firms do not make the location decision that maximizes welfare, because they disregard the environmental damage they cause. However, the laissez faire equilibrium still implements the local welfare optimum (if there is one) as long as it is not on $dd^{\prime }$.

Fig. 1

Laissez faire phase diagrams. Panel a (left): Single equilibrium ($\theta =\frac{1}{5},b=\frac{14}{ 3},n_{f}=\frac{4}{7}$). Panel b (right): Two stable equilibria ($\theta =\frac{5}{9},b=\frac{5}{3},n_{f}=\frac{4}{5},\rho _{f}=\frac{3 }{2}$). A dot represents a stable laissez faire equilibrium, a square represents a local (not global) welfare optimum, and the heart represents the global welfare optimum

The conditions for the different laissez faire equilibria and their optimality are:¹⁸

Lemma 2

• 1. There is always a stable FH laissez faire equilibrium. This equilibrium is the local welfare optimum (f, h) if:

  $$\begin{aligned} n_{f}(\theta +1)>\theta b \end{aligned}$$

  (25)

  and (fh, h) otherwise. The (fh, h) equilibrium is the local welfare optimum if the latter exists, i.e. if the second inequality of (22) holds.

• 2. There is an HF laissez faire equilibrium, which is stable, if

  $$\begin{aligned} bn_{f}<2+n_{f} \end{aligned}$$

  (26)

  The equilibrium is the global welfare optimum (h, fh) if:

  $$\begin{aligned} bn_{f}<1-\frac{n_{f}}{\theta } \end{aligned}$$

  (27)

  The equilibrium is the global welfare optimum $(h,f)\,$if:

  $$\begin{aligned} 1-\frac{n_{f}}{\theta }<bn_{f}<1 \end{aligned}$$

  (28)

  The equilibrium is (fh, f) if:

  $$\begin{aligned} 1<bn_{f}<2+n_{f} \end{aligned}$$

  (29)

  In this case, (h, f) is the global welfare optimum.

• 3. If and only if (26) holds, there is an unstable interior laissez faire equilibrium j with

  $$\begin{aligned} \left( s_{h}^{Aj},s_{f}^{Aj}\right) =\left( \frac{2b-n_{f}+bn_{f}}{2b+2}, \frac{1}{2}\right) \end{aligned}$$

  (30)

We see that four of the five possible stable laissez faire equilibria implement a (local) welfare optimum. Firms do not consider environmental damage in their location choice, but this doesn’t matter in (f, h), (fh, h) , (h, fh) and (h, f), because all firms locate in the area with the lowest land rental prices. In (fh, f) however, firms are spread over both areas in such a way that land rental prices are equalized and firms are indifferent between the two locations, resulting in too many firms in A together with the households. Two conditions must hold for the (fh, f) equilibrium to exist. First, when all households are in A, firms should be located in both areas, rather than in B only. This is achieved by the first inequality $bn_{f}>1$ of (29) which means that when all households are in A and all firms are in B, density and land rental prices are higher in B, giving firms an incentive to move from B to A. The second condition for (fh, f) to exist is that when firms are spread over A and B such that land rental prices are equalized, households prefer A to B. This is achieved by the second inequality of (29).

Figure 1 illustrates the Lemma. We denote the different domains of the graph by notation such as BA, which means that with laissez faire, households move to B and firms move to A. If the $hh^{\prime }$ and $dd^{\prime }$ curves do not cross (as in Figure 1a), there is just one laissez faire equilibrium, of the FH variety. If $hh^{\prime }$ and $dd^{\prime }$ cross (as in Figure 1b), there is also an interior equilibrium and an HF equilibrium.

Figure 1 also illustrates the stability of the equilibria. The FH and HF equilibria are always stable. The interior equilibrium is always unstable. All points to the left (right) of the saddle path SP evolve to the $FH\ (HF)$ equilibrium.¹⁹$^{,}$²⁰

5 Positive-Payment Equilibrium

Suppose households in one location (say A) hit upon the idea of incentivizing firms to move to the other location (B) and to stay there. In this section we shall first consider the form that this payment might take. We will then explore the properties of a payment equilibrium. Finally, we examine whether an economy would move from a laissez faire equilibrium to a payment equilibrium.

Households in A could pay firms in A to move to B. This would be ineffective, because firms would keep returning from B to A in order to be paid to move back again. Alternatively, households in A could pay firms in A to move to B and not to return. There are two problems with this scheme. First, it is very difficult to establish the payment amount. It requires determining a firm’s payoff difference between A and B in every possible future scenario and calculating its net present value. Secondly, the contract needs to be enforced. If a firm wants to return to A, although it has promised not to, can the households stop the firm or force it to reimburse the payment (cf. Fields 2004)?²¹

The payment method we shall analyze in this paper is for households in A to pay firms in B. This continuous stream of payment over time gives firms in A an incentive to move to B and it gives firms in B an incentive to stay there. There is no problem with enforcement, because as soon as a firm moves from B to A, the payment stops.

We shall impose some limits on the payments from households in A to firms in B:²²

Condition 1

The payment y from households in A to firms in B satisfies:

• 1. If $U_{h}^{A}\le U_{h}^{B}$ in (13) and (14), then $y=0.$

• 2. If $U_{h}^{A}>U_{h}^{B},$ then $y\le y^{\max }$ for finite t and $y<y^{\max }$ for $t\rightarrow \infty$ with $y^{\max }>0$ implicitly defined by:

  $$\begin{aligned} U_{h}^{A}-(1-s_{f}^{A})y^{\max }=U_{h}^{B} \end{aligned}$$

It seems reasonable to assume that the maximum payment that households in A will temporarily agree to is the payment that makes them indifferent between the two regions, given the current location of households and firms (Condition 1.2). The purpose of the payment is to make A a better place to live. A payment so large that households actually prefer B to A defeats the purpose. Households are willing to pay the maximum amount temporarily as they see the effect on firms’ location choice, but they will start objecting when firms hardly move anymore, or not at all. Households do not want to be trapped in a situation where they don’t see any difference between their current residence and the other region.

Households in A will then only start paying firms in B if households strongly prefer A to B (Condition 1.1). In this case, households identify with A. They consider it their home and they are interested in making it a better place to live. If households are indifferent between A and B, they don’t identify with the location they live in. They are not interested in paying firms elswhere to make their current home more pleasant. Residents of a region that households are leaving for the higher-payoff region are even less inclined to make payments. They are asking themselves whether it is time for them to move as well, not whether they can make their current home more attractive.

Let us now explore the nature of a positive-payment equilibrium where y and/or x is positive. When households are spread over both locations in equilibrium, they are indifferent between the two locations: $V_{h}^{A}=V_{h}^{B}$ in (13) and (14). There are then two scenarios. First, payoff gross of payments is weakly lower in A ($U_{h}^{A}\le U_{h}^{B}).$ Then by Condition 1.1, $y=0.$ Furthermore, by the analogoue of Condition 1.2, x cannot be so high that $V_{h}^{A}=V_{h}^{B}.$ Secondly, when $U_{h}^{A}>U_{h}^{B},$ then by Condition 1.2, y cannot be so high that $V_{h}^{A}=V_{h}^{B}$.

There can thus only be a positive-payment equilibrium with all households in the same region, strictly preferring that region over the other region. In this equilibrium, households maximize their own utility, given that firms are indifferent between the two regions. Households derive utility from land and the consumption good, and disutility from pollution. Since households are also the recipients of land rents and of the firms’ fixed factor rewards, and they are all in the same region, they take all general equilibrium effects into account. Thus in the positive-payment equilibrium, households effectively maximize total welfare, given their own location: When all households are in $A\ [B],$ they maximize $W(1,s_{f}^{A})\ [W(0,s_{f}^{A})]$ in (16).

We know from Lemma 2.1 that the local FH welfare optimum, if it exists, is always implemented by laissez faire. Thus, since a positive-payment equilibrium implements the (local) welfare optimum, there is no FH positive-payment equilibrium.

Moving on to HF,  only the laissez faire equilibria (h, f) and (h, fh) implement the global HF welfare optimum by Lemma 2.2. Neither of these equilibria exists if:

$$\begin{aligned} bn_{f}>1 \end{aligned}$$

(31)

so that the HF laissez faire equilibrium is either (fh, f) or it does not exist. In either case, there is a positive-payment equilibrium.

Condition (31) says that when all firms are in B and all households are in A, density and land rental prices are higher in B. From Lemma 1.1, this means that the HF welfare optimum is (h, f) rather than (h, fh),  because households should not be in B, where land rental prices and pollution are higher than in A. Condition (31) also implies that in laissez faire, households as well as firms prefer A to B. This is why firms need to be paid to stay in B, and households in A are willing to pay for this.

The payment ${\bar{y}}$ from each household in A to each firm in B makes firms indifferent between A and B. From (11) and (12):

$$\begin{aligned} {\bar{y}}=\theta (bn_{f}-1)>0 \end{aligned}$$

(32)

The inequality follows from (31). Yet the payment is small enough for households to still prefer A to B, since (h, f) is the welfare optimum so that by (13), (14) and (32):

$$\begin{aligned} \theta +n_{f}{\bar{y}}=\theta +n_{f}\theta (bn_{f}-1)<(b\theta +1)n_{f} \end{aligned}$$

The inequality holds because by (31) it can be rewritten as:

$$\begin{aligned} n_{f}\theta (bn_{f}-1)<\theta (bn_{f}-1)+n_{f} \end{aligned}$$

Now let us check whether the positive-payment equilibrium can be reached from a laissez faire equilibrium that is not the global welfare optimum. Payments will not arise from the laissez faire equilibrium (f, h). There is no need for households in B to pay firms in A, because all firms are already there. Payments from households in A to firms in B cannot arise, because there are no households in A.

If the laissez faire equilibrium is (fh, h),  payments will not arise either. Households are spread over both regions and are thus indifferent between them. By Condition 1.1, households will not pay firms in the other region.

Finally, if the laissez faire equilibrium is (fh, f),  payments will arise. Since households prefer A to B, they would like firms to move from A to B. Thus households in A will pay firms in B. The payments will eventually induce all firms to move to B so that the global welfare optimum (h, f) is reached.

Thus we have proved:

Proposition 1

• 1. If and only if (31) holds, there is a positive-payment equilibrium which is unique and implements the global welfare optimum (h, f).

• 2. If (h, f) is a positive-payment equilibrium, households as well as firms would prefer A to B in (h, f) under laissez faire.

• 3. The only laissez faire equilibrium from which the economy can evolve to the positive-payment equilibrium is (fh, f).

We conclude that each HF laissez faire equilibrium is a "good" equilibrium in the sense that it either implements the global welfare optimum or it can evolve to the global welfare optimum with payments from households in A to firms in B. The FH laissez faire equilibria are "bad" in the sense that they do not implement the global welfare optimum, nor can they evolve to it.

6 The Effect of Payments on Location Dynamics

If the economy is on a path to an $FH\ (HF)$ equilibrium, can payments set it on a path to an $HF\ (FH)$ equilibrium instead? We shall examine households and firms moving in opposite directions (the same direction) under laissez faire in Sect. 6.1 (6.2).

6.1 Households and Firms Moving in Opposite Directions

In domain AB, households move towards A and firms to B under laissez faire, as in Figure 1b. Since households prefer A to B, households in A will pay firms in B. This will make firms move to B even faster. The households’ move to A will slow down, possibly to zero. Eventually, the economy will exit AB or move to its edge. With regard to the former option, the economy cannot cross the $hh^{\prime }$ curve from AB into BB,  because household payment to firms would drop toward zero on approach of the $hh^{\prime }$ curve. However, it might cross the $dd^{\prime }$ curve from AB into AA. We shall see in subsection that the economy will then move back from AA into AB.

Now let us examine the edges of AB. First, when all households are in A, they will keep paying firms in B. If (h, f) is the laissez faire equilibrium, all firms will move to B, at which point households reduce their payment to zero, because firms will remain in B even without payment. If (h, fh) is the laissez faire equilibrium, then as we have seen in Sect. 5, households in A will keep paying firms in B beyond this point, crossing the $dd^{\prime }$ curve into AA and reaching the positive-payment equilibrium (h, f).

Secondly, when all firms are in B, households in A will reduce the payment to firms in B to zero, because the firms will stay in B even without any payment. Households will move from B to A until they are indifferent between A and B [laissez faire equilibrium (fh, h)] or until they are all in A [laissez faire equilibrium (h, f)].

By the same token, when households move towards B and firms to A under laissez faire (domain BA in Figures 1a and b), households in B will pay firms in A. If the economy remains within BA,  it will evolve toward the FH laissez faire equilibrium. The economy may cross $dd^{\prime }$ into BB,  but applying the analysis that we use below for AA,  we can show that the economy will still end up in the FH laissez faire equilibrium.

6.2 Households and Firms Move in the Same Direction

In this subsection we examine how payments affect the location dynamics at a certain point when households and firms move in the same direction under laissez faire. In Sect. 7 we analyze the whole path to equilibrium from any starting point. We will only examine the domain AA where both populations initially prefer A to B. The same principles apply to domain BB where both prefer B to A. However, BB is usually smaller and it is more difficult to reach the HF equilibrium from BB than from AA.²³

In AA,  firm and household payoffs are given by (11) to (14) with $x=0.$ Payments from households in A to firms in B increase firm payoff in B and decrease household payoff in A. Thus A becomes less attractive to both firms and households, slowing down the movement by both groups to A. In order to determine the direction in which the economy will move, we need to examine one population’s relocation speed relative to the other. From (11) to (15):

$$\begin{aligned} \frac{d}{dy}\left( \frac{{\dot{s}}_{f}^{A}}{{\dot{s}}_{h}^{A}}\right) =\rho _{f} \frac{d}{dy}\left( \frac{\Delta V_{f}}{\Delta V_{h}}\right) =\frac{\rho _{f}\Psi ^{A}}{\left( \Delta V_{h}\right) ^{2}} \end{aligned}$$

with:

$$\begin{aligned} \Psi ^{A}\equiv & {} -s_{h}^{A}\Delta V_{h}+n_{f}(1-s_{f}^{A})\Delta V_{f} \nonumber \\= & {} -s_{h}^{A}\left[ b(\theta +1)n_{f}(1-s_{f}^{A})+b\theta (1-s_{h}^{A})-(\theta +1)n_{f}s_{f}^{A}-\theta s_{h}^{A}\right] \nonumber \\&+n_{f}(1-s_{f}^{A})\theta \left[ bn_{f}(1-s_{f}^{A})+b(1-s_{h}^{A})-(n_{f}s_{f}^{A}+s_{h}^{A})\right] \nonumber \\= & {} -s_{h}^{A}\Delta U_{h}+n_{f}(1-s_{f}^{A})\Delta U_{f} \end{aligned}$$

(33)

Note that $\Psi ^{A}$ does not depend on y. Thus the relative relocation rate is monotonic in y. This allows us to rewrite $\Psi ^{A}$ in (33) as a function of the gross payoff differences $\Delta U_{k}\equiv U_{k}^{A}-U_{k}^{B},\ k=f,h$. If $\Psi ^{A}>(<)0,$ payments increase (decrease) firms’ relocation speed to A relative to households’ speed.

Setting $\Psi ^{A}=0$ in (33) gives the locus of points where payments do not affect the relative relocation rates. Solving for $s_{f}^{A}$ yields:

$$\begin{aligned} s_{f}^{A}=\frac{\theta \left( b+n_{f}+2bn_{f}\right) -s_{h}^{A}(1+b)(1+2\theta )\pm \sqrt{D^{A}}}{2n_{f}\theta (1+b)} \end{aligned}$$

(34)

where the discriminant $D^{A}$ is:

$$\begin{aligned} D^{A}\equiv (b+1)^{2}\left( 4\theta +1\right) \left( s_{h}^{A}\right) ^{2}-2\theta (b+1)\left( b+n_{f}\right) s_{h}^{A}+\theta ^{2}\left( b-n_{f}\right) ^{2} \end{aligned}$$

(35)

We find that $D^{A}$ has its global minimum at:

$$\begin{aligned} s_{h}^{A}=s_{h}^{AD}\equiv \frac{\theta (b+n_{f})}{(b+1)(4\theta +1)} \end{aligned}$$

(36)

Substituting this into (35) yields:

$$\begin{aligned} D^{A}\ge {\underline{D}}^{A}\equiv \frac{4\theta ^{2}}{4\theta +1}\left[ \theta (b-n_{f})^{2}-bn_{f}\right] \end{aligned}$$

(37)

If ${\underline{D}}^{A}>0,$ there are always two solutions to $\Psi ^{A}=0$ in (33). Then the locus consists of an upper and a lower y curve. In between the curves $\Psi ^{A}<0$, while $\Psi ^{A}>0$ above the upper and below the lower curve. If $\ {\underline{D}}^{A}<0,$ there is a range of $s_{h}^{A}$ for which there is no solution to $\Psi ^{A}=0$. The locus then consists of a left-hand and a right-hand y curve outside of this range. Inside (outside) these curves, $\Psi ^{A}<(>)\ 0$.

Lemma B1 and Proposition B1 in Appendix B give more detail on the shape of the y locus under different conditions. Since there are many possible scenarios, we will focus on two representative and distinct cases with a positive-payment equilibrium in the next section. Appendix B describes the two cases in detail. Figures 2 and 3 illustrate Cases 1 and 2, respectively.²⁴ In the light (dark) shaded areas, payments make households (firms) move relatively faster from B to A. Note from (33) that $\Psi ^{A}>0$ and payments make firms move relatively faster if $s_{h}^{A}$ and $\Delta U_{h}$ are low, and if $(1-s_{f}^{A})$ and $\Delta U_{f}$ are high.

Case 1 features a single laissez faire equilibrium which is (fh, h). The positive-payment equilibrium (h, f) is in AA. The y locus in AA consists of an upper curve $T^{y}(s_{h}^{A})$ connecting (h, 1) with $(1,y^{1})$ and a lower curve $I^{y}(s_{h}^{A})$ connecting (0, 1) with $(y^{A},0).$ In Figure 2 illustrating Case 1, payments leave the relative speeds unchanged on the $T^{y}$ and $I^{y}$ curves.²⁵ In the area $hh^{\prime }y^{1},$ payments make firms move faster than households. Intuitively, since households only have slightly higher payoff in A than in B ($\Delta U_{h}$ is small) close to the $hh^{\prime }$ curve, the payoff advantage of A will be affected relatively strongly when households in A start paying firms in B. Thus the payment will slow down households more than firms. In the area $y^{0}hh^{\prime }Cy^{A},$ payments make households move faster than firms. Intuitively, this area contains points close to C with many households in A and few firms in B. A high $s_{h}^{A}$ means that each firm in B will collect a large payment, making it relatively attractive for firms to stay in B. Moreover, a low $(1-s_{f}^{A})$ means that each household in A only has to make a small payment, making it relatively attractive for households to move to A. Conversely, in the area $Oy^{0}y^{A}$ there are only few households in A who can pay firms in B. The payment that each firm receives is rather small, which reduces firms’ speed less than it does households’ speed.

Case 2 features the two stable laissez faire equilibria (f, h) and (fh, f) and the positive-payment equilibrium (h, f). The y locus in AA consists of the right-hand curve only, with upper branch $TR^{y}(s_{h}^{A})$ and lower branch $IR^{y}(s_{h}^{A}),$ connecting the point $(y^{A},0)$ with the interior unstable laissez faire equilibrium j. In Figure 3a illustrating Case 2, payments leave the relative speeds unchanged on the $y^{A}j$ curve. In the area $Ohjy^{L}y^{A},$ payments make firms move faster than households. This is because, as in Figure 2, this area contains points close to the $hh^{\prime }$ curve where households only have a weak preference for A to begin with, and points where $s_{h}^{A}$ is low, so that each firm in B only receives a small payment. In the area $y^{A}y^{L}jd^{\prime }C,$ payments make households move faster than firms. This is because this area contains points close to $dd^{\prime }$ where firms only have a weak preference for A. In addition, as in Figure 2, the area contains points with high $s_{h}^{A},$ so that each firm in B receives a large payment.

7 The Path to Equilibrium

In this section we will look at the path of the economy from any starting point in AA to the equilibrium, given that payments from households in A to firms in B are possible. In Sect. 6.1 we have already analyzed this path from starting points where firms and households move in opposite directions. Thus this section focuses on starting points where firms and households move in the same direction (only to A, in order to avoid too much repetition) to the equilibrium or to points where they move in opposite directions.

We analyze Case 1 with a unique laissez faire equilibrium in Sect. 7.1 and Case 2 with multiple equilibria in Sect. 7.2. In both subsections we look at the scenario with maximum payments and the best case scenario. By Condition 1.2, the maximum payment that households will agree to pay temporarily is the one that makes them indifferent between A and B. The best case scenario is defined as maximizing the range of starting points that leads to the "good" equilibrium, implementing the global HF welfare optimum.

In Sect. 7.2 we shall also look at the effect of a small payment from households to firms. When there are multiple laissez faire equilibria, even a small payment might change the path of an economy from moving toward an FH equilibrium to moving toward an HF equilibrium (or vice versa).

7.1 Case 1: Unique Laissez Faire Equilibrium

In Figure 2, recall that payments make households (firms) move relatively faster in the light (dark) shaded areas. Let us start with the maximum payment from households in A to firms in B. This payment leaves households indifferent between A and B, so that they stop moving.

Above the $T^{y}$ curve and below the $I^{y}$ curve in Figure 2, payments slow down households more than firms. When the payment is so large that households stop moving from B to A, firms are thus still moving to A. This is indicated by an upward arrow in Figure 2. Households in A might be disappointed that firms are still moving to A and perhaps even worried that the economy is moving toward the "bad" FH equilibrium. On the other hand, at least their payoff is increasing, because it is the same as household payoff in B, which is increasing because firms are leaving B.

In between the $T^{y}$ and $I^{y}$ curves, payments slow down firms more than households. Here, the payment that stops households moving from B to A is so large that firms start moving from A to B, a reversal of the original direction. Thus is indicated by a downward arrow in Figure 2. Households in A might be concerned that their payoff is decreasing, since it equals household payoff in B, which is decreasing because firms are moving to B. On the other hand, at least the movement of firms is reversed toward B and the economy appears to be moving toward the "good" HF equilibrium.

Fig. 2

Case 1: Single laissez faire equilibrium ($\theta =\frac{1}{5},b=\frac{14}{3},n_{f}=\frac{4}{7}$ ), maximum payments from households to firms

We distinguish three areas in AA. In the area $hh^{\prime }y^{1}$ in Figure 2,  firms move to A until the economy arrives at the $hh^{\prime }$ locus, where households are indifferent between A and B without payments. After this, firms still move to A, but households start moving to B. As analyzed in Sect. 6.1, the economy will end up in the laissez faire FH equilibrium (point h in Figure 2).

In the area $y^{A}{\bar{y}}^{A}y^{1}C$ in Figure 2, firms move to B until they are all there. Households in A will then reduce their payments to the level that is just enough to keep firms indifferent between A and B. This reduction in payment will induce households in B to move to A, as indicated by the rightward arrow in Figure 2. This continues until all households are in A (point C). We know from Proposition 1.1 that point C is also the positive-payment equilibrium, where households maximize their welfare. Thus the economy will eventually end up in point C,  perhaps after some further experimentation with payments.

Finally, in the area $Oy^{0}h{\bar{y}}^{A}y^{A}$ in Figure 2, firms move to A if $s_{f}^{A}$ is low (below $I^{y})$ and to B if $s_{f}^{A}$ is high (above $I^{y}).$ Since by (15) the firms move with a speed proportional to their payoff difference, they will slow down by so much on the approach to $I^{y}$ that the economy never actually reaches $I^{y}.$ By Condition 1.2, the households in A will not agree to pay the maximum amount forever. There will come a moment when they reduce the payment. This will cause households as well as firms to move to A, a movement upward and to the right in Figure 2. If the economy started below $I^{y},$ this causes it to cross $I^{y}.$ After a while, households in A will resume the maximum payment, causing the economy to move straight down to $I^{y}$ again, but approaching it further to the right than before, as there are now more households in A. This process continues until the economy ends up to the right of $y^{A}.$ Eventually, as described above for the area $y^{A}{\bar{y}}^{A}y^{1}C,$ this will lead to the positive-payment equilibrium C.

The whole area $Oy^{0}hy^{1}C$ thus evolves to C under maximum payments. This is also the maximum area that can evolve to C in the best case scenario.²⁶ This is because area $hh^{\prime }y^{1}$ cannot be made to evolve to C. In this area, payments would only make firms move relatively faster to A. It is inevitable that the economy hits the $hh^{\prime }$ locus, after which it evolves to the FH laissez faire equilibrium.

We see that with payments, most of the area AA evolves to the "good" equilibrium C. This equilibrium did not even exist under laissez faire, let alone that the economy could evolve to it. We have also seen in Proposition 1.3 that point C cannot be reached if payments start from the laissez faire equilibrium FH. However, we have now seen that it can be reached from many points in AA.

Summarizing our results, we have:

Proposition 2

Starting in AA in Case 1, with maximum payments as well as in the best case scenario, all points and only the points with $s_{f}^{A}<\min \left[ 1,T^{y}(s_{h}^{A})\right]$ evolve to the positive-payment equilibrium (h, f).

7.2 Case 2: Multiple Laissez Faire Equilibria

In Figure 3a, recall that payments make households (firms) move relatively faster in the light (dark) shaded areas. Let us first examine the effects of a small payment in AA. The saddle path $SP(s_{h}^{A})$ to the interior unstable equilibrium splits the area into points that evolve to HF and FH. This path is increasing in $s_{h}^{A}$. By Proposition B1.1, the $y^{A}j$ curve is to the left of and decreasing toward the interior equilibrium j. Thus there is always an area close to j where the saddle path is to the right of the $y^{A}j$ curve. To the right of the $y^{A}j$ curve, payments make households move relatively faster to A. We conclude that points close to j immediately to the left of the saddle path, which would evolve to FH without payments, will evolve to HF with a small payment.

Let $j^{A}$ be the point where the saddle path intersects the $s_{h}^{A}$ axis:

$$\begin{aligned} SP(j^{A})=0 \end{aligned}$$

(38)

If the point $y^{A}$ where the y curve intersects the $s_{h}^{A}$ axis is to the right of $j^{A}$, as it is in Figure 3a, there might also be points with low $s_{h}^{A}$ that evolve to HF without payments, but to FH with a small payment.

Summarizing the effect of small payments, we have:

Proposition 3

Starting in AA in Case 2, with a small payment from households in A to firms in B:

• 1. if $y^{A}\le j^{A}$ in (38), there are starting points that switch from evolving to FH to evolving to HF. There are no starting points for which the opposite happens.

• 2. if $y^{A}>j^{A},$ there exists an $s_{h}^{A*}\in \left[ \max \left( 0,j^{A}\right) ,s_{h}^{Aj}\right)$ with $s_{h}^{Aj}$ given by (30), such that for $s_{h}^{A}\ge s_{h}^{A*},$ there are starting points that switch from evolving to FH to evolving to HF and there are no starting points for which the opposite happens.

Let us now consider the other extreme of the maximum payment from households in A to firms in B. This payment leaves households indifferent between A and B. To the left and above the $y^{A}j$ curve, firms still move from B to A, denoted by an upward arrow in Figure 3a. To the right and below the $y^{A}j$ curve, the payment causes firms to move from A to B, denoted by a downward arrow. Since the analysis is very similar to Sect. 7.1, we shall be brief and concentrate on the comparison with laissez faire.

Fig. 3

Case 2: Two stable laissez faire equilibria ($\theta =\frac{5}{9},b=\frac{5}{3},n_{f}= \frac{4}{5},\rho _{f}=\frac{3}{2}$). Panel a (left): Small payment and maximum payment from households to firms. Panel b (right): Best case scenario

Starting points in the area ${\bar{y}}^{L}y^{L}{\bar{y}}^{A}jd^{\prime }C$ in Figure 3a evolve to the payment equilibrium C.²⁷ For the area ${\bar{y}}^{L}y^{L}{\bar{y}}^{A}y^{A},$ the economy first moves toward $IR^{y},$ then slides down $IR^{y}$ before moving to C. The saddle path $\ SP(s_{h}^{A})$ toward the interior laissez faire equilibrium j intersects the area ${\bar{y}}^{L}y^{L}{\bar{y}}^{A}jd^{\prime }C$, because by Proposition B1.1, the $y^{A}j$ curve is to the left of and decreasing toward j. Thus the area contains points that would evolve to the HF equilibrium under laissez faire, but also points that would evolve to (f, h).

Starting points in the area $Ohj{\bar{y}}^{A}y^{L}{\bar{y}}^{L}$ in Figure 3a will evolve to (f, h). Without payments, much of this area would also evolve to (f, h), because it is to the left of the saddle path. However, there could be a small triangle to the left of ${\bar{y}}^{L}$ (as there is in Figure 3a) where payments would cause the economy to move to the FH equilibrium rather than the HF equilibrium.

The effect of maximum payments is then:

Proposition 4

In Case 2, starting from AA with maximum payments from households in A to firms in B:

• 1. All points with $s_{h}^{A}<{\bar{y}}^{L}\cup s_{f}^{A}>TR^{y}(s_{h}^{A})$ evolve to the stable laissez faire equilibrium (f, h). If $j^{A}>{\bar{y}} ^{L},$ all these points would evolve to (f, h) under laissez faire. If $j^{A}>{\bar{y}}^{L},$ points with $s_{f}^{A}>(<)\ SP(s_{h}^{A})$ would evolve to the $FH\ (HF)$ equilibrium under laissez faire.

• 2. All points with $s_{h}^{A}>{\bar{y}}^{L}\cap s_{f}^{A}<TR^{y}(s_{h}^{A})$ evolve to the positive-payment equilibrium (h, f). Points with $s_{f}^{A}>(<)\ SP(s_{h}^{A})$ would evolve to the $FH\ (HF)$ equilibrium under laissez faire.

Finally, we consider the best case scenario where as many starting points as possible evolve to the "good" equilibrium C with payments. Unlike in Sect. 7.1, there are points that can be made to evolve to C with a suitable strategy, although they evolve to the "bad" equilibrium (f, h) with maximum payments. This is illustrated in Figure 3b. We start by noting that the area to the right of the $y^{A}j$ curve can evolve to C by a suitable choice of payments. To the left of the saddle path, the payments should keep households moving to A and reverse the firms’ flow so that they move to B, steering the economy away from the y curve. To the right of the saddle path, the level of payments is irrelevant because the economy will move to C regardless.

The area to the left of the y curve is divided in two by the laissez faire path $P^{t}(s_{h}^{A})$ that is tangent to the y curve. This is the highest path that still has a point in common with the y curve. Formally, this path is defined by:

$$\begin{aligned} P^{t}({\bar{t}}^{y})=TR^{y}({\bar{t}}^{y}),\ \ \ \ \ \ \ \frac{dP^{t}(s_{h}^{A}) }{ds_{h}^{A}}|_{s_{h}^{A}={\bar{t}}^{y}}\equiv \frac{{\dot{s}}_{f}^{A}|_{y=0}}{ {\dot{s}}_{h}^{A}|_{y=0}}=\frac{dTR^{y}\left( s_{h}^{A}\right) }{ds_{h}^{A}} |_{s_{h}^{A}={\bar{t}}^{y}} \end{aligned}$$

(39)

with $TR^{y}$ defined by (34) with the "+" sign and ${\dot{s}} _{k}^{A}|_{y=0},k=f,h,$ by (15) with $y=x=0.$

If households in A do not pay firms in B, economies on all points below $P^{t}$ will hit the y curve between $t^{y}$ and $y^{A}$, from where they can be ushered to C. Somewhat counterintuitively, we find that in this area it is best for households not to pay firms. If they did, firms would move relatively faster than households, which means the economy might not hit the y curve anymore. In order to maximize the area that evolves to C,  households should not pay firms in the area below the $P^{t}$ path.

The area above $t^{y\prime }t^{y}j$ cannot be made to evolve towards C,  because households cannot slow down the firms’ movement toward A enough, let alone reverse it, to cross the y curve. The maximum possible area that can evolve from AA to C is thus the shaded area $Ot^{y\prime }t^{y}jd^{\prime }C$ in Figure 3b. This includes the area $Ot^{y\prime }t^{y}y^{L}{\bar{y}}^{L}$ that would evolve to (f, h) under maximum payments²⁸ as well as the sizable area $Ot^{y\prime }t^{y}jj^{A}$ to the left of the saddle path that would have evolved to (f, h) without payments.

Summarizing the best case scenario, we have:

Proposition 5

For Case 2, starting in AA in the best case scenario, all points and only the points with $\left\{ s_{h}^{A}<{\bar{t}}^{y}\cap s_{f}^{A}<P^{t}(s_{h}^{A})\right\} \cup \left\{ s_{h}^{A}>{\bar{t}}^{y}\cap s_{f}^{A}<TR^{y}(s_{h}^{A})\right\}$ evolve to the positive-payment equilibrium (h, f), with $P^{t}(s_{h}^{A})$ defined by (39) .

8 Model Extensions

In this section, we discuss several extensions to the basic model: endogenous total emissions (Sect. 8.1), transaction costs (Sect. 8.2), a more general household utility function (Sect. 8.3), nonlinear environmental damage (Sect. 8.4 ) and heterogeneous household vulnerability to pollution, combined with incomplete information (Sect. 8.5).

8.1 Endogenous Total Emissions

In our model, total emissions are fixed because the number of firms (the extensive margin) and emissions per firm (the intensive margin) are both fixed. The only way to reduce environmental damage is to separate firms from households. The model can be generalized by letting households protect themselves against pollution or pay firms to reduce their pollution. There are several problems with the latter option. If the firms have to invest in abatement equipment, it may be difficult to incentivize them in a dynamic context. A firm’s abatement actions and associated costs are more difficult to monitor than its location. Households may have to negotiate with firms about payments for abatement. Finally, payments for abatement as well as payments to distant firms may lead to excessive entry of new firms.²⁹ If pollution is the only problem, firms make the socially optimal entry and exit decisions when they have to pay for the environmental damage they cause (Spulber 1985). However, since in our model pollution gives rise to the additional problem of nonconvexities and multiple equilibria, it may be that payments to firms help the economy reach the good equilibrium. This would be analogous to compensation being better than taxation in Dijkstra and de Vries (2006).

8.2 Transaction Costs

We have assumed that there are no transaction costs of organizing the payments from households to firms. Now, let us first assume that these transaction costs are a fixed amount. We have seen in Sect. 5 that in the unique positive-payment equilibrium (h, f) where households in A pay firms in B, firms are indifferent between B and A, and households still prefer A to B. There are two cases to consider. First, if transaction costs are so large that households would prefer B to A, the positive-payment equilibrium no longer exists. In the second case, transaction costs are small enough for the positive-payment equilibrium to still exist. Then it may not be possible to reach this equilibrium from all starting points where this would be possible without transaction costs, because in many of these starting points (including laissez faire equilibrium (fh, f)) households have a weaker preference for A over B than in (h, f).

Instead of being fixed, transaction costs may be increasing in the number of households involved. This is especially detrimental to the existence of the positive-payment equilibrium, because it involves all households. Transaction costs may also be increasing in the rate at which households and firm relocate, because this requires adjustments to who is getting paid, who is paying and how much they are willing to pay. This would not affect the positive-payment equilibrium, but it would make it more difficult to reach this equilibrium from starting points with high relocation rates. Finally, the transaction costs of setting up the payment system may be especially high. The organizers need to inform and persuade the households and devise a system of collecting the sums from households and paying them to firms. These initial costs may be so high that the payment system never happens, although it would increase welfare in the long run.

8.3 Household Utility Function

We have assumed that the household’s utility function (3) is quasilinear and its subutility function of land (4) is the same as the firm’s profit function from land (1) with the fixed factor $F_{f}=1$. These assumptions greatly simplify the analysis. They mean that one firm has the same land rental demand function as one household and thus in each location, one household occupies the same amount of land as one firm. Furthermore, we can compare the number of firms to the number of households, allowing for the assumption that there are less firms than households (i.e. $n_{f}<1).$

As a result of the quasilinear utility function, a household’s rental demand for land only depends on its price. Let us now examine the impact of income effects where land is a normal good. Since households own all the land and the firms, income effects are irrelevant if all households are in the same location, making payments to firms in the other location, as in the (h, f) positive-payment equilibrium. Things are different in AA,  for instance, where the payments from households in A to firms in B ultimately end up equally distributed over all households across A and B. The net effect is a transfer from households in A to households in B. Rental land being a normal good, this leads to less (more) household demand for land in A (B). Land rental prices decrease in A and increase in B, making A relatively more attractive to firms. The income effect thus undermines the purpose of payments to keep firms in B.

8.4 Nonlinear Environmental Damage

We have specified total environmental damage in area z as $n_{f}s_{f}^{z}s_{h}^{z}$ which is linear in $s_{f}^{z}$ and $s_{h}^{z}.$ This simplifies the analysis in two ways. First, it means that payoff differences are linear in $s_{f}^{z}$ and $s_{h}^{z}.$ Secondly, $s_{f}^{z}$ and $s_{h}^{z}$ enter the total damage function symmetrically. However, environmental damage might well be non-linear in the number of firms. The standard textbook treatment assumes strictly convex damage (leading to increasing marginal damage) and concave benefits (leading to decreasing marginal benefits) of emissions, guaranteeing a unique welfare optimum (e.g. Hanley et al. 2007, pp. 49-50, 65-67). On the other hand, damage in the form of visual, light and noise pollution may well be concave: the first wind turbine does a lot of damage, but subsequent wind turbines do not add much.³⁰

In general, total environmental damage in area z can be written as $s_{h}^{z}\Omega (n_{f}s_{f}^{z}),$ with $\Omega ^{\prime }\ge 0.$ Then $\Omega ^{\prime \prime }=0$ signifies linear damage, as before, while $\Omega ^{\prime \prime }<(>)0$ means strictly concave (strictly convex) damage. Note that with nonlinear damage, payoff differences $\Delta V_{k}$ will generally not be linear in $s_{f}^{z}$ anymore, and $s_{f}^{z}$ and $s_{h}^{z}$ do not enter the total damage function symmetrically anymore.

With concave (convex) damage, it becomes more (less) attractive to locate all firms in the same area. However, the main issue we are interested in is the effect of nonlinear damage on the existence of a positive-payment equilibrium. We have seen in Sect. 5 that there is only a positive-payment equilibrium with at least partial separation if the HF laissez faire equilibrium is (fh, f) or non-existent. The condition that separates the laissez faire equilibria (fh, f) and (h, f) is whether or not firms prefer to be in B when all households are in A.³¹ Since in laissez faire firms base their decision only on land rent prices, this condition has nothing to do with enviromental damage and therefore is not affected by the properties of the damage function. Thus, nonlinear damage does not affect the existence of the HF positive-payment equilibrium.

When the damage function is very convex, there can be an interior welfare optimum with firms and households in both locations, with the FOCs (19) and (20) as well as the SOCs for a welfare optimum satisfied for $0<s_{f}^{A},s_{h}^{A}<1$. One area will have low land rent prices and high environmental damage, while the other will have high land rent prices and low environmental damage. Firms will be fairly evenly spread over the two locations, with total and marginal damage relatively low because of the very convex damage function. Payments from the households in the low-density, high-damage area to the firms in the high-density, low-damage area would be needed to implement this welfare optimum. However, since households are indifferent between the two locations in laissez faire,³² the households will not make these payments. Thus, the interior welfare optimum cannot be implemented with payments.

8.5 Heterogeneous Vulnerability and Incomplete Information

We have assumed that all households are identical. Here we will explore household heterogeneity in their vulnerability to pollution. In population games such as the game studied in this paper, agents in a given population are assumed to be identical (Sandholm 2010, p. 3). We can then model household heterogeneity by having multiple household populations, for instance one with high vulnerability and one with low vulnerability to pollution. Welfare optima and laissez faire equilibria where households (namely the less vulnerable households) mix with firms would then become more prevalent. Payments from households to distant polluting firms would still be able to implement a welfare optimum, if there was complete information about the vulnerability of each household. Without this information, each household would claim to have low vulnerability, in order to reduce its payment.³³ It may be easier for a government to identify a household’s vulnerability than it is for other households. However, households may try to avoid behaviour (such as locating in the less polluting area) that could mark them out as being more vulnerable, thereby introducing further distortions.

9 Conclusion

We have presented a model where households and polluting firms can choose from two locations: A and B. Households incur environmental damage from firms in the same location. The welfare optimum features at least partial separation. The global welfare optimum is HF: households in A, firms in B, and at most one location with both groups. This is better than FH (which could be a local optimum) because the largest group should be in the largest area. Laissez faire implements the (local) welfare optimum if it features firms in one area only.

Households in one location (say A) might hit upon the idea of paying firms in the other location (B). The payments will prompt firms to move from A to B and to stay there, thus reducing damage to households in A. We have found that there is a unique positive-payment equilibrium which implements the HF global welfare optimum when the HF laissez faire equilibrium does not. However, this equilibrium cannot be reached from an FH laissez faire equilibrium.

It is by no means certain that payments from households in A to firms in B will set the economy on a path to the "good" equilibrium HF rather than the "bad" equilibrium FH. The crucial point to bear in mind is that these payments make A less attractive not just to firms, but also to households. Thus when both groups are moving from B to A, we need to consider carefully whether payments slow down households or firms more.

There are starting points from which the economy will evolve to the bad equilibrium, regardless of payments. Other starting points will only evolve to the good equilibrium with a specific programme of payments. The government could nudge the economy towards the good equilibrium by making these payments. It should be noted that the required payment pattern can be quite counterintuitive. It could be that at the outset it is best not to have any payments, so as to let the economy drift toward a point from where payments can take it to the good equilibrium. In this situation, payments initially only risk setting the economy on a path to the bad equilibrium.

We can consider our findings in light of the Coase theorem, although the setting is different from the usual Coasean scenario. Victims of pollution pay firms that don’t harm them, but this is not as a result of a contract. There is no process of bargaining between households and firms, but payments evolve over time as households and firms relocate and households learn about the effect of payments. Nevertheless, the payments create paths to the global welfare optimum. However, this optimum cannot be reached from all starting points.

When households start making payments, or are considering to do so, they may not have the information needed to know which path the economy will take. It would be useful to develop some early warning signs that the economy is on a wrong path, and what (if anything) the households can change to move toward the good equilibrium.

We have assumed that all households are identical and they can completely overcome the free rider problem when paying firms. If there is heterogeneity in this ability across communities, polluting firms may end up mainly in communities that are less able to organize themselves. If residents in these areas also tend to be poor and from ethnic minorities, this will fuel concerns of environmental justice (Grainger and Ruangmas 2018; Banzhaf et al. 2019). A tendency for richer households to move to cleaner areas would further exacerbate these concerns (Chen et al. 2012; Binner and Day 2018).

We have considered three relatively straightforward scenarios for payments starting from a dynamic situation: a small payment, the maximum payment that leaves households indifferent between A and B, and the best case scenario. In future work, we could consider more sophisticated scenarios, based on the households’ learning and decision making behaviour. Furthermore, we have only evaluated the paths in terms of their ultimate equilibrium. Future work could consider the whole path toward the equilibrium. Since payments tend to slow down households and firms, it may take longer to reach the equilibrium with payments. This is a disadvantage of payments that we have not considered in the present paper.

We have only analyzed payments starting from laissez faire, comparing them with laissez faire itself. Future work could analyze payments starting from other regulatory regimes such as taxation, compensation (both analyzed by Dijkstra and de Vries 2006), community-based tradable permits (Yang and Kaffine 2016) and zoning (Shertzer et al. 2018), and compare payments to different regulatory regimes.

When firms are required to compensate households for environmental damage, they might want to pay households to stay away. The results would be similar to the present paper, but not an exact mirror image, because there are more households than firms in the model. However, while local government might help households overcome free riding (as with the Santa Maria feedlot), it is less clear how firms would address this problem.

Appendices

Appendix A Proof of Lemmas 1 and 2

Lemma 1

Part 1. The HF welfare optimum cannot be (fh, f). This would be the optimum if (20) could hold with $s_{f}^{A}>0$ and $s_{h}^{A}=1.$ However, the condition for this is $b\theta n_{f}>1+\theta ,$ which is incompatible with $b\theta <1.$

The HF welfare optimum is (h, fh) when (19) holds and $\partial W/\partial s_{f}^{A}<0$ in (20) with $s_{h}^{A}<1$ and $s_{f}^{A}=0$ . It is easily seen that $\partial W/\partial s_{f}^{A}<0$ holds in (h, fh), because density and land rental prices must be higher in A for (19) to hold. This requires that there are more households in A than in B. Thus if firms move from B to A, they move to a more crowded area where they cause more damage. This would reduce welfare. We then find that the HF welfare optimum is (h, fh) when (21) holds and (h, f) when it doesn’t. HF being the global welfare maximum is Dijkstra and de Vries’ (2006) Proposition 6. We can invoke Dijkstra and de Vries’ (2006) proofs for laissez faire and welfare maximization, because our model is a specific version of theirs in these respects.

Part 2. The FH welfare optimum cannot be (f, fh),  because when all households are in B, there should be no firms in B where it is crowded and they cause environmental damage. The FH welfare optimum is (fh, h) when (19) holds and $\partial W/\partial s_{f}^{A}>0$ in (20) with $s_{h}^{A}>0$ and $s_{f}^{A}=1$. In (fh, h),  condition (19) becomes:

$$\begin{aligned} n_{f}(\theta +1)+\theta s_{h}^{A}=\theta b(1-s_{h}^{A}) \end{aligned}$$

Solving for $s_{h}^{A}$:

$$\begin{aligned} s_{h}^{A}=\frac{b\theta -n_{f}\left( \theta +1\right) }{\theta (1+b)} \end{aligned}$$

(40)

We see that $s_{h}^{A}>0$ if and only if the first condition of (22) holds. If not, households should not be in A and the FH welfare optimum is (f, h).

Substituting $s_{f}^{A}=1$ and (40) into (20), the condition for $\partial W/\partial s_{f}^{A}>0$ becomes the second condition of (22). If this condition does not hold, moving firms to B increases welfare and there is no FH welfare optimum.

Part 3. This is Dijkstra and de Vries' (2006) Proposition 5.3.

Lemma 2

Since condition (23) is the same as (19), but (24) is different from (20), laissez faire equilibria $(f,h),\ (fh,h),\ (h,f)$ and (h, fh) implement a (local) welfare optimum, if it exists. This is because they all feature all firms in one location. For stability, see Dijkstra and de Vries’ (2006) Proposition 4. The remainder of the proof is as follows.

Part 1. The FH equilibrium cannot be (f, fh), because with all households in B, firms would prefer A to B.

In the (fh, h) equilibrium, $s_{h}^{A}>0$ if (25) does not hold. For households to be indifferent between A and B, density and land rental prices must be lower in A. Thus, firms always prefer A.

Part 2. The first inequality of (28) for HF equilibrium (h, f) ensures that households prefer to be in A by (13) and (14) and the second ensures that firms prefer B by (11) and (12).

In the HF equilibrium (fh, f), firms are indifferent between A and B. From (11) and (12) this implies:

$$\begin{aligned} n_{f}s_{f}^{A}+1=bn_{f}(1-s_{f}^{A}) \end{aligned}$$

so that:

$$\begin{aligned} s_{f}^{A}=\frac{bn_{f}-1}{n_{f}(1+b)} \end{aligned}$$

(41)

Thus $s_{f}^{A}>0$ implies $bn_{f}>1$, which is (31) as well as the first inequality of (29).

Households prefer to be in A, which from (13) and (14) implies:

$$\begin{aligned} \left( \theta +1\right) n_{f}s_{f}^{A}+\theta <(b\theta +1)n_{f}(1-s_{f}^{A}) \end{aligned}$$

(42)

Substituting (41), the inequality holds if and only if the second inequality of (29) holds.

In the (fh, f) equilibrium, $bn_{f}>1-n_{f}/\theta$ by (31). By Lemma 1.1, the global welfare optimum is (h, f) in this case.

Reviewing conditions (27) to (29), we see that there is only an HF equilibrium if (26) holds.

Part 3. Solving (23) and (24) yields (30). The condition for $s_{h}^{Aj}<1$ is (26).

Appendix B. The y Locus

In this Appendix we analyze the y locus given by (34) as the solution to $\Psi ^{A}=0$ in (33). This locus contains all the points in AA where payments from households in A to firms in B do not change the relocation speed of firms relative to households.

Lemma B1

Only the following points on the edges of AA satisfy $\Psi ^{A}=0$ in (33):

• 1. the point $(y^{A},0).$ This point always exists.

• 2. the point $(1,y^{1}).$ This point exists if and only if (26) does not hold.

• 3. the interior equilibrium j where $\Delta U_{h}=\Delta U_{f}=0,$ with slope $y^{\prime }$ negative but flatter than $hh^{\prime }.$ This point exists if and only if (26) holds.

• 4. the point (h, 1),  where $\Delta U_{h}=0$ and $s_{f}^{A}=1,$ with slope $y^{\prime }$ negative and steeper than $hh^{\prime },$ or positive. This point exists if and only if (25) does not hold.

• 5. the point (0, 1),  where $s_{h}^{A}=0$ and $s_{f}^{A}=1,$ with slope $y^{\prime }<0.$ This point exists if and only if (25) does not hold.

Proof

Part 1. Since (33) is a quadratic function, there can be at most two $(y^{A},0)$ points in AA. For all $s_{f}^{A}=0,$ define $y_{f}^{A}$ as the payment from each household in A to each firm in B such that firms are indifferent between A and B. From (11) and (12):

$$\begin{aligned} -\theta s_{h}^{A}=-b\theta (n_{f}+1-s_{h}^{A})+y_{f}^{A}s_{h}^{A} \end{aligned}$$

(43)

If the households’ location preference is different for the minimum $s_{h}^{A}$ than for the maximum $s_{h}^{A},$ then there is exactly one $(y^{A},0)$ point in AA.

For the minimum $s_{h}^{A},$ as $s_{h}^{A}$ goes to zero,  $y_{f}^{A}$ in ( 43) goes to infinity, and households prefer B to A.

The maximum $s_{h}^{A}$ in AA is $d^{\prime }$ if $(d^{\prime },0)$ exists and 1 if it does not (if $(h^{\prime },0)$ exists, it is to the right of $(d^{\prime },0)$). At $d^{\prime }$, $y_{f}^{A}=0$ and households prefer A to B.

If the maximum $s_{h}^{A}$ is 1, then $bn_{f}>1$ by Lemma 2.2 and $y_{f}^{A}=\theta \left( bn_{f}-1\right)$ by (43). From (13) and (14):

$$\begin{aligned} V_{h}^{A}=-\theta -\theta n_{f}(bn_{f}-1)\ \ \ \ \ \ \ \ \ V_{h}^{B}=-(b\theta +1)n_{f} \end{aligned}$$

Thus $\Delta V_{h}=n_{f}+\theta \left( 1-n_{f}\right) \left( bn_{f}-1\right) >0.$

Since $\Delta V_{h}<0$ at $s_{h}^{A}\rightarrow 0$ and $\Delta V_{h}>0$ at the maximum $s_{h}^{A},$ there is exactly one point $(y^{A},0)$ on the y curve in AA.

Part 2. First note that by Lemma 2.2, $(1,y^{1})$ only exists in AA if $bn_{f}>1.$Since (33) is a quadratic function, there can be at most two $(1,y^{1})$ points in AA. For all $s_{h}^{A}=1,$ define $y_{f}^{A}$ as the payment from each household in A to each firm in B such that firms are indifferent between A and B. From (11) and (12):

$$\begin{aligned} -\theta (n_{f}s_{f}^{A}+1)=-b\theta n_{f}(1-s_{f}^{A})+y_{f}^{1} \end{aligned}$$

If the households’ location preference is different for $s_{f}^{A}=0$ than for ${\bar{s}}_{f}^{A}\in (0,1],$ then there is exactly one $(1,y^{1})$ point with $y^{1}\in (0,{\bar{s}}_{f}^{A}).$

For $s_{f}^{A}=0,$ $\Delta V_{h}>0$ as we have seen in the proof of Part 1.

By Lemma 2.2, if $bn_{f}>2+n_{f},$ the maximum $s_{f}^{A}$ in AA is at point $h^{\prime }$ with $\Delta U_{h}=0$. Then $y_{f}^{1}>0$ there and $\Delta V_{h}<0.$ Since $\Delta V_{h}>0$ at $s_{f}^{A}=0$ and $\Delta V_{h}<0$ at the maximum $s_{f}^{A},$ there is exactly one point $(1,y^{1})$ on the y curve in AA.

By Lemma 2.2, if $1<bn_{f}<2+n_{f}$, the maximum $s_{f}^{A}$ in AA is at point $d^{\prime }$ with $\Delta U_{f}=0$. Then $y_{f}^{1}=0$ there and $\Delta V_{h}>0.$ Then there are no or two points $(1,y^{1})$ on the y curve in AA. However at (1, 1), the total payment from households in A to firms in B would be zero, because there are no firms in B. Then $\Delta V_{h}<0.$ This means there must be a point $(1,y^{1})$ outside of AA. Then there cannot be a point $(1,y^{1})$ in AA.

Part 3. By (33), the only point on $dd^{\prime }$ with $\Delta U_{f}=0$ where $\Psi ^{A}=0$ is the interior equilibrium j where $\Delta U_{h}=0$ as well (Note that a point (0, d) with $s_{h}^{A}=0,$ $\Delta U_{f}=0$ and $s_{f}^{A}<1$ cannot exist). By Lemma 2.4, point j exists if and only if (26) holds.

From (33), the slope $y^{\prime }$ of a y curve is implicitly given by:

$$\begin{aligned} -\Delta U_{h}+s_{h}^{A}(b+1)\left[ \theta +(\theta +1)n_{f}y^{\prime }\right] -n_{f}\Delta U_{f}y^{\prime }-\theta n_{f}(1-s_{f}^{A})(b+1)\left[ 1+n_{f}y^{\prime }\right] =0 \end{aligned}$$

(44)

When $\Delta U_{f}=\Delta U_{h}=0$, this becomes:

$$\begin{aligned} y^{\prime }(j)=\frac{\theta \left( n_{f}(1-s_{f}^{Aj})-s_{h}^{Aj}\right) }{ n_{f}\left( (\theta +1)s_{h}^{Aj}-\theta n_{f}(1-s_{f}^{Aj})\right) } \end{aligned}$$

Substituting $s_{h}^{Aj}$ and $s_{f}^{Aj}$ from (30) yields:

$$\begin{aligned} y^{\prime }(j)=-\frac{2\theta (b-n_{f})}{n_{f}\left( b+1\right) \left( 2\theta -n_{f}\theta +2\right) }<0 \end{aligned}$$

Comparing $y^{\prime }(j)$ to the slope of $hh^{\prime }$ from (23) yields:

$$\begin{aligned} -\frac{2\theta (b-n_{f})}{n_{f}\left( b+1\right) \left( 2\theta -n_{f}\theta +2\right) }+\frac{\theta }{\left( \theta +1\right) n_{f}}=\frac{\theta \left( 2n_{f}+2\theta +\theta n_{f}-b\theta n_{f}+2\right) }{n_{f}\left( \theta +1\right) \left( b+1\right) \left( 2\theta -n_{f}\theta +2\right) }>0 \end{aligned}$$

Thus y(j) is flatter than $hh^{\prime }.$

Part 4. By (33), the only point on $hh^{\prime }$ with $\Delta U_{h}=0$ where $\Psi ^{A}=0$ apart from point j (see Part 3) is point (h, 1) with $s_{f}^{A}=1.$ By Lemma 2.1, this point exists if and only if (25) does not hold.

For $y^{\prime },$ (44) in (h, 1) becomes:

$$\begin{aligned} s_{h}^{A}(b+1)\left[ \theta +(\theta +1)n_{f}y^{\prime }\right] -n_{f}\theta \Delta U_{f}y^{\prime }=0 \end{aligned}$$

Substituting for $\Delta U_{f}$ from (33) and solving for $y^{\prime }$ yields:

$$\begin{aligned} y^{\prime }=\frac{s_{h}^{A}(b+1)\theta }{\left( -s_{h}^{A}(b+1)(\theta +1)+\theta \left[ b(1-s_{h}^{A})-(n_{f}+s_{h}^{A})\right] \right) n_{f}} \end{aligned}$$

Substituting (40), we obtain:

$$\begin{aligned} y^{\prime }=\frac{\theta \left( b\theta -n_{f}(\theta +1)\right) }{n_{f} \left[ \theta n_{f}-\left( b\theta -n_{f}\left( \theta +1\right) \right) \left( \theta +1\right) \right] } \end{aligned}$$

The sign of the RHS is the sign of the term between square brackets in the denominator. If this term is negative, we can show that the y curve is steeper than $hh^{\prime }$ in (23):

$$\begin{aligned} \frac{\theta \left( b\theta -n_{f}(\theta +1)\right) }{n_{f}\left[ \theta n_{f}-\left( b\theta -n_{f}\left( \theta +1\right) \right) \left( \theta +1\right) \right] }<-\frac{\theta }{n_{f}(\theta +1)} \end{aligned}$$

This is because cross-multiplying and simplifying yields:

$$\begin{aligned} \left[ b\theta -n_{f}\left( \theta +1\right) \right] \left( \theta +1\right) >\left[ b\theta -n_{f}\left( \theta +1\right) \right] \left( \theta +1\right) -\theta n_{f} \end{aligned}$$

Part 5. By (33), the only point where $\Psi ^{A}=0$ with $s_{f}^{A}=1$ apart from point (h, 1) (see Part 4) is point (0, 1) with $s_{h}^{A}=1,\ s_{f}^{A}=0.$ By Lemma 2.1, this point lies in AA if and only if (25) does not hold.

For $y^{\prime },$ (44) in (0, 1) becomes:

$$\begin{aligned} -\Delta U_{h}-n_{f}\Delta U_{f}y^{\prime }=0 \end{aligned}$$

Since $\Delta U_{h}>0$ and $\Delta U_{f}>0$ in AA,  the slope $y^{\prime }$ must be negative. $\square$

Proposition B1

• 1. If and only if (26) does not hold, there exists a unique $y^{1}$ such that $(1,y^{1})$ is on a y curve in AA.

• 2. If and only if (26) holds so that an interior equilibrium j exists, j is on a y curve with negative slope, but flatter than $hh^{\prime }.$

• 3. If the FH laissez faire equilibrium is (f, h), the y locus in AA consists of the right-hand curve only, connecting the point $(y^{A},0)$ with $(1,y^{1})$ or with j.

• 4. If the FH laissez faire equilibrium is (fh, h), there are two y curves in AA.

  • (a) If ${\underline{D}}^{A}<0$ in (37), the left-hand curve connects (0, 1) with (h, 1) and the right-hand curve connects $(y^{A},0)$ with $(1,y^{1})$ or with j.

  • (b) If ${\underline{D}}^{A}>0$ in (37), the lower curve connects (0, 1) with $(y^{A},0)$ and the upper curve connects (h, 1) with $(1,y^{1})$ or with j.

Proof

For the proof of Parts 1 and 2, see Lemma B1.2 and 3 respectively.

Part 3. When the FH equilibrium is (f, h),  condition (25 ) holds. Then by Lemma B1, there are only two points on the edge of AA: $(y^{A},0)$ and either $(1,y^{1})$ or j. There can then only be one y curve. Since condition (25) for (f, h) implies ${\underline{D}} ^{A}<0$ in (37), this is either the left-hand or the right-hand curve. It is the right-hand curve because $(1,y^{1})$ is to the Northwest of $(y^{A},0)$ and the y curve is decreasing at j.

Part 4. When the FH equilibrium is (fh, h),  condition (25 ) does not hold. Then by Lemma B1, there are four points on the edge of AA: $(y^{A},0),$ $(h,1),\ (0,1)$ and either $(1,y^{1})$ or j. These points cannot all be part of the same left-hand or right-hand curve. This is because from (36) the point $s_{h}^{AD}$ where $D^{A}$ in (35) has its minimum satisfies $0<s_{h}^{AD}<1$ (the second inequality follows from $n_{f}<1).$ This means that the whole left-hand curve lies to the left of $s_{h}^{A}=1$ and the whole right-hand curve lies to the right of $s_{h}^{A}=0.$ Thus there are always two y curves, either left-hand and right-hand or upper and lower. $\square$

Since there are many possible scenarios, we focus on two representative and distinct caes with a positive-payment equilibrium in Sect. 7:

Case 1

• 1. Inequality (26) does not hold, so that:

  • (a) by Lemma 2.2, there is no HF laissez faire equilibrium,

  • (b) inequality (31) holds and the positive-payment equilibrium (h, f) exists by Proposition 1.1 and is in AA by Proposition 1.2.

• 2. $\theta (b-n_{f})^{2}>bn_{f}$ so that:

  • (a) inequality (25) does not hold and the FH laissez faire equilibrium is (fh, h) by Lemma 2.1,

  • (b) ${\underline{D}}^{A}>0$ in (37) and the y locus consists of an upper curve $T^{y}(s_{h}^{A})$ connecting (h, 1) with $(1,y^{1})$ and a lower curve $I^{y}(s_{h}^{A})$ connecting (0, 1) with $(y^{A},0)$ by Propositions B1.1 and B1.4b.

Case 2

• (a) Inequality (29) holds, so that:

  • (a) by Lemma 2.2, there is an (fh, f) laissez faire equilibrium,

  • (b) inequality (31) holds and the positive-payment equilibrium (h, f) exists by Proposition 1.1.

• (c) Inequality (25) holds, so that:

  • (a) by Lemma 2.1, the FH laissez faire equilibrium is (f, h), 

  • (b) by Propositions B1.2 and B1.3, the y locus in AA consists of the right-hand curve only, with upper branch $TR^{y}(s_{h}^{A})$ and lower branch $IR^{y}(s_{h}^{A}),$ connecting the point $(y^{A},0)$ with j.

Appendix C. Laissez Faire Saddle Path

In laissez faire, the system of dynamic equations is, from (11) to ( 15) with $x=y=0$:

$$\begin{aligned} \left( \begin{array}{c} {\dot{s}}_{f}^{A} \\ {\dot{s}}_{h}^{A} \end{array} \right) =\left( \begin{array}{cc} -\rho _{f}n_{f}\gamma &{} -\rho _{f}\gamma \\ -n_{f}(\gamma +2) &{} -\gamma \end{array} \right) \left( \begin{array}{c} s_{f}^{A} \\ s_{h}^{A} \end{array} \right) +\left( \begin{array}{c} \rho _{f}\theta (1+n_{f})b \\ \theta (1+n_{f})b+n_{f} \end{array} \right) \end{aligned}$$

(45)

with $\gamma \equiv \theta (1+b)$. The eigenvalues of the 2x2 matrix on the RHS are:

$$\begin{aligned} r_{1}=-\frac{1}{2}\sqrt{\gamma }\left[ (1+\rho _{f}n_{f})\sqrt{\gamma }+ \sqrt{\gamma (\rho _{f}n_{f}+1)^{2}+8\rho _{f}n_{f}}\right] <0 \end{aligned}$$

(46)

with eigenvector $\left( \begin{array}{cc} \mu _{1}&1 \end{array} \right) ^{\prime }$ where:

$$\begin{aligned} \mu _{1}\equiv \frac{\sqrt{\gamma }}{2n_{f}(2+\gamma )}\left[ (n_{f}\rho _{f}-1)\sqrt{\gamma }+\sqrt{\gamma (n_{f}\rho _{f}+1)^{2}+8n_{f}\rho _{f}} \right] >0, \end{aligned}$$

(47)

and

$$\begin{aligned} r_{2}=\frac{1}{2}\sqrt{\gamma }\left[ -(1+\rho _{f}n_{f})\sqrt{\gamma }+ \sqrt{\gamma (\rho _{f}n_{f}+1)^{2}+8\rho _{f}n_{f}}\right] >0 \end{aligned}$$

(48)

with eigenvector $\left( \begin{array}{cc} \mu _{2}&1 \end{array} \right) ^{\prime }$ where

$$\begin{aligned} \mu _{2}=\frac{\sqrt{\gamma }}{2n_{f}(2+\gamma )}\left[ (\rho _{f}n_{f}-1) \sqrt{\gamma }-\sqrt{\gamma (\rho _{f}n_{f}+1)^{2}+8\rho _{f}n_{f}}\right] <0 \end{aligned}$$

(49)

The solution to (45) is then:

$$\begin{aligned} s_{f}^{A}=C_{1}\mu _{1}e^{r_{1}t}+C_{2}\mu _{2}e^{r_{2}t}+\frac{1}{2},\ \ \ \ \ \ \ \ s_{h}^{A}=C_{1}e^{r_{1}t}+C_{2}e^{r_{2}t}+\frac{2b-n_{f}+bn_{f}}{ 2b+2} \end{aligned}$$

(50)

with $r_{1},\mu _{1},r_{2}$ and $\mu _{2}$ given by (46) to (49).

On the saddle path, $C_{2}=0$ in (50). Solving for $s_{f}^{A}$ as a function of $s_{h}^{A}$ then yields:

$$\begin{aligned} SP(s_{h}^{A})=\mu _{1}\left( s_{h}^{A}-\frac{2b-n_{f}+bn_{f}}{2b+2}\right) + \frac{1}{2} \end{aligned}$$