The modified Kudryashov method for solving some fractional-order nonlinear equations

Abstract

In this paper, the modified Kudryashov method is proposed to solve fractional differential equations, and Jumarie’s modified Riemann-Liouville derivative is used to convert nonlinear partial fractional differential equation to nonlinear ordinary differential equations. The modified Kudryashov method is applied to compute an approximation to the solutions of the space-time fractional modified Benjamin-Bona-Mahony equation and the space-time fractional potential Kadomtsev-Petviashvili equation. As a result, many analytical exact solutions are obtained including symmetrical Fibonacci function solutions, hyperbolic function solutions, and rational solutions. This method is powerful, efficient, and it can be used as an alternative to establish new solutions of different types of fractional differential equations applied in mathematical physics.

1 Introduction

Nonlinear partial differential equations of integer order play an important role in describing many nonlinear phenomena such as mathematical biology, electromagnetic theory, fluid mechanics, signal processing, engineering, solid state physics, and other fields of science. With the help of computerized symbolic computations many researchers implemented various methods to establish the solutions to different nonlinear differential equations. For example, the Exp-function method [1–3], the Jacobi elliptic function expansion method [4, 5], the first integral method [6, 7], $(G^{\prime }/G)$-expansion method [8, 9], the direct algebraic method [10], the Cole-Hopf transformation method [11], and others.

Nonlinear fractional differential equations (FDEs) are a generalization of classical differential equations of integer order. Recently, FDEs have attracted great interest, using the fractional derivatives. It is caused both by the development of the theory of fractional calculus itself and by the applications of such constructions in various real life problems. In the past decades the theory of fractional derivatives was represented principally as a pure theoretical field of mathematics effective only for mathematicians. However, in recent years many authors have noticed that derivatives of non-integer order are convenient for the description of the properties of various physical phenomena. It has been shown that fractional-order models are more sufficient than the formerly used integer-order models. Some physical considerations by means of the models based on derivatives of non-integer order are given in [12–15]. New exact solutions for fractional differential equations may help to understand better the corresponding wave phenomena they describe. In order to obtain the solutions for fractional differential equations, many numerical and analytical methods have been proposed so far (e.g. see [12–32]). But the application of a modified Kudryashov method to fractional differential equations has not been researched.

In this paper, we will apply the modified Kudryashov method for solving fractional partial differential equations in the sense of the modified Riemann-Liouville derivative as given by Jumarie [33, 34]. To illuminate the utility and validness of the method, we will apply it to the space-time fractional modified Benjamin-Bona-Mahony (mBBM) equation and the space-time fractional potential Kadomstev-Petviashvili (PKP) equation.

2 Preliminaries and the modified Kudryashov method

Jumarie’s modified Riemann-Liouville derivative is defined as

$$D_x^{\alpha }f(x)=\{\begin{array}{ll}\frac{1}{\mathrm{\Gamma }(-\alpha )}\frac{d}{dx}{\int }_0^x{(x-\xi )}^{-\alpha -1}[f(\xi )-f(0)]d\xi ,& \alpha <0,\\ \frac{1}{\mathrm{\Gamma }(1-\alpha )}\frac{d}{dx}{\int }_0^x{(x-\xi )}^{-\alpha }[f(\xi )-f(0)]d\xi ,& 0<\alpha <1,\\ {(f^{(n)}(x))}^{\alpha -n},& n\le \alpha <n+1,n\ge 1,\end{array}$$

(2.1)

where

$$f^{\alpha }(x):=\underset{h\downarrow 0}{lim}{h}^{-\alpha }\sum _{k=0}^{\mathrm{\infty }}{(-1)}^k\left(\genfrac{}{}{0ex}{}{a}{k}\right)f[x+(\alpha -k)h].$$

(2.2)

Moreover, some properties for the proposed modified Riemann-Liouville derivative are given in [34] as follows:

$$D_t^{\alpha }{t}^{\gamma }=\frac{\mathrm{\Gamma }(1+\gamma )}{\mathrm{\Gamma }(1+\gamma -\alpha )}{t}^{\gamma -\alpha },\gamma >0,$$

(2.3)

$$D_t^{\alpha }(f(t)g(t))=g(t)D_t^{\alpha }f(t)+f(t)D_t^{\alpha }g(t),$$

(2.4)

$$D_t^{\alpha }f[g(t)]=f_g^{\prime }[g(t)]D_t^{\alpha }g(t)=D_g^{\alpha }f[g(t)]{(g^{\prime }(t))}^{\alpha },$$

(2.5)

which are direct results of the equality $D^{\alpha }x(t)=\mathrm{\Gamma }(1+\alpha )Dx(t)$, which holds for non-differentiable functions.

We present the main steps of the modified Kudryashov method as follows [35–39].

For given nonlinear FDEs for a function u of independent variables, $X=(x,y,z,\dots ,t)$:

$$P(u,u_t,u_x,u_y,u_z,\dots ,D_t^{\alpha }u,D_x^{\alpha }u,D_y^{\alpha }u,D_z^{\alpha }u,\dots )=0,$$

(2.6)

where $D_t^{\alpha }u$, $D_x^{\alpha }u$, $D_y^{\alpha }u$, and $D_z^{\alpha }u$ are the modified Riemann-Liouville derivatives of u with respect to t, x, y and z. P is a polynomial in $u=u(x,y,z,\dots ,t)$ and its various partial derivatives, in which the highest-order derivatives and nonlinear terms are involved.

Step 1. We investigate the traveling wave solutions of Eq. (2.6) of the form

$$u(x,y,z,\dots ,t)=u(\xi ),\xi =\frac{k{x}^{\beta }}{\mathrm{\Gamma }(1+\beta )}+\frac{n{y}^{\gamma }}{\mathrm{\Gamma }(1+\gamma )}+\frac{m{z}^{\delta }}{\mathrm{\Gamma }(1+\delta )}+\cdots +\frac{\lambda t^{\alpha }}{\mathrm{\Gamma }(1+\alpha )},$$

(2.7)

where k, n, m and λ are arbitrary constants. Then Eq. (2.6) reduces to a nonlinear ordinary differential equation of the form

$$G=(u,u_{\xi },u_{\xi \xi },u_{\xi \xi \xi },\dots )=0.$$

(2.8)

Step 2. We suppose that the exact solutions of Eq. (2.8) can be obtained in the following form:

$$u(\xi )=\sum _{i=0}^N{a}_i{Q}^i(\xi ),$$

(2.9)

where $Q=\frac{1}{1\pm a^{\xi }}$ and the function Q is the solution of the equation

$$Q_{\xi }=lna(Q^2-Q).$$

(2.10)

Step 3. According to the method, we assume that the solution of Eq. (2.8) can be expressed in the form

$$u(\xi )=a_N{Q}^N+\cdots .$$

(2.11)

In the calculation of the value of N in Eq. (2.11) we have the pole order for the general solution of Eq. (2.8). In order to determine the value of N we balance the highest-order nonlinear terms in Eq. (2.8), analogously as in the classical Kudryashov method. Supposing $u^l(\xi )u^{(s)}(\xi )$ and ${(u^{(p)}(\xi ))}^r$ are the highest-order nonlinear terms of Eq. (2.8) and balancing the highest-order nonlinear terms we have

$$N=\frac{s-rp}{r-l-1}.$$

(2.12)

Step 4. Substituting Eq. (2.9) into Eq. (2.8) and equating the coefficients of $Q^i$ to zero, we get a system of algebraic equations. By solving this system, we obtain the exact solutions of Eq. (2.8). The obtained solutions can depend on the symmetrical hyperbolic Fibonacci functions proposed by Stakhov and Rozin [40]. The symmetrical Fibonacci sine, cosine, tangent, and cotangent functions are, respectively, defined as

$$\begin{array}{c}\mathit{sFs}(x)=\frac{a^x-a^{-x}}{\sqrt{5}},\mathit{cFs}(x)=\frac{a^x+a^{-x}}{\sqrt{5}},\hfill \\ \mathit{tanFs}(x)=\frac{a^x-a^{-x}}{a^x+a^{-x}},\mathit{cotFs}(x)=\frac{a^x+a^{-x}}{a^x-a^{-x}}.\hfill \end{array}$$

3 Applications

3.1 Space-time fractional modified Benjamin-Bona-Mahony (mBBM) equation

We first apply the method to the space-time fractional mBBM equation in the form

$$D_t^{\alpha }u+D_x^{\alpha }u-v{u}^2{D}_x^{\alpha }u+D_t^{\alpha }\left(D_t^{\alpha }\left(D_t^{\alpha }u\right)\right)=0,$$

(3.1)

where $0<\alpha \le 1$, $t>0$, u is the function of $(x,t)$ and v is a nonzero positive constant.

This equation was first derived to describe an approximation for surface long waves in nonlinear dispersive media. It can also characterize the hydromagnetic waves in a cold plasma, acoustic waves in harmonic crystals, and acoustic gravity waves in compressible fluids.

We proceed by considering the traveling wave transformation,

$$u(x,t)=u(\xi ),\xi =\frac{k{x}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+{\xi }_0,$$

(3.2)

where k, c, ${\xi }_0$ are constants.

Equation (3.1) can be reduced to the following ordinary differential equation:

$$c{u}^{\prime }+k{u}^{\prime }-vk{u}^2{u}^{\prime }+k^3{u}^{‴}=0.$$

(3.3)

Also we take

$$g(\xi )=u(\xi )=\sum _{i=0}^N{a}_i{Q}^i,$$

(3.4)

where $Q=\frac{1}{1\pm a^{\xi }}$. We note that the function Q is the solution of $Q_{\xi }=lna(Q^2-Q)$. Balancing $g^{″}$ and $g^3$ in Eq. (3.3), we compute

$$N=1.$$

(3.5)

Thus, we have

$$g(\xi )=u(\xi )=a_0+a_{1}Q,$$

(3.6)

and substituting derivatives of $u(\xi )$ with respect to ξ in Eq. (3.4) we obtain

$$u_{\xi }=lna(a_1{Q}^2-a_{1}Q),$$

(3.7)

$$u_{\xi \xi }={(lna)}^2(2a_1{Q}^3-3a_1{Q}^2+a_{1}Q).$$

(3.8)

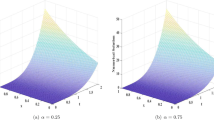
Substituting Eq. (3.7) and Eq. (3.8) into Eq. (3.3) and collecting the coefficients of each power of $Q^i$, setting each of the coefficients to zero, solving the resulting system of algebraic equations we obtain the following solutions (see Figures 1-8).

Figure 1

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{1}$ .

Figure 2

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.5}$ .

Figure 3

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.25}$ .

Figure 4

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.1}$ .

Figure 5

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{1}$ .

Figure 6

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.5}$ .

Figure 7

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.25}$ .

Figure 8

Solitary wave solutions of Eq. ( 3.1 ) are shown at $\mathit{k}\mathbf{=}\mathit{v}\mathbf{=}{\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.1}$ .

Case 1:

$$a_0=\sqrt{\frac{3}{2vk}}{k}^{3/2}(lna),a_1=-\sqrt{\frac{6}{vk}}{k}^{3/2}(lna),c=\frac{1}{2}k(-2+k^2{(lna)}^2).$$

(3.9)

Inserting Eq. (3.9) into Eq. (3.6), we obtain the following solutions of Eq. (3.1):

$$u_1(x,t)=(lna)\sqrt{\frac{3}{2kv}}{k}^{3/2}\mathit{tanFs}(\frac{k{x}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{{\xi }_0}{2}),$$

(3.10)

$$u_2(x,t)=(lna)\sqrt{\frac{3}{2kv}}{k}^{3/2}\mathit{cotFs}(\frac{k{x}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{{\xi }_0}{2}).$$

(3.11)

Case 2:

$$a_0=-\sqrt{\frac{3}{2vk}}{k}^{3/2}(lna),a_1=\sqrt{\frac{6}{vk}}{k}^{3/2}(lna),c=\frac{1}{2}k(-2+k^2{(lna)}^2).$$

(3.12)

Inserting Eq. (3.9) into Eq. (3.6), we obtain the following solutions of Eq. (3.1):

$$u_3(x,t)=-(lna)\sqrt{\frac{3}{2kv}}{k}^{3/2}\mathit{tanFs}(\frac{k{x}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{{\xi }_0}{2}),$$

(3.13)

$$u_4(x,t)=-(lna)\sqrt{\frac{3}{2kv}}{k}^{3/2}\mathit{cotFs}(\frac{k{x}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{2\mathrm{\Gamma }(1+\alpha )}+\frac{{\xi }_0}{2}).$$

(3.14)

3.2 The space-time fractional potential Kadomstev-Petviashvili (PKP) equation

We next consider the following the space-time fractional potential Kadomstev-Petviashvili (PKP) equation:

$$\frac{1}{4}{D}_t^{\alpha }\left(D_t^{\alpha }\left(D_t^{\alpha }\left(D_t^{\alpha }u\right)\right)\right)+\frac{3}{2}{D}_x^{\alpha }u{D}_x^{\alpha }\left(D_x^{\alpha }u\right)+\frac{3}{4}{D}_y^{\alpha }\left(D_y^{\alpha }u\right)+D_t^{\alpha }\left(D_x^{\alpha }u\right)=0,$$

(3.15)

where $0<\alpha \le 1$, $t>0$.

We proceed by considering the traveling wave transformation,

$$u(x,t)=u(\xi ),\xi =\frac{k{x}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+\frac{l{y}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+{\xi }_0,$$

(3.16)

where k, l, c, ${\xi }_0$ are constants.

Equation (3.15) can be reduced to the following ordinary differential equation:

$$\frac{1}{4}{k}^4{u}^{‴}+\frac{3}{2}{k}^3{\left(u^{\prime }\right)}^2{u}^{″}+\frac{3}{4}{l}^2{u}^{\prime }+kc{u}^{\prime }=0.$$

(3.17)

Also we take

$$g(\xi )=u(\xi )=\sum _{i=0}^N{a}_i{Q}^i,$$

(3.18)

where $Q=\frac{1}{1\pm a^{\xi }}$.

We note that the function Q is the solution of $Q_{\xi }=lna(Q^2-Q)$. Balancing the linear term of the highest order with the highest-order nonlinear term in Eq. (3.17), we compute

$$N=1.$$

(3.19)

Thus, we have

$$g(\xi )=u(\xi )=a_0+a_{1}Q,$$

(3.20)

and substituting derivatives of $u(\xi )$ with respect to ξ in Eq. (3.18) we obtain

$$u_{\xi }=lna(a_1{Q}^2-a_{1}Q),$$

(3.21)

$$u_{\xi \xi }={(lna)}^2(2a_1{Q}^3-3a_1{Q}^2+a_{1}Q),$$

(3.22)

$$u_{\xi \xi \xi }={(lna)}^3(6a_1{Q}^4-12a_1{Q}^3+12a_1{Q}^2+a_{1}Q).$$

(3.23)

Substituting Eq. (3.21), Eq. (3.22), and Eq. (3.23) into Eq. (3.17) and collecting the coefficient of each power of $Q^i$, setting each of the coefficients to zero, solving the resulting system of algebraic equations we obtain the following solutions (see Figures 9-16):

$$a_0=0,a_1=-2k(lna),c=\frac{-{(lna)}^2{k}^4-3l^2}{4}.$$

(3.24)

Inserting Eq. (3.24) into Eq. (3.20), we obtain the following solution of Eq. (3.15):

$$u(x,y,t)=-2k\left(\frac{1}{1\pm a^{\frac{k{x}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+\frac{l{y}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+\frac{c{t}^{\alpha }}{\mathrm{\Gamma }(1+\alpha )}+{\xi }_0}}\right).$$

(3.25)

Figure 9

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{1}$ .

Figure 10

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.5}$ .

Figure 11

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.25}$ .

Figure 12

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.1}$ .

Figure 13

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{1}$ .

Figure 14

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.5}$ .

Figure 15

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathit{e}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.25}$ .

Figure 16

Solitary wave solutions of Eq. ( 3.15 ) are shown at $\mathit{k}\mathbf{=}\mathit{l}\mathbf{=}\mathit{i}\sqrt{\mathbf{3}}$ , ${\mathit{\xi }}_{\mathbf{0}}\mathbf{=}\mathbf{1}$ , $\mathit{a}\mathbf{=}\mathbf{10}$ , $\mathit{\alpha }\mathbf{=}\mathbf{0.1}$ .

4 Conclusion

We have extended the modified Kudryashov method to solve fractional partial differential equations. As applications, for the space-time fractional potential Kadomstev-Petviashvili equation we found similar solutions to the ones previously obtained in [26, 32]. However, for the space-time fractional modified Benjamin-Bona-Mahony equation we have obtained new symmetrical hyperbolic Fibonacci function solutions with differences from the solutions obtained before [25]. If we take $a=e$, we can also find the other hyperbolic solutions similar to [25, 26, 32]. The method is based on the homogeneous balancing principle. Therefore, it can also be applied to other fractional partial differential equations where the homogeneous balancing principle is satisfied. We can easily conclude that symmetrical hyperbolic Fibonacci function solutions for the space-time fractional modified Benjamin-Bona-Mahony equation have not been reported in the previous studies.