Skip to main content
SpringerLink
Log in

Concept of Coherence in Neutrino and Antineutrino Scattering off Nuclei

  • Published:
Physics of Particles and Nuclei Aims and scope Submit manuscript

Abstract

The concept of coherence in the scattering of neutrinos and antineutrinos off nuclei is discussed. Motivated by the results of the COHERENT experiment, a new approach to coherence in these processes is proposed, which allows a unified description of the elastic (coherent) and inelastic (incoherent) contributions to the total cross section for neutrino and antineutrino scattering off nuclei at energies below 100 MeV. Experiments and physical problems for coherent scattering of (anti)neutrinos off nuclei are briefly discussed. The extended appendix covers the main points and conclusions of the proposed approach in pedagogical detail.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1.
Fig. 2.
Fig. 3.
Fig. 4.
Fig. 5.
Fig. 6.
Fig. 7.
Fig. 8.
Fig. 9.
Fig. 10.
Fig. 11.
Fig. 12.
Fig. 13.
Fig. 14.
Fig. 15.
Fig. 16.
Fig. 17.
Fig. 18.
Fig. 19.
Fig. 20.
Fig. 21.

Similar content being viewed by others

Notes

  1. In [3], the role of coherent neutrino scattering off the nuclear medium in supernova evolution is discussed. Formulas are given for the overall amplitude of coherent scattering off spin-zero (\(J = 0\)) nuclei with \(Z = N\)

    $$M({\mathbf{k}}{\kern 1pt} ',{\mathbf{k}}) \sim GA{{e}^{{ - {{q}^{2}}{{R}^{2}}}}}( - {{\sin}^{2}}{{\theta }_{W}}){{\bar {u}}_{l}}(\nu {\kern 1pt} '){{\gamma }^{0}}{{u}_{\nu }}(\nu ),\,\,\,\,\,\,\,\,\,\,\,\,$$
    (12)

    and for differential and total cross sections

    $$\begin{gathered} \frac{{d{{\sigma }_{A}}}}{{d\cos\theta }} \simeq \frac{{{{\sigma }_{0}}}}{8}{{( - {{\sin}^{2}}{{\theta }_{W}})}^{2}}{{A}^{2}}{{\omega }^{2}}(1 + \cos\theta ),\,\,\, \\ {{\sigma }_{A}} \simeq \frac{{{{\sigma }_{0}}}}{4}{{( - {{\sin}^{2}}{{\theta }_{W}})}^{2}}{{A}^{2}}{{\omega }^{2}}. \\ \end{gathered} $$

    The neutrino energy is assumed to be \(\omega \ll {{m}_{A}}\) (point nucleus). If \(J \ne 0\) and \(Z \ne N\), it is necessary to sum over individual nucleons

    $$\begin{gathered} M({\mathbf{k}}{\kern 1pt} ',{\mathbf{k}}) \sim G{{e}^{{ - {{q}^{2}}{{R}^{2}}}}}\left[ { - {{\sin}^{2}}{{\theta }_{W}}(Z + N) + \frac{{1 - 2{{\sin}^{2}}{{\theta }_{W}}}}{2}(Z - N)} \right. \\ \left. { - \frac{{{{g}_{V}}}}{2}({{Z}_{ \uparrow }} - {{Z}_{ \downarrow }}) + \frac{{{{g}_{A}}}}{2}({{N}_{ \uparrow }} - {{N}_{ \downarrow }})} \right]\bar {u}(\nu {\kern 1pt} '){{\gamma }^{0}}u(\nu ), \\ \end{gathered} $$

    where the arrow indicates nucleons with spins directed upward and downward that are scattered via axial neutral current. For all nuclei (except very light ones) the most important term in \(M({\mathbf{k}}{\kern 1pt} ',{\mathbf{k}})\) is proportional to \(( - {{\sin}^{2}}{{\theta }_{W}})(Z + N)\), in other words, relation (13) is usually valid. For spin-zero (\(J = 0\)) nuclei with \(Z \ne N\) there is a relation to the nuclear isospin \({{I}_{3}} = \tfrac{1}{2}(Z - N)\) in the form

    $$\begin{gathered} \frac{{d\sigma _{A}^{{J = 0}}}}{{d\cos\theta }} \simeq \frac{{{{\sigma }_{0}}}}{8}{{A}^{2}}si{{n}^{4}}{{\theta }_{W}}\mathop {\left[ {1 + \frac{{1 - 2{{\sin}^{2}}{{\theta }_{W}}}}{{ - {{\sin}^{2}}{{\theta }_{W}}}}\frac{{{{I}_{3}}}}{A}} \right]}\nolimits^2 {{\omega }^{2}}(1 + \cos\theta ),\,\,\, \\ \sigma _{A}^{{J = 0}} \simeq \frac{{{{\sigma }_{0}}}}{4}si{{n}^{4}}{{\theta }_{W}}{{A}^{2}}{{[1 + ...]}^{2}}{{\omega }^{2}}. \\ \end{gathered} $$

  2. Perhaps, these works are the first where attention is explicitly paid to the role of inelastic processes in coherent neutrino scattering. In [19], this role can be thought of as being formulated quantitatively.

  3. In the Standard Model, \(g_{V}^{e} = \frac{1}{2} + 2{{\sin}^{2}}{{\theta }_{W}},\,\,g_{V}^{p} = \frac{1}{2} - \) \(2{{\sin}^{2}}{{\theta }_{W}},\) \(g_{V}^{n} = - \frac{1}{2},\) \(g_{A}^{e} = \frac{1}{2},\) \(g_{A}^{p} = \frac{{{{g}_{A}}}}{2},\) \(g_{A}^{n} = - \frac{{{{g}_{A}}}}{2}.\)

  4. That is, by averaging over the initial projections of the spin and summation over all final projections of the nuclear spin, as is clearly seen in formula (23). Unfortunately, nothing is said about invariability of the final state of the nucleus, which is necessary for fulfillment of the coherence condition. This problem is discussed in more detail in appendix 8.1.

  5. In [34], there is another parameter used to characterize (partial) coherence, the relative cross section variation \(\xi \equiv \frac{{\sigma (\alpha )}}{{\sigma (\alpha = 1)}} = \alpha + (1 - \alpha )\frac{{({{\varepsilon }^{2}}Z + N)}}{{{{{(\varepsilon Z - N)}}^{2}}}}\), which linearly depends on \(\alpha \), and they both are unity in the case of complete coherence.

  6. Indeed, if in, say, the two-nucleon case there is symmetry \(\psi _{n}^{{( * )}}({\mathbf{y}},{\mathbf{x}}) = \) \(( \pm )\psi _{n}^{{( * )}}({\mathbf{x}},{\mathbf{y}})\), then \(f_{{mn}}^{{k = 1}}({\mathbf{q}}) \equiv \) \(\int {d{{{\mathbf{x}}}_{1}}} d{{{\mathbf{x}}}_{2}}\psi _{m}^{ * }({{{\mathbf{x}}}_{1}},{{{\mathbf{x}}}_{2}}){{\psi }_{n}}({{{\mathbf{x}}}_{1}},{{{\mathbf{x}}}_{2}}){{e}^{{i{\mathbf{q}}{{{\mathbf{x}}}_{1}}}}}\) can be written as \(\int {d{{{\mathbf{x}}}_{1}}} d{\mathbf{y}}\psi _{m}^{ * }({{{\mathbf{x}}}_{1}},{\mathbf{y}}){{\psi }_{n}}({{{\mathbf{x}}}_{1}},{\mathbf{y}}){{e}^{{i{\mathbf{q}}{{{\mathbf{x}}}_{1}}}}}\) and after designating \({{{\mathbf{x}}}_{1}}\) as \({{{\mathbf{x}}}_{2}}\) one arrives at \(f_{{mn}}^{{k = 1}}({\mathbf{q}})\) = \(\int {d{{{\mathbf{x}}}_{2}}d{\mathbf{y}}} \psi _{m}^{ * }({{{\mathbf{x}}}_{2}},{\mathbf{y}}){{\psi }_{n}}({{{\mathbf{x}}}_{2}},{\mathbf{y}}){{e}^{{i{\mathbf{q}}{{{\mathbf{x}}}_{2}}}}}\). Considering the abovementioned wave function symmetry condition and permutation of the integration variables, this expression takes the form \(\int {d{\mathbf{y}}d{{{\mathbf{x}}}_{2}}} \psi _{m}^{ * }({\mathbf{y}},{{{\mathbf{x}}}_{2}}){{\psi }_{n}}({\mathbf{y}},{{{\mathbf{x}}}_{2}}){{e}^{{i{\mathbf{q}}{{{\mathbf{x}}}_{2}}}}}\), which is indistinguishable from the definition of \(f_{{mn}}^{{k = 2}}({\mathbf{q}})\).

  7. \({{\left| \mathcal{A} \right|}^{2}} = \sum\nolimits_m {{{{\left| {{{\mathcal{A}}_{{mn}}}} \right|}}^{2}}} \) = \({{\left| {{{\mathcal{A}}_{0}}} \right|}^{2}}\sum\nolimits_{k,j} {\sum\nolimits_m {f_{{mn}}^{{j,\dag }}} } ({\mathbf{q}})f_{{mn}}^{k}({\mathbf{q}})\) = \({{\left| {{{\mathcal{A}}_{0}}} \right|}^{2}}\sum\nolimits_{k,j} {\left\langle n \right|} {{e}^{{ - i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_j }}}\sum\nolimits_m {\left| m \right\rangle } \left\langle m \right|{{e}^{{i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_k }}}\left| n \right\rangle \to (40).\)

  8. Indeed, the quantity \({{\left| \mathcal{A} \right|}^{2}}\) divided for simplicity by \({{\left| {{{\mathcal{A}}_{0}}} \right|}^{2}}\) can be written as a sum of two terms \(\sum\nolimits_{k = j}^A {\left\langle n \right|{{e}^{{ - i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_j }}}{{e}^{{i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_k }}}\left| n \right\rangle } \) + \(\sum\nolimits_{j,k \ne j}^A {\left\langle n \right|} {{e}^{{ - i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_j }}}{{e}^{{i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_k }}}\left| n \right\rangle \), the first of which is \(\sum\nolimits_{k = j}^A 1 \), and the second is \(G({\mathbf{q}})\sum\nolimits_{j,k \ne j}^A 1 \), and this results in \(A + A(A - 1)G({\mathbf{q}})\). Hence follows expression (42).

  9.  Equation (47) can be solved  analytically using the MATHEMATICA package or manually by temporarily designating \(E \equiv \sqrt {{{m}^{2}} + {{p}^{2}} + 2pq + {{q}^{2}}} \) – \(\sqrt {{{m}^{2}} + {{p}^{2}}} \), which yields \({{m}^{2}} + {{p}^{2}} + 2pq + {{q}^{2}}\) = \({{(E + \sqrt {{{m}^{2}} + {{p}^{2}}} )}^{2}}\) = \({{E}^{2}} + 2E\sqrt {{{m}^{2}} + {{p}^{2}}} + {{m}^{2}} + {{p}^{2}}\), and hence, cancelling identical terms on the right and on the left, we have \(2pq + {{q}^{2}}\) = \({{E}^{2}} + 2E\sqrt {{{m}^{2}} + {{p}^{2}}} \)or \(2pq + d = 2E\sqrt {{{m}^{2}} + {{p}^{2}}} \), where the designation \(d = ({{q}^{2}} - {{E}^{2}})\) is introduced. Then, raising to the second power \(4{{E}^{2}}({{m}^{2}} + {{p}^{2}})\) = \({{(2pq + d)}^{2}} = 4{{p}^{2}}{{q}^{2}} + 4dpq + {{d}^{2}}\)and transforming the resulting expression to \(4{{p}^{2}}({{E}^{2}} - {{q}^{2}})\) – \(4dpq + 4{{E}^{2}}{{m}^{2}} - {{d}^{2}} = 0\), we arrive at the quadratic equation \(4d{{p}^{2}} + 4dpq + {{d}^{2}} - 4{{E}^{2}}{{m}^{2}} = 0\). That is, we have the equation \({{p}^{2}} + pq + b = 0\), where \(b = \frac{{{{d}^{2}} - 4{{E}^{2}}{{m}^{2}}}}{{4d}}\), and two solutions \({{p}_{{1,2}}} = - \frac{q}{2}\left( {1 \pm {{{\left[ {\frac{{{{q}^{2}} - 4b}}{{{{q}^{2}}}}} \right]}}^{{1/2}}}} \right)\). Further, \({{q}^{2}} - 4b\) = E2 + \(\frac{{4{{E}^{2}}{{m}^{2}}}}{{{{q}^{2}} - {{E}^{2}}}} = \) \({{q}^{2}}\beta \left( {1 + \frac{{4{{m}^{2}}}}{{{{q}^{2}}}}\frac{1}{{1 - \beta }}} \right)\), where \(\beta = \frac{{{{E}^{2}}}}{{{{q}^{2}}}} \equiv \frac{1}{{{{q}^{2}}}}{{\left( {\frac{{{{{\mathbf{q}}}^{2}}}}{{2{{m}_{A}}}} + \Delta {{\varepsilon }_{{mn}}}} \right)}^{2}}\). Finally, one physical solution has the form \(p = - \frac{q}{2}\left( {1 - \sqrt \beta \sqrt {1 + \frac{{4{{m}^{2}}}}{{{{q}^{2}}}}\frac{1}{{1 - \beta }}} } \right)\).

  10.  Indeed, \({{T}_{A}} = {{E}_{\nu }}\) – \(\frac{{{{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} \mathord{\left/ {\vphantom {{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} 2}} \right. \kern-0em} 2} + {{E}_{\nu }}{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}{{E}_{\nu }}}}{{{{m}_{A}} + {{E}_{\nu }} - {{E}_{\nu }}\cos\theta - \Delta {{\varepsilon }_{{mn}}}}} - \) \(\Delta {{\varepsilon }_{{mn}}}\). By reducing to the common denominator, removing the parentheses and cancelling out the identical terms, we obtain \({{T}_{A}} = \frac{{E_{\nu }^{2} - E_{\nu }^{2}\cos\theta - {{E}_{\nu }}\Delta {{\varepsilon }_{{mn}}} + \Delta {{\varepsilon }_{{mn}}}{{E}_{\nu }}\cos\theta + {{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} \mathord{\left/ {\vphantom {{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} 2}} \right. \kern-0em} 2}}}{{{{m}_{A}} + {{E}_{\nu }} - {{E}_{\nu }}\cos\theta - \Delta {{\varepsilon }_{{mn}}}}}\) = \(\frac{{{{E}_{\nu }}({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}})(1 - \cos\theta ) + {{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} \mathord{\left/ {\vphantom {{{{\Delta }^{2}}{{\varepsilon }_{{mn}}}} 2}} \right. \kern-0em} 2}}}{{{{m}_{A}} + {{E}_{\nu }}(1 - \cos\theta ) - \Delta {{\varepsilon }_{{mn}}}}}.\) Hence, considering \({{m}_{A}} \gg {{E}_{\nu }},{{m}_{A}} \gg \Delta {{\varepsilon }_{{mn}}}\), there follows formula (58).

  11.  Derivation of expression (73) for the hadronic current \(h_{{mn}}^{\mu }\) from (70) is given in appendix 10.2.1.

  12.  Actually, \(i{{\mathcal{M}}_{{mn}}} = i\frac{{{{G}_{{\text{F}}}}}}{{\sqrt 2 }}{{l}_{\mu }}(k,k{\kern 1pt} ')H_{{mn}}^{\mu }({{P}_{n}},P_{m}^{'}) = i\frac{{{{G}_{{\text{F}}}}}}{{\sqrt 2 }}{{l}_{\mu }}(k,k{\kern 1pt} ')\) × \(\sqrt {2P_{n}^{0}2P{\kern 1pt}_{m}^{'0}} h_{{mn}}^{\mu }\) \( = i\frac{{{{G}_{{\text{F}}}}}}{{\sqrt 2 }}{{l}_{\mu }}(k,k{\kern 1pt} ')\sqrt {\frac{{P_{n}^{0}P{\kern 1pt}_{m}^{'0}}}{{{{E}_{{{\mathbf{\bar {p}}}}}}{{E}_{{{\mathbf{\bar {p}}} + {\mathbf{q}}}}}}}} \sum\limits_{k = 1}^A {\left\langle {m\left| {{{e}^{{i{\mathbf{q}}\mathop {{\mathbf{\hat {X}}}}\nolimits_k }}}} \right|n} \right\rangle } \,\) × \(\chi _{m}^{ * }(\{ {{r}^{{(k)}}}\} ){{\chi }_{n}}(\{ r\} )\bar {u}({\mathbf{\bar {p}}} + {\mathbf{q}},{{s}_{k}})O_{k}^{\mu }u({\mathbf{\bar {p}}},{{r}_{k}}).\)

  13.  The square of the matrix element \({{\left| {i{{\mathcal{M}}_{{mn}}}} \right|}^{2}}\) does not depend on the azimuth angle ϕ (see appendix 10.4 or formulas from Section 6.3), and therefore the integration was carried out over it.

  14.  For details see section 10.3.

  15.  This approximation can be a subject of special consideration in the future.

  16.  In the explicit form: \(\begin{gathered} \frac{{d\sigma _{{{\text{inc}}}}^{\nu }}}{{d{{T}_{A}}}} = \frac{{2G_{{\text{F}}}^{2}{{m}_{A}}}}{\pi }\sum\limits_{f = p,n} {{{F}_{f}}} \left\{ {{{A}^{f}}\left[ {{{{(g_{L}^{f})}}^{2}} + {{{(g_{R}^{f})}}^{2}}{{{(1 - y)}}^{2}} - g_{L}^{f}g_{R}^{f}\frac{{2{{m}^{2}}y}}{{s - {{m}^{2}}}}} \right]} \right. + ( + \Delta {{A}^{f}})\left[ {g_{L}^{f} - g_{R}^{f}(1 - y)} \right]\left. {\left[ {g_{L}^{f} + g_{R}^{f}\left( {1 - y\frac{{s + {{m}^{2}}}}{{s - {{m}^{2}}}}} \right)} \right]} \right\}, \\ \frac{{d\sigma _{{{\text{inc}}}}^{{\bar {\nu }}}}}{{d{{T}_{A}}}} = \frac{{2G_{{\text{F}}}^{2}{{m}_{A}}}}{\pi }\sum\limits_{f = p,n} {{{F}_{f}}} \left\{ {{{A}^{f}}\left[ {{{{(g_{R}^{f})}}^{2}} + {{{(g_{L}^{f})}}^{2}}{{{(1 - y)}}^{2}} - g_{L}^{f}g_{R}^{f}\frac{{2{{m}^{2}}y}}{{s - {{m}^{2}}}}} \right]} \right. + \left. {( - \Delta {{A}^{f}})\left[ {g_{R}^{f} - g_{L}^{f}(1 - y)} \right]\left[ {g_{R}^{f} + g_{L}^{f}\left( {1 - y\frac{{s + {{m}^{2}}}}{{s - {{m}^{2}}}}} \right)} \right]} \right\}. \\ \end{gathered} \)

  17.  More details about these form factors are given in appendix 8.1.2.

  18.  With few exclusions, e.g., the DAMA/LIBRA collaboration [91, 92].

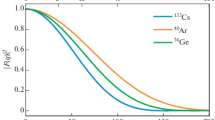
  19.  In the COHERENT experiment, target nuclei have a nonzero spin, and therefore, according to formulas (128) and (129), antineutrino-induced CEvNS differs from neutrino-induced CEvNS.

  20.  Since \({{E}_{{\mathbf{p}}}}{{\delta }^{3}}({\mathbf{p}} - {\mathbf{k}}) = {{E}_{{{\mathbf{p}}*}}}{{\delta }^{3}}({\mathbf{p}}{\text{*}} - \,\,{\mathbf{k}}*)\).

  21.  To verify the transformation, one can substitute the upper expression (177) into the lower one, take into account the spinor normalization \({{u}^{\dag }}({\mathbf{p}},s)u({\mathbf{p}},s) = 2{{E}_{{\mathbf{p}}}}\), that \(\int {\frac{{d{\mathbf{x}}{{e}^{{i({\mathbf{p}}{\kern 1pt} '\,\, - {\mathbf{p}}){\mathbf{x}}}}}}}{{{{{(2\pi )}}^{3}}}}} = \) \({{\delta }^{3}}({\mathbf{p}}{\kern 1pt} '\,\, - {\mathbf{p}})\), and obtain the identity

    $$\begin{gathered} \tilde {\psi }({\mathbf{p}},s) = \int {d{\mathbf{x}}\frac{{{{u}^{\dag }}({\mathbf{p}},s){{e}^{{ - i{\mathbf{px}}}}}}}{{\sqrt {2{{E}_{{\mathbf{p}}}}} }}} \int {\frac{{d{\mathbf{p}}{\kern 1pt} '}}{{{{{(2\pi )}}^{3}}}}} u({\mathbf{p}}{\kern 1pt} ',s) \\ \times \,\,\frac{{{{e}^{{i{\mathbf{p}}{\kern 1pt} '{\mathbf{x}}}}}}}{{\sqrt {2{{E}_{{{\mathbf{p'}}}}}} }}\tilde {\psi }({\mathbf{p}}{\kern 1pt} ',s) = \int {d{\mathbf{p}}{\kern 1pt} '} \int {\frac{{d{\mathbf{x}}{{e}^{{i({\mathbf{p}}{\kern 1pt} '\,\, - {\mathbf{p}}){\mathbf{x}}}}}}}{{{{{(2\pi )}}^{3}}}}} \\ \times \,\,\frac{{{{u}^{\dag }}({\mathbf{p}},s)u({\mathbf{p}}{\kern 1pt} ',s)}}{{\sqrt {2{{E}_{{\mathbf{p}}}}} \sqrt {2{{E}_{{{\mathbf{p'}}}}}} }}\tilde {\psi }({\mathbf{p}}{\kern 1pt} ',s) \\ = \frac{{{{u}^{\dag }}({\mathbf{p}},s)u({\mathbf{p}},s)}}{{2{{E}_{{\mathbf{p}}}}}}\tilde {\psi }({\mathbf{p}},s) = \tilde {\psi }({\mathbf{p}},s). \\ \end{gathered} $$

  22.  Or, considering (174), we calculate the matrix element \(\left\langle {{\mathbf{x}}\left| {{{e}^{{i{\mathbf{q\hat {X}}}}}}} \right|{\mathbf{p}}} \right\rangle = \int {\frac{{d{\mathbf{p}}{\kern 1pt} '}}{{{{{(2\pi )}}^{3}}}}} {{e}^{{i{\mathbf{q\hat {X}}}}}}\frac{{e{\kern 1pt} '}}{{\sqrt {2{{E}_{{{\mathbf{p}}{\kern 1pt} '}}}} }}\left\langle {\left. {{\mathbf{p}}{\kern 1pt} '} \right|{\mathbf{p}}} \right\rangle \). Since \({{e}^{{i{\mathbf{q\hat {X}}}}}} \cdot {{e}^{{i{\mathbf{xp}}}}} = {{e}^{{i{\mathbf{x}}({\mathbf{q}} + {\mathbf{p}})}}}\), then \(\left\langle {{\mathbf{x}}\left| {{{e}^{{i{\mathbf{q\hat {X}}}}}}} \right|{\mathbf{p}}} \right\rangle \int {\frac{{d{\mathbf{p}}{\kern 1pt} '}}{{{{{(2\pi )}}^{3}}}}} \frac{{{{e}^{{i{\mathbf{qx}}}}}{{e}^{{i{\mathbf{xp}}{\kern 1pt} '}}}}}{{\sqrt {2{{E}_{{{\mathbf{p}}{\kern 1pt} '}}}} }}{{(2\pi )}^{3}}2{{E}_{{\mathbf{p}}}}\delta ({\mathbf{p}} - {\mathbf{p}}{\kern 1pt} ')\) = \(\sqrt {2{{E}_{{\mathbf{p}}}}} {{e}^{{i{\mathbf{x}}({\mathbf{q}} + {\mathbf{p}})}}}\). Definition (172) of the scalar product can be written in the form \({{e}^{{i{\mathbf{x}}({\mathbf{q}} + {\mathbf{p}})}}} = \frac{{\left\langle {\left. {\mathbf{x}} \right|{\mathbf{q}} + {\mathbf{p}}} \right\rangle }}{{\sqrt {2{{E}_{{{\mathbf{p}} + {\mathbf{q}}}}}} }}\), from which follows (178).

  23.  This is verified by direct substitution of \(\psi (\{ x\} )\) into \(\tilde {\psi }(\{ p\} )\) and the use of the spinor normalization condition

    $$\begin{gathered} \tilde {\psi }(\{ p\} \equiv ({{p}_{i}},{{s}_{i}},{{m}_{i}})) \\ = \int {\left( {\prod\limits_i^n {d{{{\mathbf{x}}}_{i}}\frac{{u_{{{{m}_{i}}}}^{\dag }({{{\mathbf{p}}}_{i}},{{s}_{i}})}}{{\sqrt {2{{E}_{{{{{\mathbf{p}}}_{i}}}}}} }}{{e}^{{ - i{{{\mathbf{p}}}_{i}}{{{\mathbf{x}}}_{i}}}}}} } \right)} \\ \times \,\,\int {\left( {\prod\limits_j^n {\frac{{d{\mathbf{p}}_{j}^{'}}}{{{{{(2\pi )}}^{3}}\sqrt {2{{E}_{{{\mathbf{p}}_{j}^{'}}}}} }}{{u}_{{{{m}_{j}}}}}({\mathbf{p}}_{j}^{'},{{s}_{j}}){{e}^{{i{\mathbf{p}}_{j}^{'}{{{\mathbf{x}}}_{i}}}}}} } \right)} \tilde {\psi }(\{ p{\kern 1pt} '\} ) \\ = \int {\prod\limits_{i,j}^n {\underbrace {\frac{{d{{{\mathbf{x}}}_{i}}}}{{{{{(2\pi )}}^{3}}}}{{e}^{{i{{{\mathbf{x}}}_{i}}({\mathbf{p}}_{j}^{'} - {{{\mathbf{p}}}_{i}})}}}}_{{{\delta }^{3}}({{{\mathbf{p}}}_{i}} - {\mathbf{p}}_{j}^{'})}} } \frac{{d{{{\mathbf{p}}}_{j}}}}{{\sqrt {2{{E}_{{{{{\mathbf{p}}}_{i}}}}}2{{E}_{{{\mathbf{p}}_{j}^{'}}}}} }} \\ \times \,\,\underbrace {u_{{{{m}_{i}}}}^{\dag }({{{\mathbf{p}}}_{i}},{{s}_{i}}){{u}_{{{{m}_{j}}}}}({\mathbf{p}}_{j}^{'},{{s}_{j}})}_{2{{E}_{{{\mathbf{p}}_{j}^{'}}}}{{\delta }_{{{{s}_{i}},{{s}_{j}}}}}{{\delta }_{{{{m}_{i}},{{m}_{j}}}}}{{\delta }^{3}}({{{\mathbf{p}}}_{i}} - {\mathbf{p}}_{j}^{'})}\tilde {\psi }(\{ p{\kern 1pt} '\} \\ = (p_{j}^{'},s_{j}^{'},m_{j}^{'})) = \tilde {\psi }({{p}_{i}},{{s}_{i}},{{m}_{i}}). \\ \end{gathered} $$

  24.  Considering (60), from the relation \({{E}_{\nu }} - E_{\nu }^{'} - \Delta {{\varepsilon }_{{mn}}}\) = \(\sqrt {m_{A}^{2} + E_{\nu }^{2} + {{{(E_{\nu }^{'})}}^{2}} - 2E_{\nu }^{'}{{E}_{\nu }}\cos\theta } - {{m}_{A}}\) we have \({{({{E}_{\nu }} - E_{\nu }^{'} - \Delta {{\varepsilon }_{{mn}}} + {{m}_{A}})}^{2}}\) = \(m_{A}^{2} + E_{\nu }^{2} + {{(E_{\nu }^{'})}^{2}} - 2E_{\nu }^{'}{{E}_{\nu }}\cos\theta \), from which, using expansion (229), we obtain \(\Delta \varepsilon _{{mn}}^{2} - 2{{E}_{\nu }}\mathop {E'}\nolimits_\nu - 2{{E}_{\nu }}\Delta {{\varepsilon }_{{mn}}}\) + \(2{{E}_{\nu }}{{m}_{A}} + 2E_{\nu }^{'}\Delta {{\varepsilon }_{{mn}}} - 2E_{\nu }^{'}{{m}_{A}}\) – \(2{{m}_{A}}\Delta {{\varepsilon }_{{mn}}} = - 2E_{\nu }^{'}{{E}_{\nu }}\cos\theta .\)Then from \(\Delta \varepsilon _{{mn}}^{2} - 2{{m}_{A}}\Delta {{\varepsilon }_{{mn}}} + \) \(2{{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})\) = \(2E_{\nu }^{'}({{E}_{\nu }} - {{E}_{\nu }}\cos\theta - \Delta {{\varepsilon }_{{mn}}} + {{m}_{A}})\) there follows formula (227).

  25.  From (228) it follows that \({{T}_{A}}(\tilde {E}_{\nu }^{'}) = {{E}_{\nu }} - E_{\nu }^{'} - \Delta {{\varepsilon }_{{mn}}}\), where \(\tilde {E}_{\nu }^{'} = \tfrac{{{{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} - {{m}_{A}}\Delta {{\varepsilon }_{{mn}}}}}{{{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta )}}\). That is, \({{T}_{A}}(\tilde {E}_{\nu }^{'}) = {{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}}\) – \(\frac{{{{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} - {{m}_{A}}\Delta {{\varepsilon }_{{mn}}}}}{{{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta )}}\) \( = \,\,\,\frac{{({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}})({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta ))}}{{{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta )}}\) \( - \,\,\,\,\frac{{{{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} - {{m}_{A}}\Delta {{\varepsilon }_{{mn}}}}}{{{{m}_{A}} - \Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta )}}.\) Then, \({{T}_{A}}(\tilde {E}_{\nu }^{'})\left[ {{{m}_{A}} - } \right.\) \(\left. {\Delta {{\varepsilon }_{{mn}}} + {{E}_{\nu }}(1 - \cos\theta )} \right] = ({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}})\left[ {({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})} \right. + {{E}_{\nu }}\) \(\left. {(1 - \cos\theta )} \right]\) – \([{{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2}\,\,\,\, - \) \({{m}_{A}}\Delta {{\varepsilon }_{{mn}}}] = \) \(({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}})({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})\) \( + \,\,({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}}){{E}_{\nu }}(1 - \cos\theta ) - \) \({{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) - {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} + {{m}_{A}}\Delta {{\varepsilon }_{{mn}}} = {{E}_{\nu }}(1 - \cos\theta )\) + \(({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}})\) + \({{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) - {{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})\) \( - \,\,{{m}_{A}}\Delta {{\varepsilon }_{{mn}}} + \Delta \varepsilon _{{mn}}^{2} - {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} + {{m}_{A}}\Delta {{\varepsilon }_{{mn}}} = {{E}_{\nu }}(1 - \cos\theta )\) × \(({{E}_{\nu }} - \Delta {{\varepsilon }_{{mn}}}) + \Delta \varepsilon _{{mn}}^{2} - {{\Delta \varepsilon _{{mn}}^{2}} \mathord{\left/ {\vphantom {{\Delta \varepsilon _{{mn}}^{2}} 2}} \right. \kern-0em} 2} \to \) (230).

  26. Indeed, raising both sides to the second power and using expansion (229), we have

    $$\begin{gathered} \cos\theta = \frac{{m_{A}^{2} + E_{\nu }^{2} + {{{(E_{\nu }^{'})}}^{2}} - {{{({{E}_{\nu }} - E_{\nu }^{'} + {{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})}}^{2}}}}{{2E_{\nu }^{'}{{E}_{\nu }}}} \\ = \frac{{ - \Delta \varepsilon _{{mn}}^{2} + 2{{E}_{\nu }}E_{\nu }^{'} - 2{{E}_{\nu }}{{m}_{A}} + 2{{E}_{\nu }}\Delta {{\varepsilon }_{{mn}}} + 2E_{\nu }^{'}{{m}_{A}} - 2E_{\nu }^{'}\Delta {{\varepsilon }_{{mn}}} + 2{{m}_{A}}\Delta {{\varepsilon }_{{mn}}}}}{{2E_{\nu }^{'}{{E}_{\nu }}}} \\ = 1 + \frac{{E_{\nu }^{'}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) - {{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + \Delta {{\varepsilon }_{{mn}}}({{m}_{A}} - {{\Delta {{\varepsilon }_{{mn}}}} \mathord{\left/ {\vphantom {{\Delta {{\varepsilon }_{{mn}}}} 2}} \right. \kern-0em} 2})}}{{\mathop {E'}\nolimits_\nu {{E}_{\nu }}}} \\ = 1 + \frac{{({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}})}}{{{{E}_{\nu }}}} + \frac{{ - {{E}_{\nu }}({{m}_{A}} - \Delta {{\varepsilon }_{{mn}}}) + \Delta {{\varepsilon }_{{mn}}}({{m}_{A}} - {{\Delta {{\varepsilon }_{{mn}}}} \mathord{\left/ {\vphantom {{\Delta {{\varepsilon }_{{mn}}}} 2}} \right. \kern-0em} 2})}}{{\mathop {E'}\nolimits_\nu {{E}_{\nu }}}}. \\ \end{gathered} $$

  27.  Since \({{{\mathbf{n}}}_{{{{k}_{i}}}}} \cdot \sigma = (0,0,1) \cdot \left[ {\left( {\begin{array}{*{20}{c}} 0&1 \\ 1&0 \end{array}} \right),\left( {\begin{array}{*{20}{c}} 0&{ - i} \\ i&0 \end{array}} \right),\left( {\begin{array}{*{20}{c}} 1&0 \\ 0&{ - 1} \end{array}} \right)} \right] = \left( {\begin{array}{*{20}{c}} 1&0 \\ 0&{ - 1} \end{array}} \right)\).

  28.  Because \(\left( {\begin{array}{*{20}{c}} 1&0 \\ 0&{ - 1} \end{array}} \right)\left( {\begin{array}{*{20}{c}} 0 \\ 1 \end{array}} \right) = - \left( {\begin{array}{*{20}{c}} 0 \\ 1 \end{array}} \right)\) and \(\left( {\begin{array}{*{20}{c}} 1&0 \\ 0&{ - 1} \end{array}} \right)\left( {\begin{array}{*{20}{c}} 1 \\ 0 \end{array}} \right) = + \left( {\begin{array}{*{20}{c}} 1 \\ 0 \end{array}} \right).\)

  29.  From here on the following trigonometric relations are used: \({{e}^{{i\phi }}} = \cos\phi + i\sin\phi \to - i\cos\varphi + \sin\phi = - i{{e}^{{i\phi }}},\) \(i\cos\phi + \sin\varphi = i{{e}^{{ - i\phi }}}.\) \(cos\theta = \sin\theta = 2\sin\frac{\theta }{2}\cos\frac{\theta }{2},\) \(\tan\frac{\theta }{2}\sin\theta + \cos\theta = 1,\) \(\cot\frac{\theta }{2}\sin\theta - \cos\theta = 1.\)

  30.  A possibility of a change in (anti)neutrino helicity after the interaction is not considered. Though, from the point of view of searching for manifestations of New Physics, this effect may in principle have a right to exist if, for example, one assumes nonzero (anti)neutrino masses .

  31.  Or using in parallel the MATHEMATICA package.

  32.  The sign (\( - \)) temporarily separated out below reflects the (abovementioned and insignificant) difference in the definition of the nucleon initial spinor \({{\chi }_{ + }}({{{\mathbf{p}}}_{i}})\): \({{\chi }_{ + }}{{({{{\mathbf{p}}}_{i}})}_{{{\text{here}}}}}\) in (246) \( = - {{\chi }_{ + }}({{{\mathbf{p}}}_{i}})\) in (83) from [19].

  33. \({{g}_{{\mu \nu }}} = \left( {\begin{array}{*{20}{c}} 1&0&0&0 \\ 0&{ - 1}&0&0 \\ 0&0&{ - 1}&0 \\ 0&0&0&{ - 1} \end{array}} \right)\). For neutrinos, \(({{l}_{\nu }},{{h}_{{r{\kern 1pt} 'r}}})\bar {u}(k{\kern 1pt} ', - 1){{\gamma }^{\mu }}(1 - {{\gamma }^{5}})\) \(u(k, - 1)\bar {u}(p{\kern 1pt} ',r{\kern 1pt} '){{\gamma }_{\mu }}({{g}_{V}} - {{g}_{A}}{{\gamma }^{5}})u(p,r)\).

  34.  By virtue of the explicit equations for the four-spinors \(u({\mathbf{k}})\) and \(v({\mathbf{k}})\), and procedures for deriving formulas (262) and (263).

  35.  In [19] only the neutrino case was considered. Unfortunately, in formula (C33), \((l,h_{{ + + }}^{\eta }) = 8(s - {{m}^{2}})\cos\frac{\theta }{2}\) × \(\left( {{{g}_{L}} - {{g}_{R}}{{\sin}^{2}}\frac{\theta }{2}\frac{m}{{\sqrt s }}(1 - \frac{m}{{\sqrt s }})} \right)\), an unhappy misprint was made (the sign \( - \) of \({{g}_{R}}\), cf. (299)).

  36.  For example, for any structure similar to the current like \(Q_{{ + - }}^{\eta } = \eta _{ + }^{\dag }({{{\mathbf{p}}}_{f}})Q{{\eta }_{ - }}({{{\mathbf{p}}}_{i}})\) we have in the helicity basis, according to transformation matrices (302), \([\left[ {\sin\tfrac{\theta }{2}\chi _{ + }^{\dag }({{{\mathbf{p}}}_{f}}) + {{e}^{{ - i\phi }}}\cos\tfrac{\theta }{2}\chi _{ - }^{\dag }({{{\mathbf{p}}}_{f}})} \right]Q[( \pm 1){{\chi }_{ + }}({{{\mathbf{p}}}_{i}})]\), from where follows \(Q_{{ + - }}^{\eta } = ( \pm 1)\left\{ {\sin\tfrac{\theta }{2}Q_{{ + + }}^{\chi } + {{e}^{{ - i\phi }}}\cos\tfrac{\theta }{2}Q_{{ - + }}^{\chi }} \right\}\), which is equivalent to the top formula in (304).

  37.  In these formulas, \({{\chi }_{ + }}({{{\mathbf{p}}}_{i}}) = - {{\chi }_{ + }}{{({{{\mathbf{p}}}_{i}})}_{{{\text{here}}}}}\) for the incoming nucleon, as in [19].

  38.  In the squares of the scalars the dependence on \({{\chi }_{ + }}({{{\mathbf{p}}}_{i}}) = - {{\chi }_{ + }}{{({{{\mathbf{p}}}_{i}})}_{{{\text{mine}}}}}\) disappears.

  39.  A good approximation, when \(m \equiv {{m}_{p}} \equiv {{m}_{n}}\).

  40.  For convenience, they are given below in terms of a and b, divided by the factor \(64{{(s - {{m}^{2}})}^{2}}\),

    $$\begin{gathered} {{\left| {\left( {{{l}_{\nu }} \cdot h_{{ - - }}^{\eta }} \right)} \right|}^{2}} = (1 - a)g_{R}^{2}{{\left[ {1 - (1 - b)a} \right]}^{2}},\,\,\,\,{{\left| {\left( {{{l}_{{\bar {\nu }}}} \cdot h_{{ - - }}^{\eta }} \right)} \right|}^{2}} = (1 - a){{\left[ {{{g}_{R}} + {{g}_{L}}b(1 - b)a} \right]}^{2}}, \\ {{\left| {\left( {{{l}_{\nu }} \cdot h_{{ + + }}^{\eta }} \right)} \right|}^{2}} = (1 - a)g_{L}^{2}{{\left[ {1 - (1 - b)a} \right]}^{2}},\,\,\,\,{{\left| {\left( {{{l}_{\nu }} \cdot h_{{ + + }}^{\eta }} \right)} \right|}^{2}} = (1 - a){{\left[ {{{g}_{L}} + {{g}_{L}}b(1 - b)a} \right]}^{2}}, \\ {{\left| {\left( {{{l}_{\nu }} \cdot h_{{ - + }}^{\eta }} \right)} \right|}^{2}} = a{{({{g}_{L}} - {{g}_{R}}b\left[ {1 - (1 - b)a} \right])}^{2}},\,\,\,\,{{\left| {\left( {{{l}_{\nu }} \cdot h_{{ + - }}^{\eta }} \right)} \right|}^{2}} = a{{(1 - a)}^{2}}g_{R}^{2}{{(1 - b)}^{2}}, \\ {{\left| {\left( {{{l}_{\nu }} \cdot h_{{ + - }}^{\eta }} \right)} \right|}^{2}} = a{{({{g}_{R}} - {{g}_{L}}b\left[ {1 - (1 - b)a} \right])}^{2}},\,\,\,\,{{\left| {\left( {{{l}_{{\bar {\nu }}}} \cdot h_{{ - + }}^{\eta }} \right)} \right|}^{2}} = a{{(1 - a)}^{2}}g_{L}^{2}{{(1 - b)}^{2}}. \\ \end{gathered} $$

  41. \(X = 1 - 2(1 - b)a + {{(1 - b)}^{2}}{{a}^{2}} + (a - {{a}^{2}}){{(1 - b)}^{2}} = 1 - 2a + 2ab + (a + 2ab + a{{b}^{2}}) = 1 - a + a{{b}^{2}}.\)

  42. \(\begin{gathered} Z(P,Q) = a{{(P - \left[ {Qb - Qb(1 - b)a} \right])}^{2}} + (1 - a){{[P + Qb(1 - b)a]}^{2}} = (1 - a){{[P + Qab(1 - b)]}^{2}} + a{{[[P + Qab(1 - b)] - Qb]}^{2}} \\ = {{[P + Qab(1 - b)]}^{2}}\underbrace { - a{{{[P + Qab(1 - b)]}}^{2}} + a{{{[P + Qab(1 - b)]}}^{2}}}_0 + 2a[ - P - Qab(1 - b)]Qb + a{{Q}^{2}}{{b}^{2}} = {{P}^{2}} + 2PQab(1 - b) \\ + \,\,{{Q}^{2}}{{a}^{2}}{{b}^{2}}{{(1 - b)}^{2}} - 2PQab - 2{{Q}^{2}}{{a}^{2}}{{b}^{2}}(1 - b) + a{{Q}^{2}}{{b}^{2}} = {{P}^{2}} - 2PQa{{b}^{2}} + {{Q}^{2}}{{a}^{2}}{{b}^{2}}(1 - 2b + {{b}^{2}}) - 2{{Q}^{2}}{{a}^{2}}{{b}^{2}}(1 - b) \\ + \,\,a{{Q}^{2}}{{b}^{2}}\underbrace { + 2PQab - 2PQab}_0 = {{P}^{2}} - 2PQa{{b}^{2}} + {{Q}^{2}}{{a}^{2}}{{b}^{2}} + 2{{Q}^{2}}{{a}^{2}}{{b}^{3}} + {{Q}^{2}}{{a}^{2}}{{b}^{2}} + 2{{Q}^{2}}{{a}^{2}}{{b}^{3}} + a{{Q}^{2}}{{b}^{2}} \\ = \underbrace {{{P}^{2}} - 2PQa{{b}^{2}} + {{Q}^{2}}{{a}^{2}}{{b}^{4}} + {{Q}^{2}}{{a}^{2}}{{b}^{2}} - 2{{Q}^{2}}{{a}^{2}}{{b}^{2}}}_{{{{(P - Qa{{b}^{2}})}}^{2}}} + a{{Q}^{2}}{{b}^{2}} + \underbrace {2{{Q}^{2}}{{a}^{2}}{{b}^{3}} - 2{{Q}^{2}}{{a}^{2}}{{b}^{3}}}_0 = {{(P - Qa{{b}^{2}})}^{2}} + {{Q}^{2}}a{{b}^{2}}(1 - a). \\ \end{gathered} \)

REFERENCES

  1. D. Z. Freedman, “Coherent effects of a weak neutral current,” Phys. Rev. D 9, 1389–1392 (1974).

    Article  ADS  Google Scholar 

  2. V. B. Kopeliovich and L. L. Frankfurt, “Isotopic and chiral structure of neutral current,” JETP Lett. 19, 145–147 (1974).

    ADS  Google Scholar 

  3. D. Z. Freedman, D. N. Schramm, and D. L. Tubbs, “The weak neutral current and its effects in stellar collapse,” Ann. Rev. Nucl. Part. Sci. 27, 167–207 (1977).

    Article  ADS  Google Scholar 

  4. Yu. V. Gaponov and V. N. Tikhonov, “Elastic scattering of low-energy neutrinos by atomic systems,” Yad. Fiz. 26, 594–600 (1977).

    Google Scholar 

  5. L. M. Sehgal and M. Wanninger, “Atomic effects in coherent neutrino scattering,” Phys. Lett. B 171, 107–112 (1986).

    Article  ADS  Google Scholar 

  6. M. Cadeddu, F. Dordei, C. Giunti, K. A. Kouzakov, E. Picciau, and A. I. Studenikin, “Potentialities of a low-energy detector based on 4He evaporation to observe atomic effects in coherent neutrino scattering and physics perspectives,” [arXiv]1907.03302.

  7. J. Barranco, O. G. Miranda, and T. I. Rashba, “Probing new physics with coherent neutrino scattering off nuclei,” J. High Energy Phys., No. 12, 21 (2005); [arXiv] hep-ph/0508299.

  8. D. Akimov et al. (COHERENT Collab.), “Observation of coherent elastic neutrino-nucleus scattering,” Science 357, 1123–1126 (2017); [arXiv]1708.01294.

  9. D. Akimov et al. (COHERENT Collab.), “The COHERENT experiment at the spallation neutron source,” [arXiv]1509.08702.

  10. D. Akimov et al. (COHERENT Collab.), “COHERENT 2018 at the spallation neutron source,” [arXiv] 1803.09183.

  11. D. Akimov et al. (COHERENT Collab.), “COHERENT Collaboration data release from the first observation of coherent elastic neutrino-nucleus scattering,” [arXiv]1804.09459.

  12. Scholberg K. (COHERENT Collab.), “Observation of coherent elastic neutrino-nucleus scattering by COHERENT,” PoS NuFact2017, 020 (2018); [arXiv] 1801.05546.

  13. D. Akimov et al. (COHERENT Collab.), “Observation of coherent elastic neutrino-nucleus scattering,” Science 357, 1123–1126 (2017); [arXiv]1708.01294.

  14. J. I. Collar, N. E. Fields, M. Hai, T. W. Hossbach, J. L. Orrell, C. T. Overman, G. Perumpilly, and B. Scholz, “Coherent neutrino-nucleus scattering detection with a CsI[Na] scintillator at the SNS spallation source,” Nucl. Instrum. Methods Phys. Res., Sect. A 773, 56–65 (2015); [arXiv]1407.7524.

  15. D. Akimov et al. (CSI Collab.), “Coherent scattering investigations at the spallation neutron source: A Snowmass white paper,” [arXiv]1310.0125.

  16. A. Bolozdynya et al., “Opportunities for neutrino physics at the spallation neutron source: A white paper,” [arXiv]1211.5199.

  17. K. Scholberg, “Prospects for measuring coherent neutrino-nucleus elastic scattering at a stopped-pion neutrino source,” Phys. Rev. D 73, 033005 (2006); [arXiv] hep-ex/0511042.

  18. D. Akimov et al. (COHERENT Collab.), “First detection of coherent elastic neutrino-nucleus scattering on argon,” [arXiv]2003.10630.

  19. V. A. Bednyakov and D. V. Naumov, “Coherency and incoherency in neutrino-nucleus elastic and inelastic scattering,” Phys. Rev. D 98, 053004 (2018); [arXiv] 1806.08768.

  20. V. A. Bednyakov and D. V. Naumov, “On coherent neutrino and antineutrino scattering off nuclei,” Phys. Part. Nucl. Lett. 16, 638–646 (2019); [arXiv] 1904.03119.

  21. T. W. Donnelly and J. D. Walecka, “Electron scattering and nuclear structure,” Annu. Rev. Nucl. Part. Sci. 25, 329–405 (1975).

    Article  ADS  Google Scholar 

  22. J. Bernabeu, “Low-energy elastic neutrino-nucleon and nuclear scattering and its relevance for supernovae,” Preprint CERN-TH-2073 (CERN, Geneva, 1975).

    Google Scholar 

  23. P. S. Amanik and G. C. McLaughlin, “Neutron form factor from neutrino-nucleus coherent elastic scattering,” [arXiv]0707.4191.

  24. C. J. Horowitz, K. J. Coakley, and D. N. McKinsey, “Supernova observation via neutrino-nucleus elastic scattering in the CLEAN detector,” Phys. Rev. D 68, 023005 (2003); [arXiv]astro-ph/0302071.

  25. J. Engel, “Nuclear form factors for the scattering of weakly interacting massive particles,” Phys. Lett. B 264, 114–119 (1991).

    Article  ADS  Google Scholar 

  26. P. S. Amanik and G. C. McLaughlin, “Nuclear neutron form factor from neutrino nucleus coherent elastic scattering,” J. Phys. G 36, 015105 (2009).

    Article  ADS  Google Scholar 

  27. E. Akhmedov, G. Arcadi, M. Lindner, and S. Vogl, “Coherent scattering and macroscopic coherence: Implications for neutrino, dark matter and axion detection,” J. High Energy Phys., No 10, 45 (2018); [arXiv] 1806.10962.

  28. Yu. V. Gaponov, Yu. L. Dobrynin, and V. N. Tikhonov, “Neutrino and anti-neutrino scattering on hydrogen-like atom,” Yad. Fiz. 22, 328–334 (1975).

    Google Scholar 

  29. J. Weber, “Gravitons, neutrinos, and anti-neutrinos,” Found. Phys. 14, 1185–1209 (1984).

    Article  ADS  Google Scholar 

  30. J. Weber, “Method for observation of neutrinos and antineutrinos,” Phys. Rev. C 31, 1468–1475 (1985).

    Article  ADS  Google Scholar 

  31. J. Weber, “Apparent observation of abnormally large coherent scattering cross-sections using keV and MeV range anti-neutrinos, and solar neutrinos,” Phys. Rev. D 38, 32–39 (1988).

    Article  ADS  Google Scholar 

  32. L. D. Landau and E. M. Lifshitz, Electrodynamics of Continuous Media, 2nd ed. (Pergamon Press, Oxford, 1984) Vol. 8, Secs. 124 and 125.

  33. P. F. Smith, “Coherent neutrino scattering – relativistic and nonrelativistic,” Nuovo Cimento A 83, 263–274 (1984).

    Article  ADS  Google Scholar 

  34. S. Kerman, V. Sharma, M. Deniz, H. T. Wong, J. W. Chen, H. B. Li, S. T. Lin, C.-P. Liu, and Q. Yue (TEXONO Collab.), “Coherency in neutrino-nucleus elastic scattering,” Phys. Rev. D 93, 113006 (2016); [arXiv]1603.08786.

  35. D. K. Papoulias and T. S. Kosmas, “Standard and nonstandard neutrino-nucleus reactions cross sections and event rates to neutrino detection experiments,” Adv. High Energy Phys. 2015, 763648 (2015); [arXiv] 1502.02928.

  36. D. K. Papoulias and T. S. Kosmas, “Nuclear aspects of neutral current non-standard ν-nucleus reactions and the role of the exotic μ– → e– transitions experimental limits,” Phys. Lett. B 728, 482–488 (2014); [arXiv] 1312.2460.

  37. P. Vogel and J. Engel, “Neutrino electromagnetic form factors,” Phys. Rev. D 39, 3378 (1989).

    Article  ADS  Google Scholar 

  38. P. C. Divari, “Coherent and incoherent neutral current scattering for supernova detection,” Adv. High Energy Phys. 2012, 379460 (2012).

    Article  MATH  Google Scholar 

  39. P. Pirinen, J. Suhonen, and E. Ydrefors, “Neutral-current neutrino-nucleus scattering off Xe isotopes,” [arXiv]1804.08995.

  40. V. Tsakstara, T. Kosmas, and J. Vergados, “Weak responses of neutral current neutrino-nucleus reactions,” Rom. J. Phys. 58, 1258–1269 (2013).

    Google Scholar 

  41. M. E. Peskin and D. V. Schroeder, An Introduction to Quantum Field Theory (Addison-Wesley, Reading, 1995).

    Google Scholar 

  42. S. M. Bilenky, Introduction to Feynman Diagrams and Electroweak Interactions Physics (Ed. Frontieres, Gif-sur-Yvette, 1995).

  43. A. Drukier and L. Stodolsky, “Principles and applications of a neutral current detector for neutrino physics and astronomy,” Phys. Rev. D 30, 2295 (1984).

    Article  ADS  Google Scholar 

  44. K. Patton, J. Engel, G. C. McLaughlin, and N. Schunck, “Neutrino-nucleus coherent scattering as a probe of neutron density distributions,” Phys. Rev. C 86, 024612 (2012); [arXiv]1207.0693.

  45. N. Jachowicz, K. Heyde, and S. Rombouts, “Many body description of neutrino nucleus interactions,” Nucl. Phys. A 688, 593–595 (2001).

    Article  ADS  Google Scholar 

  46. P. C. Divari, V. C. Chasioti, and T. S. Kosmas, “Neutral current neutrino-Mo-98 reaction cross sections at low and intermediate energies,” Phys. Scripta 82, 065201 (2010).

    Article  ADS  MATH  Google Scholar 

  47. G. McLaughlin, “Theory and phenomenology of coherent neutrino-nucleus scattering,” AIP Conf. Proc. 1666, 160001 (2015).

    Article  Google Scholar 

  48. J. D. Vergados, F. T. Avignone, III, and I. Giomataris, “Coherent neutral current neutrino-nucleus scattering at a spallation source: A valuable experimental probe,” Phys. Rev. D 79, 113001 (2009); [arXiv]0902.1055.

  49. J. Papavassiliou, J. Bernabeu, and M. Passera, “Neutrino-nuclear coherent scattering and the effective neutrino charge radius,” PoS HEP2005, 192 (2006); [arXiv]hep-ph/0512029.

  50. V. V. Belov et al., “Investigation of neutrino properties with the low-background germanium spectrometer gemma-iii,” in JINR Program Advisory Committee, 2018.

  51. D. Barker and D. M. Mei, “Germanium detector response to nuclear recoils in searching for dark matter,” Astropart. Phys. 38, 1–6 (2012); [arXiv]1203.4620.

  52. V. Belov et al., “The NuGeN experiment at the Kalinin Nuclear Power Plant,” J. Instrum. 10, P12011 (2015).

    Article  Google Scholar 

  53. P. Agnes et al. (DarkSide Collab.), “Low-mass dark matter search with the DarkSide-50 experiment,” [arXiv]1802.06994.

  54. J. Piekarewicz, A. R. Linero, P. Giuliani, and E. Chicken, “Power of two: Assessing the impact of a second measurement of the weak-charge form factor of 208Pb,” Phys. Rev. C 94, 034316 (2016); [arXiv] 1604.07799.

  55. R. H. Helm, “Inelastic and elastic scattering of 187-MeV electrons from selected even-even nuclei,” Phys. Rev. D 104, 1466–1475 (1956).

    Article  ADS  Google Scholar 

  56. M. Cadeddu and F. Dordei, “Reinterpreting the weak mixing angle from atomic parity violation in view of the Cs neutron rms radius measurement from COHERENT,” Phys. Rev. D 99, 033010 (2019); [arXiv]1808.10202.

  57. C. Boehm, D. G. Cerdeño, P. A. N. Machado, A. O.‑D. Campo, and E. Reid, “How high is the neutrino floor?” J. Cosmol. Astropart. Phys. 1901, 043 (2019); [arXiv]1809.06385.

  58. V. Brdar, W. Rodejohann, and X.-J. Xu, “Producing a new fermion in coherent elastic neutrino-nucleus scattering: From neutrino mass to dark matter,” J. High Energy Phys., No. 12, 24 (2018); [arXiv]1810.03626.

  59. M. Cadeddu, C. Giunti, K. A. Kouzakov, Y. F. Li, A. I. Studenikin, and Y. Y. Zhang, “Neutrino charge radii from COHERENT elastic neutrino-nucleus scattering,” Phys. Rev. D 98, 113010 (2018); [arXiv] 1810.05606.

  60. A. Millar, G. Raffelt, L. Stodolsky, and E. Vitagliano, “Neutrino mass from bremsstrahlung endpoint in coherent scattering on nuclei,” Phys. Rev. D 98, 123006 (2018); [arXiv]1810.06584.

  61. W. Altmannshofer, M. Tammaro, and J. Zupan, “Non-standard neutrino interactions and low energy experiments,” [arXiv]1812.02778.

  62. C. Blanco, D. Hooper, and P. Machado, “Constraining sterile neutrino interpretations of the LSND and MiniBooNE anomalies with coherent neutrino scattering experiments,” [arXiv]1901.08094.

  63. D. Aristizabal Sierra, J. Liao, and D. Marfatia, “Impact of form factor uncertainties on interpretations of coherent elastic neutrino-nucleus scattering data,” [arXiv]1902.07398.

  64. X.-R. Huang and L.-W. Chen, “Neutron skin in CsI and low-energy effective weak mixing angle from COHERENT data,” [arXiv]1902.07625.

  65. O. G. Miranda, G. Sanchez Garcia, and O. Sanders, “Testing new physics with future COHERENT experiments,” [arXiv]1902.09036.

  66. D. K. Papoulias, T. S. Kosmas, R. Sahu, V. K. B. Kota, and M. Hota, “Constraining nuclear physics parameters with current and future COHERENT data,” [arXiv] 1903.03722.

  67. J. I. Collar, A. R. L. Kavner, and C. M. Lewis, “Response of CsI[Na] to nuclear recoils: Impact on coherent elastic neutrino-nucleus scattering (CEνNS),” [arXiv]1907.04828.

  68. D. K. Papoulias, “COHERENT constraints after the Chicago-3 quenching factor measurement,” [arXiv] 1907.11644.

  69. A. N. Khan and W. Rodejohann, “New physics from COHERENT data with improved quenching factors,” [arXiv]1907.12444.

  70. D. V. Naumov and V. A. Naumov, “A diagrammatic treatment of neutrino oscillations,” J. Phys. G 37, 105014 (2010); [arXiv]1008.0306.

  71. D. V. Naumov, “On the theory of wave packets,” Phys. Part. Nucl. Lett. 10, 642–650 (2013); [arXiv] 1309.1717.

  72. D. Karlovets, “Scattering of wave packets with phases,” J. High Energy Phys., No. 03, 49 (2017); [arXiv]1611.08302.

  73. J. R. Wilson, “Coherent neutrino scattering and stellar collapse,” Phys. Rev. Lett. 32, 849–852 (1974).

    Article  ADS  Google Scholar 

  74. S. Rombouts and K. Heyde, “Calculation of neutrino nucleus scattering cross-sections relevant for supernova processes,” Nucl. Phys. A 621, 371–374 (1997).

    Article  ADS  Google Scholar 

  75. E. P. O’Connor and S. M. Couch, “Two dimensional core-collapse supernova explosions aided by general relativity with multidimensional neutrino transport,” Astrophys. J. 854, 63 (2018); [arXiv]1511.07443.

  76. Y. Giomataris and J. D. Vergados, “A network of neutral current spherical TPC’s for dedicated supernova detection,” Phys. Lett. B 634, 23–29 (2006); [arXiv] hep-ex/0503029.

  77. J. D. Vergados and Y. Giomataris, “Neutral current coherent cross sections. Implications on detecting SN and earth neutrinos with gaseous spherical TPC,” Int. J. Mod. Phys. 2017. V. E26, no. 01n02, P. 1740030, [arXiv]1603.03966.

  78. P. C. Divari, S. Galanopoulos, and G. A. Souliotis, “Coherent scattering of neutral-current neutrinos as a probe for supernova detection,” J. Phys. G 39, 095204 (2012).

    Article  ADS  Google Scholar 

  79. G. I. Lykasov and V. A. Bednyakov, “Neutrino-nucleus interactions at low energies within Fermi-liquid theory,” Phys. Rev. 76, 014622 (2007); [arXiv]nucl-th/0703036.

  80. L. Coraggio, L. De Angelis, T. Fukui, A. Gargano, and N. Itaco, “Calculation of Gamow-Teller and two-neutrino double-β decay properties for 130Te and 136Xe with a realistic nucleon-nucleon potential,” Phys. Rev. C 95, 064324 (2017); [arXiv]1703.05087.

  81. R. H. Helm, “Inelastic and elastic scattering of 187-MeV electrons from selected even-even nuclei,” Phys. Rev. 104, 1466–1475 (1956).

    Article  ADS  Google Scholar 

  82. D. W. L. Sprung and J. Martorell, “The symmetrized Fermi function and its transforms,” J. Phys. A: Math. Gen. 30, 6525–6534 (1997).

    Article  ADS  MathSciNet  MATH  Google Scholar 

  83. S. Klein and J. Nystrand, “Exclusive vector meson production in relativistic heavy ion collisions,” Phys. Rev. C 60, 014903 (1999); [arXiv]hep-ph/9902259.

  84. M. Cadeddu, C. Giunti, Y. F. Li, and Y. Zhang, “Average CsI neutron density distribution from COHERENT data,” [arXiv]1710.02730.

  85. E. Ciuffoli, “Measuring the neutron distribution from coherent elastic neutrino nucleus scattering,” [arXiv] 1903.12120.

  86. I. Sick, “Proton charge radius from electron scattering,” Atoms 6, 2 (2018); [arXiv]1801.01746.

  87. M. Baldo and G. F. Burgio, “The nuclear symmetry energy,” Prog. Part. Nucl. Phys. 91, 203–258 (2016); [arXiv]1606.08838.

  88. M. Cadeddu, F. Dordei, C. Giunti, Y. F. Li, and Y. Y. Zhang, “Neutrino, electroweak and nuclear physics from COHERENT elastic neutrino-nucleus scattering with refined quenching factor,” [arXiv] 1908.06045.

  89. C. Giusti and M. V. Ivanov, “Neutral current neutrino-nucleus scattering theory,” [arXiv]1908.08603.

  90. C. G. Payne, S. Bacca, G. Hagen, W. Jiang, and T. Papenbrock, “Coherent elastic neutrino-nucleus scattering on 40Ar from first principles,” [arXiv]1908.09739.

  91. R. Bernabei et al., “First model independent results from DAMA/LIBRA-Phase2,” Universe 4, 116 (2018); [arXiv]1805.10486; Nucl. Phys. At. Energy 19, 307 (2018).

    Article  ADS  Google Scholar 

  92. R. Bernabei et al., “Improved model-dependent corollary analyses after the first six annual cycles of DAMA/LIBRA-phase2,” [arXiv]1907.06405.

  93. J. Billard, L. Strigari, and E. Figueroa-Feliciano, “Implication of neutrino backgrounds on the reach of next generation dark matter direct detection experiments,” Phys. Rev. D 89, 023524 (2014); [arXiv]1307.5458.

  94. V. A. Bednyakov, “Is it possible to discover a dark matter particle with an accelerator?” Phys. Part. Nucl. 47, 711–774 (2016); [arXiv]1505.04380.

  95. A. Gutlein et al., “Impact of coherent neutrino nucleus scattering on direct dark matter searches based on CaWO4 crystals,” Astropart. Phys. 69, 44–49 (2015); [arXiv]1408.2357.

  96. A. J. Anderson, J. M. Conrad, E. Figueroa-Feliciano, K. Scholberg, and J. Spitz, “Coherent neutrino scattering in dark matter detectors,” Phys. Rev. D 84, 013008 (2011); [arXiv]1103.4894.

  97. P. deNiverville, M. Pospelov, and A. Ritz, “Light new physics in coherent neutrino-nucleus scattering experiments,” Phys. Rev. D 92, 095005 (2015); [arXiv] 1505.07805.

  98. S.-F. Ge and I. M. Shoemaker, “Constraining photon portal dark matter with Texono and Coherent data,” [arXiv]1710.10889.

  99. C. Giunti and A. Studenikin, “Neutrino electromagnetic interactions: A window to new physics,” Rev. Mod. Phys. 87, 531 (2015); [arXiv]1403.6344.

  100. K. A. Kouzakov and A. I. Studenikin, “Electromagnetic properties of massive neutrinos in low-energy elastic neutrino-electron scattering,” Phys. Rev. D 95, 055013 (2017); [arXiv]1703.00401; Erratum: Phys. Rev. D 96, 099904 (2017).

    ADS  Google Scholar 

  101. D. K. Papoulias and T. S. Kosmas, “COHERENT constraints to conventional and exotic neutrino physics,” Phys. Rev. D 97, 033003 (2018); [arXiv] 1711.09773.

  102. H. Novales-Sanchez, A. Rosado, V. Santiago-Olan, and J. J. Toscano, “Effects of physics beyond the standard model on the neutrino charge radius: An effective Lagrangian approach,” Phys. Rev. D 78, 073014 (2008); [arXiv]0805.4177.

  103. A. J. Anderson, J. M. Conrad, E. Figueroa-Feliciano, C. Ignarra, G. Karagiorgi, K. Scholberg, M. H. Shaevitz, and J. Spitz, “Measuring active-to-sterile neutrino oscillations with neutral current coherent neutrino-nucleus scattering,” Phys. Rev. D 86, 013004 (2012); [arXiv]1201.3805.

  104. O. G. Miranda, D. K. Papoulias, M. Tortola, and J. W. F. Valle, “Probing neutrino transition magnetic moments with coherent elastic neutrino-nucleus scattering,” [arXiv]1905.03750.

  105. R. E. Shrock, “Electromagnetic Properties and decays of Dirac and Majorana neutrinos in a general class of gauge theories,” Nucl. Phys. B 206, 359–379 (1982).

    Article  ADS  Google Scholar 

  106. W. Grimus, M. Maltoni, T. Schwetz, M. A. Tortola, and J. W. F. Valle, “Constraining Majorana neutrino electromagnetic properties from the LMA-MSW solution of the solar neutrino problem.” Nucl. Phys. B 648, 376–396 (2003); [arXiv]hep-ph/0208132.

  107. B. Dutta, S. Liao, S. Sinha, and L. E. Strigari, “Searching for beyond the standard model physics with COHERENT energy and timing data,” [arXiv] 1903.10666.

  108. T. S. Kosmas, O. G. Miranda, D. K. Papoulias, M. Tortola, and J. W. F. Valle, “Probing neutrino magnetic moments at the Spallation Neutron Source facility,” Phys. Rev. D 92, 013011 (2015); [arXiv] 1505.03202.

  109. T. S. Kosmas, O. G. Miranda, D. K. Papoulias, M. Tortola, Valle J. W. F. and, “Sensitivities to neutrino electromagnetic properties at the TEXONO experiment,” Phys. Lett. B 750, 459–465 (2015); [arXiv] 1506.08377.

  110. M. Tanabashi et al. (Particle Data Group Collab.), Review of Particle Physics, Phys. Rev. D 98, 030001 (2018).

    Article  ADS  Google Scholar 

  111. J. Billard, J. Johnston, and B. J. Kavanagh, “Prospects for exploring new physics in coherent elastic neutrino-nucleus scattering,” [arXiv]1805.01798.

  112. B. C. Canas, E. A. Garces, O. G. Miranda, M. Tortola, and J. W. F. Valle, “The weak mixing angle from low energy neutrino measurements: A global update,” Phys. Lett. B 761, 450–455 (2016); [arXiv]1608.02671.

  113. B. C. Canas, E. A. Garces, O. G. Miranda, and A. Parada, “Future perspectives for a weak mixing angle measurement in coherent elastic neutrino nucleus scattering experiments,” [arXiv]1806.01310.

  114. G. Arcadi, M. Lindner, J. Martins, and F. S. Queiroz, “New physics probes: Atomic parity violation, polarized electron scattering and neutrino-nucleus coherent scattering,” [arXiv]1906.04755.

  115. I. Esteban, M. C. Gonzalez-Garcia, M. Maltoni, I. Martinez-Soler, and J. Salvado, “Updated constraints on non-standard interactions from global analysis of oscillation data,” [arXiv]1805.04530.

  116. J. B. Dent, B. Dutta, S. Liao, J. L. Newstead, L. E. Strigari, and J. W. Walker, “Accelerator and reactor complementarity in coherent neutrino scattering,” [arXiv]1711.03521.

  117. M. Abdullah, J. B. Dent, B. Dutta, G. L. Kane, S. Liao, and L. E. Strigari, “Coherent elastic neutrino nucleus scattering (CEνNS) as a probe of Z′ through kinetic and mass mixing effects,” [arXiv]1803.01224.

  118. J. Liao and D. Marfatia, “COHERENT constraints on nonstandard neutrino interactions,” [arXiv] 1708.04255.

  119. D. Aristizabal Sierra, V. De Romeri, and N. Rojas, “COHERENT analysis of neutrino generalized interactions,” [arXiv]1806.07424.

  120. Y. Farzan and M. Tortola, “Neutrino oscillations and non-standard interactions,” Front. Phys. 6, 10 (2018); [arXiv]1710.09360.

  121. M. Lindner, W. Rodejohann, and X.-J. Xu, “Coherent neutrino-nucleus scattering and new neutrino interactions,” J. High Energy Phys., No. 03, 97 (2017); [arXiv] 1612.04150.

  122. Y. Farzan, M. Lindner, W. Rodejohann, and X.-J. Xu, “Probing neutrino coupling to a light scalar with coherent neutrino scattering,” J. High Energy Phys., No. 05, 66 (2018); [arXiv]1802.05171.

  123. J. Hakenmuller et al., “Neutron-induced background in the CONUS experiment,” [arXiv]1903.09269.

  124. P. B. Denton, Y. Farzan, and I. M. Shoemaker, “A plan to rule out large non-standard neutrino interactions after COHERENT data” [arXiv]1804.03660.

  125. D. Aristizabal Sierra, V. De Romeri, and N. Rojas, “CP violating effects in coherent elastic neutrino-nucleus scattering processes,” [arXiv]1906.01156.

  126. C. Giunti, “General COHERENT constraints on neutrino nonstandard interactions.” Phys. Rev. D 101, 035039 (2020); [arXiv]1909.00466.

  127. A. Parada, “Constraints on neutrino electric millicharge from experiments of elastic neutrino-electron interaction and future experimental proposals involving coherent elastic neutrino-nucleus scattering,” [arXiv]1907.04942.

  128. B. Canas, E. Garces, O. Miranda, A. Parada, and G. Sanchez Garcia, “Interplay between nonstandard and nuclear constraints in coherent elastic neutrino-nucleus scattering experiments,” Phys. Rev. D 101, 035012 (2020); [arXiv]1911.09831.

  129. B. Dutta, R. F. Lang, S Liao., S. Sinha, L. Strigari, and A. Thompson, “A global analysis strategy to resolve neutrino NSI degeneracies with scattering and oscillation data,” [arXiv]2002.03066.

  130. W.-F Chang. and J. Liao, “Constraints on light singlet fermion interactions from coherent elastic neutrino-nucleus scattering,” [arXiv]2002.10275.

  131. O. Miranda, D. Papoulias, M. Tόrtola, and J. Valle, “Probing new neutral gauge bosons with CEνNS and neutrino-electron scattering, Phys. Rev. D 101, 073005 (2020); [arXiv]2002.01482.

  132. L. Flores, N. Nath, and E. Peinado, “Non-standard neutrino interactions in U(1)′ model after COHERENT data,” [arXiv]2002.12342.

  133. M. Abdullah, D. Aristizabal Sierra, B. Dutta, and L. E. Strigari, “Coherent elastic neutrino-nucleus scattering with directional detectors,” [arXiv] 2003.11510.

  134. O. Miranda, D. Papoulias, G. S. Garcia, O. Sanders, M. Tόrtola, and J. Valle, “Implications of the first detection of coherent elastic neutrino-nucleus scattering (CEvNS) with liquid argon,” [arXiv]2003.12050.

  135. M. Cadeddu, F. Dordei, C. Giunti, Y. Li, E. Picciau, and Y. Zhang, “Physics results from the first COHERENT observation of CEνNS in argon and their combination with cesium-iodide data,” [arXiv]2005.01645.

  136. T. Li, X.-D. Ma, and M. A. Schmidt, “Generic neutrino interactions with sterile neutrinos in light of neutrino-nucleus coherent scattering and meson invisible decays,” [arXiv]2005.01543.

  137. X. Qian and J.-C. Peng, “Physics with reactor neutrinos,” Rep. Prog. Phys. 82, 036201 (2019); [arXiv] 1801.05386.

  138. J. Sinatkas, V. Tsaktsara, and O. Kosmas, “Simulated neutrino signals of low and intermediate energy neutrinos on Cd detectors,” [arXiv]1904.01056.

  139. M. F. Carneiro, “Neutrino cross sections: Status and prospects,” in Proceedings of the Prospects in Neutrino Physics, NuPhys2017 (London. UK, 2017), pp. 13–22; [arXiv]1804.03238.

  140. K. Mahn, C. Marshall, and C. Wilkinson, “Progress in measurements of 0.1–10 GeV neutrino–nucleus scattering and anticipated results from future experiments,” Ann. Rev. Nucl. Part. Sci. 68, 105–129 (2018); [arXiv]1803.08848.

  141. D. Baxter et al., “Coherent elastic neutrino-nucleus scattering at the European Spallation Source,” J. High Energy Phys., No. 02, 123 (2020); [arXiv]1911.00762.

  142. Wong H. T., Li H.-B., Li J., Yue Q., and Zhou Z.-Y., “Research program towards observation of neutrino-nucleus coherent scattering,” J. Phys. Conf. Ser. 39, 266–268 (2006); [arXiv]hep-ex/0511001.

  143. H. T. Wong, “Neutrino-nucleus coherent scattering and dark matter searches with sub-keV germanium detector,” Nucl. Phys. A 844, 229C–233C (2010).

    Article  ADS  Google Scholar 

  144. D. Yu. Akimov et al. (RED Collab.), “Prospects for observation of neutrino-nuclear neutral current coherent scattering with two-phase Xenon emission detector,” J. Instrum. 8, P10023 (2013); [arXiv]1212.1938.

  145. Akimov D. Yu. et al., “The RED-100 two-phase emission detector,” Instrum. Exp. Tech. 60, 175–181 (2017).

    Article  Google Scholar 

  146. A. V. Kopylov, I. V. Orekhov, V. V. Petukhov, and A. E. Solomatin, “Gaseous detector of ionizing radiation for registration of coherent neutrino scattering on nuclei,” Tech. Phys. Lett. 40, 185–187 (2014).

    Article  ADS  Google Scholar 

  147. J. A. Formaggio, E. Figueroa-Feliciano, and A. J. Anderson, “Sterile neutrinos, coherent scattering and oscillometry measurements with low-temperature bolometers,” Phys. Rev. D 85, 013009 (2012); [arXiv] 1107.3512.

  148. S. J. Brice et al., “A method for measuring coherent elastic neutrino-nucleus scattering at a far off-axis high-energy neutrino beam target,” Phys. Rev. D 89, 072004 (2014); [arXiv]1311.5958.

  149. G. Fernandez Moroni, J. Estrada, E. E. Paolini, G. Cancelo, J. Tiffenberg, and J. Molina, “Charge coupled devices for detection of coherent neutrino-nucleus scattering,” Phys. Rev. D 91, 072001 (2015); [arXiv]1405.5761.

  150. A. Aguilar-Arevalo et al. (CONNIE Collab.), “The CONNIE experiment,” J. Phys. Conf. Ser. 761, 012057 (2016); [arXiv]1608.01565.

  151. A. Aguilar-Arevalo et al. (CONNIE Collab.), “Exploring low-energy neutrino physics with the Coherent Neutrino Nucleus Interaction Experiment (CONNIE),” [arXiv]1906.02200.

    Google Scholar 

  152. J. Billard et al., “Coherent neutrino scattering with low temperature bolometers at Chooz reactor complex,” J. Phys. G 44,105101 (2017); [arXiv]1612.09035.

  153. R. Strauss et al., “The ν-cleus experiment: A gram-scale fiducial-volume cryogenic detector for the first detection of coherent neutrino-nucleus scattering,” Eur. Phys. J. C 77, 506 (2017); [arXiv]1704.04320.

  154. L. Oberauer, “Coherent neutrino nucleus scattering,” Prog. Part. Nucl. Phys. 48, 301–304 (2002).

    Article  ADS  Google Scholar 

  155. S. Fallows, T. Kozynets, and C. B. Krauss, “Sensitivity of the PICO-500 bubble chamber to supernova neutrinos through coherent nuclear elastic scattering,” [arXiv] 1806.01417.

  156. Agnolet G. et al. (MINER Collab.), “Background studies for the MINER coherent neutrino scattering reactor experiment,” Nucl. Instrum. Methods Phys. Res., Sect. A 853, 53–60 (2017); [arXiv]1609.02066.

  157. S. Sangiorgio, A. Bernstein, J. Coleman, M. Foxe, C. Hagmann, T. H. Joshi, I. Jovanovic, K. Kazkaz, K. Movrokoridis, and S. Pereverzev, “R&D for the observation of coherent neutrino-nucleus scatter at a nuclear reactor with a dual-phase argon ionization detector,” Phys. Procedia 37, 1266–1272 (2012).

    Article  ADS  Google Scholar 

  158. M. Lindner, “The CONUS coherent neutrino scattering experiment,” Presented at the Conference on Neutrino and Nuclear Physics, 15–20 October 2017, Catania, Italy.

  159. C. Bellenghi, D. Chiesa, L. Di Noto, M. Pallavicini, E. Previtali, and M. Vignati, “Coherent elastic nuclear scattering of 51Cr neutrinos,” [arXiv]1905.10611.

  160. Q. Arnaud et al. (EDELWEISS Collab.), “Optimizing EDELWEISS detectors for low-mass WIMP searches,” Phys. Rev. D 97, 022003 (2018); [arXiv] 1707.04308.

  161. Armengaud E. et al. (EDELWEISS Collab.), “Searching for low-mass dark matter particles with a massive Ge bolometer operated above-ground,” Phys. Rev. D 99, 082003 (2019); [arXiv]1901.03588.

  162. R. R. Lewis, “Coherent detector for low-energy neutrinos,” Phys. Rev. D 21, 663 (1980).

    Article  ADS  Google Scholar 

  163. S. Bilenky, “Introduction to the physics of massive and mixed neutrinos,” Lect. Notes Phys. 947, 1–273 (2018).

    Book  MATH  Google Scholar 

Download references

ACKNOWLEDGMENTS

The authors are grateful to Yu. Efremenko, A. Konovalov, V. Rubakov, V. Naumov, E. Yakushev, and other colleagues for important comments and discussions. V.A. Bednyakov dedicates this work to the memory of Yu.V. Gaponov who passed away in 2009 (just 10 years before this review was prepared).

Author information

Authors and Affiliations

  1. Dzhelepov Laboratory of Nuclear Problems, Joint Institute for Nuclear Research, 141980, Dubna, Russia

    V. A. Bednyakov & D. V. Naumov

Authors
  1. V. A. Bednyakov
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. D. V. Naumov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. A. Bednyakov.

Additional information

Translated by M. Potapov

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bednyakov, V.A., Naumov, D.V. Concept of Coherence in Neutrino and Antineutrino Scattering off Nuclei. Phys. Part. Nuclei 52, 39–154 (2021). https://doi.org/10.1134/S1063779620060039

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1063779620060039

Search

Navigation