Rock fines breakage by flow-induced stresses against drag: geo-energy applications

Abstract

The paper presents a strength-failure mechanism for colloidal detachment by breakage and permeability decline in reservoir rocks. The current theory for permeability decline due to colloidal detachment, including microscale mobilisation mechanisms, mathematical and laboratory modelling, and upscaling to natural reservoirs, is developed only for detrital particles with detachment that occurs against electrostatic attraction. We establish a theory for detachment of widely spread authigenic particles due to breakage of the particle-rock bonds, by integrating beam theory of particle deformation, failure criteria, and creeping flow. Explicit expressions for stress maxima in the beam yield a graphical technique to determine the failure regime. The core-scale model for fines detachment by breakage has a form of maximum retention concentration of the fines, expressing rock capacity to produce breakable fines. This closes the governing system for authigenic fines transport in rocks. Matching of the lab coreflood data by the analytical model for 1D flow exhibits two-population particle behaviour, attributed to simultaneous detachment and migration of authigenic and detrital fines. High agreement between the laboratory and modelling data for 16 corefloods validates the theory. The work is concluded by geo-energy applications to (i) clay breakage in geological faults, (ii) typical reservoir conditions for kaolinite breakage, (iii) well productivity damage due to authigenic fines migration, and (iv) feasibility of fines breakage in various geo-energy extraction technologies.

Article Highlights

• Detachment of authigenic particles from rock surface by breakage during viscous flow.

• Critical velocity equation for all cases of particle detachment by tensile and shear-stress failure.

• Feasibility of clay detachment by breakage in 12 field cases of geo-energy engineering.

1 Introduction

The geomechanics of rock failure under high stress with consequent permeability alteration is a wide and long-studied topic in geo-energy engineering. An incomplete list includes mining operation studies in gas-bearing coals (Wang et al. 2022a; Xu et al. 2022), geo-engineering applications in granites (Kumari et al. 2019), loading of lamellar continental shales (Duan et al. 2022), cyclic hydraulic fracturing for geothermal reservoir stimulation (Li et al. 2022), drainage technology of CBM fields (Xue et al. 2022), mechanical failure of hydrate‑bearing sediments (Hou et al. 2022), and wellbore stability during drilling (Wang et al. 2022b).Yet, the studies of detachment of a single reservoir fines by breakage and the migration-induced permeability decline are not available.

However, transport in porous and fractured media strongly depend on colloidal detachment, migration, straining, and consequent permeability decline (Chen et al. 2008; Teitelbaum et al. 2022; Cao et al. 2023). The permeability decrease yields well productivity and injectivity decline, while preferential permeability decline in high-conductivity layers and patterns of natural reservoirs uniformises the flux and increases sweep efficiency (Bedrikovetsky 2013). Mineral dissolution and precipitation reactions during injection of CO₂ or hydrogen into underground gas storages, cause fines mobilisation and migration; the resulting sweep enhancement leads to the storage capacity increase (Iglauer et al. 2015, 2021, 2022; Alzate-Espinosa et al. 2023). Detachment of colloidal and nano particles highly affects oil and gas production under migration of natural reservoir fines, water injection into aquifers and petroleum reservoirs, geothermal energy recovery, coal bed methane production, storage of fresh and hot water in aquifers, propagation of contaminants, bacteria and viruses, invasion of ocean into aquifers, soil erosion and construction collapse, and analysis of seismic earthquake events (Zhao et al. 2014; Fox et al. 2018; Drummond et al. 2019; Hu et al. 2021; Lehman et al. 2021; Liao et al. 2021; Lu et al. 2021; Sun and Xiang 2021; Wurgaft et al. 2021).

Typical migrating fines comprise clays, while silt, silica, and coal particles can be mobilised too (Fox et al. 2018; Farrell et al. 2021; Cao et al. 2023). Two types of natural fines are distinguished in the rocks: authigenic fines, that have been grown up on the grain surfaces during geological times and are bonded to the surface, and the detrital fines that have been carried by subterranean waters to a given reservoir point and attached to the rock surface (Wilson and Pittman 1977; Appelo and Postma 2004; Farrell et al. 2021). Detrital kaolinite is attached to the grain surface by electrostatic attraction (Fig. 1a), while the authigenic kaolinite bond the grain surface (Fig. 1b). The detachment of detrital particles against electrostatic attraction and authigenic particles by breakage, under high velocities in pore throat is presented in Fig. 1c.

Fig. 1

Detachment for detrital and authigenic clay particles: a force (torque) balance at attached detrital fine; b representation of attached authigenic particle by deformable beam (Obermayr et al. 2013); c schematic for detachment against electrostatic attraction and by breakage at the pore scale

Figures 2 and 3 present evidence of the fines breakage off the grain surfaces due to viscous flow. Figure 2 shows a number of pores before and after the flow; breakage of the matrix with liberation of some fines is indicated by the arrow 3.

Fig. 2

Evidence of fines detachment from the rock by breakage: a prior to viscous flow; b after the flow. In a and b, arrow 3 delineates breakage of a grain's structure (Wang et al. 2022d)

Fig. 3

SEM images a quartz fine (right) and kaolinite fine with carbonate cement (left) produced from Berea core sample during 60 g/L NaCl water-saturated super critical CO₂ injection (Othman et al. 2019)

Figure 3 presents SEM image of fines collected from the effluent during CO₂ flood. The image shows a kaolinite fine, likely detached by breakage of the carbonate-cement particle-grain bond, and the quartz particle detached against the electrostatic attraction.

Current mathematical and lab modelling for detachment of detrital fines is based on the mechanical equilibrium of a particle situated on the solid substrate; Fig. 1a shows drag, electrostatic, lift, and gravity forces exerting on an isolated particle. Presence of a second phase adds capillary force, which is wettability dependent (Roshan et al. 2016; Siddiqui et al. 2019). The linear-kinetics model for fines detachment assumes that detachment rate is proportional to the difference between current and equilibrium retention concentrations, with an empirical proportionality coefficient that is equal to the inverse of detachment time (Bradford et al. 2013; Johnson and Pazmino 2023). In the alternative theory, the maximum retention concentration of the attached fines, as a function of velocity, salinity, temperature, and pH, defines fines detachment (Bedrikovetsky et al. 2011, 2012). This maximum retention function (MRF) closes the system of governing equations for colloidal transport in porous media and yields several exact solutions for 1D flows (Polyanin 2002; Polyanin and Zaitsev 2012). The MRF model has been validated by extensive laboratory studies and is widely used for prediction of colloidal transport (Yuan and Shapiro 2011; Guo et al. 2016; Yuan and Moghanloo 2018, 2019; Zhai and Atefi-Monfared 2021).

Detachment of authigenic fines by breakage under flows in porous media has been observed by Turner and Steel (2016) and Wang et al. (2020) during well acidizing, by Tang et al. (2016) and Othman et al. (2018) for cement dissolution in sandstones, by Hadi et al. (2019) for carbonate rock dissolution in water, by Mishra and Ojha (2016) during sand production, and by Liu et al. (2019) for hydraulic fracturing. Yet, a geo-mechanical analysis and mathematical model for authigenic particle detachment and migration is not available.

The current paper fills the gap. A novel micro-scale model for authigenic particle detachment is derived by integration of beam theory of elastic particle deformation and strength failure with viscous flow model around the attached fines. Introduction of tensile-stress and tensile-shear diagrams allows determining the regime of particle-rock-bond failure. The breakage condition has a form of breakage velocity versus micro-scale geo-mechanical parameters, which yields maximum concentration of authigenic particles versus velocity, that closes the system of governing equations. We successfully match 16 coreflood tests under piecewise-constant increasing rate by the analytical model for colloidal flow with breakage; the data are taken from the literature. The laboratory-based analytical model for particle detachment by breakage (i) shows feasibility of authigenic fines mobilisation in geological faults, (ii) allows calculating the fraction of detachable authigenic fines in natural rocks and estimation the range of breaking velocities, (iii) permits predicting of well productivity decline, and (iv) claims that fines breakage is feasible in major geo-energy technologies.

Figure 4 illustrates the structure of the paper. Section 2 derives the microscale mechanical conditions of attached fines mobilisation by breakage. Section 3 defines maximum retention concentration (MRF) for authigenic fines as a rock-scale model for fines mobilisation by breakage and the analytical model for 1D flows. Section 4 matches the laboratory coreflood data by the analytical model and validates the breakage-detachment model. Section 5 investigates breakage of authigenic clays in geological faults. Section 6 determines the fractions of detachable fines and of authigenic particles using the analytical model. Section 7 recalculates lab results into the well-productivity data. Section 8 investigates the feasibility of fines breakage in various technological geo-energy processes. Section 9 discusses the limitations of the developed breakage-detachment model. Section 10 concludes the paper.

Fig. 4

The logic diagram of this work: a breakage criteria based on elastic beam theory; b maximum retention concentration of attached particles based on the breakage criteria; c matching the lab data by the analytical model, which is based on maximum retention function; d lab-based well impedance prediction

2 Model for beam deformation under creeping flow

Derivation of the microscale model for fines detachment by breakage during viscous flow encompasses mechanical equilibrium of attached particle (Sect. 2.1 and Appendix 1), expressions for stress maxima (Sect. 2.2 and Appendix 2), and graphical classification of the breakage regimes (Sect. 2.3).

2.1 Definition of mechanical equilibria of detaching particles

The model assumes single-phase flow of Newtonian fluid around an elastic particle of spheroidal form with circular contact area between the particle and the rock, negligible effect of particle deformation on the drag and its moment, and negligible stresses in the particle outside its stem (beam) part. The lift and gravitational forces are negligible if compared with the drag. Particles exhibit brittle behaviour with breakage. The failure criterium is the point where the tensile (shear) exceeds the strength the tensile (shear) stress.

The microscale model of a single fine detachment by breakage, presented in this section, integrates beam theory for elastic cylinder deformation (Timoshenko and Goodier 1970) with strength failure criteria for particle-rock bond (Fjaer et al. 2008; Jaeger et al. 2009) and creeping flow around the particle (Tu et al. 2018). The model assumes negligible lift exerting the particle from the flux and low beam deformations under slow Darcy’s flows in porous media. We discuss spheroidal and thin-cylinder shapes for kaolinite, chlorite, and silica fines, and long cylinders for illite fines (Appelo and Postma 2004). A circular particle-rock contact area is assumed. The rock deformation outside the beam stem is significantly lower than beam deformation; this supports the assumption that maximum stress in the contact particle–substrate area due to drag is fully determined by the deformation of the cylindric beam with the base on substrate (Obermayr et al. 2013; Wagner et al. 2016; Chen et al. 2022) (Fig. 1b). Following the lab data by Abousleiman et al. (2016), Han et al. (2019), Yang et al. (2019), Feng et al. (2020), Ren et al. (2021), brittle breakage of the particle–substrate bond is assumed.

Drag F_d and its moment M_b for oblate spheroidal and cylindric particles are extensions of the Stokes formula that is valid for spheres (Ting et al. 2021, 2022). The dimensionless shape factors are calculated from numerical solution of Navier–Stokes flow around the particle:

$$F_{d} = 6\pi \mu_{f} r_{s} Vf_{d} \left( {\alpha_{s} } \right),\quad M_{b} = F_{d} bf_{M} \left( {\alpha_{s} } \right) = 6\pi \mu_{f} r_{s} Vf_{d} \left( {\alpha_{s} } \right)bf_{M} \left( {\alpha_{s} } \right),\quad \alpha_{s} = {\raise0.7ex\hbox{$b$} \!\mathord{\left/ {\vphantom {b a}}\right.\kern-0pt} \!\lower0.7ex\hbox{$a$}}$$

(1)

where a and b are the semi-major and semi-minor of spheroid, respectively, α_s is the aspect ratio, μ_f is the fluid viscosity, r_s is the particle radius, and V is the interstitial flow velocity. We derive the empirical formulae for drag and torque factors—f_d(α_s) and f_M(α_s)—for long cylinders, given by Eqs. (20) and (21), based on numerous runs of CFD software ANSYS/CFX.

The drag and torque (1) determine an external load in Timoshenko’s solution for elastic beam, given by Eqs. (22–25). More detailed formulation of 3D elasticity problem is available from Hashemi et al. 2023a. The principal stresses σ₁, σ₂, and σ₃ are calculated as eigen values of the stress tensor (26); their maxima are determined using the Mohr circles and are determined by Eq. (27). Finally, maximum tensile (σ) and shear (τ) stresses are:

$$\max \sigma = \sigma_{3} ,\quad \max \tau = \frac{{\sigma_{1} - \sigma_{3} }}{2}$$

(2)

The expressions for tensile and shear stresses over the beam base follow from the solution of elastic beam deformation (22–25); here axi are shown in Fig. 1b:

$$\frac{{\sigma_{3} }}{{T_{0} }} = \frac{1}{\kappa }\left( {X - \sqrt {X^{2} + \chi \left( {1 - X^{2} - \frac{{\left( {1 - 2\upsilon } \right)}}{{\left( {3 + 2\upsilon } \right)}}Y^{2} } \right)^{2} + \chi \frac{{4\left( {1 + 2\upsilon } \right)^{2} }}{{\left( {3 + 2\upsilon } \right)^{2} }}\left( {XY} \right)^{2} } } \right)$$

(3)

$$\frac{{\sigma_{1} - \sigma_{3} }}{{2S_{0} }} = \frac{\eta }{\kappa }\sqrt {X^{2} + \chi \left( {1 - X^{2} - \frac{{\left( {1 - 2\upsilon } \right)}}{{\left( {3 + 2\upsilon } \right)}}Y^{2} } \right)^{2} + \chi \frac{{4\left( {1 + 2\upsilon } \right)^{2} }}{{\left( {3 + 2\upsilon } \right)^{2} }}\left( {XY} \right)^{2} }$$

(4)

where X and Y are dimensionless coordinates, T₀ and S₀ are the tensile and shear strengths, respectively, and ν is the Poisson ratio. Here the dimensionless groups κ, χ, and η reflecting the interaction between the creeping flow around an attached particle and elastic deformation inside the particle—strength-drag number κ, shape-Poisson number χ, and strength number η—are defined as

$$\frac{1}{\kappa } = \frac{{F_{d} }}{{2T_{0} }}\frac{{r_{b} bf_{M} }}{I} = \frac{{F_{d} }}{{2T_{0} }}\frac{{\delta \alpha_{s} f_{M} }}{I},\quad \chi = \left[ {\frac{{r_{b} }}{{bf_{M} }}\frac{3 + 2\upsilon }{{4\left( {1 + \upsilon } \right)}}} \right]^{2} ,\quad \eta = \frac{{T_{0} }}{{S_{0} }},\quad \delta = \frac{{r_{b} }}{a},\quad X = \frac{x}{{r_{b} }},\quad Y = \frac{y}{{r_{b} }}$$

(5)

where r_b is the bond radius, I is the moment of inertia, and x and y are dimensional coordinates.

The strength-drag number κ is proportional to the ratio between the tensile strength T₀ and the average pressure imposed by drag and incorporates the bond δ and aspect α_s ratios. The shape-Poisson number χ includes the bond δ and aspect α_s ratios along with the Poisson’s ratio υ.

Breakage of particle-rock bond is defined by the strength failure criterium, where either tensile or shear stress reaches the corresponding strength value; this maximum normalised stress becomes equal to one, while another normalised stress remains less than one upon the breakage (Jaeger et al. 2009):

$$\mathop {\max }\limits_{{X^{2} + Y^{2} \le 1}} \frac{{ - \sigma_{3} \left( {X,Y} \right)}}{{T_{0} }} \le 1,\quad \mathop {\max }\limits_{{X^{2} + Y^{2} \le 1}} \frac{{\sigma_{1} \left( {X,Y} \right) - \sigma_{3} \left( {X,Y} \right)}}{{2S_{0} }} \le 1$$

(6)

2.2 Derivation of stress maxima

Consider maxima of both tensile and shear stresses, which are given by Eqs. (3, 4). The detailed derivations are presented in Hashemi et al. (2023a). If maxima points (X_m,Y_m) are located inside the base circle, X_m² + Y_m² < 1, partial derivatives of both expressions (3, 4) over Y must be zero. It is possible to show that only along the middle of the beam base Y = 0, partial derivatives over Y are equal zero and second partial derivatives over Y are negative. Therefore, all maxima inside the base circle X_m² + Y_m² < 1 are reached along the middle of the base, i.e., axis Y = 0. Otherwise, tensile or shear stresses reaches maxima at the beam base over the boundary X_m² + Y_m² = 1.

The stresses along the beam middle and its boundary are functions of variable X alone. The profiles for tensile stress in the middle of the beam, tensile stress at the beam boundary, and shear stress at the boundary are shown in Fig. 5a, d, and g, respectively. Figure 5b, e, and h show the point X_m where maximum is reached, for those 3 cases. Figure 5c, f, and i present the maxima values.

Fig. 5

Maximum dimensionless stresses at the beam base versus shape-Poisson number χ: a maximum tensile stress along the axes Y = 0; b maximum tensile stress at the beam boundary; c maximum shear stress along the axes Y = 0; d Maximum shear stress along the beam boundary

Tensile stress T⁰(X,χ) reaches maximum in the advanced point X_m =  − 1 and then monotonically decreases for small shape-Poisson numbers. At the bifurcation value χ₁ = 3.38 the profile reaches second maximum at X_m1 =  − 0.33. For χ > χ₁, maximum point moves to the right, and maximum increases. Maximum point X_m and corresponding tensile stress maximum T⁰_m depend on shape-Poisson number alone. The maximum point and its value are calculated from the conditions of zero first derivative and negative second derivative in X:

$$T_{m}^{0} \left( \chi \right) = \frac{1}{\kappa }\left\{ {\begin{array}{*{20}l} {2,} \hfill & {\quad \chi \le \chi_{1} } \hfill \\ { - X_{m} \left( \chi \right) + \sqrt {X_{m}^{2} + \chi \left( {1 - X_{m}^{2} } \right)^{2} } ,} \hfill & {\quad \chi > \chi_{1} } \hfill \\ \end{array} } \right.;\quad X_{m} = \left\{ {\begin{array}{*{20}l} { - 1,} \hfill & {\quad \, \chi \le \chi_{1} } \hfill \\ { - \sqrt { - \frac{{\sqrt {\left( {4\chi - 1} \right)\left( {4\chi - 9} \right)} - 4\chi + 3}}{8\chi }} ,} \hfill & {\quad \chi > \chi_{1} } \hfill \\ \end{array} } \right.$$

(7)

Profile of tensile stress over the beam boundary also depends on parameter ξ alone that incorporates χ and υ:

$$T_{m}^{1} \left( {\chi ,\nu } \right) = \frac{1}{\kappa }\left\{ {\begin{array}{*{20}l} {2,} \hfill & {\quad \xi \le 2} \hfill \\ {\xi \left( {\xi - 1} \right)^{ - 0.5} ,} \hfill & {\quad \xi > 2} \hfill \\ \end{array} } \right.;\quad X_{m} = \left\{ {\begin{array}{*{20}l} { - 1,} \hfill & {\quad \xi \le 2} \hfill \\ { - \left( {\xi - 1} \right)^{ - 0.5} ,} \hfill & {\quad \xi > 2} \hfill \\ \end{array} } \right.;\quad \xi = \chi \frac{{4\left( {1 + 2\upsilon } \right)^{2} }}{{\left( {3 + 2\upsilon } \right)^{2} }}$$

(8)

The profile for T¹(X,ξ) reaches maximum at advanced point X_m =  − 1 and monotonically decreases for X >  − 1 from advanced point X_m =  − 1 for small ξ. From bifurcation value ξ = 2 on, T¹(X,ξ) loses monotonicity, maximum point X_m moves from advanced point X_m =  − 1 to the right.

At low χ, maximum for shear in the base middle is reached at advanced and receded points. This occurs until bifurcation value χ = 1, where X_m jumps into the origin X_m = 0. For χ > 1, the maximum point for shear stress in the base middle remains in origin and monotonically increases:

$$S_{m}^{0} \left( \chi \right) = \frac{\eta }{\kappa }\left\{ {\begin{array}{*{20}l} {1,} \hfill & {\quad \chi < 1} \hfill \\ {\sqrt \chi ,} \hfill & {\quad \chi > 1} \hfill \\ \end{array} } \right.;\quad X_{m} = \left\{ {\begin{array}{*{20}l} { \pm 1,} \hfill & {\quad \chi < 1} \hfill \\ {0,} \hfill & {\quad \chi > 1} \hfill \\ \end{array} } \right.;\quad \eta = \frac{{T_{0} }}{{S_{0} }}$$

(9)

Maximum of shear over the boundary is lower than the shear maximum in the base middle for all values of shape-Poisson numbers and Poisson’s ratio—S¹_m(χ,υ) < S⁰_m(χ,υ)—and is not considered to fulfil failure criteria.

Equations (7–9) show that stress maxima along the axis Y = 0 are determined by aspect-Poisson number χ alone, while the maxima at the beam boundary are determined by both aspect-Poisson number χ and Poisson’s ratio υ.

2.3 Classification of breakage regimes

Depending on shape-Poisson number and Poisson’s ratio, either of three stresses (7), (8), or (9) can exceed the other two and fulfil the strength failure criteria (6). Let us first define the largest from the two tensile stress maxima, (7) or (8). Their equality T⁰_m(χ,υ) = T¹_m(χ,υ) divides plane (χ,υ), which further in the text we call tensile stress diagram, into 5 regions. Black curve corresponds to ξ = 2. Blue and red curves are calculated from Eqs. (7) and (8) for ξ > 2:

$$\upsilon_{m} \left( \chi \right) = \frac{{3\sqrt {\left( {\kappa T_{m}^{0} \left( \chi \right)} \right)^{2} \pm \kappa T_{m}^{0} \left( \chi \right)\sqrt {\left( {\kappa T_{m}^{0} \left( \chi \right)} \right)^{2} - 4} } - \sqrt {8\chi } }}{{4\sqrt {2\chi } - 2\sqrt {\left( {\kappa T_{m}^{0} \left( \chi \right)} \right)^{2} \pm \kappa T_{m}^{0} \left( \chi \right)\sqrt {\left( {\kappa T_{m}^{0} \left( \chi \right)} \right)^{2} - 4} } }}$$

(10)

and correspond to positive and negative values of the root in Eq. (10). Along the red curve, inequality ξ > 2 holds, while domain V is located below black curve, so the red curve must be ignored.

The black curve, the vertical straight line χ = χ₁, and the blue curve divide (χ,υ) plane into 5 domains, depending on superiority of either of the two tensile stress maxima (Fig. 6a). The three lines cross in one point (χ = χ₁,υ = υ₁) where υ₁ = 0.125 is obtained from Eq. (10).

Fig. 6

Classification of bond breakage regimes by tensile and shear stresses: a tensile stress diagram; b shear-tensile diagram

Now let us determine whether shear S⁰_m exceeds maximum of two tensile stresses. Define the breakage regime function

$$g\left( {\chi ,\nu } \right) = \frac{{T_{m} \left( {\chi ,\upsilon } \right)}}{{S^{0}_{m} \left( \chi \right)}} > \frac{{T_{0} }}{{S_{0} }} = \eta ;\quad T_{m} \left( {\chi ,\upsilon } \right) = \max \left\{ {T^{0}_{m} \left( \chi \right),\quad T^{1}_{m} \left( {\chi ,\upsilon } \right)} \right\}$$

(11)

At breakage, maximum normalised stress is equal one. Therefore, as it follows from Eq. (6), the breakage occurs due to tensile stress if g(χ,υ) > η. Otherwise, the breakage occurs due to shear stress. Further in the text, (χ,η)-plane with the curve η = g(χ,υ) is called the shear-tensile diagram (Fig. 6b).

For all χ and υ values, breakage regime function exceeds one and does not exceed two. Depending on stress η and shape-Poisson numbers, and Poisson’s ratio, the breakage curve exhibits 4 cases presented in plane (χ,η):

• I. For strength ratios exceeding 2, particles are detached by shear stress for all values of χ and υ;

• II. For strength ratios below two and above one, the particles are detached by shear stress for shape-Poisson number χ that does not exceed the value determined by g(χ,υ) = η;

• III. For strength ratios below two and above one, the particles are detached by tensile stress for shape-Poisson number χ that exceeds the value determined by g(χ,υ) = η;

• IV. For strength ratios lower than one, particles are detached by tensile stress for all values of χ and υ.

So, either of the 5 domains in tensile-stress diagram determines maximum tensile stress, and then either of the 4 cases in tensile-shear diagram determines which stress causes the breakage. For either of three stress cases (7), (8), or (9), the normalised stress is equal one, allowing calculating stress-drag number κ. For the cases of domination of normalised tensile stress in the middle, tensile stress on the boundary, and shear stress in the middle, the formulae for strength-drag numbers κ are:

$$\kappa = \left\{ {\begin{array}{*{20}l} {2,} \hfill & {\quad \chi \le \chi_{1} } \hfill \\ {\sqrt { - \frac{{\sqrt {\left( {4\chi - 1} \right)\left( {4\chi - 9} \right)} - 4\chi + 3}}{8\chi }} + \sqrt {\left( {\sqrt { - \frac{{\sqrt {\left( {4\chi - 1} \right)\left( {4\chi - 9} \right)} - 4\chi + 3}}{8\chi }} } \right)^{2} + \chi \left( {1 - \left( {\sqrt { - \frac{{\sqrt {\left( {4\chi - 1} \right)\left( {4\chi - 9} \right)} - 4\chi + 3}}{8\chi }} } \right)^{2} } \right)^{2} } } \hfill & {\quad \chi > \chi_{1} } \hfill \\ \end{array} } \right.$$

(12)

$$\kappa = \left\{ {\begin{array}{*{20}l} {2,} \hfill & {\quad \xi \le 2} \hfill \\ {\xi \left( {\xi - 1} \right)^{ - 0.5} ,} \hfill & {\quad \xi > 2} \hfill \\ \end{array} } \right.;\quad \xi = \chi \frac{{4\left( {1 + 2\upsilon } \right)^{2} }}{{\left( {3 + 2\upsilon } \right)^{2} }}$$

(13)

$$\kappa = \frac{{T_{0} }}{{S_{0} }}\left\{ {\begin{array}{*{20}l} {1,} \hfill & {\quad \chi < 1} \hfill \\ {\sqrt \chi ,} \hfill & {\quad \chi > 1} \hfill \\ \end{array} } \right.,$$

(14)

3 Macroscale model for fines migration with detachment by breakage

This section defines rock-scale model for detachment of authigenic fines by breakage (Sect. 3.1) and its implementation into transport equations for the authigenic particles (Sect. 3.2).

3.1 Maximum retention function as a rock-scale detachment model

Substitution of drag from Eq. (1) into the expression for strength-drag number (5) allows for exact expression for the breakage velocity:

$$U_{cr}^{b} = \frac{{\delta \phi T_{0} r_{b}^{2} }}{{12\alpha_{s} f_{M} f_{d} \mu_{f} r_{s} }}\kappa^{ - 1}$$

(15)

where κ is given by either of Eqs. (12–14). Consider the manifold of the particles attached to rock surface. The particles are stochastically distributed by fluid velocity around them near to asperous rock surface in pores of different forms and sizes, aspect, Poisson’s, and bond ratios, sizes, strength, and bond radii. However, Eq. (15) determines the critical breakage velocity for each particle, defining whether the particle remains attached or breaks off the rock at a given flow velocity U. The concentration of the particles attached to rock at a given velocity is called the breakage maximum retention function (MRF) σ^b_cr(U). MRF can be obtained by upscaling of Eq. (15) accounting for probabilistic distributions of coefficients δ, r_b, α_s, and r_s.

The maximum retention function for detachment against electrostatic DLVO attraction for detrital particles is determined by the torque balance between drag and electrostatic DLVO forces (Bradford et al. 2013):

$$M_{b} = 6\pi \mu_{f} r_{s} Vf_{d} \left( {\alpha_{s} } \right)bf_{M} \left( {\alpha_{s} } \right) = F_{e} \left( h \right)l_{n} ,\quad F_{d} = 6\pi \mu_{f} r_{s} Vf_{d} \left( {\alpha_{s} } \right) = \nu_{C} F_{e} \left( h \right),$$

(16)

where h is the distance between the particle and substrate and ν_c is the Coulomb friction coefficient.

Figure 7 presents the energy profile for the DLVO forces (Israelachvili 2015). Figure 7a shows the potential for coal fines and substrate with one energy minimum, while Fig. 7b shows two minima of the energy profile. During favourable attachment, the particle moves to the left from zero energy state to a single primary minimum. During unfavourable attachment, the particle moves to the left from zero energy to shallow secondary energy minimum and needs to overcome the energy barrier to get into primary energy minimum (Fig. 7b).

Fig. 7

DLVO energy profiles: a coal fines and coal substrate; b kaolinite fines and silica substrate

Assuming that authigenic and detrital fines detach independently, the overall MRF is the total of individual ones:

$$\sigma_{cr} \left( U \right) = \sigma_{cr}^{e} \left( U \right) + \sigma_{cr}^{b} \left( U \right),\quad \Delta \sigma \left( {U_{n} } \right) = \sigma_{cr} \left( {U_{n - 1} } \right) - \sigma_{cr} \left( {U_{n} } \right)$$

(17)

The total MRF defines the mobilised concentration by velocity increase from U_n−1 to U_n. After mobilisation, the detached fines migration is described by system of population balance accounting for particle capture by the rock. MRF defines initial concentration of detached particles after abrupt change of flow rate.

3.2 Macroscale analytical model for colloidal transport in porous media

1D problems for fines migration with any arbitrary particle capture (filtration) function λ(σ_s) and suspension function f(c) allow for exact solutions (Polyanin and Manzhirov 2006; Polyanin and Zaitsev 2012). In the case of continuous rate increase, MRF determines the sources term in mass balance, closing the governing system for colloidal-suspension transport (fines migration) in porous media (Bedrikovetsky et al. 2019). Here, for the sake of simplicity, we discuss the model with constant filtration coefficient λ = const and f(c) = c, in order to treat the limited literature information on the corefloods with piecewise-constant increasing velocity.

Appendix 3 presents system of governing equations, which consists of the mass balance of suspended and strained particles (30), straining rate (31), and Darcy’s law accounting for permeability decline due to particle straining (32). For the case the corefloods with piecewise-constant increasing velocity U₀ = 0, U₁, U₂…, fines detachment occurs at moments of switching from velocity U_n-1 to U_n, n = 1, 2, 3…, which is expressed by initial condition (33). The exact solution for 1D flow problem is expressed by Eqs. (35) and (36). The exact solution allows for explicit expression of impedance (38). Next section uses Eqs. (35) and (38) to treat the lab data on breakthrough concentration c(1,t) and dimensionless pressure drop (impedance) J(t) measured during the multi-rate corefloods.

4 Laboratory study and model validation

Huang et al. (2017) used core sample from a coal seam reservoir located in the southern part of the Qinshui Basin (China) for coreflooding. The laboratory study was comprised of water injection with 2% (weight percent) of KCl into the anthracite coal core with permeability 21 mD and porosity 0.08. Core length was 5.16 cm. Figure 8 shows lab data during application of eight injection rates. The breakthrough concentration and pressure drop across the core, have been measured during the overall test. Figure 8a shows breakthrough concentration data and their matching by the model. Figure 8b presents the history of impedance.

Fig. 8

Matching lab data on authigenic and detrital fines migration in coal cores: a accumulated breakthrough concentrations for 8 flow rates; b impedance; c detached particle concentration under each of 8 rates; d approximation of detached density function by the total of two log-normal distributions; e maximum retention functions (MRFs) for detrital and authigenic fines, and the total MRF

Figure 8a shows exponential growth of the accumulated breakthrough concentrations, which corresponds to exponential decrease of the momentum breakthrough concentrations c_n(x = 1,t). This behaviour is typical for deep bed filtration of low-concentration colloids with constant filtration coefficient (Chen et al. 2008; You et al. 2015).

The mathematical model (30–34) contains four dimensionless parameters—the drift delay factor, α, the formation damage factor, β, the filtration coefficient, λ, and the detached concentration during each stage, Δσ(U_n)—which must be tuned for each flow rate U_n, n = 1, 2 … 8. Table 1 presents the tuning results. Figure 8c shows the tuned and measured values of detached particle concentrations. The probabilistic density of velocity distribution of the detached fines are presented in Fig. 8d. The PDF is bimodal, which is attributed to fines detachment by breakage and against electrostatic forces, where the particles are authigenic and detrital, respectively. The PDF allows the approximation by the total of two log-normal distributions with high accuracy. Figure 8e shows two individual MRFs and their total, which is MRF for the overall colloid. Eight experimental points for the total detached concentrations match well with the accumulated MRF curve.

Table 1 The tuning result of matching accumulated concentration and impedance

Tensile strength T₀ along with the bond radius r_b can be calculated from Eq. (15) and the histogram for detachment velocity (Fig. 8d). Assume the typical value of coefficient of variation of the bond radius as C_v = 0.03 (Ting et al. 2022). Equation (15) can be used for mean detachment velocity, which is taken from the breakage-velocity histogram in Fig. 8d—U^b_mean = 1.21 × 10⁻⁴ m/s. Minimum value of detachment velocity—U^b_min = 0.63 × 10⁻⁴ m/s—corresponds to minimum value of bond radius, which can be estimated as r_b(1–3C_vr_b). Applying Eq. (15) to mean and minimum breakage velocities allows calculating two unknowns T₀ = 0.03 MPa and r_b = 3.0 × 10⁻⁷ m. Stability of calculations of tensile strength and bond radius from mean and minimum breakage velocities is determined by high difference in their values—U^b_mean is 1.92 times higher than U^b_min.

Torkzaban et al. (2015) conducted lab tests on consolidated (sandstone) core sample from the Yarragadee Formation (Perth Basin, Western Australia). The laboratory study was comprised of water injection with concentrations 47 mg/L of sodium chlorite and 9 mg/L of calcium chlorite into the sandstone core with permeability 2697 mD and porosity 0.32. Core length was 7 cm. Figure 9 shows lab data during application of six injection rates. Table 2 presents the results of tuning the model coefficients. The breakthrough concentration has been measured during the overall test. The data on pressure drop across the core are not available from the original paper.

Fig. 9

Matching lab data on authigenic and detrital fines migration in sandstone cores: a accumulated breakthrough concentrations for 6 flow rates; b detached particle concentration under each of 6 rates; c approximation of detached density function by the total of two log-normal distributions; d maximum retention functions (MRFs) for detrital and authigenic fines, and the total MRF

Table 2 The tuning result of matching accumulated concentration

Figure 9 shows lab data and their matching by the model (30–34) during application of six injection rates: breakthrough concentration data (Fig. 9a), detached concentrations at each rate (Fig. 9b), density distributions for detachment velocity for authigenic and detrital fines (Fig. 9c), and individual MRFs for authigenic and detrital fines along with overall MRF.

Like in the previous test, the detachment velocity distribution has a clear bimodal structure, which also supports the two-population hypothesis.

Table 2 presents the tuning results for dimensionless parameters—α, β, λ, and Δσ(U_k)—which have been determined for each flow rate U_k, k = 1, 2 … 8.

Applying Eq. (15) for mean and minimum detachment velocities, which are taken from the histogram in Fig. 9c—U^b_mean = 2.75 × 10⁻³ m/s and U^b_min = 1.22 × 10⁻³ m/s, respectively—we obtain T₀ = 0.56 MPa and r_b = 1.35 × 10⁻⁷ m.

For both tests, the tuned parameters vary within common intervals, earlier presented in the literature (Chen et al. 2008; Bradford et al. 2013; You et al. 2015; Guo et al. 2016). The obtained tensile strength for kaolinite is also typical (Han et al. 2019; Yang et al. 2019). The detached concentrations Δσ_cr(U) versus velocity for both kinds of particles increases at small rates from zero and declines at high velocities, which complies with typical form of the maximum retention curve (Bedrikovetsky et al. 2011, 2012). High values of the coefficient of determination show high match between the experimental and modelling data, which validates the model.

Close match by single-population model has been achieved for lab data of 16 corefloods with piecewise-constant increasing rates (Table 5). The tuned parameters have the same order of magnitude as those presented in Tables 1 and 2.

5 Breakage of authigenic clays in geological faults

Mobilization and migration of authigenic clays cause the permeability decrease in geological faults and faulted zones, which is important for sealing capacities of CO₂ and hydrogen storage, and for interpretation of various seismic events (Farrell et al. 2021). Let us discuss whether authigenic clay detachment by breakage due to viscous water flux under fault conditions is feasible.

Table 3 presents water velocities in faults in different basins as reported in papers the referred papers. Papers by Matthäi and Roberts (1996), Liu et al. (2018), and Yu et al. (2020) took the velocity values from the basin data to use in simulation, via paper by Eichhubl and Boles (2000) retrieved the velocity directly from tracer test, and paper by Maloszewski et al. (1999) inferred it by the size of entrained rock fragments. Maloszewski et al. (1999) present the probability distribution function (PDF) for velocity detachment by breakage and the velocities of water in faults as presented all 5 papers. The data for calculations are presented in Table 4 and taken from publications Ting et al. (2021) and Farrell et al. (2021). Ting et al. (2021) shows that particle radius, aspect ratio, bond radius and their variation coefficients, presented in Table 4, are the most influential parameters on PDF.

Table 3 Flow velocities in faults in different basins

Table 4 Particle parameters to calculate PDF for breakage velocity

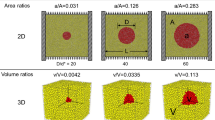
Figure 10 shows that breakage of kaolinite in the case 1 almost does not occur; some authigenic fines are broken in the case 2. Significant part of authigenic fines is broken in the case 3. In the cases 4 and 5, all authigenic particles are detached by breakage.

Fig. 10

Probability distribution function (PDF) for breakage velocity and flow velocities in the geological faults

6 Determining the fractions of detachable clays and authigenic fines in rocks

Tuning the model coefficients by matching the lab coreflood data by the analytical model for 1D deep bed filtration with constant filtration coefficient λ, given by Eqs. (34–38) allows calculating the fraction of detachable fines with respect to initial clay content in the rock (column 9 in Table 5), fraction of detached fines produced during corefloods (column 10), and fraction of authigenic fines in the overall detachable fines (11th column). Table 5 presents the results of 16 coreflood data matching. Tests 1–3 have been performed by Ochi and Vernoux (1998), test 4—by Torkzaban et al. (2015), tests 5 and 6—by Shang et al. (2008), tests 7, 8—by Huang et al. (2017), tests 9, 10—by Huang et al. (2018), test 11—by Guo et al. (2016), tests 12–14—by Huang et al. (2021), tests 15, 16—by Hashemi et al. (2022, 2023a).

Table 5 The fraction of detachable kaolinite rocks, fraction of detached fines produced during corefloods, and fraction of authigenic fines in the produced particle concentration

The fraction of detachable fines in consolidated rocks and high-salinity water injection varies from 0.01 to 0.19% (Lines 1–3, 6–11, 15, and 16), which agrees with the previously published data (Russell et al. 2017). This percentage is so low due to small fraction of clay particles located at the rock surface, where they are accessible to water flux; vast majority of clays are located inside the rock skeleton and matrix. In grinded rocks and high-salinity water injection, the fraction increases up to 19–75% (Lines 12–14) due to high accessibility of grain surfaces to the water flux in porous space. In high porosity sandstone and packed sediment, the fraction increases to 18 and 90% under low-salinity and deionized water injection (Lines 4 and 5, respectively) due to disappearance of DLVO particle-rock attraction at low salinities.

The fraction of authigenic fines of the overall concentration of detached fines varies from 0.36 to 0.83. For those rocks, the ratio between the maximum rate at the coreflood and minimum breakage velocity exceeds one, indicating fines b) breakage (11th column in Table 5). Authigenic particles haven’t been observed in packed sediments and in artificial packed sediment cores (Lines 5, 6 and 9, 10, respectively). In other 12 cases, the minimum breakage velocity is below the maximum velocity applied in the corresponding test, so authigenic fines have been observed in the production.

The breakage velocity of authigenic fines is widely distributed—11th column of Table 5 shows that the ratio of maximum and minimum breakage velocities varies from 1.2 to 4.5, i.e., the calculation method for tensile strength T₀ and bond radius r_b using Eq. (15) is stable.

The analytical model for 1D fines migration with constant particle capture (filtration) coefficient λ, given by Eq. (35) for c(x,t), allows calculating the ratio between the stabilised accumulated concentration of produced fines and the detached overall detached concentration (8th column in Table 5):

$$\frac{{\phi c_{acc} }}{{\sigma_{0} }} = \frac{{1 - \exp \left( { - \lambda L} \right)}}{\lambda L},$$

(18)

so the ratio depends on the dimensionless filtration coefficient λL only, where L is the system length. Plot of curve (18) and the points from 6th column are placed in Fig. 11a. The curve and the 16-test data show the clear tendency that the higher is the filtration coefficient the faster is the particle capture and the lower fraction of the mobilised fines is produced. The fraction varies from one for zero filtration coefficient, to zero where the filtration coefficient tends to infinity. Some point scattering and mismatch with the curve is explained by heterogeneous colloid, including varying particle properties and different forms and capture probabilities for detrital and authigenic fines.

Fig. 11

Effects of particle capture by the rock on fines migration: a fraction of produced fines in the detached concentration versus dimensionless filtration coefficient; b impedance (reciprocal to normalised average permeability) versus filtration coefficient

The stabilised impedance is also calculated from the analytical model (35–38) for

$$J\left( {t \to \infty } \right) = J_{st} = 1 + \beta \Delta \sigma \left[ {1 - \frac{{1 - e^{ - \lambda L} }}{\lambda L}} \right]$$

(19)

Besides the dimensionless filtration coefficient, the stabilised impedance (19) depends on formation damage coefficient β and the detached fines concentration Δσ, which explained the scattering of the points in Fig. 11b. The curve J_st(λL) increases from 1 at zero filtration coefficient to 1 + βΔσ where filtration coefficient tends to infinity. Figure 11b presents three curves corresponds to tuning of three lab cases; the curves correspond to points with the same colour.

The above plots show how the number of produced fines and permeability decline vary with filtration coefficient—increase of filtration coefficient yields decrease of produced fines and growth of permeability damage. Several papers claim insignificant fines migration based on the data of low produced concentrations. Yet, low produced concentration could be due to high filtration coefficient, so fines migration must be indicated by the impedance increase along with the number of produced fines.

Mineral dissolution chemical reactions weaken the rocks, decreasing strength in Eq. (15) and resulting in additional fines liberation in situ the reservoir yielding the additional sweep enhancement. Those increase the fraction of authigenic fines. The dissolution reactions make CO₂ and hydrogen storages susceptible to fines breakage.

7 Effects of colloidal breakage detachment on reservoir and well behaviour

1D radial problem for fines detachment and flow toward well allows for exact solution under constant production rate (You et al. 2015, 2019). Detached fines straining results in permeability decline and increase of the pressure drop between the well and the reservoir. Treatment of 16 coreflood tests, presented in Table 5, yields calculation of filtration and formation damage coefficients along with maximum retention functions for detachment against electrostatic forces and by breakage, like it was performed in Sect. 4. Implementing these values into the solution for fines migration in radial flow permits the estimation of wellbore impedance as well as the relative impacts of authigenic and detrital fines on well injectivity. For the parameter values of case 16 in Table 5 the critical retention function and impedance are presented in Fig. 12a and b respectively. The red curves in this plot correspond to the experimental conditions of the test by Hashemi et al. (2023a) (salinity, γ = 0.6 M, viscosity, μ_f = 1 cp, and temperature T = 25 °C). The critical retention function shows a clear distinction between the detachment of detrital particles at low velocity and detachment of authigenic particles for higher velocities. For this σ_cr(U) curve, the corresponding impedance in Fig. 12b is close to one, indicating negligible formation damage. This is a result of the high velocities required for particle detachment.

Fig. 12

Formation damage during injection of water under different conditions: a critical retention functions, b impedance

The conditions of the experimental test are not indicative of those in most field injection scenarios. Due to explicit calculation of the critical retention function based on physical considerations, the effect of changing environmental conditions can be examined by changing relevant parameters. In this way the results of experiments can be extended beyond the conditions they were performed under. Here we consider three different scenarios covering a range of applications.

The three cases considered are low salinity (corresponding to freshwater recharge wells), high viscosity (corresponding to the injection of fracturing fluid), and high temperature (corresponding to geothermal or deep petroleum wells). Changing salinity to 0.01 M decreases the velocities required to detach detrital particles but has no effect on authigenic particles, as illustrated by the critical retention function in Fig. 12a. Increasing viscosity increases drag and lift, increasing detachment of all particles. Lastly increasing temperature mostly affects detrital particles, as it decreases the electrostatic force, but it also results in a decrease in the viscosity, slightly affecting authigenic particles. All three cases result in larger values of impedance as shown in Fig. 12b. This demonstrates the importance of fines migration across a range of applications.

Changes of these system parameters clearly do not affect authigenic and detrital detachment uniformly. Thus, we consider the relative importance of each kind of detachment under the three reservoir conditions. Impedance curves showing the predicted impedance if particles detached only by DLVO (detrital) or breakage (authigenic) are shown in Fig. 13. At low salinity, breakage is negligible, and all formation damage occurs due to detrital particles which are weakly held to the rock’s surface at low γ. For high viscosity, both detrital and authigenic particles contribute to formation damage, with more than half of the damage caused by breakage. Lastly, Fig. 13c shows that the formation damage at high temperatures results almost entirely from the weakening of electrostatic forces.

Fig. 13

Impact on injectivity of detachment via two mechanisms: DLVO (detrital particles) and breakage (authigenic particles) under different conditions, a low salinity water injection, b injection of high viscosity fluid, c injection under high temperatures

Geological site selection for CO₂ and hydrogen storage highly depends on well performance and storage capacity. Authigenic fines breakage along with detrital particle detachment can cause significant permeability reduction with detrimental well productivity and injectivity decline, but to storage capacity increase. High velocity in highly permeable layers and patterns yields higher fines detachment and permeability reduction, resulting in homogenisation of injectivity and productivity profiles and, finally, in enhanced sweep (Bedrikovetsky 2013). In the case of CO₂ injection, sweep increase leads to enhancement of the pore volume where capillary, stratigraphic and chemical CO₂ capture occurs, increasing the storage capacity. In the case of cyclic hydrogen injection and production, sweep enhancement results in increase of water-free hydrogen production.

The competitive effects of well index decline and sweep enhancement with CO₂ and hydrogen storage are in odds with each other: the higher is the injection rate, the higher is the sweep and storage capacity, but the higher is the well index decrease. The optimal injection and production rates can be determined using the mathematical modelling that includes Eqs. (12–15).

8 Breakage of authigenic fines during well exploitation

In this section we investigate whether fines breakage can occur in the vicinity of production and injection wells for different reservoir conditions. Table 6 presents the data for heavy oil production (Ado 2021), polymer injection (Gao 2021), dewatering of coal bed methane (CBM) reservoir (Shi et al. 2008), injection of supercritical CO₂ into carbonate reservoir (Spivak et al. 1989), hydraulic fracturing using water as a fracturing fluid (HFW) (Prasetio et al. 2021), hydraulic fracturing using high-viscosity fracturing fluid (HF) (Prasetio et al. 2021), natural gas production (NG) (Peischl et al. 2015), water production from geothermal reservoirs (GWP) (Ishido et al. 1992), water injection into aquifers (WI) (De Lino 2005), and water production from aquifers (WP) at low and high rates (Kulakov and Berdnikov 2020). The data for calculations are taken from those literature sources. For all cases, spherical particle shape (α_s = 1) corresponds to kaolinite booklet; typical fine size is taken as r_s = 2 μm.

Table 6 Occurrence of fines breakage in well vicinity

The breakage velocity is calculated using Eqs. (12–15) and is given in nineths column. The well rates are taken from the corresponding papers. Column nine shows the velocity on well walls for well radius r_w = 0.1 m.

The velocity on the well wall exceeds minimum breakage velocity for heavy oil production, polymer injection, both cases of hydraulic fracturing, production of natural gas and geothermal water, water injection into aquifers and water production from artesian well with high rate, so fines breakage can occur under those conditions. The velocity on well wall is lower than minimum breakage velocity for dewatering in CBM, CO₂ injection, and water production with low rates.

Similar effects of well injectivity and productivity as well as in situ bond strength decline by chemical reactions occur during geothermal exploitation, where fines detachment occurs due to DLVO forces decrease at high temperature, and in fractured reservoirs that are highly susceptible to fines breakage due to high flow velocity (Altree-Williams et al. 2019; Wang et al. 2022c). During water and CO₂ injection in carbonate reservoirs, where rock dissolution yields massive release of various size particles, the effects of particle mobilisation by breakage is expected to be significantly more pronounced.

9 Discussions

The current version of the breakage-detachment model is limited to single-phase flows. Adding the capillary force exerting by menisci on the attached fines into torque balance (16) would cover the detachment by breakage during gas flow in shale rocks and CO₂ and hydrogen storage (Roshan et al. 2016; Siddiqui et al. 2019). The appropriate two-phase flow model accounts for moving interface (Shapiro 2015, 2018).

The primary limitation of the model is brittle behaviour of the particle–substrate bond during breakage; the study of ductile bonds would complicate the failure criteria (6) and phase diagrams. Accounting for non-elasticity of the rock and non-Newtonian fluid, the rheology yields in more complex expressions for stress maxima than (12–15); in this case the breakage regime will be velocity-dependent.

The model developed in this paper is limited to single-population colloidal transport, given by Eqs. (30–32) with further separation of MRF into those by authigenic and detrital fines, while Hashemi et al. (2023a) apply two-population balance model with two different filtration functions for authigenic and detrital populations. A more general approach would encompass multicomponent colloidal transport with non-linear fines straining (Bedrikovetsky et al. 2019).

The analytical model for well inflow performance under fines migration using the model constants obtained from 12 corefloods, where the authigenic fines mobilisation have been observed (Table 5), yields well index decrease up to 1.4 times. We expect significantly higher effects based on more representative corefloods. Besides, all 16 tests have been performed in sandstones. Significantly higher formation damage is expected during waterflooding or CO₂ injection in carbonates, where rock dissolution yields reduction of particle-rock bond radius with consequent bond breakage and massive fines release.

Numerous geomechanics studies determine the strength and other failure parameters of the rocks, while the bond-breakage criteria in Eq. (12–15) contain those for a single particle and substrate. Those measurements require significantly more sophisticated equipment (Su et al. 2022; Roshan et al. 2023). Currently, those parameters for mineral particles are unavailable. Derivation of the fine-breakage model in Sect. 2 may stimulate those experimental studies.

The breakage-detachment models (11–15) and (17, 33) correspond to particle and rock scales, respectively. The current work does not include a method for calculating maximum retention function from torque balance, and vice versa—calculation of mechanical equilibrium coefficients of the attached particles from the MRF. A recent work by Hashemi et al. (2023b) presents a stochastic model for the mechanical equilibrium of detrital particles, where the equilibrium conditions are given by Eq. (16). Averaging yields the MRF for detrital fines. Performing similar theoretical development for authigenic fines accounting for mechanical equilibrium given by Eqs. (12–15) would results in an expression for maximum retention function for fines detachment by breakage.

10 Conclusions

The model derivations for particle detachment by breakage, integrating the Timoshenko’s beam theory with CFD flow modelling and strength criteria, and applying the model to different geo-energy topics allow concluding as follows.

Maximum stresses are reached either at the beam base middle Y = 0 or at its boundary. Breakage conditions, where either of tensile or shear stresses reaches the strength value, are determined by three dimensionless parameters: strength-drag number κ, aspect-Poisson number χ, and strength ratio η. The tensile-stress diagram in plane (χ,υ) determines which of tensile stresses is higher. The shear-stress diagram in plane (χ,η) determines 4 breakage regimes depending on aspect-Poisson number χ, Poisson’s ratio υ, and strength ratios η.

The definition of breakage regime—by either tensile or shear stress—is independent of flow velocity. For an identified breakage regime, breakage velocity is determined by the strength-drag number κ(χ,υ) alone. For a given particle shape, the critical breakage velocity is proportional to strength and particle size, and it is inversely proportional to viscosity.

The expression for critical breakage velocity allows determining the breakage maximum retention function MRF, which is a mathematical model for particle detachment by breakage of particle-rock surface bond. MRF closes the governing system for colloidal transport with breakage detachment.

The lab-based analytical model for fines breakage shows that under strong subterranean water fluxes, the authigenic fines mobilisation by breakage can occur in geological faults, resulting in permeability decline and affecting sealing capacities during CO₂ and hydrogen injection for storage.

Matching of 16 coreflood tests exhibits high agreement between the laboratory and modelling data. Besides, the model coefficient values as obtained by tuning, belong to their common intervals of variation. This validates the developed model for migration of authigenic and detrital fines in rocks.

The matching allows determining the detachable fines fraction in the overall clay content, and the authigenic fines fraction in the detachable fines. The detachable fines fraction in consolidated rocks and high-salinity water injection varies from 0.01 to 0.19%. It increases up to 19–90% in grinded rocks or by deionised water injection. The authigenic fines fraction of the detachable clay particles varies in the interval 0.4–0.8.

In 12 corefloods from 16, where authigenic fines have been found, the breakthrough curves during corefloods exhibit two-population behaviour, which is attributed to commingled production of the authigenic and detrital particles. Besides, size distributions of produced fines are bimodal.

Analytical 1D model for axi symmetric flow allows recalculating coreflood tests with migration of authigenic and detrital fines into the well productivity curve, permitting estimating the formation damage due to fines migration.

Calculations of minimum breakage velocities/well rates using Eq. (15) for typical values of the breakage-model parameters show high feasibility of rock fines breakage during heavy oil production, polymer injection, both cases of hydraulic fracturing, production of natural gas and geothermal water, water injection into aquifers and water production from artesian well with high rate.

Appendices

Appendix 1: CFD calculations for drag and its torque

The formulae for drag and torque factors in Eq. (1) for spheroidal and thin cylindrical particles are available from Ting et al. (2021). In this work, those factors for long thin cylinders, which model illite clay particles, are calculated for α_s > 1:

$$f_{d} = \left( {0.9014\alpha_{s}^{2} + 1.599\alpha_{s} + 2.265} \right)\left( {\alpha_{s} + 1.752} \right)^{ - 1}$$

(20)

$$f_{M} = \left( {0.0002161\alpha_{s}^{3} + 1.34\alpha_{s}^{2} + 44.18\alpha_{s} + 21.27} \right)\left( {\alpha_{s}^{2} + 31.34\alpha_{s} + 10.38} \right)^{ - 1}$$

(21)

Appendix 2: Stresses in elastic beam by Timoshenko’s model

Stress distributions in cylindric elastic beam (Timoshenko and Goodier 1970) are

$$\sigma_{z} = \frac{{F_{d} bf_{M} x}}{I}$$

(22)

$$\tau_{xz} = \frac{{\left( {3 + 2\upsilon } \right)}}{{8\left( {1 + \upsilon } \right)}}\frac{{F_{d} }}{I}\left( {r_{b}^{2} - x^{2} - \frac{{\left( {1 - 2\upsilon } \right)}}{{\left( {3 + 2\upsilon } \right)}}y^{2} } \right)$$

(23)

$$\tau_{yz} = - \frac{{\left( {1 + 2\upsilon } \right)}}{{4\left( {1 + \upsilon } \right)}}\frac{{F_{d} xy}}{I}$$

(24)

$$\sigma_{x} = \sigma_{y} = \tau_{xy} = 0$$

(25)

The stress tensor, as per solution (12–15) is:

$$\left[ {\begin{array}{*{20}c} 0 & 0 & {\tau_{xz} } \\ 0 & 0 & {\tau_{yz} } \\ {\tau_{xz} } & {\tau_{yz} } & {\sigma_{z} } \\ \end{array} } \right]$$

(26)

The principal stresses are eigen values of the stress tensor (26):

$$\sigma_{1} = \frac{{\sigma_{z} + \sqrt {\sigma_{z}^{2} + 4\left( {\tau_{xz}^{2} + \tau_{yz}^{2} } \right)} }}{2},\quad \sigma_{2} = 0,\quad \sigma_{3} = \frac{{\sigma_{z} - \sqrt {\sigma_{z}^{2} + 4\left( {\tau_{xz}^{2} + \tau_{yz}^{2} } \right)} }}{2}$$

(27)

where, σ₁, σ₂, σ₃ are principal stresses in order of decreasing of their values, and

$$\sigma_{1} > \sigma_{2} = 0 > \sigma_{3} .$$

(28)

The equation for largest Mohr circle for the case of σ₂ = 0 is

$$\left[ {\sigma - \left( {\frac{{\sigma_{1} + \sigma_{3} }}{2}} \right)} \right]^{2} + \tau^{2} = \left[ {\frac{{\left( {\sigma_{1} - \sigma_{3} } \right)}}{2}} \right]^{2}$$

(29)

where σ and τ are tensile and shear stresses acting on unitary planes with different orientations.

Appendix 3: Population balance model for colloidal-suspension transport in porous media

We discuss deep bed filtration of the total particle population for detrital and authigenic fines. The state variables are the volumetric concentrations of suspended and strained particle, c and σ_s, respectively, and the pore pressure p. Mass balance and capture rate equations and Darcy’s law for the colloidal flux are:

$$\frac{\partial }{\partial t}\left( {\phi c + \sigma_{s} } \right) + \frac{\partial }{\partial x}\left( {c\alpha U_{n} } \right) = 0$$

(30)

$$\frac{{\partial \sigma_{s} }}{\partial t} = \lambda c\alpha U_{n} ,\quad n = 0,1,2 \ldots$$

(31)

$$U_{n} = - \frac{{k_{0} }}{{\left( {1 + \beta \sigma_{s} } \right)\mu_{f} }}\frac{\partial p}{{\partial x}}$$

(32)

where ϕ is the porosity, λ is the constant filtration coefficient, α is the drift-delay factor, U_n is the flow velocity at n-th injection, k₀ is the initial undamaged permeability, p is the pressure, and β is the formation damage coefficient. Index n is attributed to injection velocity, and U₀ = 0. The filtration coefficient for the low-concentration fines population is constant.

Initial suspended concentration is equal to concentrations of mobilised fines after velocity increase. It is posed at each moment ϕL/αU_n−1 of the velocity switch from U_n−1 to U_n

$$t = t_{n} {:}\quad c_{n0} = \frac{{\sigma_{cr} \left( {U_{n - 1} } \right) - \sigma_{cr} \left( {U_{n} } \right)}}{\phi } = \frac{{\Delta \sigma_{cr} \left( {U_{n} } \right)}}{\phi }$$

(33)

Here the overall MRF is a total of two individuals MRFs by Eq. (17).

Inlet boundary condition corresponds to injection of particle-free water

$$x = 0,\quad t_{n} < t < t_{n + 1} {:}\,c_{n} = 0$$

(34)

The exact solution of the problem (30–34) can be found in handbooks Polyanin and Manzhirov (2006), or Polyanin and Zaitsev (2012). Breakthrough concentration is obtained by the method of characteristics:

$$c_{n} \left( {x,t} \right) = \left\{ {\begin{array}{*{20}l} {\frac{{\Delta \sigma_{cr} \left( {U_{n} } \right)}}{\phi }\exp \left( { - \frac{{\lambda \alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \right),} \hfill & {\quad x > \frac{{\alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \hfill \\ {0,} \hfill & {\quad x < \frac{{\alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \hfill \\ \end{array} } \right.$$

(35)

Breakthrough concentration becomes zero at the moment of the concentration front arrival at the moment ϕL/αU_n. At this moment, all concentrations and pressure drop stabilise. Yet, even where the switch times t_n+1-t_n are lower than the arrival times, we neglect suspension concentration which was formed before the switch.

Strained concentration is obtained integration of Eq. (31) in t accounting for expression (35):

$$\sigma_{s,n} \left( {x,t} \right) = \left\{ {\begin{array}{*{20}l} {\sigma_{s,n - 1} \left( {x,t_{n} } \right) + \Delta \sigma_{cr} \left( {U_{n} } \right)\left( {1 - \exp \left( { - \frac{{\lambda \alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \right)} \right),} \hfill & {\quad x > \frac{{\alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \hfill \\ {\sigma_{s,n - 1} \left( {x,t_{n} } \right) + \Delta \sigma_{cr} \left( {U_{n} } \right)\left( {1 - \exp \left( { - \lambda x} \right)} \right),} \hfill & {\quad x < \frac{{\alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \hfill \\ \end{array} } \right.$$

(36)

The dimensionless pressure drop (impedance) J is defined as:

$$J\left( t \right) = \frac{\Delta p\left( t \right)}{{\Delta p\left( 0 \right)}}\frac{U\left( 0 \right)}{{U\left( t \right)}}$$

(37)

The expression for impedance is derived from Eq. (32) by integrating pressure gradient in x from x = 0 to core length x = L:

$$\begin{aligned} J_{n} \left( t \right) & = \left\{ {\begin{array}{*{20}l} {J_{n - 1} \left( {t_{n} } \right) + \beta \Delta \sigma_{cr} \left( {U_{n} } \right)I_{n} \left( t \right),} \hfill & {\quad t - t_{n} < \frac{L\phi }{{\alpha U_{n} }}} \hfill \\ {J_{n - 1} \left( {t_{n} } \right) + \beta \Delta \sigma_{cr} \left( {U_{n} } \right)\left( {\left( {1 + \frac{1}{\lambda L}\exp \left( { - \lambda L} \right) - \frac{1}{\lambda L}} \right)} \right),} \hfill & {\quad t - t_{n} > \frac{L\phi }{{\alpha U_{n} }}} \hfill \\ \end{array} } \right. \\ I_{n} \left( t \right) & = \frac{{\alpha U_{n} }}{\phi L}\left( {t - t_{n} } \right) + \frac{1}{\lambda L}\exp \left( { - \lambda \frac{{\alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \right) \\ & \quad - \frac{1}{\lambda L} + \left( {1 - \frac{{\alpha U_{n} }}{\phi L}\left( {t - t_{n} } \right)} \right)\left[ {1 - \exp \left( { - \frac{{\lambda \alpha U_{n} }}{\phi }\left( {t - t_{n} } \right)} \right)} \right] \\ \end{aligned}$$

(38)