Abstract
Water coning is a complex phenomenon observed in conventional and unconventional reservoirs. This phenomenon takes place due to the imbalance between viscous and gravitational forces during simultaneous production of oil and water. In a fractured reservoir, controlling of water coning is challenging due to the complexity originates from large number of uncertain variables associated with such reservoirs system. This paper presents a fully coupled poroelastic multiphase fluid-flow numerical model to provide a new insight and understanding of water coning phenomenon in naturally fractured reservoir under effect of various rock and fluid properties. These properties include anisotropy ratio, fracture permeability, mobility ratio, and production rate. The simulation workflow of the developed numerical model is based on upstream flux weighted finite element discretization method and a new hybrid methodology, which combines single-continuum and discrete fracture approach. Moreover, the capillary pressure effect is included during the discretization of the partial differential equations of multiphase fluid flow. The numerical system is decoupled using implicit pressure and explicit saturation (IMPES) approach. Discretization of water saturation equation using standard finite element method produces solution with spatial oscillations due to its hyperbolic nature. To overcome this, Galerkin’s least square technique (GLS) is employed to stabilize the equation solutions. The developed numerical scheme is validated successfully against Eclipse-100 and then applied to a case study of fractured reservoir taken from Southern Vietnam. The results showed that the break through time is very sensitive to the distributions of fracture network, anisotropy ratio between fracture horizontal, vertical permeability, and mobility ratio. Furthermore, it has been concluded that aquifer strength has a little effect on coning behavior during oil production process.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
Oil production from wells existing in reservoirs supported with a strong aquifer leads to changing of pressure drawdown around the wellbore and causing the movement of oil/water interface toward the producing interval. The fluid interface deforms from its initial shape into a cone shape and that is why this phenomenon is referred to coning (see Fig. 1). There are three essential forces controlling the mechanism of water coning which include capillary, gravity, and viscous forces. At initial reservoir conditions, the gravity force is dominant and once the wells start to flow the pressure drawdown increases and viscous force arises to be part of the controlling mechanisms (Hoyland et al. 1989). The oil/water interface moves up until viscous force is balanced with the gravitational force at a certain elevation. This balance can be achieved at a certain production flow rate and if such balance never occurred, the cone will be dragged up until invading the wellbore.
As a result of invading the wellbore by water cone, excessive water will be produced and kills the producing well or limiting its economic life (Beattie and Roberts 1996). The shape and the nature of the cone in conventional reservoirs depend on numerous factors such as completion interval, production rate, anisotropy ratio, and mobility ratio (Inikori 2002). While, in naturally fractured reservoirs, the problem becomes more complicated due to the existence of two interacting porous media (fracture and matrix) which results in the formation of two cones. The first cone is the fastest and developed in fractures, while the slowest one is developed in the matrix porous media. The relative position of the two cones is rate sensitive and is a function of rock properties (Namani et al. 2007). If the well flows above the critical rate, the viscous force dominates and the cone will break into the wellbore. Therefore, the production flow rate plays a key role in limiting coning effects.
Hoyland et al. (1989) presented an analytical solution for critical flow rate calculations. The presented correlation is validated against numerical solutions. The authors concluded that the critical flow rate is independent on shape of water/oil relative permeability curve between endpoints, water viscosity, and wellbore radius, but it has a strong dependency on completion interval and permeability ratio k v /k h .
Al-Afaleg and Ershaghi (1993) used an empirical correlation for homogenous reservoirs to calculate the critical production rate and breakthrough time for naturally fractured reservoirs. Al Afaleg stated that no correlation or even simulation study has the capability to predict the estimation of critical production rate and breakthrough time if the fracture network is not accurately distributed.
Saad et al. (1995) performed an experimental work to assess the problem of water coning in naturally fractured reservoirs. The outcome of their experimental work was that the main factor influencing the breakthrough time is the difference in viscosities between oil and water phase. Furthermore, they stated that capillary forces may be neglected if the distance between oil water contact and fluid entry is sufficiently large compared to capillary rise.
Bahrami et al. (2004) assessed the coning phenomenon using actual water and gas coning data from Iranian natural fractured reservoirs. By analyzing the results, it was concluded that the ratio k v /k h is the main factor controlling coning occurrence. Bahrami et al. (2004) presented a developed method suitable for calculation of breakthrough time and water cut at each specific oil production rate. They mentioned that the water invades the wellbore through fracture network and the breakthrough time is strongly dependant on fracture porosity. In addition, their study proved that the breakthrough time is very sensitive to horizontal and vertical fracture permeability. Perez-Martinez et al. (2012) evaluated the occurrence of coning in naturally fractured reservoirs using fine coning radial grid with one-meter thick layers concentric around the well and 2-inch thick layers in the annulus, with and without cement. They found that water coning takes place in fractured porous medium with permeability up to 10 Darcy’s in both good and poorly cemented wells. Moreover, they developed a new correlation to determine maximum height of water coning, the breakthrough time, and well shut in time to reverse water cone.
Most of the previous studies simplify simulation of coning phenomenon by assuming that the dominant forces is only viscous force and capillary forces are therefore neglected. In the current study, the capillary forces are included during the derivation of the multiphase flow equations. The fluid production or injection alters the pressure state in fractured reservoirs that causes rock deformation and leads to generation of seismic activities. Moreover, the rock deformation leads to the change of porosity and permeability, which affects fluid flow, oil recovery, and coning phenomenon. To simulate these physical processes accurately, coupled effects need to be considered during simulation of mechanical and fluid-flow responses. For the above reasons, this study presents a new poroelastic numerical model to evaluate coning phenomenon in naturally fractured reservoirs. The model is for two-phase fluid flow through matrix and fractured medium. Furthermore, the model used a new hybrid methodology for fluid-flow simulation. In hybrid methodology, a threshold value for fracture length is defined. Fractures, which are smaller than the threshold value, are used to generate the grid-based permeability tensor. The reservoir domain is divided into a number of grid blocks, and the fluid-flow simulation is carried out using the single-continuum approach in the nominated blocks. Fractures, which are longer than the threshold value, are explicitly discretized in the domain using tetrahedral elements and the fluid-flow is modeled using the discrete fracture approach.
The model is validated against Eclipse-100 using horizontal fractures. In addition, the current paper presented a real case study of fractured reservoir taken from Southern Vietnam to evaluate parameters that affect water coning phenomenon.
Eclipse-100 is used as a dual porosity/permeability approach to simulate fluid flow in naturally fractured reservoirs. The dual porosity/permeability approach has a lot of limitations which include (1) the fluid distribution within the matrix blocks remains constant during the simulation period, (2) the model cannot be applied to disconnected and discrete fractured (oriented fractures) media and a small number of large scale fractures can be considered for flow simulation. Therefore, the developed numerical model that is presented in this paper overcomes all the abovementioned limitations by account flow through matrix porous media and discrete fractures by taking into account all fracture properties which include fracture orientation, radius, and location.
Methodology
Three-dimensional flow equations used for permeability tensor calculations are given by Darcy’s law and continuity equations as follows:
where v is the fluid velocity vector, \(\vec{\vec{k}}\) is the permeability tensor, and ∇p is the local pressure gradient. The grid-based permeability tensors for short to medium fractures are calculated using finite element technique. Each fracture is represented as a sandwiched element (triangular element) between three-dimensional tetrahedral elements representing the matrix porous media as shown in Fig. 2. Single-phase fluid-flow governing equation through matrix porous medium can be written as follows:
where \(\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\rightharpoonup}$}} {k} }\) is a full permeability tensor, \(\mu\) is the fluid viscosity, Q H is fluid source/sink term represents the fluid exchange between matrix and fracture, or fluid extraction (injection) from the wellbore.
Single-phase fluid-flow governing equation through discrete fractures can be expressed as follows:
where \(q^{ + } {\text{and }}q^{ - }\) are the leakage fluxes across the boundary interfaces, \(\overline{\nabla }\) is the divergence operator in local coordinates system (Watanabe et al. 2010), and P f is the pressure inside the fracture. The permeability of the fracture can be expressed by a parallel plate concept (cubic law) as shown in Eq. (6). It has been assumed that the fracture surfaces are parallel and the fluid-flow through a single discrete fracture is laminar (Snow 1969).
where b is the fracture aperture, and k f is the fracture permeability.
Finite element method formulation
The weighted residual method is used to derive the weak formulation of the governing equation of fluid flow through a fractured system. Standard Galerkin method is applied to discretize the weak forms with appropriate boundary conditions (Zimmerman and Bodvarsson 1996; Woodbury and Zhang 2001).
Equations (3) and (5) are written separately for matrix porous medium and discrete fractures in finite element formulation. The matrix is discretized using 3D tetrahedral elements and fractures are discretized using 2D triangle elements. If FEQ represents the flow equation, the integral form can be written as follows:
where \(\bar{\varOmega }_{f}\) represents the fracture part of the domain as a 2D entity, Ω m represents matrix domain, and Ω is the entire domain. 2D integral fracture equation is multiplied by the fracture aperture b for consistency of the integral form.The formulation of the governing equation for hydraulic process through matrix porous medium can be written as follows:
Introducing weak formulation, Eq. (8) is expanded as follows:
where [w = w(x, y, z)] is a trial function. The weak formulation for discrete fractures of Eq. ( 9 ) is expanded as follows:
Galerkin finite element method
Three basic steps in finite element approximation are (1) domain discretization by finite elements, (2) discretization of integral (weak) formulation, and (3) shape functions generation of field variables unknowns. Galerkin finite element method is used to discretize the integral weak forms of Eqs. (9) and (10). Unknown variables are approximated using appropriate shape functions.
where N p is the pressure shape function and \(\bar{p}\) is the vector of nodal values of the unknown variable. Finite element formulation of the governing equation for hydraulic process for matrix and fracture system can be written in a matrix form as follows:
where m is referring to matrix porous medium and f to fracture network.
Boundary conditions
Pressure and flux boundary conditions that have been presented by Durlofsky (1991) are used for calculations of permeability tensor in fractured porous medium. These conditions are listed in Eqs. (16)–(21) and Fig. 3.
where n is the outward normal vector at the boundaries, ∂D is the grid block face, and G is the pressure gradient. Specifying a zero pressure gradient along the y-, z- faces (\(\frac{\partial p}{\partial y} = 0\), and \(\frac{\partial p}{\partial z} = 0\)) and solving Eq. ( 12 ) with using the abovementioned periodic boundary conditions, the average velocity along x-, y-, and z- directions can be calculated as follows:
Equation (1) can be expressed in an explicit form as follows:
where v x , v y , and v z are velocities in x, y, and z directions, respectively. Since pressure gradient through x direction is assumed and v x , v y , and v z are known, then k xx , k xy , and k xz are easily determined. The rest of permeability tensor elements can be calculated using the same boundary conditions as abovementioned but in other directions.
Governing equations for multiphase poroelastic numerical model
In general, behavior of two-phase fluid-flow system through fractures network and matrix porous medium is controlled by generalized Darcy’s law and continuity equation of fluid flow for each fluid phase.
The Darcy’s law:
General continuity equation for wetting phase incorporating the concept of effective stress can be expressed as follows:
General continuity equation for non-wetting phase can be expressed as follows:
where ϕ is the porosity of the media, s π is the saturation for each phase, u π sis the relative velocity vector between fluid phase and solid phase, k ij is the permeability tensor, k rπ is the relative permeability for each fluid phase π, μ π , ρ π , and p π are dynamic viscosity, density of fluid, and fluid pressure for each phase, respectively, g i is the gravity acceleration vector, β π is the fluid formation volume factor, K m is the bulk modulus of solid grain, D is the elastic stiffness matrix, and Q π represents external sources or sinks.
Saturation equations
Oil saturation equation:
Water saturation equation:
where s i and v i are the saturation and velocity for phase i, respectively.
The discretization of these equations has been implemented using standard finite element method; also the partial differential equation used for calculating the saturation changes in fractures and matrix was discretized using Galerkin’s least square technique (GLS) to stabilize the equation solutions. To obtain the numerical solution of this highly nonlinear equations system, suitable initialization and boundary conditions should be designated at first, then some of auxiliary functions are employed which are known as the constitutive relationships.
Auxiliary equations
Saturation equation
The porous medium voids are assumed to be filled with water and oil and the sum of their saturation is unity:
The phase’s pressures in porous media are related though the capillary pressure equation as follows:
where p cow is the capillary pressure between oil and water phase. The relative permeability coefficient of wetting and non-wetting phase depends on the degree of saturation and can be calculated either experimentally or using various relationships that have been proposed by different authors as shown in Table 1.
s e is the effective water phase saturation, and it equals to
where s wr is the residual water saturation, S wmax is the maximum water saturation, λ is the pore size distribution index, and γ is an empirical constant to fit this relationship with the experimental data.
Finite element discretization
Finite element method has been used to discretize two-phase fluid-flow governing equations in a poroelastic environment. where p c is the capillary pressure, K w is the water bulk modulus, and K o is the oil bulk modulus. If FEQ represents the flow Eq. (36), the integral form for two-phase fluid flow through matrix and fracture can be written as follows:
where \(\bar{\varOmega }_{f}\) represents the fracture part of the domain as a 2D entity, Ω m represents matrix domain, and Ω is the entire domain. The following flow chart explains how the developed numerical model works to simulate two-phase fluid flow in a poroelastic environment (see Fig. 4).
Validation of the developed numerical model
The performance of the developed 3D poroelastic numerical model is evaluated against Eclipse-100. A simple radial reservoir model consisting of 15 horizontal layers was built, eight layers represent the matrix porous medium, and the rest represents the fractures as shown in Fig. 5. Figure 6 shows the 3D generated mesh with the same physical dimensions of the reservoir model that has been created using Eclipse 100. A production well with radius of 0.2 ft is placed at the center of the model with producing interval in layer number 7 and 8. The rock and fluid properties used in this simulation model are extracted from Eclipse data files and presented in Tables 2 and 3. The same reservoir model has been studied with the developed poroelastic numerical model in this paper as well to prove its capability of modeling various real-field applications. The relative permeability curves used during the simulation process are presented in Figs. 7 and 8.
Simulation work
A base case run was performed under constant bottomhole pressure as a constraint to compare between Eclipse and developed numerical model results. Figure 9 shows oil production rate with time, while water cut at the production well is shown in Fig. 10.
As can be seen form Figs. 9 and 10, the oil production rate and water cut at the producer resulted from Eclipse-100 and the developed numerical simulation model is in agreement. Oil rate decreases with time, due to the increase of water rate production as a result of moving oil–water contact toward the producing interval. The model was simulated without the effect of stresses because the main aim was to validate the numerical model to be used in real-field applications. All fractures that used in this model are horizontal fractures where Eclipse 100 can handle.
Water flooding test in arbitrarily oriented fracture system
In example (1), water flooding is simulated in a single fracture (see Fig. 11a) and results of water saturation profile are presented in Fig. 11b. The reservoir dimensions are 10 m × 10 m × 4 m in x, y, and z directions, respectively. The aperture of the fracture is assumed to be 0.15 mm and the matrix permeability is 2 md. Initial reservoir pressure is set at 3600 psi, one producer with constant bottomhole pressure set at 1500 psi, and the water injection rate is kept at 250 bbls/day. In example (2) same reservoir data are used, but the number of fractures is increased from one to three (see Fig. 12a). In Fig. 12b, the water saturation profile after 1 h of water injection is presented. It can be seen form Figs. 11b and 12b that injected water displaced oil from the fractures first and then the matrix porous media.
Case study
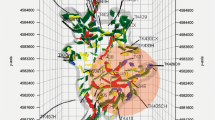
The test case is taken from granitic oil-bearing formation in Southern offshore Vietnam. The formation is highly fractured and most of the fractures have short length identified from image logs and form the storage capacity of the reservoir. Geological interpretation showed that the reservoir has very low matrix porosity; permeability and pore spaces in the rock were formed through the fractures network and digenetic processes. The authors used an innovative methodology to generate the 3D subsurface fracture map of the studied reservoir by integrating field data, such as wellbore images and conventional well logs. In this approach, an object-based conditional global optimization technique is used to generate the subsurface fracture map of the reservoir which combines statistical analysis of different sources of data as mentioned above (see Fig. 13a).
Generation of discrete fracture map of a typical basement reservoir
In this paper, object-based simulation technique is used to generate subsurface discrete fracture maps (Gholizadeh and Rahman 2012). In this model, fractures are treated as objects and placed in the domain stochastically. The number of generated fractures is controlled by fracture intensity and fractal dimension parameters. The fractures are treated as objects with varying radii, dips, and azimuth angles.
Study of water coning phenomenon
The coning phenomenon is studied using the generated fractured map (see Fig. 13a, b) under effect of different rock and fluid properties. Therefore, the model is initialized by applying vertical and horizontal stresses on x-, y-, and z- directions as shown in Fig. 13b. Because of the lack of information about far field stresses, the horizontal stresses are estimated based on overburden stress (Zoback 2010).
The fracture aperture is initialized using a value of 0.04 mm and its changes due to stresses effect are calculated using shear slippage criteria similar to Chen et al. (2000). Chen et al.’s (2000) criteria was based on the concept that when the pressure inside fracture becomes higher than the given in situ stress, the fracture opens and its surfaces no longer remain in contact. The oil–water contact is introduced to the model at depth = 250 m with 100 % water saturation and 0 % of oil saturation. Fluid and rock properties used in the simulation model are provided in Table 4. Relative permeability curves used for simulate fluid flow through matrix porous media and discrete fractures are presented in Fig. 14.
The permeability tensors for short fractures with length smaller than 100 m are calculated using the method that has been described in the first part of the current paper. Fracture with length longer than 100 m is discretized explicitly in the domain using the triangular elements. The reservoir parameters that used for the sensitivity analysis are (1) anisotropy ratio k v /k h , (2) fracture permeability, and (3) oil production rate.
Anisotropy ratio k v /k h
First simulation runs are to evaluate the coning under effect of different anisotropy ratios k v /k h as shown in Table 5.
Figure 15 shows the effect of anisotropy ratio on water cut. The result indicates that the water cut increases as a result of increasing the anisotropy ratio value. The physical explanation behind that is the increase of vertical permeability will enhance the coning into the fractured network due to the pressure drawdown around the wellbore.
Mobility ratio
Four mobility ratios are selected to perform the sensitivity analysis as shown in Fig. 17. As expected at high mobility ratio (µ = 10), the breakthrough time occurs very fast (5.5 days). On the other hand, when mobility ratio becomes very small (µ = 0.5), the breakthrough time occurs after 200 days of oil production.
Aquifer strength
One strong aquifer and weak aquifer are tested in the fractured reservoir model. Simulation results in Fig. 18 show that the aquifer characteristics have no effect on the breakthrough time and the water cut after breakthrough.
Conclusion
A 3D multiphase poroelastic numerical model is developed to assess the water coning phenomenon in naturally fractured reservoirs. The developed numerical model has the ability to simulate large numbers of discrete fracture in the reservoir domain. Several parameters have been used to assess their effects and contribution to the water coning phenomenon in naturally fractured reservoirs. These parameters are anisotropy ratio, mobility ratio, oil production, and the aquifer strength.
The conclusion that can be drawn from the simulation study are as follows:.
-
Increase in the vertical permeability [i.e., increase in anisotropy ratio (k v /k h )] leads to increase of water cut and water saturation at the producing interval without any significant effect on the oil production rate from the fractured reservoir.
-
Decrease of oil production rate leads to decrease of produced water cut. The physics explanation is to the fact that at low rate, water cut is controlled by fast moving cone inside the fractures.
-
Aquifer strength has a little effect on produced water cut.
-
Investigation of the effective parameters is necessary to understand the mechanism of water coning in naturally fractured reservoirs. Simulation of this phenomenon helps to optimize the conditions in which the breakthrough time of water cone is delayed.
References
Al-Afaleg NI, Ershaghi I (1993) Coning phenomena in naturally fractured reservoirs. Society of Petroleum Engineers Paper, SPE 26083
Bahrami H, Shadizadeh SR, Goodarzniya I (2004) Numerical simulation of coning phenomena in naturally fractured reservoirs. Paper presented at the 9th Iranian Chemical of Engineering Congress (IchEC9), Iran University of Science and Technology (IUST), 23–25 November
Beattie DR, Roberts BE (1996) Water coning in naturally fractured gas reservoirs. Society of Petroleum Engineers Paper, SPE 35643
Brooks RH, Corey AT (1964) Hydraulic properties of porous media. Colorado State University Hydrology Paper 3. State University, Fort Collins, CO
Chen Z, Narayan SP, Yang Z, Rahman SS (2000) An experimental investigation of hydraulic behaviour of fractures and joints in granitic rock. Int J Rock Mech Min Sci 37:1061–1071
Corey AT (1954) Interrelation of gas and oil relative permeabilities. Prod Mon 19(l):38–41
Durlofsky LJ (1991) Numerical calculation of equivalent grid block permeability tensors for heterogeneous porous media. Water resour res 27(5):699–708
Farag SM, Le HV, Mas C, Maizeret P-D, Li B, Dang T (2009) Advances in granitic basement reservoir evaluation. Society of Petroleum Engineers. doi:10.2118/123455-MS
Gholizadeh DN, Rahman SS (2012) 3D hybrid tectono-stochastic modeling of naturally fractured reservoir: Application of finite element method and stochastic simulation technique. Tectonophysics 541–543(0):43–56
Hoyland AL, Papatzacos P, Skjæveland MS (1989) Critical Rate for water coning: correlation and analytical solution. Society of Petroleum Engineers Paper, SPE 15855
Inikori SO (2002) Numerical study of water coning control with downhole water sink (DWS) well completions in vertical and horizontal wells. The Department of Petroleum Engineering, Louisiana State University. Doctor of Philosophy, p 238
Namani M, Asadollahi M, Haghighi M (2007) Investigation of water coning phenomenon in Iranian carbonate fractured reservoirs. Society of Petroleum
Perez-Martinez E, Rodriguez-de la Garza F, Samaniego-Verduzco F (2012) Water coning in naturally fractured carbonate heavy oil reservoir—a simulation study. Society of Petroleum Engineers Paper, SPE 152545
Saad SM, Darwich TD, Asaad Y (1995) Water coning in fractured basement reservoirs. Society of Petroleum Engineers Paper, SPE 29808
Snow DT (1969) Anisotropie permeability of fractured media. Water Resour Res 5(6):1273–1289
Van Genuchten MTH (1980) A closed form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci Soc Am J 44(5):892–898
Watanabe N, Wang W, McDermott CI, Taniguchi T, Kolditz O (2010) Uncertainty analysis of thermo-hydro-mechanical coupled processes in heterogeneous porous media. Comput Mech 45(4):263–280
Woodbury A, Zhang K (2001) Lanczos method for the solution of groundwater flow in discretely fractured porous media. Adv Water Resour 24(6):621–630
Zimmerman RW, Bodvarsson GS (1996) Hydraulic conductivity of rock fractures. Transp porous media 23(1):1–30
Zoback MD (2010) Reservoir geomechanics. Cambridge University Press, Cambridge
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Abdel Azim, R. Evaluation of water coning phenomenon in naturally fractured oil reservoirs. J Petrol Explor Prod Technol 6, 279–291 (2016). https://doi.org/10.1007/s13202-015-0185-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s13202-015-0185-7