1 Introduction

Surface-subsurface filtration transport phenomena of coastal aquifers with seawater intrusion, connected to surface reservoirs are formulated and analyzed as coupled multimedia of macro-hybrid mixed pairs, modeled by variational evolution free boundary interface problems.

The innovative modeling aspect of the paper is to treat variational incompressible Darcian subsurface flows coupled to Stokesian surface flows, in the context of open coastal aquifers. Specifically it deals with local internal boundary synchronizing transmissions of spatial nonoverlapping multidomain decompositions, as well as proper coupling interface flow-transport mechanical local transmissions, via dual Lagrangian fields solutions to corresponding subpotential maximal monotone subdifferential equations (Alduncin 2022).

Accordingly, internal boundary synchronizing transmission constraints due to multidomain spatial nonoverlapping macrohybrid decompositions, are modeled by variational Lagrangian dual subpotential maximal monotone inclusions (Alduncin 2007b). On the other hand, mass flux-velocitiy-pressure Lagrange multipliers of filtration transport coupling interface transmission constraints, are also treated as solutions of dual subpotential subdifferental equations.

As mixed flow models, incompressible surface mixed velocity-stress Stokesian contaminant flows are considered coupled with subsurface mixed velocity-pressure fractional Darcian flows. In particular, two-phase air-fresh water above the sea level in conjunction with fresh water-seawater two phase characterizations are utilized, in the context of immobile air-seawater phases.

Regarding, the corresponding surface-subsurface filtration transport coupling interface transmissions, variational mass flux primal-dual traction and dual-primal velocity constraints are once again systematically modeled by Lagrange multipliers as solutions of dual subpotential subdifferental equations.

The flow models of this study correspond to the incompressible fractional two-phase subsurface Darcian model of Chen and Ewing (1999), Chen (2001), coupled to an evolution Stokesian surface incompressible flow (Gurtin et al. 2010). Assuming the fresh water velocity as the wetting phase velocity, the subsurface flow model results to be an stationary total velocity-global pressure incompressible flow, coupled with an evolutionary wetting velocity-complementary pressure compressible-like flow.

In relation with evolution coastal filtration models, we refer at a first instance to Alduncin (2013), Esquivel-Avila and Alduncin (1990). In Alduncin (2013) we have considered this evolution filtration problem as an slightly compressible Darcian velocity-pressure mixed single phase flow, which corresponds to the classical variational pressure model treated in Esquivel-Avila and Alduncin (1990). Such coastal models are in fact variational extensions to general three-dimensional aquifers with seawater intrusion, of original filtration problems based on the pioneer stationary Baiochi’s transform models (Baiocchi et al. 1973), treated further as evolutionary free boundary problems in DiBenedetto and Friedman (1986), Torelli (1975), Torelli (1977), Friedman and Torelli (1977), Gilardi (1979).

Here, our interest is to apply the special two-phase approach to evolution coastal filtration problems that we have recently considered (Alduncin 2015), taking into account its hydraulic interaction with evolution Stokesian surface reservoirs, as free boundary interface multidomain macro-hybrid mixed variational problems.

Importantly, such a mixed two-phase flow modeling is appropriate for the application of composition duality methods (Alduncin 2005, 2007b, 2014), in the solvability analysis of the systems via duality principles and fixed-point characterizations. For parallel computing purposes, suitable spatial decompositions based on nonoverlapping multidomains, below the sea level as well as on the surface reservoirs, are variationally introduced with internal boundary trace fields dualized as weak interior transmission macro-hybrid constraints (Alduncin 2007a, 2008, 2011). Further, we should emphasize that these decomposed reformulations lead to macro-hybrid mixed localized models, which are very appropriate for internal functional variational finite dimensional approximations, implementable in terms of non-matching finite element discretizations with resolution parallel iterative algorithms (Alduncin 2003, 2009).

Some works on seawater intrusion in coastal aquifers to be mentioned are Mohammed et al. (2019), Prusty and Farooq (2020), Dibaj et al. (2021), Chala et al. (2022): Specifically paper (Mohammed et al. 2019) presents a review of available hydraulic and physical management strategies, for the reduction of seawater intrusion in coastal aquifers; a review of processes to control seawater intrusion is provided by study (Prusty and Farooq 2020), presenting applications of some methods; work (Dibaj et al. 2021) considers coupled frameworks linking surface hydrodynamics river networks with salinity intrusion. Study (Chala et al. 2022) has to do with diverse factors related to the salinity intrusion, as a common contaminant that affects unconfined coastal aquifers.

Further, we refer to some current representative flow surface-subsurface studies, on numerical mixed partial differential equation (PDE) models (Edward et al. 2008; ZhiGuo and WeiMing 2009; Delfs et al. 2013). In work (Edward et al. 2008) numerical simulations of a coupled surface-subsurface flow and transport model, through interconnected aquifers separated by aquitards are implemented; study (ZhiGuo and WeiMing 2009) is concened with a physical integrated hydrologic model, that simulates rainfall surface water flow and saturated subsurface flow, as well as contaminant transport in the coupled system. Work (Delfs et al. 2013) develops a soil-air model, as a numerical coupled system of PDEs for hydrostatic shallow flow and two-phase flow in a porous medium.

Lastly, we describe surface-subsurface mixed variational models where, in contrast to our subpotential evolution mixed subdifferential methodology, divers classical approaches are applied (Lipnikov et al. 2014; Magiera et al. 2016; Caucao et al. 2022): In work(Lipnikov et al. 2014) locallized mass conservative variational approximations of Darcy-Stokes flows, are implemented by surface Stokes discontinuous Galerkin finite elements, and mimetic Darcy subsurface finite difference technics, determining optimal convergence estimates; paper (Magiera et al. 2016) considers coupled surface-subsurface flows with a nonlinear kinematic wave equation for the surface fluid, and a Brinkman model governing the subsurface fluid pressure–velocity dynamics, implemented utilizing coupled hyperbolic-elliptic finite volume numerical discretizations. And finally, study (Caucao et al. 2022) has to do with a new multipoint stress-flux mixed finite element method for a coupled problem, governed by the Stokes-Biot equations, of a free fluid and a poroelastic medium, having fluid velocity and poroelastic pressure Lagrange interface transmission constraints.

A description of the paper is the following. In Sect. 2 the variational real functional macro-hybrid mixed reflexive Banach frameworks for the filtration transport systems, as coupled media pairs, are introduced. Primal and dual abstract general variational multidomain mixed initial/boundary-value problems are stated in Sect. 3 in terms of subpotential maximal monotone inclusion systems, whose resolvent fixed-point existence-uniqueness qualitative results are established in Sect. 4 via corresponding evolution duality principles. Section 5 is concerned about the mixed filtration underground free boundary fractional two-phase coastal hydraulic Darcian system, with immovile seawater intrusion, leading to a mixed pressure–velocity qualitative physical description of the incompressible flow process; whose variational analysis is performed as a macro-hybridized subpotential inclusion mixed system. Section 6 has to do with the multidomain surface reservoirs, coupled incompressible primal and dual evolution Stokes mixed flow models, as well as with their variational formulation and analysis, as subpotential initial/boundary-value subdifferential problems. In Sect. 7 the surface-subsurface contaminant transport flow coupled process is treated variationally, via a multidomain dual evolution mixed formulation. Lastly, in Sect. 8, the variational macro-hybrid mixed filtration transport coupled systems of the study are established. Once appropriate surface-subsurface flux-traction-velocity interface mechanical transmissions, and corresponding Lagrangian dual solution subspaces, are determined, – these related to interface multidomains, – dual evolution transport coupled systems are concluded: a primal-dual traction coupled system and a dual-primal velocity coupled one. Last Sect. 9, states the conclusions of the work.

2 Macro-hybrid mixed functional frameworks

In this section, we start introducing the variational macro-hybrid mixed reflexive Banach real functional frameworks, for the surface-subsurface filtration transport systems to be treated in this paper as coupled media pairs.

Let \(\Omega \subset \Re ^d\), \(d \in \{1, 2, 3\}\), be a bounded domain with a Lipschitz boundary \(\partial \Omega \), which for the material continuous mechanical coupled Darcy/Stokes flow system, will has the spatial disjoint decomposition defined by

$$\begin{aligned} \begin{array}{rcll} {\overline{\Omega }} &{} = &{} \displaystyle { {\overline{\Omega }}_{D} \cup {\overline{\Omega }}_{S} } \, &{} \text{ the } \ \textit{D/S} \ \text{ media } \text{ pair, } \\ \Gamma _{D/S} &{} = &{} \displaystyle { \partial \Omega _{D} \cap \partial \Omega _{S} } \, &{} \text{ the } \ \textit{D/S} \ \text{ interface } \text{ pair, } \end{array} \end{aligned}$$
(1)

where the interface \(\Gamma _{D/S}\) is assumed to be Lipschitz. In this way, the global domain \(\Omega \) is decomposed into a nonoverlapping Darcy/Stokes (D/S) multimedia pair. Then, utilizing the media subindex \(M \in \{D,S\}\), as the stationary functional multi-framework we consider the mixed primal and dual reflexive Banach spaces \(V(\Omega _{M})\) and \( Y^*(\Omega _{M})\), with topological duals \(V^*(\Omega _{M})\) and \( Y(\Omega _{M})\). Further, we introduce their primal and dual pivot Hilbert spaces \(H(\Omega _{M})\) and \(Z^*(\Omega _{M})\); i.e., \(V(\Omega _{M}) \subset H(\Omega _{M}) \subset V^*(\Omega _{M})\) and \(Y^*(\Omega _{M}) \subset Z^*(\Omega _{M}) \subset Y(\Omega _{M})\), with continuous and dense embeddings. Also, the primal and dual boundary trace spaces are defined by the reflexive Banach space \(B(\partial \Omega _{M})\) and its topological dual \(B^*(\partial \Omega _{M})\).

Next, in accordance with Alduncin (2007b), Alduncin (2011), we introduce the the multimedia M-pair \(\Omega _{M}\), which on the basis of connected disjoint spatial local subdomains \(\{\Omega _{M_e}\}\), \(e \in \{1,...,{E_{M}}\}\), is expressed by

$$\begin{aligned} \begin{array}{rcll} \Omega _{M} &{} = &{} \displaystyle { \bigcup ^{E_{M}}_{e=1} \Omega _{M_{e}}}, \, &{} \hbox {the }M\hbox {-multidomain}, \\ \Sigma _{M_{e}} &{} = &{} \partial \Omega _{M_{e}} \cap \partial \Omega _{M} \, \ e = 1, 2,..., E_{M}, \, &{} \hbox {the}\, M \hbox {-external boundaries}, \\ \Gamma _{M_{e}} &{} = &{} \partial \Omega _{M_{e}} \cap \Omega _{M} \, \ \ \ e = 1, 2,..., E_{M}, \, &{} \hbox {the}\, M\hbox {-internal boundaries}, \\ \Gamma _{M_{{ek}}} &{} = &{} \Gamma _{M_{e}} \cap \Gamma _{M_{k}} \, \ \ \ 1 \le e < k \le E_{M}, \, &{} \,\hbox {the}\, M\hbox {-interfaces}, \\ \end{array} \end{aligned}$$
(2)

with piecewise Lipschitz boundaries. Then, as a macro-hybrid mixed M-functional framework we have the product spaces: \({{\varvec{V}}_ {{\varvec{MH}}_{\varvec{M}}}} = \prod ^{E_{M}}_ {e=1} V(\Omega _{M_{e}})\), \({{\varvec{Y}}}^\mathbf{*}_ {{\varvec{MH}}_{\varvec{M}}} = \prod ^{E_{M}}_ {e=1} Y^*(\Omega _{M_{e}})\), with duals \( {{\varvec{V}}}^\mathbf{*}_ {{\varvec{MH}}_{\varvec{M}}} = \prod ^{E_{M}}_ {e=1} V^*(\Omega _{M_{e}})\), \({{\varvec{Y}}_ {{\varvec{MH}}_{\varvec{M}}}} = \prod ^{E_{M}}_ {e=1} Y(\Omega _{M_{e}})\), as well as the primal internal boundary product space \({{\varvec{B}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}} \equiv \prod ^{E_{M}}_{e=1} B(\Gamma _ {M_{e}})\) and its dual \({{{\varvec{B}}}^\mathbf{*}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}} \equiv \prod ^{E_{M}}_{e=1} B^*(\Gamma _ {M_{e}})\).

Thereby, for a macro-hybridization of the multidomain multisystem pairs of the study, we shall consider that the primal \(\Omega _M\)-field stationary space \(V(\Omega _M)\) is decomposable in terms of a macro-hybrid internal boundary transmission condition

$$\begin{aligned} V(\Omega _{M}) \ = \ \Big \{{{\varvec{v}}_{\varvec{M}}} \in {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}: \ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{M}}}}} {{\varvec{v}}_{\varvec{M}}} \in {{\varvec{Q}}_{\varvec{M}}} \Big \}, \end{aligned}$$
(3)

where \({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}\) is the primal linear continuous internal boundary trace operator, satisfying in the context of Sobolev spaces the fundamental compatibility condition (Adams and Fournier 2003)

$$\begin{aligned} ({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}}) \ \ \ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} \ \in \ {{\mathcal {L}}}({{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}, {{\varvec{B}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}}) \ \text{ is } \text{ surjective }. \end{aligned}$$

Hence, \({{\varvec{Q}}_{\varvec{M}}} \subset {{\varvec{B}}_{{\varvec{{MH}}}_{\varvec{\Gamma }_{\varvec{M}}}}}\) corresponds to the continuity transmission variational subspace guaranteeing a global internal boundary primal weak continuity. We should note that, significantly, trace surjectivity condition \(({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}})\) is characterized by the lower boundedness of its own transpose trace operator \({\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}}} \ \in \ {\varvec{{\mathcal {L}}}}({{\varvec{B}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, {{\varvec{V}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}})\), property that in turn is equivalent to the own transpose injectivity with a closed range Yosida (1974).

On the other hand, the dual \(\Omega _M\)-field stationary space and Hilbert pivot spaces are supposed to be decomposable in the natural unconstrained product forms: \(Y^*(\Omega _M) = {{\varvec{Y}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}\), \(H(\Omega _{M}) = {{\varvec{H}}_{{{\varvec{MH}}_{\varvec{M}}}}} \equiv \prod ^{E_{M}}_{e=1} H(\Omega _{M_{e}})\) and \(Z^*(\Omega _{M}) = {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \equiv \prod ^{E_{M}}_{e=1} Z^*(\Omega _{M_{e}})\).

As a last result in this stationary context, we state the central macro-hybrid variational composition dualization lemma that allows the primal variational incorporation of internal boundary transmission conditions, which follows by a process of convex and composition dualizations (cf. Alduncin 2005, Lemma 2.1).

Lemma 1

Due to primal trace surjective compatibility condition \(({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}})\), for \({\varvec{u}}_{\varvec{M}} \in {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}\) and \({\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {{\varvec{B}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}\), the macro-hybrid compositional dualization

$$\begin{aligned} {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \ \Longleftrightarrow \ {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial }} ({{\mathcal {I}}}_{Q_{M}} \circ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}) {{\varvec{u}}_{\varvec{M}}} \end{aligned}$$
(4)

holds true, where \({{\mathcal {I}}}^*_{Q_{M}}\) stands as the conjugate of indicator functional \({{\mathcal {I}}}_{Q_{M}}\).

Proof

By convex dualization \({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}}\) \(\Leftrightarrow \) \({\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\mathcal {I}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}}\). Then, compositional characterization (4) is satisfied, since under condition \(({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_\mathbf{}{M}}}})\) the variational inequalities of primal inclusions \({\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\mathcal {I}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}}\) and \({\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial }} ({{\mathcal {I}}}_{Q_{M}} \circ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}) {{\varvec{u}}_{\varvec{M}}}\) turn out to be equivalent:

$$\begin{aligned} \begin{array}{l} {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \in {{\varvec{Q}}_{\varvec{M}}} \subset {{\varvec{B}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}}: \\ {{\mathcal {I}}}_{Q_{M}} ({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{v}}_{\varvec{M}}}) \ge {{\mathcal {I}}}_{Q_{M}} ({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}}) + \big<{\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}}, {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{v}}_{\varvec{M}}} - {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \big>_{B_{MH_{\Gamma _M}}}, \\ \forall \ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{v}}_{\varvec{M}}} \in \ {{\varvec{B}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}} \, \\ \text{ and } \\ {{\varvec{u}}_{\varvec{M}}} \in {{\mathcal {D}}}({{\mathcal {I}}}_{Q_{M}} \circ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}) \subset {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}: \\ ({{\mathcal {I}}}_{Q_{M}} \circ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}) ({{\varvec{v}}_{\varvec{M}}}) \ge ({{\mathcal {I}}}_{Q_{M}} \circ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}) ({{\varvec{u}}_{\varvec{M}}}) + \big <{\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}}, {{\varvec{v}}_{\varvec{M}}} - {{\varvec{u}}_{\varvec{M}}}\big >_{V_{{MH_{M}}}}, \\ \forall \ {{\varvec{v}}_{\varvec{M}}} \in {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}.\end{array} \end{aligned}$$

\(\square \)

Additionally, we introduce the multidomain media pair evolution mixed functional frameworks that will be required by the mechanical incompressible flow models, of subsurface Darcy and surface Stokes evolution variational problems. Given a time interval (0, T), \(T > 0\) arbitrary and fixed, we define the primal and dual evolution reflexive Banach spaces, for \(2 \le p < \infty \) and \(q^* = p / (p - 1)\),

$$\begin{aligned} {\varvec{{\mathcal {V}}}_{{{\varvec{MH}}_{\varvec{M}}}}}= & {} {{\varvec{L}}^{\varvec{p}}}(0,T; {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}}) \nonumber \\\equiv & {} \displaystyle { \bigg \{ \{v_{M_e}\}: [0, T] \rightarrow {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}} \ / \ \Vert \{v_{M_e}\} \Vert _{{{\mathcal {V}}}_{{{MH_M}}}} } \nonumber \\= & {} \bigg [ \int ^T_0 \Vert \{v_{M_e}\}(t)\Vert ^p_{V_{{{MH_M}}} } dt \bigg ]^{1/p}< \infty \bigg \}, \\ {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}= & {} {{\varvec{L}}^{{\varvec{q}}^{{\varvec{*}}}}}(0,T; {{\varvec{Y}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}})\nonumber \\\equiv & {} \displaystyle { \bigg \{ \{y^*_{M_{M_e}}\}:[0, T] \rightarrow {{\varvec{Y}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \ / \ \Vert \{y^*_{M_e}\}\Vert _{{{\mathcal {Y}}}^*_{{{MH_M}}} } } \nonumber \\= & {} \bigg [ \int ^T_0 \Vert \{y^*_{M_e}\}(t)\Vert ^{q^*}_{Y^*_{{{MH_M}}}} dt \bigg ]^{1/q^*} < \infty \bigg \}, \nonumber \end{aligned}$$
(5)

whose respective topological duals are \({\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} = {{\varvec{L}}^{{\varvec{q}}^{{\varvec{*}}}}}(0,T; {{\varvec{V}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}})\), \({\varvec{{\mathcal {Y}}}_{{{\varvec{MH}}_{\varvec{M}}}}} = {{\varvec{L}}^{\varvec{p}}}(0,T; {{\varvec{Y}}_{{{\varvec{MH}}_{\varvec{M}}}}})\).

Then, appropriate evolution primal and dual solution reflexive Banach spaces are given by

$$\begin{aligned} {\varvec{{\mathcal {W}}}_{{{\varvec{MH}}_{\varvec{M}}}}}= & {} \Big \{\{v_{M_e}\}: \{v_{M_e}\} \in {\varvec{{\mathcal {V}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, d \{v_{M_e}\} / d t \in {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \Big \},\nonumber \\ {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}= & {} \Big \{\{y^*_{M_e}\}: \{y^*_{M_e}\} \in {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, d \{y^*_{M_e}\} / d t \in {\varvec{{\mathcal {Y}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \Big \}, \end{aligned}$$
(6)

with corresponding operator norms and . In this manner the following important structural properties are guaranteed, indispensable for the solvability consistency of variational initial value problems (Lions 1969): primal solution space \({\varvec{{\mathcal {W}}}_{{{\varvec{MH}}_{\varvec{M}}}}}\) is continuous densely embedded in , the space of \({{\varvec{H}}_ {{{{\varvec{MH}}_{\varvec{M}}}}}}\)-continuos functions, with initial values set ; and dual solution space is continuous densely embedded in , the space of -continuos functions, with initial values set .

Regarding the associated evolution internal boundary macro-hybrid trace spaces, we have the reflexive Banach space and its dual .

3 Primal and dual evolution macro-hybrid mixed variational constrained initial/boundary-value problems

Next, we formulate and analyze the abstract macro-hybrid evolution mixed constrained boundary-value problems, which will be applied to the surface-subsurface filtration transport multidomain phenomena of the paper, with seawater intrusion in relation to a three-dimensional nonhomogeneous anisotropic unconfined coastal aquifer. Existence-uniqueness resolvent fixed-point results will be established.

Applying the macro-hybrid mixed functional reflexive Banach frameworks of Sect. 2, let us consider the evolution variational macro-hybridized mixed constrained boundary-value problems of the theory, set on the evolution framework of reflexive Banach spaces (5) and (6). In a general sense, we shall utilize the media index \(M \in \{D,S\}\), for D-Darcy and S-Stokes models (to be presented below in Sects. 5 and 6).

As a primal evolution mixed model problem, we have the variational macro-hybridized constrained initial/boundary-value problem

$$\begin{aligned} ({\varvec{{\mathcal {M}}_{\varvec{\mathcal{M}\mathcal{H}}}}_{\varvec{M}}}) \left\{ \begin{array}{l} \text{ Given } \ {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {R}}} (-{\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{{\varvec{MH}}}_{\varvec{M}}}} \, \ {{\varvec{g}}_{\varvec{M}}} \in {\varvec{{\mathcal {Y}}}_ {{{\varvec{MH}}}_{\varvec{M}}}}, \, \\ \text{ and } \ {{\varvec{\widehat{u}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{H}}_ {{{\varvec{MH}}}_{\varvec{M}}}}: \\ \text{ find } \ ( {{\varvec{u}}_{\varvec{M}}}, {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}) \in {\varvec{{\mathcal {W}}}_ {{{\varvec{MH}}}_{\varvec{M}}}} \times {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ - {\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} \\ \hspace{0.5cm} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{u}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } {\varvec{F}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{u}}_{\varvec{M}}}, \\ \hspace{0.8cm} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{{\varvec{N}}}}} {{\varvec{\widehat{p}}}^{{\varvec{*}}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{M}}}}}) {{\varvec{u}}_{\varvec{M}}} - {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}}, \, \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{{\varvec{MH}}}_{\varvec{M}}}}, \, \\ {\varvec{\Lambda }_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{g}}_{\varvec{M}}}, \, \text{ in } \ {\varvec{\mathcal {Y}}_ {{{\varvec{MH}}}_{\varvec{M}}}}, \, \\ {{\varvec{u}}_{\varvec{M}}}(0) = {{\varvec{\widehat{u}}}_{{\varvec{M}}_{\varvec{0}}}}, \end{array} \right. \end{aligned}$$

where, for M-material local systems, primal operator \({\varvec{\partial } {\varvec{F}}_{\varvec{M}}}: {\varvec{{\mathcal {V}}}_ {{{\varvec{MH}}}_{\varvec{M}}}} \rightarrow {{\varvec{2}}^{{\varvec{\mathcal {V}}}^{{\varvec{*}}}_ {{{\varvec{MH}}}_{\varvec{M}}}}}\) models local mechanical balance or constitutive subpotential maximal monotone inclusions, and for computing purposes dual operator \({\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}}: {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {{\varvec{2}}^{\varvec{{\mathcal {Y}}}_ {{{\varvec{MH}}}_{\varvec{M}}}}}\) states dualized distributed primal constraints. Operators \({\varvec{\Lambda }_{\varvec{M}}} \in {{\mathcal {L}}}({\varvec{\mathcal {V}}_ {{\varvec{MH}}_{\varvec{M}}}}, {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}})\) and its transpose \({\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}} \in {\mathcal {L}}({\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}, {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}})\) are single-valued linear continuous local mixed coupling operators. Further, the primal external boundary composition operator \({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}: {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {{\varvec{2}}^{\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}}\) incorporates variationally the local essential Dirichlet boundary conditions and constraints of the pairs system. Dual trace \({\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}}\) denotes the prescribed local natural Neumann boundary condition. Also, the internal boundary composition subpotential operator \({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{M}}}}}: {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {{\varvec{2}}^{{\varvec{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}}\) imposes the macro-hybrid local constraint in (3).

For a variational maximal monotonicity structure of evolution problem \(({\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\), a fundamental property for its qualitative analysis, as well as numerical analysis, we consider the following composition duality result applicable to variational composition subpotential subdifferential operators (cf. Alduncin 2010, Lemma 2.1; and Lemma 5.1 in Alduncin 2017).

Lemma 2

In the context of mixed reflexive Banach spaces, proper lower semicontinuous convex functionals \(J: E \rightarrow \Re \cup \{+ \infty \}\) and linear continuous operators \(S \in {\mathcal L} (D, E)\), with transpose \(S^T \in {{\mathcal {L}}} (E^*, D^*)\), under the compatibility condition

$$\begin{aligned} ({{\varvec{C}}_{\varvec{S}}}) \ \ \ S \in {{\mathcal {L}}} (D, E) \ \text{ is } \text{ surjective } \end{aligned}$$

(equivalent to the traspose \(S^T\) injevtivity with a closed range (Yosida 1974)) the composition duality relation

$$\begin{aligned} S^T \partial J \circ S \ = \ \partial (J \circ S) \end{aligned}$$
(7)

holds true; i.e., subpotential subdifferential composition operator \(S^T \partial J \circ S: \ D \rightarrow {{\varvec{2}}^{{\varvec{D}}^{{\varvec{*}}}}}\) results to be maximal monotone.

Proof

Since compatibility condition \(({{\varvec{C}}_{\varvec{S}}})\) implies the injectivity of transpose operator \(S^T \in {{\mathcal {L}}} (E^*, D^*)\), for any functional \(d^* \in {{\mathcal {R}}}(S^T) \subset D^*\) and its \(S^T\)-preimage \(e^* \in E^*\) necessarily, for \(\widetilde{d} \in D\),

$$\begin{aligned} d^* \in S^T \partial R(S \widetilde{d}) \ \Longleftrightarrow \ e^* \in \partial R(S \widetilde{d}) \end{aligned}$$

holds true. Moreover, in turn, the equivalence

$$\begin{aligned} e^* \in \partial R(S \widetilde{d}) \ \Longleftrightarrow \ d^* \in \partial (R \circ S) (\widetilde{d}) \end{aligned}$$

is further satisfied since their corresponding variational inequalities

$$\begin{aligned} \begin{array}{rcl} S \widetilde{d} \in {{\mathcal {D}}}(R) \subset E: \ \ R (e) &{} \ge &{} R (S\widetilde{d}) + \big<e^*, e - S \widetilde{d}\big>_{E^*,E}, \ \forall e \in E, \\ \widetilde{d} \in {{\mathcal {D}}}(R \circ S) \subset D: \ \ (R \circ S) (d) &{} \ge &{} (R\circ S) ( \widetilde{d}) + \big < d^*, d - \widetilde{d} \big >_{D^*,D}, \ \forall d \in D, \end{array} \end{aligned}$$

are equivalent due to the S-surjectivity. Then the desired result is concluded. \(\square \)

Furthermore, applying the interior domain condition for the sum of maximal monotone operators to be maximal monotone in a setting of reflexive Banach spaces (Rockafellar 1970; Barbu 2010), we have the following conclusive result that establishes the maximal monotonicity structure of the problem.

Corollary 3

Under primal trace compatibility condition \(({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}}})\), the primal variational composition monotone operator of macro-hybridized evolution problem \(({\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\),

$$\begin{aligned} {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}} = {\varvec{\partial } {\varvec{F}}_{\varvec{M}}} + {\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial \Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}} + {\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{\Gamma }{\varvec{M}}}}}}: \ {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {{\varvec{2}}^{\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}}, \end{aligned}$$
(8)

is maximal monotone whenever its own interior domain condition

$$\begin{aligned}({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}}) \ \ \ \text{ int } \ {\varvec{{\mathcal {D}}}} ({\varvec{\partial } {\varvec{F}}_{\varvec{M}}}) \cap \Big ({\varvec{{\mathcal {D}}}} ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}})} \cap {\varvec{{\mathcal {D}}}} ({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{M}}}}})\Big ) \ne {\varvec{\emptyset }} \end{aligned}$$

is satisfied, and in such a case, problem \(({\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) becomes a maximal monotone variational inclusion problem, due to the additional maximal monotonicity of the variational time differentiation operator densely defined by (cf. Zeidler 1990, Proposition 32.10)

$$\begin{aligned} {{\varvec{d}} / {\varvec{dt}}}: {\varvec{{\mathcal {D}}}} ({{\varvec{d}} / {\varvec{dt}}}) = \{{{\varvec{v}}_{\varvec{M}}} \in {\varvec{{\mathcal {W}}}_ {{\varvec{MH}}_{\varvec{M}}}}: {{\varvec{v}}_{\varvec{M}}}(0) = {{\varvec{0}}}\} \subset {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}. \end{aligned}$$
(9)

Regarding the boundary conditions and constrains of problem \(({\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\), we shall assume that the local external boundaries \(\Sigma _{M_{e}} = \partial \Omega _{M_{e}} \cap \partial \Omega _M\), \(e = 1, 2,..., E_M\), are decomposed in terms of disjoint complementary sub-boundaries, \(\Sigma _{M_{e}} = \Sigma _{M_{{D_e}}} \cup \Sigma _{M_{{N_e}}} \cup \Sigma _{M_{{C_e}}}\). Then, as prescribed essential primal Dirichlet and natural dual Neumann boundary conditions, we introduce the following ones: \({\varvec{\pi }_{{\varvec{M}}_{{\varvec{D}}}}} {{\varvec{u}}_{\varvec{M}}} = {\varvec{\widehat{u}}_{\varvec{M}}}\in {\varvec{{\mathcal {B}}}_{{\varvec{MH}}_{{\varvec{M}}_{\varvec{D}}}}} = \prod ^{E_{M}}_{e=1} {{\mathcal {B}}}(\Sigma _{M_{{D_e}}})\) and \({\varvec{\delta }^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} = {\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{M}}}} = \prod ^{E_M}_{e=1} {{\mathcal {B}}}^*(\Sigma _{M_e})\), \({\varvec{\delta }^{{\varvec{*}}}_{\varvec{M}}} \ \in \ {\mathcal {L}}({\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, {\varvec{\mathcal {B}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{\Gamma }_{\varvec{M}}}}})\) denoting the dual external boundary trace operator. For local external boundary constraints, we consider on the complementary boundary space \({\varvec{{\mathcal {B}}}_{{\varvec{MH}}_{{\varvec{M}}_{\varvec{C}}}}} = \prod ^{E_M}_{e=1} {{\mathcal {B}}}(\Sigma _{M_{{C_e}}})\) that boundary constraints are modeled by means of subpotential maximal monotone mechanisms \({\varvec{\partial } \varvec{\Psi }_{{\varvec{M}}_{{{\varvec{e}}_{\varvec{C}}}}}} {\varvec{{\mathcal {B}}}_ {{\varvec{MH}}_{{\varvec{M}}_{\varvec{C}}}}} \rightarrow {{\varvec{2}}^{\varvec{{\mathcal {B}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{{\varvec{M}}_{\varvec{C}}}}}}\) (Duvaut and Lions 1972).

Thereby, as an explicit variational primal external boundary composition subdifferential component, of the general model problem, the following one is achieved:

$$\begin{aligned} \begin{array}{l} ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{u}}_{\varvec{M}}} \\ \hspace{0.5cm} = ({\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{\varvec{D}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{\varvec{{\widehat{}u}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{M}}_{{\varvec{D}}}}}) {{\varvec{u}}_{\varvec{M}}} + {\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{{\varvec{N}}}}} {\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{\varvec{C}}}} {\varvec{\partial } \varvec{\Psi }_{{\varvec{M}}_{\varvec{C}}} } \circ {\varvec{\pi }_{{\varvec{M}}_{{\varvec{C}}}}}) {{\varvec{u}}_{\varvec{M}}} \, \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \, \end{array} \end{aligned}$$
(10)

were the essential Dirichlet boundary condition is implemented by the indicator functional \({{\mathcal {I}}}_{\widehat{u}_M}\).

Similarly, a dual evolution general abstract variational problem is given by

$$\begin{aligned} ({\varvec{{\mathcal {M}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}}) \left\{ \begin{array}{l} \text{ Given } \ {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {R}}} (-{\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \, \ {{\varvec{g}}_{\varvec{M}}} \in {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}},\\ \text{ and } \ {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{Z}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ \text{ find } \ ( {{\varvec{u}}_{\varvec{M}}}, {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \times {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ - {\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{F}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{u}}_{\varvec{M}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{{\varvec{N}}}}} {\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}} \\ \hspace{2.0cm} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{M}}}}}) {{\varvec{u}}_{\varvec{M}}} - {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ {\varvec{\Lambda }_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{g}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}} ,\\ {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}(0) = {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}}. \end{array} \right. \end{aligned}$$

Finally, we can conclude from the above external boundary result, in conjunction with macro-hybrid composition duality result (4) of Lemma 1 (on interior internal boundary transmission conditions), the macro-hybrid variational versions of macro-hybridized problems \((\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}})\) and \(({\varvec{{\mathcal {M}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}})\):

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}) \left\{ \begin{array}{l} \text{ Given } \ {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{{\mathcal {R}}}} (-{\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \, \ {{\varvec{g}}_{\varvec{M}}} \in {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ \text{ and } \ {\varvec{\widehat{u}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{H}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ \text{ find } \ ( {{\varvec{u}}_{\varvec{M}}}, {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}) \in {\varvec{{\mathcal {W}}}_ {{\varvec{MH}}_{\varvec{M}}}} \times {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ - {\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}}\\ \hspace{1.0cm} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{u}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } {\varvec{F}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{u}}_{\varvec{M}}} \\ \hspace{1.5cm} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{{\varvec{N}}}}} {\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} ,\\ {\varvec{\Lambda }_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{g}}_{\varvec{M}}} , \\ \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ {{\varvec{u}}_{\varvec{M}}}(0) = {\varvec{\widehat{u}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

and

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{M}}}) \left\{ \begin{array}{l} \text{ Given } \ {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {R}}} (-{\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \, \ {{\varvec{g}}_{\varvec{M}}} \in {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}}, \\ \text{ and } \ {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{Z}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ \text{ find } \ ( {{\varvec{u}}_{\varvec{M}}}, {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \times {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ - {\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} - \varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{M}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{F}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{u}}_{\varvec{M}}} \\ \hspace{4.2cm} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{M}}_{{\varvec{N}}}}} {\varvec{\widehat{p}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{f}}^{{\varvec{*}}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ {\varvec{\Lambda }_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{g}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}}(0) = {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

which are both synchronized in terms of the macro-hybrid evolution subpotential subdifferential dual problem, which implements their local internal boundary \({\varvec{\Gamma }_{\varvec{M}}}\)-transmission multidomain constraints:

$$\begin{aligned} ({\varvec{{\mathcal {T}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{{\mathcal {B}}^{{\varvec{*}}}}_{{{\varvec{MH}}_{\varvec{M}}}}}: \\ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \ \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \, \ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{M}}}}}. \end{array} \right. \end{aligned}$$

Such evolution variational abstract problems, will correspond specifically to the subsurface Darcy and surface Stokes flow macro-hybrid mixed problems to be treated below in Sects. 5 and 6, respectively.

4 Macro-hybrid mixed resolvent fixed-point solvability analysis

Regarding qualitative solvability results for multimedia mixed pair evolution variational systems, we next apply primal and dual fixed-point mixed results established in Alduncin (2010), Alduncin (2014), via primal and dual duality principles and resolvent fixed-point characterizations.

For the variational existence analysis of primal and dual evolution macro-hybrid mixed constrained boundary-value problems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{M}}})\), equivalent to their macro-hybridized versions \(({\varvec{{\mathcal {M}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}})\) and \(({\varvec{{\mathcal {M}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}})\), primal and dual evolution duality principles are required. Thus, we introduce the respective classical macro-hybrid compatibility domain interior conditions (cf.Alduncin 2007b, Sect. 3):

$$\begin{aligned} \begin{array}{rl} \Big ({{\varvec{C}}_{{\varvec{G}}_{\varvec{M}}, \varvec{\Lambda }_{\varvec{M}}}}\Big ) &{} \text{ int } \ {\varvec{{\mathcal {D}}}} ({{\varvec{G}}_{\varvec{M}}}) \cap {\varvec{{\mathcal {R}}}} ({\varvec{\Lambda }_{\varvec{M}}}) \ne {\varvec{\emptyset }} , \\ \\ \Big ({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}^{{\varvec{*}}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}, -\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}})}}\Big ) &{} \text{ int } \ {\varvec{{\mathcal {D}}}} \Big ({\varvec{\partial } \varvec{\widetilde{F}}^{{\varvec{*}}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}}\Big ) \cap {\varvec{ {\mathcal {R}}}} (-{\varvec{\Lambda }}^{\varvec{T}}_{\varvec{M}}) \ne {\varvec{\emptyset }} , \end{array} \end{aligned}$$

under which the composition duality relations

$$\begin{aligned} \begin{array}{rl} {\varvec{\Lambda }}^{\varvec{T}}_{\varvec{M}} {\varvec{\partial }}\varvec{G}_{\varvec{M}} \circ {\varvec{\Lambda }}_{\varvec{M}} &{} = \ {\varvec{\partial } ({\varvec{G}}_{\varvec{M}}} \circ {\varvec{\Lambda }_{\varvec{M}}}), \\ \\ {-\varvec{\Lambda }_{{\varvec{M}}}} \ {\varvec{\partial } \varvec{\widetilde{F}}^{{\varvec{*}}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}} \circ -{\varvec{\Lambda }^{{\varvec{T}}_{\varvec{M}}}} &{} = \ {\varvec{\partial }\Big ( \varvec{\widetilde{F}}^{{\varvec{*}}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}} \circ \ -{\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}}\Big ), \end{array} \end{aligned}$$
(11)

hold true (Ekeland and Temam 1974). Thereby, primal and dual duality principles for the macro-hybrid evolution problems are necessarily achieved as follows (cf.Alduncin 2007b. Lemma 3.1).

Lemma 4

Let primal trace compatibility condition \(({{\varvec{C}}_{[\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{\alpha }_{\varvec{e}}}}}]}})\), as well as compatibility domain interior conditions \(\Big ({{\varvec{C}}_{{\varvec{G}}_{\varvec{M}}, \varvec{\Lambda }_{\varvec{M}}}}\Big )\) and \(\Big ({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}^{{\varvec{*}}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}, -\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}})}}\Big )\) be satisfied. Then primal and dual evolution macro-hybrid mixed problems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{M}}})\) are respectively equivalent in a solvability sense to their evolution macro-hybridized primal and dual problems:

$$\begin{aligned} ({\varvec{{\mathcal {P}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}}) \left\{ \begin{array}{l} \text{ Find } \ {{\varvec{u}}_{\varvec{M}}} \in {\varvec{{\mathcal {W}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ \begin{array}{ll} {{\varvec{0}}^{{\varvec{*}}}_{\varvec{M}}} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{u}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} + {\varvec{\partial }} (G_M \circ \Lambda _M) ({{\varvec{u}}_{\varvec{M}}} -{{\varvec{w}}_{{{\varvec{g}}_{\varvec{M}}}}}) - {\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}}, \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ \hspace{1.2cm} {{\varvec{u}}_{\varvec{M}}} (0) = {\varvec{\widehat{u}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \end{array} \right. \end{aligned}$$

and

$$\begin{aligned} ({\varvec{{\mathcal {D}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}}) \left\{ \begin{array}{l} \text{ Find } \ {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {X}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ {{\varvec{0}}_{\varvec{M}}} \in \displaystyle {\frac{{{\textit{\textbf{d p}}}^{{\varvec{*}}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } {{\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}}} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} + {\varvec{\partial }} (\widetilde{F}^*_{M_ {/_{V_{MH_M}}}} \circ (-\Lambda ^T_M)) ({{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} -{{\varvec{q}}^{{\varvec{*}}}_{\varvec{{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}}}}) \\ \hspace{1.0cm} + {{\varvec{g}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ \hspace{1.2cm} {{\varvec{p}}^{{\varvec{*}}}_{\varvec{M}}} (0) = {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

where \({{\varvec{w}}_{{\varvec{g}}_{\varvec{M}}}}\) is a \(\varvec{\Lambda }_{\varvec{M}}\)-preimage of functional \({{\varvec{g}}_{\varvec{M}}}\) and \({{\varvec{q}}^{{\varvec{*}}}_{\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}}}\) a \(-\varvec{\Lambda }^{\varvec{T}}_{\varvec{M}}\)-preimage of \(\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}\).

The sufficiency of these evolution duality principles is due to the fact that, indeed, such principles apply as well to the primal and dual macro-hybridized systems \(({\varvec{{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) and \(({\varvec{{\mathcal {M}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\). Further, by condition \(({{\varvec{C}}_{{[\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{\alpha }_{\varvec{e}}}}}]}}})\) and the Closed Range Theorem, even such macro-hybridized systems turn out to be equivalent (in a solvability sense) to their own macro-hybrid mixed systems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{M}}})\) (cf. Alduncin 2007b, Theorems 3.2 and \(3.2^*\)).

Next, we state existence results for primal and dual evolution problems \(({\varvec{{\mathcal {P}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) and \(({\varvec{{\mathcal {D}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) (Alduncin 2014), which correspond to a fixed-point analysis via a resolvent characterization of preconditioned augmented and exactly penalized formulations, of their primal and dual variational inclusions, respectively. Because of the important role played by such results in our study, we shall provide the details of their validity.

Theorem 5

Let the macro-hybrid monotone primal and dual operator conditions

$$\begin{aligned} ({\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}) \left\{ \begin{array}{l} {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}: {\varvec{\mathcal {V}}_{{{\varvec{MH}}_{\varvec{M}}}}} \rightarrow {{\varvec{2}}^{{\varvec{\mathcal {V}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}} \ \text{ is } \text{ strongly } \text{ monotone; } \text{ i.e., } \ \exists \ \widetilde{\beta }_M> 0: \\ \big <{{\varvec{w}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{v}}^{{\varvec{*}}}_{\varvec{M}}}, {{\varvec{w}}_{\varvec{M}}} - {{\varvec{v}}_{\varvec{M}}}\big >_{{\mathcal {V}}_{{MH_M}}} \ge \widetilde{\beta }_M\Vert {{\varvec{w}}_{\varvec{M}}} - {{\varvec{v}}_{\varvec{M}}}\Vert ^2_{{{\mathcal {V}}}_{{MH_M}}} , \\ \forall \ {{\varvec{w}}_{\varvec{M}}}, {{\varvec{v}}_{\varvec{M}}} \in {\varvec{{\mathcal {V}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, \ {{\varvec{w}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}} {{\varvec{w}}_{\varvec{e}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}} {{\varvec{v}}_{\varvec{M}}} , \end{array} \right. \end{aligned}$$

and

$$\begin{aligned} ({{\varvec{C}}_{\varvec{\partial } {\varvec{G}}^{\varvec{{\varvec{*}}}}_{\varvec{M}}}}) \left\{ \begin{array}{l} {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}}: {\varvec{\mathcal {Y}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \rightarrow {{\varvec{2}}^{{\varvec{\mathcal {Y}}}_{{{\varvec{MH}}_{\varvec{M}}}}}} \ \text{ is } \text{ strongly } \text{ monotone; } \text{ i.e., } \ \exists \ \beta ^*_M> 0: \\ \big < {{\varvec{s}}_{\varvec{M}}} - {{\varvec{q}}_{\varvec{M}}}, {{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{q}}^{{\varvec{*}}}_{\varvec{M}}} \big >_{{{\mathcal {Y}}}^*_{{MH_M}}} \ge \beta ^*_M \Vert {{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{q}}^{{\varvec{*}}}_{\varvec{M}}}\Vert ^2_{{{\mathcal {Y}}}^*_{{MH_M}}} , \\ \forall \ {{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}}, {{\varvec{q}}^{{\varvec{*}}}_{M}} \in {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, \ {{\varvec{s}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}}, {{\varvec{q}}_{\varvec{M}}} \in {\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}} {{\varvec{q}}^{{\varvec{*}}}_{\varvec{M}}} , \end{array} \right. \end{aligned}$$

be fulfilled. Then for primal and dual initial conditions as regular as

$$\begin{aligned} \begin{array}{l} {\varvec{\widehat{u}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{V}}_{{{\varvec{MH}}_{\varvec{M}}}}} \subset {{\varvec{H}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \\ \text{ and } \\ {\varvec{\widehat{p}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{Y}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \subset {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}} , \end{array} \end{aligned}$$
(12)

evolution macro-hybridized primal problem \(({\varvec{{\mathcal {P}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) and dual problem \(({\varvec{{\mathcal {D}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) have a unique solution, respectively.

Proof

First taking advantage of the initial condition regularity \((12)_1\), we can consider the primal evolution inclusion, with homogeneous initial data,

$$\begin{aligned} ({\varvec{\widetilde{{{\mathcal {P}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}}}) \left\{ \begin{array}{l} \text{ Find } \ {{\varvec{s}}_{\varvec{M}}} \in {\varvec{{\mathcal {W}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ {{\varvec{0}}^{{\varvec{*}}}_{\varvec{M}}} \in \displaystyle {\frac{{{\varvec{d}} {\varvec{s}}_{\varvec{M}}}}{{{\varvec{dt}}}}} + {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}} {{\varvec{s}}_{\varvec{M}}} + {\varvec{\partial }} (G_M \circ \Lambda _M) ({{\varvec{s}}_{\varvec{M}}} -{{\varvec{p}}_{{{\varvec{g}}_{\varvec{M}}}}}) - {\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}} , \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} , \\ \hspace{1.0cm} {{\varvec{s}}_{\varvec{M}}} (0) = {\varvec{\widehat{0}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

whose solvability, for \({{\varvec{s}}_{\varvec{M}}} = {{\varvec{u}}_{\varvec{M}}} + {\varvec{\widehat{u}}_{{\varvec{M}}_{\varvec{0}}}}\), is readily equivalent to that of problem \(({\varvec{\mathcal {P}}_{{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}})\). Further, taking into account the maximal monotonicity of the linear variational time derivative operator \({{\varvec{d}} / {\varvec{dt}}}: {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}\) densely defined in (9), we can apply a fixed-point subdifferential approach for the existence analysis following Alduncin (2010). Indeed, we then have that primal monotone condition \( ({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}})\) is equivalent to the combined primal operator similar condition

$$\begin{aligned} ({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{A}}_{\varvec{M}}}}) \left\{ \begin{array}{l} {\varvec{\widetilde{A}}_{\varvec{M}}} = {{\varvec{d}} / {\varvec{dt}}} + {\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}: {\varvec{{\mathcal {V}}}_{{{\varvec{MH}}_{\varvec{M}}}}} \rightarrow {{\varvec{2}}^{\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{M}}}}}} \ \text{ is } \text{ strongly } \text{ monotone }; \\ \text{ i.e., } \ \exists \ \widetilde{\alpha }_M> 0: \\ \big <{{\varvec{w}}^{{\varvec{*}}}_{\varvec{M}}} - {{\varvec{v}}^{{\varvec{*}}}_{\varvec{M}}}, {{\varvec{w}}_{\varvec{M}}} - {{\varvec{v}}_{\varvec{M}}}\big >_{{\mathcal {V}}_{{MH_M}}} \ge \widetilde{\alpha }_M\Vert {{\varvec{w}}_{\varvec{M}}} - {{\varvec{v}}_{\varvec{M}}}\Vert ^2_{{{\mathcal {V}}}_{{MH_M}}} , \\ \forall \ {\varvec{w}}_{\varvec{M}}, {{\varvec{v}}_{\varvec{M}}} \in {\varvec{{\mathcal {V}}}_{{{\varvec{MH}}_{\varvec{M}}}}}, \ {{\varvec{w}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\widetilde{A}}_{\varvec{M}}} {{\varvec{w}}_{\varvec{e}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\widetilde{A}}_{\varvec{M}}} {{\varvec{v}}_{\varvec{M}}}. \end{array} \right. \end{aligned}$$

In fact, since \(\text{ int } {\varvec{{\mathcal {D}}}} ({{\varvec{d}} / {\varvec{dt}}}) \cap {\varvec{{\mathcal {D}}}} ({\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{M}}}) \ne {\varvec{\emptyset }}\), operator \({\varvec{\widetilde{A}}_{\varvec{M}}}\) is maximal monotone. For a precondition augmented and exact penalized formulation of primal inclusion problem \(({\varvec{\widetilde{{{\mathcal {P}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}}})\), we introduce an m-linearly strongly monotone and a-Lipschitz continuous preconditioning operator \( {\varvec{{\mathcal {M}}}_{\varvec{M}}}: \ {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}}\) and an exact penalization parameter \(r > 0\), having then the augmented primal inclusion version, for \({{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\widetilde{A}}_{\varvec{M}}} ({{\varvec{s}}_{\varvec{M}}})\),

$$\begin{aligned} \begin{array}{l} {\varvec{{\mathcal {M}}}_{\varvec{M}}} ({{\varvec{s}}_{\varvec{M}}}) - r ({{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}}) \in ({\varvec{{\mathcal {M}}}_{\varvec{M}}} + r {\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}}) ) ({{\varvec{s}}_{\varvec{M}}}) \\ \\ \hspace{1.0cm} \Longleftrightarrow \ {{\varvec{s}}_{\varvec{M}}} = {{\varvec{F}}^{\varvec{r}}_{{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}}} ({{\varvec{s}}_{\varvec{M}}}) \equiv {{\varvec{J}}^{\varvec{r}}_{ {\varvec{\mathcal {M}}}_{\varvec{M}},\varvec{\partial } ({\varvec{G}}_{\varvec{M}} \varvec{\circ } \varvec{\Lambda }_{{\varvec{M}}_{{\varvec{w}}_{\varvec{g}}}})}} ({\varvec{{\mathcal {M}}}_{\varvec{M}}} ({{\varvec{s}}_{\varvec{M}}}) - r ({{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\widetilde{f}}^{{\varvec{*}}}_{\varvec{M}}})). \end{array} \end{aligned}$$
(13)

Here \({{\varvec{J}}^{\varvec{r}}_{ {\varvec{\mathcal {M}}}_{\varvec{M}},\varvec{\partial } ({\varvec{G}}_{\varvec{M}} \varvec{\circ } \varvec{\Lambda }_{{\varvec{M}}_{{\varvec{w}}_{\varvec{g}}}})}} = ({\varvec{{\mathcal {M}}}_{\varvec{M}}} + r {\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}}) )^{-1}: {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{M}}}} \rightarrow {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}}\) is the \({\varvec{{\mathcal {M}}}_{\varvec{M}}} \)-resolvent operator of the maximal monotone operator \({\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}}) = {\varvec{\partial }} (G_M \circ \Lambda _M) ((\cdot ) - w_g) \), which is a well defined 1/m-firm contraction (Alduncin 2005). Thereby, primal problem \(({\varvec{\widetilde{{{\mathcal {P}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}}})\) has the \({\varvec{{\mathcal {M}}}_{\varvec{M}}}\)-resolvent fixed-point problem characterization

$$\begin{aligned} ({\varvec{\widetilde{{\widetilde{{{\mathcal {P}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}}}}}) \ \left\{ \begin{array}{l} \text{ Find } \ {{\varvec{s}}_{\varvec{M}}} \in {\varvec{\mathcal {D}}}({\varvec{\widetilde{A}}_{\varvec{M}}} + {\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}})) \subset {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{M}}}}: \\ \text{ for } \ {{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\widetilde{A}}_{\varvec{M}}} ({{\varvec{s}}_{\varvec{M}}}), \\ {{\varvec{s}}_{\varvec{M}}} = {{\varvec{F}}^{\varvec{r}}_{{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}}} ({{\varvec{s}}_{\varvec{M}}}). \end{array} \right. \end{aligned}$$

Then by the Banach fixed-point theorem, such a fixed-point problem has a unique solution since the 1/m-firm contraction resolvent property implies the contraction of the operator \({{\varvec{F}}^{\varvec{r}}_{{\varvec{s}}^{{\varvec{*}}}_{\varvec{M}}}}: {\varvec{{\mathcal {D}}}}({\varvec{\widetilde{A}}_{\varvec{M}}} + {\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}})) \rightarrow {\varvec{{\mathcal {D}}}}({\varvec{\widetilde{A}}_{\varvec{M}}} + {\varvec{\partial }} (G_M \circ \Lambda _{M_{w_{g}}}))\), for \(r > (a - m) / \alpha \ge 0\), with contraction parameter \(1/m (a - r\alpha ) < 1\). Finally, in the same fashion, the dual existence result case is demonstrated. \(\square \)

Therefore, the following solvability result is lastly concluded.

Corollary 6

Under the duality principle compatibility condition of Lemma 4 and the fixed-point existence qualifying condition of Theorem 5, the evolution macro-hybrid mixed constrained boundary-value problems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{M}}})\) attain a solution, with respective unique primal and dual solution component.

5 Multidomain two-phase dual mixed filtration with seawater intrusion

In this section, we present and analyze the mixed variational coastal aquifer flow component of the hydrological surface-subsurface system of the paper. Once we give a qualitative characterization of the Darcian mixed pressure–velocity evolution flow, we consider an adaptation of the two phase fractional mixed flow model treated by Chen and Ewing (1999), Chen (2001), that amount to an innovative evolution variational coastal aquifer approach of a stationary total velocity-global pressure and an evolution wetting velocity-complementary pressure coupled underground flow system. For more details we refer to our study (Alduncin 2015).

5.1 The qualitative mixed physical model

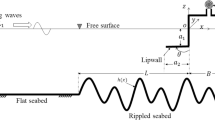
Let \(\Omega _D \subset \Re ^3\) denote the spatial configuration of an open coastal aquifer, a bounded connected three-dimensional domain with a Lipschitz boundary \(\partial \Omega _D\), which corresponds to the Darcian domain component of the material continuous mechanical coupled Darcy/Stokes (D/S) flow system (1) described in Sect. 2. For this subsystem, we shall consider the decomposed Darcian (D) aquifer boundary \(\partial \Omega _D\) components (see Fig.1):

$$\begin{aligned} \begin{array}{lcl} \partial \Omega _{D_{im}} &{} = &{} \ \text{ the } \text{ impervious } \text{ part }, \\ \partial \Omega _{D_{fw}} &{} = &{}\ \text{ the } \text{ part } \text{ under } \text{ surface } \text{ water } \ ( \text{ the } \text{ interface } \text{ pair } \ \Gamma _{D/S}), \\ \partial \Omega _{D_{sw}} &{} = &{} \ \text{ the } \text{ part } \text{ in } \text{ contact } \text{ with } \text{ seawater }, \\ \partial \Omega _{D_{oa}} &{} = &{} \ \text{ the } \text{ part } \text{ in } \text{ contact } \text{ with } \text{ open } \text{ air } \ \text{(the } \text{ seepage } \text{ face) }. \end{array} \end{aligned}$$
(14)
Fig. 1
figure 1

A section of an open coastal aquifer and surface reservoirs

Full size image

Thereby, under the assumption of immobile air and seawater phases, the aquifer flow problem amounts to analyze the evolution of the fresh water flow in the wet set \(\Lambda \subset Q_D = \Omega _D \times (0, T)\). Thus, relative to \(\partial \Omega _D\), the wet set of sub-boundaries for \(\partial \Lambda \subset \partial Q_D = \partial \Omega _D \times (0, T)\) turn out to be:

$$\begin{aligned} \begin{array}{lcl} \Sigma _{D_{im}} &{} = &{} \partial \Lambda \cap \partial Q_{D_{im}} \, \\ \Sigma _{{fw}} &{} = &{} \partial \Lambda \cap \partial Q_{D_{fw}} (= \partial \Lambda \cap \partial Q_S \ \text{ the } \text{ evolutionary } \text{ interface } \text{ pair } \ \Gamma _{D/S}), \\ \Sigma _{D_{sw}} &{} = &{} \partial \Lambda \cap \partial Q_{D_{sw}} \, \\ \Sigma _{D_{oa}} &{} = &{} \partial \Lambda \cap \partial Q_{oa} \ \text{(the } \text{ evolutionary } \text{ seepage } \text{ face) }, \\ \Gamma _{D_{sfb}} &{} = &{} \text{ the } \text{ saturation } \text{ interface } \text{ free } \text{ boundary } \ \subset Q_D \, \\ \Gamma _{D_{ifb}} &{} = &{} \text{ the } \text{ fresh } \text{ and } \text{ seawater } \text{ interface } \text{ free } \text{ boundary } \ \subset Q_D. \end{array} \end{aligned}$$
(15)

For the aquifer incompressible flow process, we introduce the parameters: \(\rho _f\) and \(\rho _s\), the constant aquifer fresh water and seawater mass densities, such that \(\rho _s> \rho _f > 0\), and its negative hydraulic relation \(\rho \equiv 1 - \rho _s / \rho _f < 0\). Further, the time-varying ordinate levels \(\widehat{h} \in \{\widehat{h}_1, \widehat{h}_2,..., \widehat{h}_{n_r}\}\) of the \(n_r\) fresh water surface coupled reservoirs, relative to the fixed sea level as the origin (\(y = 0\)), and the bottom lowest ordinates of the jth reservoir \(\widehat{b}_j\) are given (see Figure 1).

On the other hand, according to Alduncin (2013), we introduce the conditions

$$\begin{aligned} \begin{array}{lcl} (\textbf{H1}) \ \ \ \widehat{b}_j &{} \ge &{} \widehat{h}_j / \rho , \ j = 1, 2,...,n_r \, \\ (\textbf{H2}) \ \ \ n_y &{} \equiv &{} \text{ grad } y \ {\varvec{\cdot }} \ {{\varvec{n}}} = 0 \, \ \text{ on } \ \Sigma _{D_i} \, \end{array} \end{aligned}$$
(16)

under which, the fundamental modeling constraints: seawater intrusion cannot be in contact with the fresh water surface reservoirs, and that the (possibly empty) impervious flow boundary \(\Sigma _{D_{im}} = \partial \Lambda \cap \partial \Omega _{D_{im}}\) be geometrically vertical, are guaranteed. Then, the maximum principle applies for the Darcian pressure functions \(p^*_D\) and \(p^*_D - (\rho - 1)y\) in \(\Lambda \), and then the flow pressure field is bounded from below in accordance with

$$\begin{aligned} p^*_D > \varphi ^* \equiv max \ \Big (0, (\rho - 1) y \Big ) = \left\{ \begin{array}{ll} 0 \, &{} y \ge 0 \, \\ (\rho - 1) y, &{} y < 0 \, \end{array} \right. \ \ \text{ in } \ \Lambda . \end{aligned}$$
(17)

Thereby, the coastal aquifer flow domain is characterized by

$$\begin{aligned} Q_D = \Lambda ^+ \cup \Lambda \cup \Lambda ^{-} \left\{ \begin{array}{l} \Lambda ^+ = [p^*_D = 0] \equiv \{(x, y, z, t) \in Q_D: p^*_D(x, y, z, t) = 0 \}, \\ \Lambda \ \ = [p^*_D> \varphi ^*] \equiv \{(x, y, z, t) \in Q_D: p^*_D(x, y, z, t) > \varphi \}, \\ \Lambda ^- = [p^*_D = (\rho - 1) y] \equiv \{(x, y, z, t) \\ \hspace{1.0cm} \in Q_D: p^*_D(x, y, z, t) = (\rho - 1) y \}, \end{array} \right. \end{aligned}$$
(18)

and consequently the following qualitative result can be concluded (Alduncin 2013).

Theorem 7

Any solution system \((p^*_D, {{\varvec{u}}_{\varvec{D}}}, \Lambda )\) of the evolution filtration problem may be extended to all of \(Q_D\) in pressure and Darcian velocity by

$$\begin{aligned} p^*_D + \{(1 - \rho )H_0(p^*_D) + \rho H_0(p^*_D - \varphi ^*_{Q_D})\} y = \left\{ \begin{array}{ll} 0 \, &{} \text{ in } \ \Lambda ^+ \, \\ p^*_D + y, &{} \text{ in } \ \Lambda \, \\ 0 \, &{} \text{ in } \ \Lambda ^- \, \end{array} \right. \end{aligned}$$
(19)

and

$$\begin{aligned} \begin{array}{l} {{\varvec{u}}_{\varvec{D}}} = - {{\varvec{grad}}} \ p^*_D - \{(1 - \rho )H_0(p^*_D) + \rho H_0(p^*_D - \varphi ^*_{Q_D})\} {{\varvec{grad}}} \ y \\ \hspace{0.6cm} = \left\{ \begin{array}{ll} \textbf{0} \, &{} \text{ in } \ \Lambda ^+ \, \\ - {{\varvec{grad}}} \ (p^*_D + y), &{} \text{ in } \ \Lambda \, \\ \text{0 } \, &{} \text{ in } \ \Lambda ^- \, \end{array} \right. \end{array} \end{aligned}$$
(20)

respectively, where \(H_0\) denotes the Heaviside function, and \(\varphi ^*_{Q_D}\) is the continuous extension to \(Q_D\) of the obstacle function in (17), by zero in \(\Lambda ^+\) and by \((\rho - 1)y\) in \(\Lambda ^-\).

Lastly, we introduce the pressure (hydraulic charge) boundary conditions of this qualitative model

$$\begin{aligned} \begin{array}{ll} p^*_D + y = \widehat{h}, &{} \text{ on } \ \Sigma _{D_{fw}} (= \Gamma _{D/S} = \partial \Omega _D \cap \partial \Omega _S), \\ p^*_D + y = \rho y, &{} \text{ on } \ \Sigma _{D_{sw}} \cup \Gamma _{D_{ifb}} \, \\ p^*_D + y = y, &{} \text{ on } \ \Sigma _{D_{oa}} \cup \Gamma _{D_{sfb}} \, \end{array} \end{aligned}$$
(21)

as well as the complementary impervious boundary condition, and the seepage flux constraint

$$\begin{aligned} \begin{array}{ll} {{\varvec{u}}_{\varvec{D}}} \ \mathbf{\cdot } \ \textbf{n} \ = \ 0, &{} \text{ on } \ \Sigma _{D_{im}} \cup \Gamma _{D_{ifb}} \, \\ {{\varvec{u}}_{\varvec{D}}} \ \mathbf{\cdot } \ \textbf{n} \ \ge \ 0 \, &{} \text{ on } \ \Sigma _{D_{oa}}. \end{array} \end{aligned}$$
(22)

Additionally, we introduce the prescribed initial conditions

$$\begin{aligned} \Lambda = \Lambda _0 \subset \Omega _D, \ \text{ at } \ t = 0, \ \ \text{ and } \ \ p^*_D(0) = \widehat{p}^*_{D_0}, \ \text{ in } \ \Lambda _0 \, \end{aligned}$$
(23)

assuming that \(\widehat{p}^*_{D_0} > \varphi ^* \equiv max \ (0, (\rho - 1)y)\) in \(\Lambda _0\).

In our previous work (Alduncin 2013), utilizing qualitative incompressible Darcian flow properties (17)–(20) and boundary-value/initial conditions (21)–(23), the classical single-phase primal evolution mixed variational coastal flow model was treated extending its formulation from the flow wet set domain \(\Lambda \subset Q_D = \Omega _D \times (0, T)\) to the whole domain \(Q_D\), à la Baiocchi Baiocchi et al. (1973). In the following subsections, following Alduncin (2015), we generate and analyze a general three-dimensional evolution mixed variational two-phase flow model which results to be, indeed, more suitable for: macro-hybridization, variational macro-hybrid mixed finite element approximations, parallel proximal-point algorithms as well as proximation semi-implicit time marching schemes (cf.Alduncin 2015, Sects. 3 and 4).

5.2 The fractional two-phase mixed physical model

Next, we proceed to apply the abstract macro-hybridized evolution constrained boundary-value problems formulated and analyzed in Sects.  3 and 4, to a two-phase dual mixed physical version of the coastal aquifer model described qualitatively in the previous subsection.

Let us consider an immiscible two-phase Darcian incompressible flow, neglecting the effect of evaporation and assigning to the atmospheric pressure a zero value, without mass transfer. We shall denote a wetting phase by \(\alpha = w\) and a nonwetting phase by \(\alpha = n\), in the whole aquifer space-time domain \(Q_D = \Omega _D \times (0, T)\). Hence, for a nonhomogeneous anisotropic coastal aquifer, we have with the dependent \(\alpha \)-phase variables of velocity \({{\varvec{u}}}_{D_\alpha }\), pressure \(p^*_{D_\alpha }\) and saturation \(s_{D_\alpha }\), the governing constitutive and mass-conservation mixed equations:

$$\begin{aligned} \left. \begin{array}{l} \displaystyle { {{\varvec{u}}}_{D_\alpha } = - \frac{\kappa _{r_{D_\alpha }}(s_{D_\alpha })}{\mu _{D_\alpha }} {{\varvec{K}}} ( {{\varvec{grad}}} \ p^*_{D_\alpha } - \rho _{D_\alpha } {{\varvec{g}}} ), } \\ \displaystyle { \phi \frac{\partial (\rho _{D_\alpha } s_{D_\alpha })}{\partial t} + div (\rho _{D_\alpha } {{\varvec{u}}}_{D_\alpha }) = \rho _{D_\alpha } \widehat{q}_{D_\alpha }(s_{D_{\alpha }}), } \end{array} \right\} \ \text{ in } \ Q_D. \end{aligned}$$
(24)

Here, as hydraulic parameters we have: \({{\varvec{K}}}\), the symmetric positive definite absolute permeability tensor, \(\kappa _{r_{D_\alpha }}\), \(\mu _{D_\alpha }\), \(\rho _{D_\alpha }\) and \(\widehat{q}_{D_\alpha }\), the \(\alpha \)-phase relative permeability, viscosity, mass density and volumetric flow rate, respectively. Further, \(\phi \) stands as the porosity of the media and \({{\varvec{g}}}\) is the gravity acceleration vector.

Additionally, as complementary equations to model (24), we have the flow volume balance constraint and the capillary pressure relation

$$\begin{aligned} \begin{array}{ll} s_{D_w} + s_{D_n} &{} = \ 1 \, \\ p^*_{D_c}(s_{D_w}) &{} = \ p^*_{D_n} - p^*_{D_w}. \end{array} \end{aligned}$$
(25)

\(\bullet \) The two-phase fractional flow model.

Next, we reformulate physical two-phase model (24), (25), as a fractional one: a coupled mixed model of a stationary total velocity-global pressure \({{\varvec{u}}_{\varvec{D}}}\)-\(p^*_D\) and an evolutionary wetting velocity-complementary pressure \({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}\)-\(\theta ^*_D\); due to Chen and Ewing (1999), Arbogast (1992):

$$\begin{aligned} \begin{array}{ll} {{\varvec{u}}_{\varvec{D}}} &{} = \ {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + {{\varvec{u}}_{{\varvec{D}}_{\varvec{n}}}} \, \\ p^*_D &{} \displaystyle { = \ p^*_{D_n} - \int ^{s_{D_w}}_0 \bigg (f_{D_w} \frac{\partial p^*_{D_c}}{\partial s_{D_w}}\bigg )(s) \ ds \, } \\ \theta ^*_D &{} \displaystyle { = \ - \int ^{s_{D_w}}_0 \bigg (f_{D_w} f_{D_n} \frac{\partial p^*_{D_c}}{\partial s_{D_w}}\bigg ) (s) \ ds \, } \end{array} \end{aligned}$$
(26)

where \(f_{D_\alpha } (s_{D_w}) = \lambda _{D_\alpha } / \lambda _D\) stand as \(\alpha \)-phase fractional flow functions, \(\alpha \in \{w, n\}\), defined in terms of the phase mobilities \(\lambda _{D_\alpha } = \kappa _{r_{D_\alpha }}/\mu _{D_\alpha }\) and total mobility \(\lambda _D = \lambda _{D_w} + \lambda _{D_n}\).

Thereby, the dual mixed fractional flow system corresponds to

$$\begin{aligned} \left. \begin{array}{l} \lambda _D^{-1}(\theta ^*_D) {{\varvec{K}}}^{-1} {{\varvec{u}}_{\varvec{D}}} = - {{\varvec{grad}}} \ p^*_D - {\varvec{\gamma }}_1(\theta ^*_D), \\ div \ {{\varvec{u}}_{\varvec{D}}} = \widehat{q}(\theta ^*_D); \\ \\ \lambda _D^{-1}(\theta ^*_D) {{\varvec{K}}}^{-1} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} = - {{\varvec{grad}}} \ \theta ^*_D - {\varvec{\gamma }}_2(\theta ^*_D), \\ \displaystyle {\frac{\partial \theta ^*_D}{\partial t} + div \ {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} = \widehat{q}_D(\theta ^*_D), } \end{array} \right\} \ \text{ in } \ Q_D \, \end{aligned}$$
(27)

with \(\widehat{q}_D = \widehat{q}_{D_w} + \widehat{q}_{D_{n}}\) the total volumetric flow rate, and

$$\begin{aligned} \ \ \ \ \ {\varvec{\gamma }}_1 = - f_{D_w} {{\varvec{grad}}} \ p^*_{D_c} + \int ^{s_{D_w}}_0 {{\varvec{grad}}} \bigg (f_{D_w} \frac{\partial p^*_{D_c}}{\partial s_{D_w}} \bigg ) (s) ds - (f_{D_w} \rho _{D_w} + f_{D_n} \rho _{D_n}) {{\varvec{g}}_{\varvec{D}}} \, \end{aligned}$$
$$\begin{aligned} \begin{array}{l} \displaystyle { {\varvec{\gamma }}_2 = f_{D_w} {{\varvec{grad}}} \ p^*_D - f_{D_w} {{\varvec{grad}}} \ p^*_{D_c} + f_{D_w} \int ^{s_{{D_w}}}_0 {{\varvec{grad}}} \bigg (f_{D_w} \frac{\partial p^*_{D_c}}{\partial s_{D_w}} \bigg ) (s) \ ds } \\ \hspace{0.8cm} \displaystyle { + \int ^{s_{D_w}}_0 {{\varvec{grad}}} \bigg (f_{D_w} f_{D_n} \frac{\partial p^*_{D_c}}{\partial s_w}\bigg ) (s) \ ds - f_{D_w} \rho _{D_w} {{\varvec{g}}_{\varvec{D}}}. } \end{array} \end{aligned}$$

We lastly note that the nonwetting phase velocity is related to complementary pressure \(\theta ^*_D\) by

$$\begin{aligned}{} & {} {{\varvec{u}}_{{\varvec{D}}_{\varvec{n}}}} = {{\varvec{K}}} (\lambda (\theta ^*_D) {{\varvec{grad}}} \ \theta ^*_D - \lambda _{D_n}(\theta ^*_D) {{\varvec{grad}}} \ p^*_D + {\varvec{\gamma }}_3(\theta ^*_D)) \, \nonumber \\{} & {} \displaystyle { {\varvec{\gamma }}_3 = - \lambda _{D_n} \int ^{s_{D_w}}_0 {{\varvec{grad}}} \bigg (f_{D_w} \frac{\partial p^*_{D_c}}{\partial s_{D_w}}\bigg ) (s) \ ds } \nonumber \\{} & {} \quad \quad \quad \displaystyle { + \lambda _D \int ^{s_{D_w}}_0 {{\varvec{grad}}} \bigg (f_{D_w} f_{D_n} \frac{\partial p^*_{D_c}}{\partial s_w}\bigg ) (s) \ ds } + \ \lambda _{D_n} \rho _{D_n} {{\varvec{g}}_{\varvec{D}}}. \end{aligned}$$
(28)

5.3 Variational macro-hybridized wetting velocity-complementary pressure models

We next proceed to apply the abstract general macro-hybridized and macro-hybrid primal-dual variational models \(({\varvec{\mathcal {M}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}})\)-\(({\varvec{{\mathcal {M}}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}})\)-\(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{{\varvec{M}}}})\) of Sect. 3, to the evolutionary component of fractional two-phase flow system \((27)_2\). The complementary instantaneous component of flow system \((27)_1\), could be treated in a similar manner, but we shall concentrate here only on the evolutionary flow case.

Toward this end we apply the duality procedures of Alduncin (2005), Alduncin (2010) (also, see Alduncin 2009), taking into account the pressure and velocity boundary conditions and constraints of the aquifer system, (21) and (22), expressed subdifferentially as variational inclusions for the wetting-velocity and complementary-pressure fields \({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}\) and \(\theta ^*_D\), as follows:

$$\begin{aligned} \begin{array}{ll} \delta ^*_{im} \theta ^*_D \in \partial \psi _{im} (\pi _{im} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) = \partial I_{\{0_{im}\}}(\pi _{im} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}), &{} \text{ in } \ B^*(\partial \Omega _{im}) \, \\ \delta ^*_{fw} \theta ^*_D \in \partial \psi _{fw}(\pi _{fw} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) = \{\widehat{h}(t) - y\}, &{} \text{ in } \ B^*(\partial \Omega _{fw}), \\ \delta ^*_{sw} \theta ^*_D \in \partial \psi _{sw}(\pi _{sw} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) = \{\rho y - y\}, &{} \text{ in } \ B^*(\partial \Omega _{sw}) \, \\ \delta ^*_{oa\cup sfb} \theta ^*_D \in \partial \psi _{oa}(\pi _{oa\cup sfb} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) = \{0_{oa\cup sfb}\}, &{} \text{ in } \ B^*(\partial \Omega _{oa\cup sfb}) \, \end{array} \end{aligned}$$
(29)

with the seepage flux constraint

$$\begin{aligned} \delta ^*_{oa} \theta ^*_D \in \partial \psi _{oa}(\pi _{oa} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) = \partial I_{\{u_{D_\nu } \ge 0_{oa}\}}(\pi _{oa} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}), \ \ \text{ in } \ B^*(\partial \Omega _{oa}). \end{aligned}$$
(30)

5.3.1 Variational macro-hybridized primal evolution initial/boundary-value problem

Acordingly, the primal evolution variational macro-hybridized complementary pressure-wetting velocity model turns out to be

$$\begin{aligned} ({{\varvec{\mathcal {M}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{D}}}}}) \left\{ \begin{array}{l} \text{ Given } \ {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} \in {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\widehat{q}}_{\varvec{D}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \text{ find } \ ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) \in {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{D}}}: \\ {{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.0cm} \in \displaystyle {\frac{{{\varvec{d}} \varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{{{\varvec{dt}}}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}} \varvec{\partial } \varvec{\Psi }_{\varvec{D}} \varvec{\circ } \varvec{\pi }_{\varvec{D}}}) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.4cm} + \ ({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{D}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{D}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{D}}}}}) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \hspace{1.4cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}(0) = {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} , \\ - {{\varvec{grad}}_{\varvec{D}}} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} \in \lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \end{array} \right. \end{aligned}$$

where the explicit variational external boundary composition subdifferential primal component is given by

$$\begin{aligned} ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{D}}} \circ {\varvec{\pi }_{\varvec{D}}}) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}= & {} \Big ({\varvec{\pi }^{\varvec{T}}_{\varvec{im}}} {\varvec{\partial } {\varvec{I}}_{\{{\varvec{0}}_{\varvec{im}}\}}} \circ {\varvec{\pi }_{\varvec{im}}}) + ({\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{\partial } {\varvec{I}}_{ \{\varvec{\widehat{h}}{\varvec{(t)}} - {\varvec{y}}\}}} \circ {\varvec{\pi }_{\varvec{fw}}}) \nonumber \\{} & {} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{\partial } {\varvec{I}}_{\{\varvec{\rho } {\varvec{y}} - {\varvec{y}}\}}} \circ {\varvec{\pi }_{\varvec{sw}}}\Big ) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} \nonumber \\{} & {} + \ {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} + {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}}, \ \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}}. \end{aligned}$$
(31)

Moreover, from macro-hybrid composition duality result (4) of Lemma 1, the macro-hybrid version of problem \({{\varvec{(}}{\mathcal { M}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) is obtained.

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}}) \left\{ \begin{array}{l} \text{ Given } \ {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} \in {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\widehat{q}}_{\varvec{D}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ {\varvec{\widehat{\theta }}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \text{ find } \ ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}) \in {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{D}}}}: \\ {{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{D}}}} {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \\ \hspace{1.0cm} \in \displaystyle {\frac{{{\varvec{d}} \varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{{{\varvec{dt}}}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}} \varvec{\partial \Psi }_{\varvec{D}} \varvec{\circ \pi }_{\varvec{D}}}) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.4cm} - \ {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \hspace{1.4cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}(0) = {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} , \\ - {{\varvec{grad}}_{\varvec{D}}} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} \in \lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \end{array} \right. \end{aligned}$$

synchronized by the internal boundary \({\varvec{\Gamma }_{\varvec{D}}}\)-transmission subpotential subdifferential dual problem

$$\begin{aligned} ({\varvec{\widetilde{\mathcal {T}}}^*_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \in {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{D}}}}: \\ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{D}}}} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}\ \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{D}}}} {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \, \ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}. \end{array} \right. \end{aligned}$$

5.3.2 Variational macro-hybridized dual evolution initial/boundary-value problem

Next, as the dual version of primal evolution problem \(({\varvec{{\mathcal {M}}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\), we have the following one.

$$\begin{aligned} ({\varvec{{\mathcal {M}}^*_\mathcal{M}\mathcal{H}}_{\varvec{D}}}) \left\{ \begin{array}{l} \text{ Given } \ {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} \in {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\widehat{q}}_{\varvec{D}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \text{ find } \ ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}}: \\ - {{\varvec{grad}}_{\varvec{D}}} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} \\ \hspace{1.0cm} \in \lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{D}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}} \varvec{\partial } \varvec{\Psi }_{\varvec{D}} \varvec{\circ } \varvec{\pi }_{\varvec{D}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.3cm} - \ {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{\Gamma }_{\varvec{D}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{D}}}} \circ {\varvec{\pi }_{{\varvec{\Gamma }_{\varvec{D}}}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.3cm} + \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{D}}}} , \\ {{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \in \displaystyle {\frac{\partial {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{\partial t}} - {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \hspace{2.3cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}(0) = {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} , \\ \end{array} \right. \end{aligned}$$

where the explicit variational external boundary composition subdifferential primal component is given by (cf. (31))

$$\begin{aligned}{} & {} ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}}} {\varvec{\partial \Psi }_{\varvec{D}}} \circ {\varvec{\pi }_{\varvec{D}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \nonumber \\{} & {} \quad = (\varvec{\pi }^{\varvec{T}}_{\varvec{im}} \varvec{\partial } {\varvec{I}}_{\{{\varvec{\widehat{u}}_{{\varvec{D}}}}_{{{{\varvec{w}}}}_{\varvec{\nu }}} \varvec{\ge } {\varvec{0}}_{\varvec{oa}}\}} \circ {\varvec{\pi }_{\varvec{im}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} + {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}}, \ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}}. \end{aligned}$$
(32)

Furthermore, as before, from macro-hybrid composition duality Lemma 1, the macro-hybrid version of dual macro-hybridized problem \({({\varvec{\mathcal {M}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}}})\) is obtained.

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\mathcal {D}}}}) \left\{ \begin{array}{l} \text{ Given } \ {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} \in {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\widehat{q}}_{\varvec{D}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}}, \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{D}}}) \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \text{ find } \ ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}}: \\ - {{\varvec{grad}}_{\varvec{D}}} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{D}}}} {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \\ \hspace{1.0cm} \in \lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}} \varvec{\partial \Psi }_{\varvec{D}} \varvec{\circ \pi }_{\varvec{D}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.3cm} - \ {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} + \ {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ {{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \in \displaystyle {\frac{\partial {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{\partial t}} - {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ \hspace{2.3cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}(0) = \varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}} , \\ \end{array} \right. \end{aligned}$$

synchronized as in the primal case by the internal boundary \({\varvec{\Gamma }_{\varvec{M}}}\)-transmission subpotential dual inclusion problem

$$\begin{aligned} ({\varvec{\widetilde{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \in {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}}: \\ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{D}}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \ \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{D}}}} {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}} \, \ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}}. \end{array} \right. \end{aligned}$$

5.3.3 Variational primal and dual evolution resolvent fixed-point solvability results

For evolutionary wetting velocity-complementary pressure primal and dual mixed variational systems, macro-hybridized \(({\varvec{{\mathcal {M}}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) and \(({\varvec{{\mathcal {M}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}}})\) problems, as well as macro-hybrid \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{D}}})\) problems, in the context of Sect. 4 on resolvent fixed-point solvability analysis, we have the following existence qualitative results. Indeed, taking into account the corresponding macro-hybrid domain interior conditions \(({{\varvec{C}}_{(\varvec{\partial \widetilde{F}}_{{\varvec{D}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{D}}}}}} {\varvec{-div}}^{\varvec{T}}_{\varvec{D}})}}\Big )\) and \(({{\varvec{C}}_{{{\varvec{G}}_{\varvec{D}}}, {\varvec{div}}_{\varvec{D}}}}\Big )\), as well as the strong monotonicity subpotential subdifferential operator conditions \(({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{D}}}})\) and \(({{\varvec{C}}_{\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{D}}}})\), we conclude:

Theorem 8

Let the macro-hybrid compatibility conditions \(({{\varvec{C}}_{{\varvec{G}}_{\varvec{D}}, {\varvec{div}}_{\varvec{D}}}}\Big )\), \(({\varvec{C}}_(\varvec{\partial } \varvec{\widetilde{F}}_{{\varvec{D}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{D}}}}}}, -{\varvec{div}}^{\varvec{T}}_{\varvec{D}})\Big )\), as well as the qualifying conditions \(({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{D}}}})\), \( ({{\varvec{C}}_{\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{D}}}})\), be satisfied. Then for dual initial condition regularity

$$\begin{aligned} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}} \, \end{aligned}$$
(33)

evolution macro-hybridized primal problem \(({\varvec{{\mathcal {P}}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) and dual problem \(({\varvec{{\mathcal {D}}^{{\varvec{*}}}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) have a unique solution.

Moreover, as a conclusive result we have the following one.

Corollary 9

Under the conditions of Lemma 4 on primal and dual duality principles, and those of fixed-point existence Theorem 5, evolution macro-hybrid mixed constrained initial/boundary-value problems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{D}}})\) possess a unique solution.

6 Multidomain evolution mixed incompressible stokes surface flow system

In this section, we treat the surface fresh water reservoirs of the hydraulic coupled coastal aquifer system of the paper. We shall consider incompressible surface flows modeled by the stress-stretching constitutive equation

$$\begin{aligned} {{\varvec{T}}} = - p {{\varvec{I}}} + 2 \mu {{\varvec{D}}}, \end{aligned}$$
(34)

where \({{\varvec{D}}}\) stands as the streching tensor, symmetric component of the velocity gradient, and for the spherical stress \(p {{\varvec{I}}}\), p denotes the pressure field whose expanded stress-velocity form may be expressed by

$$\begin{aligned} - {{\varvec{grad}}^{\varvec{T}}} \ {{\varvec{v}}^{{\varvec{*}}}}= - 1 / \mu \ {{\varvec{T}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}} \ {{\varvec{v}}^{{\varvec{*}}}}, \end{aligned}$$
(35)

where the parameter \(\mu \ge 0\) corresponds to the constant shear viscosity of the fluid, (cf. Gurtin et al. 2010, Chapter 46).

In addition, we shall consider the complementary non-convective momentum balance motion equation

$$\begin{aligned} \displaystyle { \rho \frac{\partial {{\varvec{v}}^{{\varvec{*}}}}}{\partial t} } = div \ {{\varvec{T}}} + {{\varvec{b}}}, \end{aligned}$$
(36)

with a body force \({{\varvec{b}}}\). We recall that the velocity material change rate, has as spatial description the convective acceleration field \(\rho \frac{\partial {{\varvec{v}}^{{\varvec{*}}}}}{\partial t} + \rho ({{\varvec{grad}}} \ {{\varvec{v}}^{{\varvec{*}}}}) {{\varvec{v}}^{{\varvec{*}}}}\) (Gurtin et al. 2010).

Next, we shall formulate and analyze Stokes flow model (34)–(36), variationally in the sense of multidomain evolution mixed constrained initial/boundary-value problems of Sect. 3.

6.1 Multidomain primal and dual evolution macro-hybrid mixed stokes surface flow systems

In preparation for the surface-subsurface interface transmission analysis of Sect. 8, we consider the primal and dual evolution macro-hybrid mixed velocity-stress variational incompressible Stokes system models, whose macro-hybridized versions are stated as follows:

$$\begin{aligned} ({\varvec{{\mathcal {M}}_\mathcal{M}\mathcal{H}}_{\varvec{S}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {{\varvec{b}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{S}}}) \subset {\varvec{\mathcal {V}}_{{\varvec{MH}}_{\varvec{S}}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \in {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \, \\ \text{ find } \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \in {\varvec{\mathcal {W}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \ \text{ and } \ {{\varvec{T}}} \in {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \, \\ {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{T}}} \in \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial \Psi }_{\varvec{S}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{s}}} \\ \hspace{1.9cm} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{S}}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} - {{\varvec{b}}}, \ \text{ in } \ {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} \, \\ \hspace{2.0cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \, \\ - {{\varvec{grad}}^{\varvec{T}}_{\varvec{S}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} = - 1 / \mu \ {{\varvec{T}}_{\varvec{e}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} \, \end{array} \right. \end{aligned}$$

where the external boundary primal composition subdifferential has the explicit form

$$\begin{aligned} \begin{array}{l} ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{{S}}}} \circ {\varvec{\pi }_{\varvec{S}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} = ({\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{D}}}} {\varvec{\partial } {\varvec{I}}_{\{\varvec{\widehat{v}}^{{\varvec{*}}}_{\varvec{S}}\}}} \circ {\varvec{\pi }_{{\varvec{S}}_{\varvec{D}}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} + {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{s}}}, \ \text{ in } \ {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{S}}}} \; \end{array} \end{aligned}$$
(37)

and whose dual evolution macro-hybrid mixed alternative flow system is given by

$$\begin{aligned} ({\varvec{{\mathcal {M}}^*_\mathcal{M}\mathcal{H}}_{\varvec{S}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {{\varvec{b}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{S}}}) \subset {\varvec{\mathcal {V}}_{{\varvec{MH}}_{\varvec{S}}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \in {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \text{ find } \ {{\varvec{T}}} \in {\varvec{\mathcal {Y}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \ \text{ and } \ {{\varvec{{v}}}^{{\varvec{*}}}_{S}} \in {\varvec{{\mathcal {W}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \subset {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} : \\ - {{\varvec{grad}}^{\varvec{T}}_{\varvec{S}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \in - 1 / \mu \ {{\varvec{T}}_{\varvec{e}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \\ \hspace{2.6cm} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}} \varvec{\partial } \varvec{\Psi }_{{\varvec{S}}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{T}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{v}}^{{\varvec{*}}}_{\varvec{S}}} \\ \hspace{2.6cm} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{\varvec{S}}} \varvec{\circ } \varvec{\pi }_{{\varvec{S}}}}) {{\varvec{T}}} \ \text{ in } \ {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{T}}} \in \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} - {{\varvec{b}}}, \ \text{ in } \ {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{2.2cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

with an explicit external boundary primal composition subdifferential

$$\begin{aligned} \begin{array}{l} ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } \varvec{\Psi }_{{\varvec{S}}} \varvec{\circ } \varvec{\pi }_{{\varvec{S}}}}) {{\varvec{T}}} = ({\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{D}}}} {\varvec{\partial } {\varvec{I}}_{\{\varvec{\widehat{s}}_{\varvec{S}}\}}} \circ {\varvec{\pi }_{{\varvec{S}}_{\varvec{D}}}}) {{\varvec{T}}} + {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{v}}^{{\varvec{*}}}_{\varvec{S}}}, \ \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{S}}}}. \end{array} \end{aligned}$$
(38)

On the other hand, the macro-hybrid versions of such macro-hybridized evolution Stokes problems follow as before from the macro-hybrid composition duality result (4) of Lemma 1:

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{\mathcal {S}}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {{\varvec{b}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{S}}}) \subset {\varvec{\mathcal {V}}_{{\varvec{MH}}_{\varvec{S}}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \in {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \text{ find } \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \in {\varvec{\mathcal {W}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \subset {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \ \text{ and } \ {{\varvec{T}}} \in {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} : \\ {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{T}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{S}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}} \in \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } \varvec{\Psi }_{\varvec{S}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{s}}} - {{\varvec{b}}}, \\ \ \text{ in } \ {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{4.0cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} , \\ - {{\varvec{grad}}^{\varvec{T}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} = - \ 1 / \mu \ {{\varvec{T}}_{\varvec{e}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} ; \end{array} \right. \end{aligned}$$

as well as the dual macro-hybrid variational version

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\mathcal {S}}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {{\varvec{b}}} \in {\varvec{{\mathcal {R}}}} ({{\varvec{grad}}_{\varvec{S}}}) \subset {\varvec{\mathcal {V}}_{{\varvec{MH}}_{\varvec{S}}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \in {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \text{ find } \ {{\varvec{T}}} \in {\varvec{\mathcal {Y}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \ \text{ and } \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \in {\varvec{{\mathcal {W}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} \subset {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} : \\ - {{\varvec{grad}}^{\varvec{T}}_{\varvec{S}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{S}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}} \\ \hspace{1.0cm} \in - 1 / \mu \ {{\varvec{T}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \\ \hspace{1.5cm} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } \varvec{\Psi }_{\varvec{S}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{T}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{v}}^{{\varvec{*}}}_{\varvec{S}}} \, \ \text{ in } \ {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{T}}} \in \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} - {{\varvec{b}}}, \ \text{ in } \ {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{2.2cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

synchronized both systems by the internal boundary \({\varvec{\Gamma _{S}}}\)-transmission subpotential dual subdifferential inclusion problem

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{S}}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}} \in {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{S}}}}}: \\ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{S}}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \ \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{S}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}} \, \ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{S}}}}}. \end{array} \right. \end{aligned}$$

6.2 Evolution resolvent fixed-point solvability results for primal \(({\varvec{{\mathcal {M}}_\mathcal{M}\mathcal{H}}_{\varvec{S}}})\) and dual \(({\varvec{{\mathcal {M}}^{{\varvec{*}}}_\mathcal{M}\mathcal{H}}_{S}})\) problems

Existence-uniqueness results for primal and dual evolution macro-hybrid mixed Stokes problems, once again, in accordance with Lemma 4, Theorem 5 and Corollary 6 established in Sect. 4, correspond to the domain interior conditions \(({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}_{{\varvec{S}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{S}}}}}} -{\varvec{grad}}^{\varvec{T}}_{\varvec{S}})}}\Big )\), \(({{\varvec{C}}_{{{\varvec{G}}_{\varvec{S}}}, {\varvec{-grad}}^{\varvec{T}}_{\varvec{S}}}}\Big )\), and to the strong monotonicity subpotential subdifferential operator conditions \(({{\varvec{C}}_{\varvec{\partial } \varvec{\widetilde{F}}_{\varvec{S}}}})\), \(({{\varvec{C}}_{\varvec{\partial } {\varvec{G}}^{\varvec{{\varvec{*}}}}_{\varvec{S}}}})\).

Lemma 10

Under compatibility conditions \(\Big ({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{{\varvec{MH}}_{\varvec{M}}}}}}, -{\varvec{div}}^{\varvec{T}}_{\varvec{M}})}}\Big )\) and \(({{\varvec{C}}_{{{\varvec{G}}_{\varvec{M}}}, {{\varvec{div}}_{\varvec{M}}}}})\), Stokes evolution macro-hybridized primal problem \(({\varvec{\mathcal {P}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{S}}}})\) and dual problem \(({\varvec{{\mathcal {D}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{S}}}}})\) are equivalent in a solvability sense to macro-hybrid incompressible Stokes problems \(({\varvec{\mathcal{M}\mathcal{H}}_{{\varvec{S}}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{{\varvec{S}}}})\), respectively.

Lastly, we have the conclusive result.

Theorem 11

Let compatibility conditions of Lemma 10, as well as the monotonicity qualifying conditions \(({{\varvec{C}}_{\varvec{\partial \widetilde{F}}_{M}}})\) and \( ({\varvec{C}}_{\varvec{\partial } {\varvec{G}}^{{\varvec{*}}}_{\varvec{M}}})\) be fulfilled. Then for regular primal initial condition

$$\begin{aligned} {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} \in {{\varvec{H}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{S}}}}} \, \end{aligned}$$
(39)

macro-hybrid mixed constrained initial/boundary-value incompressible Stokes problems \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{S}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{S}}})\) possess a unique solution.

7 Dual evolution macro-hybrid mixed transport systems

We continue with the transport model applicable to both the underground Darcian and free Stokeisian flow mechanical coupled systems of the coastal aquifer. For \(M \in \{D, S\}\), we shall consider transport processes with a distributed contaminant M-chemical solute, modeled in terms of mixed fields of a primal scalar mass concentration \({{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}\) and a dual vector flux \({{{\varvec{d}}_{\varvec{M}}}} = - {{\varvec{D}}_{{{\varvec{u}}_{\varvec{M}}}}}\) \({{\varvec{grad}}} \ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}\), and a distributed intrinsic mass concentration control primal vector field \({{\varvec{s}}_{\varvec{M}}}\). Here, \({{\varvec{D}}_{{{\varvec{u}}_{\varvec{M}}}}}\) denotes the diffusion-dispersion tensor dependent on the flow M-velocity vector field \({{{\varvec{u}}_{\varvec{M}}}}\) (cf., e.g., de Marsil 1986). In addition the field \({\varvec{\widehat{f}}_{\varvec{M}}}\) will be a corresponding given contaminant source.

Then, related to the multidomain \(\Omega _{M} = \bigcup ^{E_{M}}_{e=1} \Omega _{M_{e}}\), \((2)_1\), in the context of Sect. 2, local mass conservation principles for the concentration field \(c^*_{M_e}\) are established as follows. For instantaneous material connected parts of each M-subdomain \(\Omega _{M_e}\), \({{\mathcal {P}}}_{M_{e_t}}(x)\), surrounding any point \(x \in \Omega _{M_e}\) at a time \(t \in (0, T)\), the Reynolds’ transport theorem states that local mass balances must hold true (cf. e.g., Gurtin et al. 2010):

$$\begin{aligned} \displaystyle { \frac{d}{dt} \int _{{{\mathcal {P}}}_{M_{e_t}}} c^*_{M_e} \ d\Omega _{M_e} }&= \displaystyle { \int _{{{\mathcal {P}}}_{M_{e_t}}} \frac{d c^*_{M_e}}{d t} + div (\{{{\varvec{u}}_{{\varvec{M}}_{\varvec{e}}}} \ c^*_{M_e}) \ d\Omega _{M_e} } \nonumber \\&= \displaystyle { - \int _{{{\mathcal {P}}}_{M_{e_t}}} s_{M_e} \ d\Omega _{M_e} + \int _{{{\mathcal {P}}}_{M_{e_t}}} \widehat{f}_{M_e} \ d\Omega _{M_e} } \nonumber \\&\quad \displaystyle { - \int _{\partial {{\mathcal {P}}}_{M_{e_t}}} {{\varvec{d}}_{{{\varvec{M}}_{\varvec{e}}}}} {\varvec{\cdot }} {{\varvec{n}}_{{\varvec{M}}_{\varvec{e}}}} \ d\partial \Omega _{M_e}. } \end{aligned}$$
(40)

Hence, applying the divergence theorem to (40)-boundary term and a continuous integral localization, the following dual evolution mixed flux-concentration M-multidomain physical transport model (utilizing a boldface compact notation) is achieved.

$$\begin{aligned} \left. \begin{array}{rcl} {{{\varvec{d}}_{\varvec{M}}}} &{} = &{} - {{\varvec{D}}_{{\varvec{u}}_{\varvec{M}}}} {{\varvec{grad}}_{\varvec{M}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}, \\ \displaystyle { \frac{ d {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} }{d t} } &{} + &{} {{\varvec{u}}_{\varvec{M}}} {\varvec{\cdot }} {{\varvec{grad}}_{\varvec{M}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{div}}_{\varvec{M}}} {{\varvec{u}}_{\varvec{M}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} + {{\varvec{div}}_{\varvec{M}}} {{{\varvec{d}}_{\varvec{M}}}} \\ &{} = &{} - {{{\varvec{s}}_{\varvec{M}}}} + {\varvec{\widehat{f}}_{\varvec{M}}} , \\ {{\varvec{s}}_{\varvec{M}}} &{} \in &{} {\varvec{\partial \varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \ \Leftrightarrow \ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\partial } \varvec{\varphi }_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{s}}_{\varvec{M}}} , \end{array} \right\} \ \text{ in } \ \Omega _M \times (0, T). \end{aligned}$$
(41)

Here, subpotential subdifferential \({\varvec{\partial \varphi _{\tau _{M}}}}\) denotes a primal distributed local M-control mechanism for the intrinsic mass concentration control, the transport constraint of the process, with an inverse M-control mechanism \({\varvec{\partial \varphi ^*_{\tau _{M}}}}\), subdifferential of the conjugate functional \(\varphi ^*_{\tau _M}\) (cf. Duvaut and Lions 1972).

Importantly, in contrast to the usual primal approach (cf. Alduncin 2019), here we shall be considering a dual evolution transport formulation that results to be more suitable for semi-implicit time marching schemes, and associated iterative proximation algorithms (cf. Alduncin 1997, 2007b).

7.1 Macro-hybrid mixed variational dual evolution transport systems

As a constrained initial/boundary-value problem, localized macro-hybrid mixed constrained M-transport system (41) has the following variational dual evolution macro-hybridized formulation (cf. Alduncin 2007b, a).

$$\begin{aligned} ({\varvec{\mathcal {M}}^{{\varvec{*}}}_{{\varvec{\tau }}_{\varvec{\mathcal{M}\mathcal{H}}_{{\varvec{M}}}}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {\varvec{\widehat{f}}_{\varvec{M}}} \in {\varvec{{\mathcal {R}}}} (- {{\varvec{div}}_{\varvec{\tau _{M}}} }) \subset {\varvec{{\mathcal {Y}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}}, \ {{\varvec{\widehat{c}}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}} ,\\ \text{ find } \ {{\varvec{d}}_{\varvec{M}}} \in {\varvec{{\mathcal {V}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}} \ \text{ and } \ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {X}}^{{\varvec{*}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}} :\\ {{\varvec{div}}^{\varvec{T}}_{{\varvec{\tau }}_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \in {{\varvec{D}}^{\varvec{-1}}_{{\varvec{u}}_{{\varvec{M}}}}} {{\varvec{d}}_{\varvec{M}}} + ({{\varvec{\pi }}^{\varvec{T}}_{{\varvec{M}}}} {{\varvec{\partial }} \varvec{\Psi }_{\varvec{M}}} \circ {{\varvec{\pi }}_{{\varvec{M}}}}) {{\varvec{d}}_{\varvec{M}}} - {{\varvec{\pi }}^{\varvec{T}}_{{\varvec{\tau }}_{{\varvec{N}}_{\varvec{M}}}}} {{\varvec{\widehat{c}}}^{{\varvec{*}}}_{\varvec{M}}}, \\ + ({{\varvec{\pi }}^{\varvec{T}}_{{{\varvec{\Gamma }}_{\varvec{M}}}}} {{\varvec{\partial }} {\varvec{\mathcal {I}}}_{{\varvec{Q}}_{{\varvec{M}}}}} \circ {{\varvec{\pi }}_{{{\varvec{\Gamma }}_{\varvec{M}}}}}) {{\varvec{d}}_{\varvec{M}}} \, \text{ in } \ {\varvec{\mathcal {V}}^{{\varvec{*}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}} ,\\ - {{\varvec{div}}_{{\varvec{\tau }}_{\varvec{M}}} } {{\varvec{d}}_{\varvec{M}}} \in \displaystyle {\frac{d {{\varvec{c}}^{{\varvec{*}}}_{{\varvec{M}}}}}{d t} + {{\varvec{u}}_{{\varvec{M}}}} \cdot {{\varvec{grad}}_{{\varvec{\tau }}_{{\varvec{M}}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} } + {{\varvec{div}}_{{\varvec{\tau }}_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \\ + {{\varvec{\partial }} \varvec{\varphi }^{{\varvec{*}}}_{{\varvec{\tau }}_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\widehat{f}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_{{\varvec{\tau }}_{{\varvec{MH}}_{\varvec{M}}}}}, \\ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}(0) = {{\varvec{\widehat{c}}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \, \end{array} \right. \end{aligned}$$

with an explicit variational external boundary composition subdifferential component

$$\begin{aligned} \begin{array}{l} ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{{\varvec{M}}}} \circ {\varvec{\pi }_{{\varvec{M}}}}) {{\varvec{d}}_{{\varvec{M}}}} = ({\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{{\varvec{D}}_{{\varvec{M}}}}}} {\varvec{\partial } {\varvec{\mathcal {I}}}_{\{\varvec{\widehat{d}}_{{\varvec{n}}_{{\varvec{M}}}}\}}} \circ {\varvec{\pi }_{\varvec{\tau }_{{\varvec{D}}_{\varvec{M}}}}}) {\varvec{d}_{{\varvec{M}}}} + {\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{{\varvec{N}}_{{\varvec{M}}}}}} {\varvec{\widehat{c}}^{{\varvec{*}}}_{\varvec{M}}} , \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} . \end{array} \end{aligned}$$
(42)

Once again, applying the macro-hybrid composition duality result (4) of Lemma 1, the macro-hybrid version of dual evolution transport problem \(({\varvec{\mathcal {M}}^*_{\varvec{\tau }_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{M}}}}})\) turns out to be as follows.

$$\begin{aligned} ({{\varvec{\mathcal{M}\mathcal{H}}}^{{\varvec{*}}}_{{{\varvec{\tau }}}_{{\varvec{M}}}}}) \ \left\{ \begin{array}{ll} \text{ Given } \ {\varvec{\widehat{f}}_{\varvec{M}}} \in {\varvec{{\mathcal {R}}}} (- {{\varvec{div}}_{\varvec{\tau }_{\varvec{M}}} }) \subset {\varvec{{\mathcal {Y}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}}, \ {\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{ Z}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} , \\ \text{ find } \ {{\varvec{d}}_{\varvec{M}}} \in {\varvec{{\mathcal {V}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} \ \text{ and } \ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{\mathcal {X}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} : \\ {{\varvec{div}}^{\varvec{T}}_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{\varvec{\Gamma }_{\varvec{M}}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}} \\ \hspace{1.0cm} \in {{\varvec{D}}^{\varvec{-1}}_{{\varvec{u}}_{\varvec{M}}}} {{\varvec{d}}_{\varvec{M}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{M}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{M}}} \circ {\varvec{\pi }_{\varvec{M}}}) {{\varvec{d}}_{\varvec{M}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{{\varvec{N}}_{\varvec{M}}}}} {\varvec{\widehat{c}}^{{\varvec{*}}}_{\varvec{M}}} , \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} , \\ - {{\varvec{div}}_{\varvec{\tau }_{\varvec{\alpha }}} } {{\varvec{d}}_{\varvec{M}}} \in \displaystyle {\frac{d {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}}{d t} + {{\varvec{u}}_{\varvec{M}}} \cdot {{\varvec{grad}}_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} } + {{\varvec{div}}_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} \\ \hspace{2.7cm} + {\varvec{\partial } \varvec{\varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}} - {\varvec{\widehat{f}}_{\varvec{M}}} \, \text{ in } \ {\varvec{{\mathcal {Y}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}} , \\ {{\varvec{c}}^{{\varvec{*}}}_{\varvec{M}}}(0) = {\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

synchronized by the macro-hybrid evolution subpotential subdifferential dual problem

$$\begin{aligned} ({\varvec{\mathcal {T}}^*_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}} \in {\varvec{{\mathcal {B}}^{{\varvec{*}}}}_{{{\varvec{MH}}_{\varvec{\tau }_{{\varvec{M}}}}}}}: \\ {\varvec{\pi }_{\varvec{\tau }_{\varvec{\Gamma }_{{\varvec{M}}}}}}{{\varvec{d}}_{\varvec{M}}} \ \in {\varvec{\partial } {\varvec{I}}_{{\varvec{Q}}^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}} \, \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{\tau }_{{\varvec{M}}}}}}} , \end{array} \right. \end{aligned}$$

which implements the local internal boundary \({\varvec{\Gamma }_{{\varvec{M}}}}\)-transmission multidomain transport constraints.

Next, applying the resolvent fixed-point solvability analysis of Sect. 4, to the dual evolution mixed flux-concentration transport system, modeled by the equivalent macro-hybridized and macro-hybrid variational problems \(({\varvec{\mathcal {M}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) and \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }}}_{\varvec{M}})\), we identify the primal macro-hybrid domain interior condition \(({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{\varvec{\tau }{{\varvec{MH}}_{\varvec{M}}}}}}} {{\varvec{div}}^{\varvec{T}}}_{\varvec{\tau }_{\varvec{M}}})}}\Big )\) as well as the strong monotonicity subpotential subdifferential dual operator condition \(({{\varvec{C}}_{\varvec{\partial \varphi ^*}_{\varvec{\tau }_{{\varvec{M}}}}}})\), concluding the following existence results:

Theorem 12

Let the macro-hybrid qualifying condition \(({{\varvec{C}}_{\varvec{\partial \varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}}})\) and the compatibility condition \(\Big ({{\varvec{C}}_{(\varvec{\partial } \varvec{\widetilde{F}}_{{\varvec{M}}_ {/_{{\varvec{V}}_{\varvec{\tau }{{\varvec{MH}}_{\varvec{M}}}}}}} {\varvec{div}}^{\varvec{T}}_{\varvec{\tau }_{\varvec{M}}})}}\Big )\) be satisfied. Then for regular dual initial conditions in the sense

$$\begin{aligned} {\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{M}}_{\varvec{0}}}} \in {{\varvec{Z}}^{{\varvec{*}}}_{{\varvec{\tau }_{{\varvec{MH}}_{\varvec{M}}}}}} \, \end{aligned}$$
(43)

evolution macro-hybridized dual tranport problem \(({\varvec{\mathcal {D}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{\mathcal{M}\mathcal{H}}}_{\varvec{M}}}}})\) has a unique solution;

then the following result is achieved.

Corollary 13

Under the condition (4) of Lemma 1 on a dual duality principle, and the condition of fixed-point existence Theorem 5, in a transport sense, dual evolution macro-hybrid mixed constrained initial/boundary-value problem \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }}}_{\varvec{M}})\) possesses a solution with a unique dual component.

We lastly note that due to qualifying condition \(({{\varvec{C}}_{\varvec{\partial \varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}}})\), the dual constraints of the transport processes should be governed by strongly monotone intrinsic M-control concentration maximal monotone mechanisms \({\varvec{\partial \varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{M}}}}\) (Duvaut and Lions 1972).

8 Multidomain evolution mixed incompressible darcy/stokes surface-subsurface filtration transport coupled systems

In this last section, we formulate and analyze the continuum mechanical coupling of the physical filtration transport Darcy/Stokes incompressible flow models of the coastal aquifer system under consideration. We shall treat the primal and dual evolution macro-hybrid mixed Darcian two phase wetting velocity-complementary pressure and Stokesian velocity-stress variational initial/boundary-value flow problems of Subsect. 5.3 and Subsect. 6.1, as well as the transport dual one of Subsect. 7.1. We will discuss the implementation of the corresponding mass flux-traction-velocity interface transmission conditions in the sense of dual subpotential composition subdifferential Lagrangian constraints.

8.1 Surface-subsurface transport flow interface transmission conditions

As it is natural for the coupling of subsystems in multiphysics, the basic interface transmission fields for filtration transport flow coupled processes are the \({{\varvec{d}}_{\varvec{M}}}\)-fluxes, \({{\varvec{s}}_{\varvec{M}}}\)-tractions and \({{\varvec{v}}_{\varvec{M}}}\)-velocities, \({\varvec{\nu }_{\varvec{M}}}\)-normal and \({\varvec{\tau }_{\varvec{M}}}\)-tangential components, for \(M\in \{D,S\}\).

Hence, on the surface-subsurface Lipschitz interface \(\Gamma _{DS} = \partial \Omega _D \cap \partial \Omega _S\) of the coastal aquifer phenomena under investigation, we have the

\(\bullet \) Darcy/Stokes flux-traction-velocity transmission fields.

$$\begin{aligned} \begin{array}{rlcrl} d_{\nu _M} = &{} {{\varvec{d}}_{\varvec{M}}} {\varvec{\cdot }} {\varvec{\nu }_{\varvec{M}}} &{} \text{ and } &{} {{\varvec{d}}_{\varvec{\tau }_{\varvec{M}}}} &{} = \ ({{\varvec{I}}} - {\varvec{\nu }_{\varvec{M}}} {\varvec{\otimes }} _{\varvec{\nu }_{\varvec{M}}}) {{\varvec{d}}_{\varvec{M}}} \, \\ {{\varvec{s}}_{\varvec{S}}} = &{} {{\varvec{T}}} {\varvec{\nu }_{\varvec{S}}} &{} \text{ and } &{} {{\varvec{s}}_{\varvec{\tau }_{\varvec{S}}}} &{} = \ ({{\varvec{I}}} - {\varvec{\nu }_{\varvec{S}}} {\varvec{\otimes }} {\varvec{\nu }_{\varvec{S}}}) {{\varvec{s}}_{\varvec{S}}} \, \\ v_{\nu _M} = &{} {{\varvec{v}}_{\varvec{M}}} {\varvec{\cdot }} {\varvec{\nu }_{\varvec{M}}} &{} \text{ and } &{} {{\varvec{v}}_{\varvec{\tau }_{\varvec{M}}}} &{} = \ ({{\varvec{I}}} - {\varvec{\nu }_{\varvec{M}}} {\varvec{\otimes }} _{{\varvec{\nu }_{\varvec{M}}}}) {{\varvec{v}}_{\varvec{M}}} \, \end{array} \end{aligned}$$
(44)

where \({{\varvec{T}}}\) stands as the stress field defined by incompressible (34) Stokes fluid constitutivity.

Based on transmission fields (44), the surface-subsurface interface constraints of the transport flow processes should resemble mechanical continuity conditions. For instance, as natural interface constraints we have the following ones:

$$\begin{aligned} \left. \begin{array}{rcll} d_{\nu _D} &{} = &{} - d_{\nu _S} , &{} (\text{ normal } \text{ fluxes } \text{ continuity}), \\ {\varvec{\theta }^{{\varvec{*}}}_{\varvec{\nu }_{\varvec{D}}}} \equiv \theta ^*_D {\varvec{\nu }_{\varvec{D}}} &{} = &{} - {{\varvec{s}}_{\varvec{S}}} \equiv - {{\varvec{T}}} {\varvec{\nu }_{\varvec{S}}} , &{} (\text{ tractions } \text{ continuity}), \\ v_{\nu _D} &{} = &{} - v_{\nu _S} , &{} (\text{ normal } \text{ velocities } \text{ continuity}), \\ {{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}} &{} = &{} - {{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}} , &{} (\text{ tangential } \text{ velocities } \text{ continuity}), \end{array} \right\} \ \text{ on } \ \Gamma _{DS}. \end{aligned}$$
(45)

Taking into account the experimental work of Beavers-Joseph-Saffman on interface conditions (Beavres and Joseph 1967; Saffman 1971), the tangential velocities constraint \((45)_4\) should be modified in the sense (Cimolin 2013)

$$\begin{aligned} {{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}} = - 2\mu G ({{\varvec{I}}} - {\varvec{\nu }_{\varvec{S}}} {\varvec{\otimes }} {\varvec{\nu }_{\varvec{S}}}) {{\varvec{D}}} {\varvec{\nu }_{\varvec{S}}} \, \ \ (\text{ BJS-tangential } \text{ surface } \text{ velocity}) \ \text{ on } \ \Gamma _{DS}, \end{aligned}$$
(46)

with \(G > 0\) an experimental constant.

8.2 Mixed Darcy/stokes variational interface transmission constraints

Next, towards a variational macro-hybrid formulation of surface-subsurface transmission conditions (44), (45), related to the global spatial disjoint decomposition (1) of Sect. 2

$$\begin{aligned} \begin{array}{rcll} {\overline{\Omega }} &{} = &{} {\overline{\Omega }}_D \cup {\overline{\Omega }}_S , &{} \text{ the } \text{ subsurface-surface } \text{ media }, \\ \Gamma _{DS} &{} = &{}\partial \Omega _D \cap \partial \Omega _S , &{} \text{ the } \text{ subsurface-surface } \text{ interface }, \end{array} \end{aligned}$$

we shall consider the special M-multidomain nonoverlapping interface-noninterface (int-nonint) decompositions:

$$\begin{aligned} \begin{array}{rcll} \Omega _{M_{int}} &{} = &{} \displaystyle { \bigcup ^{E_{int}}_{e =1} \Omega _ {M_e} } , &{} \hbox {the} M \hbox {-interface multidomain}, \\ \Omega _{M_{nonint}} &{} = &{} \displaystyle {\bigcup ^{E_M}_{e=E_{int + 1}} \Omega _ {M_e} } , &{} \hbox {the} M\hbox {-noninterface multidomain}, \\ \Omega _M &{} = &{} \Omega _{M_{int}} \cup \Omega _{M_{nonint}} , &{} \hbox {the} M\hbox {-multidomain}, \\ \Gamma _{DS} &{} = &{} \partial \Omega _{D_{int}} \cap \partial \Omega _{S_{int}}, &{} \text{ the } \text{ Darcy/Stokes } \text{ interface }. \end{array} \end{aligned}$$
(47)

Thereby, as a central requirement for matching interface traces, we introduce the interface multidomain condition

$$\begin{aligned} ({{\varvec{C}}_{\varvec{\partial }_{{\varvec{D}}_{\varvec{int}}},\varvec{\partial }_{{\varvec{S}}_{\varvec{int}}}}}) \ \ \ \partial \Omega _{D_{int}} \cap \Gamma _{DS} \ = \ \Gamma _{DS} \cap \partial \Omega _{S_{int}}.\end{aligned}$$

In this manner, for compatible conforming local interface mechanical flux-traction-velocity transmissions, we have the conditions, for \(e=1,...,E_{int}\),

$$\begin{aligned} \left. \begin{array}{rcll} \{d_{\nu _{D_e}}\} &{} = &{} - \{d_{\nu _{S_e}}\}, &{} (\text{ fluxes } \text{ continuity}), \\ \{{\varvec{\theta }^{{\varvec{*}}}_{\varvec{\nu }_{{\varvec{D}}_{\varvec{e}}}}}\} &{} = &{} - \{{{\varvec{s}}_{{\varvec{S}}_{\varvec{e}}}}\}, &{} (\text{ tractions } \text{ continuity}), \\ \{v_{\nu _{D_e}}\} &{} = &{} - \{v_{\nu _{S_e}}\}, &{} (\text{ normal } \text{ velocities } \text{ continuity}), \\ \{{{\varvec{v}}_{\varvec{\tau }_{{\varvec{D}}_{\varvec{e}}}}}\} &{} = &{} - \{{{\varvec{v}}_{\varvec{\tau }_{{\varvec{S}}_{{\varvec{BJS}}_{\varvec{e}}}}}}\}, &{} (\text{ tangential } \text{ velocities } \text{ continuity}), \end{array} \right\} \ \text{ on } \ \Gamma _{DS} , \end{aligned}$$
(48)

where \(\{- {{\varvec{v}}_{\varvec{\tau }_{{\varvec{s}}_{{\varvec{BJS}}_{\varvec{e}}}}}}\}\) stands for the Beavers-Joseph-Saffman surface local interface tangential velocity, (46).

Hence, adapting the framework functional notation of Sect. 2 to special M-multidomain decompositions (47), we introduce the stationary primal and dual reflexive Banach M-interface spaces \({\varvec{\widetilde{V}}_{\varvec{M}}} = \prod ^{E_{int}}_ {e=1} V(\Omega _ {M_e})\) and \({\varvec{\widetilde{Y}}^{{\varvec{*}}} _{\varvec{M}}} = \prod ^{E_{int}}_ {e=1} Y^*(\Omega _ {M_e})\), with respective duals \({\varvec{\widetilde{V}}^{{\varvec{*}}}_{\varvec{M}}} = \prod ^{E_{int}}_ {e=1} V^*(\Omega _ {M_e})\) and \({\varvec{\widetilde{Y}}_{\varvec{M}}} = \prod ^{E_{int}}_ {e=1} Y(\Omega _ {M_e})\). Similarly, we introduce the \(\Gamma _{DS}\)-interface boundary reflexive Banach space \({\varvec{\widetilde{B}}_{\varvec{M}}} \equiv \prod ^{E_{int}}_{e=1} B_M (\Gamma _{{DS}_e})\) and its dual \({\varvec{\widetilde{B}}^{{\varvec{*}}}_{\varvec{M}}} \equiv \prod ^{E_{int}}_{e=1} B^*_M(\Gamma _{{DS}_e})\), as well as the corresponding interface linear continuous primal trace operator \({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{DS}}}}\), assuming that it satisfies the compatibility condition

$$\begin{aligned} \big ({{\varvec{C}}_{\varvec{\pi }_{\varvec{\Gamma }_{\varvec{DS}}}}}\big ) \ \ \ {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{DS}}}}} \in {{\mathcal {L}}} \big ({\varvec{\widetilde{V}}_{\varvec{M}}}, {\varvec{\widetilde{B}}_{\varvec{M}}}\big ) \ \text{ is } \text{ surjective }. \end{aligned}$$

Further, as evolution reflexive Banach spaces, for \(2 \le p < \infty \) and \(q^* = p / (p - 1)\), we have primal and dual M-interface spaces \({\varvec{\widetilde{{{\mathcal {V}}}}}_{\varvec{M}}} = {{\varvec{L}}^{\varvec{p}}}(0,T; {\varvec{\widetilde{V}}_{\varvec{M}}})\) with dual \({\varvec{\widetilde{{{\mathcal {V}}}}}^{{\varvec{*}}}_{\varvec{M}}} = {{\varvec{L}}^{{\varvec{q}}^{{\varvec{*}}}}}(0,T; {\varvec{\widetilde{V}}^{{\varvec{*}}}_{\varvec{M}}})\), and \({\varvec{\widetilde{{{\mathcal {Y}}}}}^{{\varvec{*}}}_{\varvec{M}}} = {{\varvec{L}}^{\varvec{p}}}(0,T; {\varvec{\widetilde{Y}}^{{\varvec{*}}}_{\varvec{M}}})\) with dual \({\varvec{\widetilde{{{\mathcal {Y}}}}}_{\varvec{M}}} = {{\varvec{L}}^{{\varvec{q}}^{{\varvec{*}}}}}(0,T; {\varvec{\widetilde{Y}}_{\varvec{M}}})\), and as evolution interface boundary spaces we have \({\varvec{\widetilde{{{\mathcal {B}}}}}_{\varvec{M}}} = {{\varvec{L}}^{\varvec{p}}}(0,T; {\varvec{\widetilde{B}}_{\varvec{M}}})\) with dual \({\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{\varvec{M}}} = {{\varvec{L}}^{{\varvec{q}}^{{\varvec{*}}}}}(0,T; {\varvec{\widetilde{B}}^{{\varvec{*}}}_{\varvec{M}}})\).

Having stated the M-interface reflexive Banach frameworks, we define the product flux-traction-velocity transmission continuity solution subspaces, for \(e=1,...,E_{int}\)  

$$\begin{aligned} {\varvec{\widetilde{{{\mathcal {T}}}}}_{\varvec{fl}}} = \Big \{\big ({\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}}\big ) ({{\varvec{d}}_{\varvec{\nu }_{\varvec{D}}}}, {{\varvec{d}}_{\varvec{\nu }_{\varvec{S}}}}) \ \in {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{S}}}}: \ \nonumber \\ {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}} {{\varvec{d}}_{\varvec{\nu }_{\varvec{D}}}} = - {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}} {{\varvec{d}}_{\varvec{\nu }_{\varvec{S}}}}\Big \}, \nonumber \\ {\varvec{\widetilde{{{\mathcal {T}}}}}_{\varvec{tr}}} = \Big \{\big ({\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}}\big ) ({{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {{{\varvec{s}}_{\varvec{S}}}}) \ \in {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{tr}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{tr}}_{\varvec{S}}}}: \ \nonumber \\ {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}} {{\varvec{\theta }^{{\varvec{*}}}_{\varvec{\nu }_{\varvec{D}}}}} = - {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}} {{{\varvec{s}}_{\varvec{S}}}}\Big \}, \nonumber \\ \varvec{\widetilde{{\mathcal {T}}}_{{{\varvec{v}}}_{\varvec{\nu }}}} = \Big \{\big ({\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}}\big ) ({{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}) \ \in {\varvec{\widetilde{\mathcal {B}}}_{{{\varvec{v}}}_{{\varvec{\nu }}_{\varvec{D}}}}} \times {\varvec{\widetilde{\mathcal {B}}}_{{{{\varvec{v}}}_{{\varvec{\nu }}_{\varvec{S}}}}}}: \ \nonumber \\ {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}} {{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}} = - {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}} {{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}} \Big \}, \nonumber \\ {\varvec{{\widetilde{\mathcal {T}}}}_{{{\varvec{v}}}_{\varvec{\tau }}}} = \Big \{\big ({\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}}\big ) ({{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {{{\varvec{v}}_{\varvec{\tau }_{{\varvec{S}}_{\varvec{BJS}}}}}}) \ \in {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}} \times {\varvec{\widetilde{\mathcal {B}}}_{{{\varvec{v}}\varvec{\tau }}_{\varvec{S}}}}: \ \nonumber \\ {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{D}}_{\varvec{DS}}}}} {{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}} = - {\varvec{\pi }_{\varvec{\Gamma }_{{\varvec{S}}_{\varvec{DS}}}}} {{{\varvec{v}}_{\varvec{\tau }_{{\varvec{S}}_{\varvec{BJS}}}}}}\Big \}. \end{aligned}$$
(49)

Now, we are in a position to implement such interface transmissions by means of Lagrange multipliers, product dual trace fields solutions of subpotential subdifferential variational dual problems, in the sense of the variational macro-hybrid internal boundary transmission dual component of the general primal and dual evolution internal/boundary-value problems \(({\varvec{{\mathcal {M}}}_{\varvec{M}}})\) and \(({\varvec{{\mathcal {M}}}^{{\varvec{*}}}_{\varvec{M}}})\) of Sect. 3

$$\begin{aligned} ({\varvec{{\mathcal {T}}^{{\varvec{*}}}_\mathcal{M}\mathcal{H}}_{\varvec{M}}}) \left\{ \begin{array}{ll} \text{ Find } \ {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \in {\varvec{{\mathcal {B}}^{{\varvec{*}}}}_{{{\varvec{MH}}_{\varvec{M}}}}}: \\ {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{M}}}} {{\varvec{u}}_{\varvec{M}}} \ \in {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{M}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{M}}} \, \ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{M}}}}}. \end{array} \right. \end{aligned}$$

8.3 Mixed Darcy/stokes variational macro-hybrid coupled systems

For the multidomain variational dual evolution macro-hybrid mixed constrained coupled transport system \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{D}}}})\)-\( ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{S}}}})\) of Subsect. 7.1, we have the subpotential subdifferential variational dual problem for the transport flux interface product Lagrange multiplier \(({\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{D}}}}}, {{\varvec{\widetilde{\mu }}}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{S}}}}})\),

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{{\varvec{f}}{\varvec{l}}}}) \ \ \ \left\{ \begin{array}{l} \text{ Find } \ ({{\varvec{\widetilde{\mu }}}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{D}}}}}, {\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{fl}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{fl}}_{\varvec{S}}}} , \\ \text{ satisfying } \text{ the } \text{ dual } \text{ flux } \ {\varvec{\Gamma }_{\varvec{DS}}}-\text{ tranmission } \text{ condition } \\ \big ({\varvec{\pi }_{{\varvec{D}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}, {\varvec{\pi }_{{\varvec{S}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}\big ) ({{\varvec{d}}_{{\varvec{D}}}}, {{\varvec{d}}_{{\varvec{S}}}}) \\ \hspace{1.0cm} \in {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{\varvec{fl}}}} ({\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{D}}}}}, {\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{S}}}}}), \ \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{S}}}} , \end{array} \right. \end{aligned}$$

where \({\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{f}}{\varvec{l}}}}\) is the transport flux continuity transmission subspace defined by \((49)_1\).

Concerning the dual-primal evolution variational macro-hybrid mixed constrained coupled incompresssible flow system \(({\varvec{{\mathcal {M}}^{{\varvec{*}}}_\mathcal{M}\mathcal{H}}_{\varvec{D}}})\)-\(({\varvec{{\mathcal {M}}_\mathcal{M}\mathcal{H}}_{\varvec{S}}})\) of Subect. 5.4.2 and Subect. 6.1, for a traction interface product Lagrange multiplier \(({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{D}}}}}, ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}})\), we consider the subdifferential dual problem

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{tr}}}) \ \ \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{D}}}}}, {\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{tr}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{tr}}_{\varvec{S}}}} , \\ \text{ satisfying } \text{ the } \text{ dual } \text{ traction } \ {\varvec{\Gamma }_{\varvec{DS}}}-\text{ tranmission } \text{ condition } \\ \big ({\varvec{\pi }_{{\varvec{D}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}, {\varvec{\pi }_{{\varvec{S}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}\big ) ({\varvec{\theta }^{{\varvec{*}}}_{{\varvec{D}}}}, {{{\varvec{T}}}}) \\ \hspace{1.0cm} \in {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{tr}}}}} ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{D}}}}}, ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}}), \ \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{tr}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{tr}}_{\varvec{S}}}} , \end{array} \right. \end{aligned}$$

with \({\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{tr}}}}\) standing as the traction continuity transmission subspace \((49)_2\).

Similarly, in the case of the dual-primal evolution constrained coupled incompresssible flow system \(({\varvec{\mathcal {M}}^{{\varvec{*}}}_{{\varvec{\mathcal{M}\mathcal{H}}}_{{\varvec{D}}}}})\)-\(({\varvec{{\mathcal {M}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{S}}}}})\) of subsections 5.3.2 and 6.1, we have the variational dual problems, with the normal velocity and tangential velocity interface product Lagrange multipliers \(({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{{\varvec{D}}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}})\) and \(({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{{\varvec{D}}_{\varvec{e}}}}}} {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{{\varvec{S}}_{\varvec{e}}}}}})\) respectively:

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{{\varvec{v}}_{\varvec{\nu }}}}) \ \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{{\varvec{v}}_{{\varvec{\nu }}_{\varvec{D}}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{{\varvec{v}}_{{\varvec{\nu }}_{\varvec{S}}}}}} , \\ \text{ satisfying } \text{ the } \text{ dual } \text{ normal } \text{ velocity } \ {\varvec{\Gamma }_{\varvec{DS}}}-\text{ tranmission } \text{ condition } \\ \big ({\varvec{\pi }_{{{\varvec{D}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}, {\varvec{\pi }_{{{\varvec{S}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}\big ) ({{{\varvec{v}}_{{\varvec{\nu }_{\varvec{D}}}}}}, {{{\varvec{v}}_{{\varvec{\nu }_{\varvec{S}}}}}}) \\ \hspace{1.0cm} \in {\varvec{\partial }{\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{v}}_{\varvec{\nu }}}}} ({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}), \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{{\varvec{v}}_{{\varvec{\nu }}_{\varvec{D}}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{{\varvec{v}}_{{\varvec{\nu }}_{\varvec{S}}}}}} , \end{array} \right. \end{aligned}$$
$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{{{\varvec{v}}_{\varvec{\tau }}}}}) \ \ \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{{\varvec{v}}_{{\varvec{\tau }}_{\varvec{D}}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{{\varvec{v}}_{{\varvec{\tau }}_{\varvec{S}}}}}} , \\ \text{ satisfying } \text{ the } \text{ dual } \text{ tangential } \text{ velocity } \ {\varvec{\Gamma }_{\varvec{DS}}}-\text{ tranmission } \text{ condition } \\ \big ({\varvec{\pi }_{{{\varvec{D}}_{{\varvec{\tau }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}, {\varvec{\pi }_{{{\varvec{S}}_{{\varvec{\tau }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}\big ) ({{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {{\varvec{v}}_{\varvec{\tau }_{{\varvec{S}}_{\varvec{BJS}}}}}) \\ \hspace{1.0cm} \in {\varvec{\partial }{\varvec{I}}_{\varvec{\widetilde{{\mathcal {T}}}}_{{\varvec{v}}_{\varvec{\tau }}}}} ({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}} {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}), \ \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{{\varvec{v}}_{{\varvec{\tau }}_{\varvec{D}}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{{\varvec{v}}_{{\varvec{\tau }}_{\varvec{S}}}}}}, \end{array} \right. \end{aligned}$$

\({\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{v}}_{\varvec{\nu }}}}\) and \({\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{v}}_{\varvec{\tau }}}}\) being the normal and tangential velocity continuity transmission subspaces \((49)_3\) and \((49)_4\).

Therefore, in this manner, the surface-subsurface mechanical transmission conditions of the local filtration transport incompressible flow processes, in the coastal aquifer, are implemented as subpotential maximal monotone subdifferential inclusions, which, importantly, permit their incorporation as primal variational coupling prescribed constraints via compositional dualization (cf. Alduncin 2007b, Lemma 2.1).

8.3.1 Variational multidomain dual mixed transport coupled system \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{D}}}}})\)-\( ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{S}}}}})\)

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{{\varvec{\tau }_{\varvec{D}}-\varvec{\tau }_{\varvec{S}}}}}) \ \left\{ \begin{array}{l} \text{ Given } \ ({\varvec{\widehat{f}}_{\varvec{D}}}, {\varvec{\widehat{f}}_{\varvec{S}}}) \in {\varvec{\mathcal {Y}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}}\times {\varvec{\mathcal {Y}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}}, \\ ({\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}}, {\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}}) \in {{\varvec{Z}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}}\times {{\varvec{Z}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}} , \\ \text{ find } \ ({{{\varvec{d}}_{\varvec{D}}}}, {{{\varvec{d}}_{\varvec{S}}}}) \in {\varvec{{\mathcal {V}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {V}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}}: \\ \Big ({{\varvec{div}}^{\varvec{T}}_{\varvec{\tau }_{\varvec{D}}}}, {{\varvec{div}}^{\varvec{T}}_{\varvec{\tau }_{\varvec{S}}}}\Big ) ({{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}) - \Big ({\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{\varvec{\Gamma }_{\varvec{D}}}}}, {\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{\varvec{\Gamma }_{\varvec{S}}}}}\Big ) ({\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{D}}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{S}}}}) \\ - \Big ({\varvec{\pi }^{{\varvec{T}}_{{{\varvec{D}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{{{\varvec{DS}}}}}}}}}}}, {\varvec{\pi }^{{\varvec{T}}_{{{\varvec{S}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}}\Big ) ({\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{{\varvec{D}}}}}}, {\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{{\varvec{S}}}}}}) \\ \hspace{1.0cm} \in \Big ({{\varvec{D}}^{\varvec{-1}}_{{\varvec{u}}_{{\varvec{w}}_{\varvec{D}}}}}, {{\varvec{D}}^{\varvec{-1}}_{{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}\Big ) ({{\varvec{d}}_{{\varvec{D}}}}, {{\varvec{d}}_{{\varvec{S}}}}) \ \\ \hspace{1.4cm} + \Big ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{D}}} \circ {\varvec{\pi }_{\varvec{D}}}, {\varvec{\pi }^{\varvec{T}}_{\varvec{S}}} {\varvec{\partial } \varvec{\Psi }_{\varvec{S}}} \circ {\varvec{\pi }_{\varvec{S}}}\Big ) \\ \hspace{1.4cm} ({{\varvec{d}}_{{\varvec{D}}}}, {{\varvec{d}}_{{\varvec{S}}}}) - \Big ({\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{{\varvec{N}}_{\varvec{D}}}}}, {\varvec{\pi }^{\varvec{T}}_{\varvec{\tau }_{{\varvec{N}}_{\varvec{S}}}}}\Big ) ({\varvec{\widehat{c}}^{{\varvec{*}}}_{\varvec{D}}},{\varvec{\widehat{c}}^{{\varvec{*}}}_{\varvec{S}}}), \\ \text{ in } \ {\varvec{\mathcal {V}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{\mathcal {V}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}} ; \\ \text{ and } \text{ find } \ ({{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}},{{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}}: \\ - \Big ({{\varvec{div}}_{\varvec{\tau }_{\varvec{D}}}}, {{\varvec{div}}_{\varvec{\tau }_{\varvec{S}}}}\Big ) ({{{\varvec{d}}_{\varvec{D}}}},{{\varvec{d}}_{\varvec{S}}}) \\ \hspace{1.0cm} \in \displaystyle {\bigg (\frac{d {{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}}{d t},\frac{d {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}}{d t}\bigg )} + \big ({{\varvec{u}}_{\varvec{D}}} {\varvec{\cdot }} {{\varvec{grad}}_{\varvec{\tau }_{\varvec{D}}}},{{\varvec{u}}_{\varvec{S}}} {\varvec{\cdot }} {{\varvec{grad}}_{\varvec{\tau }_{\varvec{S}}}}\big ) ({{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}) \\ \hspace{1.5cm} + \Big ({{\varvec{div}}_{\varvec{\tau }_{\varvec{D}}}}, {{\varvec{div}}_{\varvec{\tau }_{\varvec{S}}}}\Big ) ({{\varvec{u}}_{\varvec{D}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{u}}_{\varvec{S}}} {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}) \\ \hspace{1.5cm} + \Big ({\varvec{\partial } \varvec{\varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{D}}}}, {\varvec{\partial } \varvec{\varphi }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{S}}}}\Big ) ({{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}) - ({\varvec{\widehat{f}}_{\varvec{D}}},{\varvec{\widehat{f}}_{\varvec{S}}}), \\ \text{ in } \ {\varvec{{\mathcal {Y}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {Y}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}} , \\ \hspace{1.5cm} ({{\varvec{c}}^{{\varvec{*}}}_{\varvec{D}}}(0), {{\varvec{c}}^{{\varvec{*}}}_{\varvec{S}}}(0)) = ({\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}}, {\varvec{\widehat{c}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}}), \end{array} \right. \end{aligned}$$

synchronized by the macro-hybrid internal boundary dual \({\varvec{\Gamma }_{\varvec{DS}}}\)-transmission coupled subdifferential problem

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{{\mathcal{M}\mathcal{H}}}_{{\varvec{\tau }_{\varvec{D}}-\varvec{\tau }_{\varvec{S}}}}}}) \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{D}}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{S}}}}) \in {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{\mathcal {B}}^{{\varvec{*}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}}: \\ \Big ({\varvec{\pi }_{\varvec{\tau }_{\varvec{\Gamma }_{{\varvec{D}}}}}}, {\varvec{\pi }_{\varvec{\tau }_{\varvec{\Gamma }_{\varvec{S}}}}}\Big ) ({{\varvec{d}}_{\varvec{D}}}, {{\varvec{d}}_{\varvec{S}}}) \in \Big ({\varvec{\partial } {\varvec{I}}_{{\varvec{Q}}^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{D}}}}},{\varvec{\partial } {\varvec{I}}_{{\varvec{Q}}^{{\varvec{*}}}_{\varvec{\tau }_{\varvec{S}}}}}\Big ) ({\varvec{\lambda }^{{\varvec{*}}}_{{\varvec{\tau }_{\varvec{D}}}}}, {\varvec{\lambda }^{{\varvec{*}}}_{{\varvec{\tau }_{\varvec{S}}}}}), \\ \text{ in } \ {\varvec{\mathcal {B}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{D}}}}}\times {\varvec{{\mathcal {B}}}_{\varvec{\tau }_{{\varvec{MH}}_{\varvec{S}}}}} ,\end{array} \right. \end{aligned}$$

and subjected to the dual transport flux \({\varvec{\Gamma }_{\varvec{DS}}}\)-interface transmission constraints

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{{\varvec{fl}}_{{\varvec{D-fl}}_{{\varvec{S}}}}}}) \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{D}}}}}, {\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{{\varvec{S}}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{fl}}_{{\varvec{D}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{fl}}_{\varvec{S}}}}: \\ \Big ({\varvec{\pi }_{{\varvec{D}}_{\varvec{\nu }_{\varvec{\Gamma }_{{{{\varvec{DS}}}}}}}}}, {\varvec{\pi }_{{\varvec{S}}_{\varvec{\nu }_{\varvec{\Gamma }_{{{{\varvec{DS}}}}}}}}}\Big ) ({{{\varvec{d}}_{{\varvec{D}}}}},{{{\varvec{d}}_{{\varvec{S}}}}}) \in {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{\varvec{fl}}}} ({\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{D}}}}}, {\varvec{\widetilde{\mu }}^{{\varvec{*}}}_{{{\varvec{fl}}_{\varvec{S}}}}}), \\ \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{fl}}_{\varvec{S}}}}. \end{array} \right. \end{aligned}$$

8.3.2 Variational multidomain primal-dual mixed flow traction coupled system \(({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{D}}})\)-\( ({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{S}}})\)

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}_{{\varvec{tr}}_{{\varvec{D-tr}}_{{\varvec{S}}^{{\varvec{*}}}}}}}) \left\{ \begin{array}{l} \text{ Given } \ ({\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}}, {\varvec{\widehat{q}}_{\varvec{D}}}, {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}) \in {\varvec{\mathcal {B}}_{{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {{\varvec{Z}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \\ \times {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}}, \ ({{\varvec{b}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}}) \in {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} \times {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \text{ find } \ ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) \in {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {Y}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}}: \\ \Big ({{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}} - {{\varvec{grad}}^{\varvec{T}}_{\varvec{S}}}\Big ) ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) - ({\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{D}}}} {\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\pi }^{\varvec{T}}_{\varvec{\Gamma }_{\varvec{S}}}} {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}) \\ -\Big ({\varvec{\pi }^{\varvec{T}}_{{\varvec{D}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{{{\varvec{DS}}}}}}}}},{\varvec{\pi }^{\varvec{T}}_{{{\varvec{S}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}\Big ) ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{D}}}}}, {\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}}) \\ \hspace{1.0cm} \in \bigg (\displaystyle {\frac{{{\varvec{d}} \varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{{{\varvec{dt}}}}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{D}} \varvec{\partial } \varvec{\Psi }_{\varvec{D}} \varvec{\circ } \varvec{\pi }_{\varvec{D}}}) {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.5cm} - {\varvec{\pi }^{{\varvec{T}}_{\varvec{sw}}}} {\varvec{\widehat{u}}_{{\varvec{D}}_{\varvec{w}}}} - {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \ \\ \\ \hspace{1.5cm} - 1 / \mu \ {{\varvec{T}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}_{\varvec{S}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \\ \hspace{1.5cm} + \ ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } \varvec{\Psi }_{\varvec{S}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{T}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{v}}^{{\varvec{*}}}_{\varvec{S}}}\bigg ), \\ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}}; \\ \hspace{1.5cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} (0) = {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}} , \\ \\ \text{ and } \text{ find } \ ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {W}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}}): \\ \Big (- {{\varvec{grad}}_{\varvec{D}}} + {{\varvec{grad}}_{\varvec{S}}}\Big ) \ ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) \\ \hspace{1.0cm} \in \bigg (\lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \ \\ \hspace{1.4cm} \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} - {{\varvec{b}}}\bigg ), \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{1.5cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}} , \end{array} \right. \end{aligned}$$

synchronized by the internal boundary \({\varvec{\Gamma }_{\varvec{DS}}}\)-transmission subpotential subdifferential dual problem

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{\mathcal{M}\mathcal{H}}_{\varvec{DS}}}}) \left\{ \begin{array}{ll} \text{ Find } \ ({\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{\mathcal {B}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{S}}}}}: \\ \Big ({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{D}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{S}}}}\Big ) ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) \ \in \Big ( {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{D}}}}, {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{S}}}}\Big ) ({\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}), \\ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{S}}}}}, \, \end{array} \right. \end{aligned}$$

and subjected to the dual traction \({\varvec{\Gamma }_{\varvec{DS}}}\)-interface transmission constraints

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^{{\varvec{*}}}_{\varvec{tr}}}) \ \ \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{\varvec{tr}}_{\varvec{D}}}}, {\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{tr}}_{\varvec{D}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{tr}}_{\varvec{S}}}}, \\ \Big ({\varvec{\pi }_{{\varvec{D}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}},{\varvec{\pi }_{{{\varvec{S}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}\Big ) ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) \in {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{\varvec{tr}}}} ({\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{D}}}}}, {\varvec{\widetilde{\chi }}^{{\varvec{*}}}_{{{\varvec{tr}}_{\varvec{S}}}}}), \ \ \quad \text{ in } \ {\varvec{\widetilde{{{\mathcal {B}}}}}_{{\varvec{tr}}_{\varvec{D}}}} \times {\varvec{\widetilde{\mathcal {B}}}_{{\varvec{tr}}_{\varvec{S}}}} . \end{array} \right. \end{aligned}$$

8.3.3 Variational multidomain dual-primal mixed flow velocity coupled system \(({\varvec{\mathcal{M}\mathcal{H}}^{{\varvec{*}}}_{\varvec{D}}})\)-\( ({\varvec{\mathcal{M}\mathcal{H}}_{\varvec{S}}})\)

$$\begin{aligned} ({\varvec{\mathcal{M}\mathcal{H}}_{{\varvec{D}}^{{\varvec{*}}}{\varvec{S}}}}) \left\{ \begin{array}{l} \text{ Given } \ ({\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\widehat{q}}_{\varvec{D}}}, {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}}, {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}}) \in {\varvec{\mathcal {B}}_{{{{{\varvec{MH}}_{\varvec{D}}}}}}} \times {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {{\varvec{Z}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \\ \times {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} , \\ ({{\varvec{b}}}, {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}}) \in {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} \times {{\varvec{H}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \text{ find } \ ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{{\mathcal {V}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {W}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{S}}}}: \\ \Big (- {{\varvec{grad}}_{\varvec{D}}}, {{\varvec{grad}}_{\varvec{S}}}\Big ) ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) - \Big ({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{D}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{S}}}}\Big ) ({\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}) \\ -\Big ({\varvec{\pi }_{{{\varvec{D}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}},{\varvec{\pi }_{{{\varvec{S}}_{{\varvec{\nu }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}\Big ) ({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}) \\ -\Big ({\varvec{\pi }_{{{\varvec{D}}_{{\varvec{\tau }}_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}},{\varvec{\pi }_{{\varvec{S}}_{\varvec{\tau }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}\Big ) ({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}) \\ \hspace{1.0cm} \in \bigg (\lambda ^{-1}( {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}) {{\varvec{K}}^{\varvec{-1}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} + ({\varvec{\pi }^{\varvec{T}}_{{\varvec{D}}} \varvec{\partial } \varvec{\Psi }_{\varvec{D}} \varvec{\circ } \varvec{\pi }_{\varvec{D}}}) {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}} \\ \hspace{1.7cm} - {\varvec{\pi }^{\varvec{T}}_{\varvec{fw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} - {\varvec{\pi }^{\varvec{T}}_{\varvec{sw}}} {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{\varvec{D}}} + {\varvec{\gamma }^{{\varvec{*}}}_{{\varvec{2}}_{\varvec{D}}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), \\ \hspace{1.7cm} \displaystyle {\rho _S \frac{\partial {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}}{\partial t}} + ({\varvec{\pi }^{\varvec{T}}_{\varvec{S}} \varvec{\partial } \varvec{\Psi }_{\varvec{S}} \varvec{\circ } \varvec{\pi }_{\varvec{S}}}) {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} - {\varvec{\pi }^{\varvec{T}}_{{\varvec{S}}_{\varvec{N}}}} {\varvec{\widehat{s}}} - {{\varvec{b}}}\bigg ) \\ \ \text{ in } \ {\varvec{{\mathcal {V}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {V}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{2.0cm} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} (0) = {\varvec{\widehat{v}}^{{\varvec{*}}}_{{\varvec{S}}_{\varvec{0}}}}; \\ \\ \text{ and } \text{ find } \ ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}, {{\varvec{T}}}) \in {\varvec{{\mathcal {X}}}^{{\varvec{*}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {Y}}^{{\varvec{*}}}}_{{\varvec{MH}}_{\varvec{S}}}} : \\ \Big ( {{\varvec{grad}}^{\varvec{T}}_{\varvec{D}}}, - {{\varvec{grad}}^{\varvec{T}}_{\varvec{S}}}\Big ) ({{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) \\ \hspace{1.0cm} \in \bigg (\displaystyle { \frac{\partial {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}}{\partial t}} - {\varvec{\widehat{q}}_{\varvec{D}}} ({\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}}), - 1 / \mu \ {{\varvec{T}}} - 1 / \mu \ p {{\varvec{I}}} + {{\varvec{grad}}} \ {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}} \bigg ), \\ \text{ in } \ {\varvec{{\mathcal {Y}}}_ {{\varvec{MH}}_{\varvec{D}}}} \times {\varvec{{\mathcal {Y}}}_{{\varvec{MH}}_{\varvec{S}}}} , \\ \hspace{2.0cm} {\varvec{\theta }^{{\varvec{*}}}_{\varvec{D}}} (0) = {\varvec{{\widehat{\theta }}}^{{\varvec{*}}}_{{\varvec{D}}_{\varvec{0}}}}. \end{array} \right. \end{aligned}$$

synchronized by the internal boundary \({\varvec{\Gamma }_{\varvec{DS}}}\)-transmission subpotential subdifferential dual proble

$$\begin{aligned} ({\varvec{\widetilde{\mathcal {T}}}^{{\varvec{*}}}_{{\varvec{MH}}_{\varvec{DS}}}}) \left\{ \begin{array}{ll} \text{ Find } \ ({\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{\mathcal {B}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {B}}}^{{\varvec{*}}}_{{{\varvec{MH}}_{\varvec{S}}}}}: \\ \Big ({\varvec{\pi }_{\varvec{\Gamma }_{\varvec{D}}}} {{\varvec{u}}_{{\varvec{D}}_{\varvec{w}}}}, {\varvec{\pi }_{\varvec{\Gamma }_{\varvec{S}}}} {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}\Big ) \\ \in \Big ({\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{D}}}}, {\varvec{\partial } {\varvec{\mathcal {I}}}^{{\varvec{*}}}_{{\varvec{Q}}_{\varvec{S}}}}\Big ) ({\varvec{\widetilde{\lambda }}^{{\varvec{*}}}_{\varvec{D}}}, {\varvec{\lambda }^{{\varvec{*}}}_{\varvec{S}}}) , \\ \text{ in } \ {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{D}}}}} \times {\varvec{{\mathcal {B}}}_{{{\varvec{MH}}_{\varvec{S}}}}}. \end{array} \right. \end{aligned}$$

and subjected to the dual normal-tangential velocity \({\varvec{\Gamma }_{\varvec{DS}}}\)-interface transmission constraints

$$\begin{aligned} ({\varvec{{\mathcal {T}}}^*_{{\varvec{v}}_{\varvec{\nu },{\varvec{v}}_{\varvec{\tau }}}}}) \ \ \ \left\{ \begin{array}{l} \text{ Find } \ ({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}) \in {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}: \\ \Big ({\varvec{\pi }_{{{\varvec{D}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}}, {\varvec{\pi }_{{\varvec{S}}_{\varvec{\nu }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}\Big ) ({{\varvec{w}}_{\varvec{D}}}, {{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) \in {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{v}}_{\varvec{\nu }}}}} ({\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}}, {\varvec{\widetilde{\zeta }}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}}), \\ \text{ in } \ {\varvec{\widetilde{{\mathcal {B}}}}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{D}}}}} \times {\varvec{\widetilde{{\mathcal {B}}}}_{{\varvec{v}}_{\varvec{\nu }_{\varvec{S}}}}} ; \\ \text{ and } \ ({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}) \in {\varvec{\widetilde{ {{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}} \times {\varvec{\widetilde{{{\mathcal {B}}}}}^{{\varvec{*}}}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}: \\ \Big ({\varvec{\pi }_{{\varvec{D}}_{\varvec{\tau }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}},{\varvec{\pi }_{{\varvec{S}}_{\varvec{\tau }_{\varvec{\Gamma }_{{\varvec{DS}}}}}}}\Big ) ({{\varvec{w}}_{\varvec{D}}},{{\varvec{v}}^{{\varvec{*}}}_{\varvec{S}}}) \in \ {\varvec{\partial } {\varvec{I}}_{\varvec{\widetilde{{{\mathcal {T}}}}}_{{\varvec{v}}_{\varvec{\tau }}}}} ({\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}}, {\varvec{\widetilde{\xi }}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}), \\ \text{ in } \ {\varvec{\widetilde{{\mathcal {B}}}}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{D}}}}} \times {\varvec{\widetilde{{\mathcal {B}}}}_{{\varvec{v}}_{\varvec{\tau }_{\varvec{S}}}}}. \end{array} \right. \end{aligned}$$

9 Conclusions

On the basis of reflexive Banach functional frameworks, multidomain mixed variational constrained transport filtration processes of a coastal aquifer have been studied. Coupled media pairs of incompressible surface Stokesian and subsurface Darcian evolutionary flows, modeled the mechanical systems, with variational macro-hybrid spatial nonoverlapping decompositions for parallel computing purposes. Primal and dual abstract general macro-hybrid mixed variational initial/boundary-value problems were proposed for a systematic modeling and analyisis of the divers transport flow coupled phenomena, which, importantly, corresponded to subpotential maximal monotone subdifferential inclusions. Concerning the qualitative solvability analysis, existence-uniqueness fixed-point results where established via evolution duality principles. The internal boundary macro-hybrid and interface surface-subsurface transmission constraints of the free boundary coastal transport flow system, have been variationally modeled as Lagrangian solutions of dual subpotential inclusions, the innovative modeling of the study. Lastly, the coupled surface-subsurface transport flow of the evolutionary local systems was established in terms of dual mass fluxes, primal-dual coupling tractions and dual-primal coupling velocities, via product variational Lagrangian dual fields.

Impotant technologycal extensions of the present study, would be those concerning hydrological systems with seawater intrusion, interconnected with lake-river-stream-reservoir multisystems, which could be feasible.