Skip to main content
SpringerLink
Log in

Stability Analysis and Existence Criteria with Numerical Illustrations to Fractional Jerk Differential System Involving Generalized Caputo Derivative

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

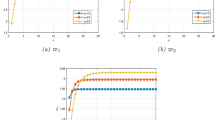
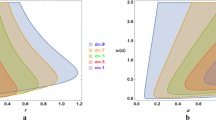
This inquire about ponder is committed to investigating a few properties in connection to behaviors of solutions to an extended fractional structure of the standard jerk equation. Here, we define the scheme of the general fractional jerk problem using the generalized G operators. The existence result of such a new model is derived and analyzed based on some inequalities and fixed point tools. Furthermore, analysis of its Ulam–Hyers–Rassias type stability is performed and finally, we give numerical simulations for the existing parameters of the mentioned fractional G-jerk system in the Katugampola, Caputo–Hadamard and Caputo settings under different arbitrary orders.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Data Availability and Materials

Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Ghanbari, B.: On the modeling of the interaction between tumor growth and the immune system using some new fractional and fractional-fractal operators. Adv. Differ. Equ. 2020, 585 (2020). https://doi.org/10.1186/s13662-020-03040-x

    Article  MathSciNet  Google Scholar 

  2. Ghanbari, B.: On approximate solutions for a fractional prey-predator model involving the Atangana–Baleanu derivative. Adv. Differ. Equ. 2020, 679 (2020). https://doi.org/10.1186/s13662-020-03140-8

    Article  MathSciNet  Google Scholar 

  3. Itik, M., Banks, S.P.: Chaos in a three-dimensional cancer model. Int. J. Bifurc. Chaos 20(1), 71–79 (2010). https://doi.org/10.1142/S0218127410025417

    Article  MathSciNet  Google Scholar 

  4. Elsonbaty, A.R., El-Sayed, A.M.: Further nonlinear dynamical analysis of simple Jerk system with multiple attractors. Appl. Math. Lett. 87, 1169–1186 (2017). https://doi.org/10.1007/s11071-016-3108-3

    Article  Google Scholar 

  5. Liu, C.S., Chang, J.R.: The periods and periodic solutions of nonlinear Jerk equations solved by an iterative algorithm based on a shape function method. Appl. Math. Lett. 102, 106151 (2020). https://doi.org/10.1016/j.aml.2019.106151

    Article  MathSciNet  Google Scholar 

  6. Gottlieb, H.P.W.: Harmonic balance approach to periodic solutions of nonlinear Jerk equations. J. Sound Vib. 271(3–5), 671–683 (2004). https://doi.org/10.1016/S0022-460X(03)00299-2

    Article  Google Scholar 

  7. Ma, X., Wei, L., Guo, Z.: He’s homotopy perturbation method to periodic solutions of nonlinear Jerk equations. J. Sound Vib. 314(1–2), 217–227 (2008). https://doi.org/10.1016/j.jsv.2008.01.033

    Article  Google Scholar 

  8. Saifur Rahman, M., Hasan, A.S.M.Z.: Modified harmonic balance method for the solution of nonlinear Jerk equations. Results Phys. 8, 893–897 (2018). https://doi.org/10.1016/j.rinp.2018.01.030

    Article  Google Scholar 

  9. Prakash, P., Rajagopal, K., Singh, J.P., Roy, B.K.: Megastability in a quasi-periodically forced system exhibiting multistability, quasi-periodic behaviour, and its analogue circuit simulation. AEU-Int. J. Electron. Commun. 92, 111–115 (2018). https://doi.org/10.1016/J.AEUE.2018.05.021

    Article  Google Scholar 

  10. Prakash, P., Singh, J.P., Roy, B.K.: Fractional-order memristor-based chaotic jerk system with no equilibrium point and its fractional-order backstepping control. IFAC-PapersOnLine 51(1), 1–6 (2018). https://doi.org/10.1016/j.ifacol.2018.05.001

    Article  Google Scholar 

  11. Alqarni, M.M., Amin, R., Shah, K., Nazir, S., Awais, M., Alshehri, N.A., Mahmoud, E.E.: Solution of third order linear and nonlinear boundary value problems of integro-differential equations using Haar wavelet method. Results Phys. 25, 104176 (2021). https://doi.org/10.1016/j.rinp.2021.104176

    Article  Google Scholar 

  12. Amin, R., Shah, K., Mlaiki, N., Yüzbaşı, Ş, Abdeljawad, T., Hussain, A.: Existence and numerical analysis using Haar wavelet for fourth-order multi-term fractional differential equations. Comput. Appl. Math. 41(7), 329 (2022). https://doi.org/10.1007/s40314-022-02041-8

    Article  MathSciNet  Google Scholar 

  13. Ahmad, S., Ullah, A., Shah, K., Akgül, A.: Computational analysis of the third order dispersive fractional PDE under exponential-decay and Mittag–Leffler type kernels. Numer. Methods Partial Differ. Equ. 39(6), 4533–4548 (2023). https://doi.org/10.1002/num.22627

    Article  MathSciNet  Google Scholar 

  14. Abuasbeh, K., Qureshi, S., Soomro, A., Awadalla, M.: An optimal family of block techniques to solve models of infectious diseases: Fixed and adaptive stepsize strategies. Mathematics 11(5), 1135 (2023). https://doi.org/10.3390/math11051135

    Article  Google Scholar 

  15. Alquran, M., Ali, M., Gharaibeh, F., Qureshi, S.: Novel investigations of dual-wave solutions to the Kadomtsev–Petviashvili model involving second-order temporal and spatial-temporal dispersion terms. Partial Differ. Equ. Appl. Math. 8(5), 100543 (2023). https://doi.org/10.1016/j.padiff.2023.100543

    Article  Google Scholar 

  16. Ragusa, M.A., Razani, A., Safari, F.: Existence of radial solutions for a \(p(x)\)-Laplacian Dirichlet problem. Adv. Differ. Equ. 2021, 215 (2021). https://doi.org/10.1186/s13662-021-03369-x

    Article  MathSciNet  Google Scholar 

  17. Etemad, S., Rezapour, S., Samei, M.E.: On a fractional Caputo-Hadamard inclusion problem with sum boundary value conditions by using approximate endpoint property. Math. Model. Appl. Sci. 43(17), 9719–9734 (2020). https://doi.org/10.1002/mma.6644

    Article  MathSciNet  Google Scholar 

  18. Rezapour, S., Samei, M.E.: On the existence of solutions for a multi-singular pointwise defined fractional \(q\)-integro-differential equation. Boundary Value Problems 2020, 38 (2020). https://doi.org/10.1186/s13661-020-01342-3

    Article  MathSciNet  Google Scholar 

  19. Abbas, M.I., Ragusa, M.A.: On the hybrid fractional differential equations with fractional proportional derivatives of a function with respect to a certain function. Symmetry 13, 264 (2021). https://doi.org/10.3390/sym13020264

    Article  Google Scholar 

  20. Samei, M.E., Rezapour, S.: On a system of fractional \(q\)-differential inclusions via sum of two multi-term functions on a time scale. Boundary Value Problems 2020, 135 (2020). https://doi.org/10.1186/s13661-020-01433-1

    Article  MathSciNet  Google Scholar 

  21. Akdemir, A.O., Karaoglan, A., Ragusa, M.A., Set, E.: Fractional integral inequalities via Atangana–Baleanu operators for convex and concave functions. J. Function Spaces 2021, 1055434 (2021). https://doi.org/10.1155/2021/1055434

    Article  MathSciNet  Google Scholar 

  22. Baitiche, Z., Derbazi, C., Matar, M.M.: Ulam stability for nonlinear Langevin fractional differential equations involving two fractional orders in the \(\psi \)-Caputo sense. Applicable Analysis (2021). https://doi.org/10.1080/00036811.2021.1873300

  23. Boutiara, A., Guerbati, K., Benbachir, M.: Caputo-Hadamard fractional differential equation with three-point boundary conditions in Banach spaces. AIMS Math. 5(1), 259–272 (2019). https://doi.org/10.3934/math.2020017

    Article  MathSciNet  Google Scholar 

  24. Boutiara, A., Guerbati, K., Benbachir, M.: Caputo type fractional differential equation with nonlocal Erdelyi–Kober type integral boundary conditions in Banach spaces. Surveys Math. Appl. 15, 399–418 (2020)

    MathSciNet  Google Scholar 

  25. Etemad, S., Rezapour, S., Samei, M.E.: \(\alpha \)-\(\psi \)-contractions and solutions of a \(q\)-fractional differential inclusion with three-point boundary value conditions via computational results. Adv. Differ. Equ. 2020, 218 (2020). https://doi.org/10.1186/s13662-020-02679-w

    Article  MathSciNet  Google Scholar 

  26. Mahmudov, N., Matar, M.M.: Existence of mild solution for hybrid differential equations with arbitrary order. TWMS J. of Pure Appl. Math. 8(2), 160–169 (2017)

    MathSciNet  Google Scholar 

  27. Shah, K., Khalil, H., Khan, R.A.: Investigation of positive solution to a coupled system of impulsive boundary value problems for nonlinear fractional order differential equations. Chaos Solitons Fract. 77, 240–246 (2015). https://doi.org/10.1016/j.chaos.2015.06.008

    Article  MathSciNet  Google Scholar 

  28. Hajiseyedazizi, S.N., Samei, M.E., Alzabut, J., Chu, Y.: On multi-step methods for singular fractional \(q\)-integro-differential equations. Open Math. 19, 1378–1405 (2021). https://doi.org/10.1515/math-2021-0093

    Article  MathSciNet  Google Scholar 

  29. Samei, M.E., Ahmadi, A., Selvam, A.G.M., Alzabut, J., Rezapour, S.: Well-posed conditions on a class of fractional \(q\)-differential equations by using the Schauder fixed point theorem. Adv. Differ. Equ. 2021, 482 (2021). https://doi.org/10.1186/s13662-021-03631-2

    Article  MathSciNet  Google Scholar 

  30. Baleanu, D., Etemad, S., Mohammadi, H., Rezapour, S.: A novel modeling of boundary value problems on the glucose graph. Commun. Nonlinear Sci. Numer. Simul. 100, 105844 (2021). https://doi.org/10.1016/j.cnsns.2021.105844

    Article  MathSciNet  Google Scholar 

  31. Sousa, J.V.D.C., de Oliveira, E.C.: On the Ulam-Hyers-Rassias stability for nonlinear fractional differential equations using the \(\psi \)-Hilfer operator. J. Fixed Point Theory Appl. 20, 96 (2018). https://doi.org/10.1007/s11784-018-0587-5

    Article  MathSciNet  Google Scholar 

  32. Ben Chikh, S., Amara, A., Etemad, S., Rezapour, S.: On Ulam–Hyers–Rassias stability of a generalized Caputo type multi-order boundary value problem with four-point mixed integro-derivative conditions. Adv. Differ. Equ. 2020, 680 (2020). https://doi.org/10.1186/s13662-020-03139-1

    Article  MathSciNet  Google Scholar 

  33. Abdeljawad, T., Agarwal, R.P., Karapinar, E., Kumari, P.S.: Solutions of the nonlinear integral equation and fractional differential equation using the technique of a fixed point with a numerical experiment in extended \(b\)-metric space. Symmetry 11(5), 686 (2019). https://doi.org/10.3390/sym11050686

    Article  Google Scholar 

  34. Matar, M.M., Abbas, M.I., Alzabut, J., Kaabar, M.K.A., Etemad, S., Rezapour, S.: Investigation of the \(p\)-Laplacian nonperiodic nonlinear boundary value problem via generalized Caputo fractional derivatives. Adv. Differ. Equ. 2021, 68 (2021). https://doi.org/10.1186/s13662-021-03228-9

    Article  MathSciNet  Google Scholar 

  35. Padder, A., Almutairi, L., Qureshi, S., Soomro, A., Afroz, A., Hincal, E., Tassaddiq, A.: Dynamical analysis of generalized Tumor model with Caputo fractional-order derivative. Fractal Fract. 7(3), 258 (2023). https://doi.org/10.3390/fractalfract7030258

    Article  Google Scholar 

  36. Qureshi, S., Akanbi, M.A., Shaikh, A.A., Wusu, A.S., Ogunlaran, O.M., Mahmoud, W., Osman, M.S.: A new adaptive nonlinear numerical method for singular and stiff differential problems. Alex. Eng. J. 74, 585–597 (2023). https://doi.org/10.1016/j.aej.2023.05.055

    Article  Google Scholar 

  37. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam, Netherlands (2006)

    Google Scholar 

  38. Samko, S.G., Kilbas, A.A., Marichev, O.I.: Fractional Integrals and Derivatives: Theory and Applications. Gordon and Breach, Philadelphia (1993)

    Google Scholar 

  39. Almeida, R.: A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 44, 460–481 (2017). https://doi.org/10.1016/j.cnsns.2016.09.006

    Article  MathSciNet  Google Scholar 

  40. Almeida, R., Malinowska, A.B., Teresa, N., Monteiro, T.: Fractional differential equations with a Caputo derivative with respect to a kernel function and their applications. Math. Methods Appl. Sci. 41(1), 336–352 (2018). https://doi.org/10.1002/mma.4617

    Article  MathSciNet  Google Scholar 

  41. Granas, A., Dugundji, J.: Fixed Point Theory. Springer, New York (2003)

    Book  Google Scholar 

Download references

Acknowledgements

The third and fifth authors were supported by Azarbaijan Shahid Madani University. J. Alzabut is thankful to Prince Sultan University and OSTİM Technical University for their endless support for writing this paper.

Funding

Not applicable.

Author information

Authors and Affiliations

  1. Department of Mathematics, Al-Azhar University-Gaza, Gaza Strip, Palestine

    Mohammed M. Matar

  2. Department of Mathematics, Faculty of Basic Science, Bu-Ali Sina University, Hamedan, 65178-38695, Iran

    Mohammad Esmael Samei

  3. Department of Mathematics, Azarbaijan Shahid Madani University, Tabriz, Iran

    Sina Etemad & Shahram Rezapour

  4. Laboratory of Applied Mathematics, University of Kasdi Merbah, Ouargla, 30000, Algeria

    Abdelkader Amara

  5. Department of Mathematics, Kyuing Hee University, 26 Kyungheedae-ro, Dongdaemun-gu, Seoul, Republic of Korea

    Shahram Rezapour

  6. Department of Medical Research, China Medical University Hospital, China Medical University, Taichung, Taiwan

    Shahram Rezapour

  7. Department of Industrial Engineering, OSTÍM Technical University, 06374, Ankara, Turkey

    Jehad Alzabut

  8. Department of Mathematics and General Sciences, Prince Sultan University, 12435, Riyadh, Saudi Arabia

    Jehad Alzabut

Authors
  1. Mohammed M. Matar
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohammad Esmael Samei
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Sina Etemad
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Abdelkader Amara
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Shahram Rezapour
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Jehad Alzabut
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors have equal contributions. All authors read and approved the final manuscript. MMM: Initial draft, methodology, actualization, formal analysis, validation, investigation, and initial draft. MES: Actualization, formal analysis, validation, investigation, software, simulation, and was a major contributor in writing the manuscript. SE: Actualization, methodology, formal analysis, validation, investigation, software, simulation, initial draft and was a major contributor in writing the manuscript. AA: Actualization, validation, methodology, formal analysis, investigation, and initial draft. SR: Validation, methodology, actualization, formal analysis, investigation, and initial draft. JA: Investigation, initial draft, formal analysis, actualization, methodology, validation, and supervision of the original draft and editing. All authors read and approved the final manuscript.

Corresponding authors

Correspondence to Mohammad Esmael Samei or Shahram Rezapour.

Ethics declarations

Conflict of interest

The authors declare that they have no competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Matar, M.M., Samei, M.E., Etemad, S. et al. Stability Analysis and Existence Criteria with Numerical Illustrations to Fractional Jerk Differential System Involving Generalized Caputo Derivative. Qual. Theory Dyn. Syst. 23, 111 (2024). https://doi.org/10.1007/s12346-024-00970-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-024-00970-9

Keywords

Mathematics Subject Classification

Search

Navigation