1 Introduction

This paper begins with a brief treatment of the notion of seeds of mathematical thinking, in particular, the seed of sameness. This is followed by relevant literature on equivalence, structure/structuring, and properties, as well as on students’ views of sameness, all of which provide a backdrop for our study on 10- to 12-year-old Mexican students’ interpretations of sameness within the activity of rewriting numerical equalities in such a way as to explicitly show their truth-value. Because we believe that the development of algebraic thinking at the primary school level should foreshadow central components of algebraic activity at the secondary level (see Kieran, 2007)—components that include form and transformation—our conceptual framework, which is mathematical in nature, elaborates on some of the parallels that exist between transforming numerical equalities and the corresponding activity with algebraic equations. (Note that we use the term equality when only numerical terms are involved and reserve the term equation for those cases where an unknown or a variable is present; we take primary school to mean Grades 1–6, and secondary school Grades 7–11.) The aim of the study is to investigate how our primary school participants write and talk about sameness when they transform numerical equalities into equivalent equalities that indicate their truth-value—and without explicitly computing each side of the equality. After presenting the results, we discuss three aspects: the language that students used to express sameness, properties, and equivalence; the way in which they collapsed the characteristics of invisible and visible sameness in justifying their structure-based equivalence transformations; and the inextricable linking of exchanging and sameness in their structural approaches to generating and justifying equivalences.

2 Literature review

The nature of our research aim leads to a literature review that encompasses three thematic aspects, the first two being more theoretical and the third more empirical: (1) mathematical sameness, (2) equivalence, structure/structuring, and properties, and (3) primary school students’ views of sameness within numerical equalities.

2.1 On mathematical sameness

Walkoe and Levin (2020) propose that children have an enormous bank of experiences and awarenesses that have the potential to be tapped into for developing algebraic thinking. They point to how children’s natural powers can be harnessed to enrich the emergence of algebraic thinking. Walkoe and Levin (2020) refer to these powers as “seeds of algebraic thinking”—such seeds having “long histories that are abstracted over many experiences” (p. 28). One of the examples they focus on involves the seed of balance, which they suggest is instrumental in children coming to interpret the equals sign relationally rather than as an operator symbol. While they do not include sameness in their discussion of various seeds of algebraic thinking, we would argue that it too has a long experiential history for students and is particularly relevant to this paper.

Sameness is not a well-defined term within mathematics. Nevertheless, some topics within mathematics lend themselves to sameness-oriented discourse, such as equivalence and equality. Within the broader literature on sameness, scholars have noted that the perception of sameness can vary according to the attributes or properties that are being focused on. For example, Cable (2014) characterizes sameness as: “a relation involving one or more attributes, and things that are the same with respect to one attribute may be different with respect to another” (p. 228). In particular, Cable (2013) argues that attributes are of three types: (a) the non-quantitative attributes like shape, colour and direction, which may conveniently be called qualities; (b) the continuous attributes like length, area, volume, mass, and duration, “which correspond roughly to what Euclid called ‘magnitudes’, but … should be called quantities from the Latin quantum, meaning how much”; and (c) the attribute of “how-many-ness, which … is one meaning of ‘number’, sometimes distinguished as ‘cardinal number’ or ‘cardinality’” (pp. 6–7). In the classic example of Piaget’s conservation studies, children were found to rely on different types of attributes in deciding whether two sets of beads were the same or not. The key question would appear to be: “Same in what way?”.

Similarly, Melhuish and Czocher (2020) treat this question by distinguishing between, on the one hand, objects that are the same in every respect, that is, for all properties, and on the other hand, objects that share some subset of common properties. The shared-property paradigm, they argue, is a more useful stance because when we speak of sameness “we often mean that the two objects are the same in some (meaningful) respect but are not the same in every respect” (p. 39, italics in original). Sfard (2008) makes a related point when she asks, “what makes people collapse a number of dissimilar things into one?” (p. 169). In this regard, she defines the process of saming as the act of calling different things the same name. She cites Poincaré who stresses that, although the process of saming-with-names is not unique to mathematical discourses, it plays a particularly prominent role in this discourse. Before examining some of the research regarding existing findings on primary students’ views of sameness, we take a brief, but necessary, detour into the topic of equivalence.

2.2 On equivalence, structure/structuring, and properties

In contrast to sameness, mathematical equivalence is a well-defined term—its modern definition having been formulated around the 1940s (Asghari, 2018) and stating that it is a binary relation that is reflexive, symmetric, and transitive—the relation “is equal to” being its canonical example. Equality is a relationship between two quantities, or more generally two mathematical expressions, asserting that the quantities have the same value, or that the expressions represent the same mathematical object, or that an object is being defined.

Within the numerical world, equivalence has two dimensions: the computational and the structural. The computational dimension arises from that part of the definition whereby the two quantities “have the same value”—as in 5 + 9 + 3 = 10 + 7 is true because both sides evaluate to 17. But, equivalence also has a structural dimension, an example of which capitalizes on the reflexive property where a = a with a being a mathematical expression—as in 5 + 9 + 3 = 5 + 9 + 3, which could have been derived structurally from the previous equality by decomposing and recomposing the right side (10 + 7) in such a way as to match the left side.

While equivalence is well-defined, the term structure is without a widely accepted definition in the mathematics education world. For our purposes, we have found it useful to turn to Freudenthal for his approach to structure and structuring. Freudenthal (1991) points out that the system of whole numbers constitutes an order structure where addition can be derived from the order in the structure, such that for each pair of numbers a third, its sum, can be assigned. The relations of this system are of the form a + b = c, which he refers to as an addition structure. In his related discussions on the properties of both the addition and multiplicative structures, Freudenthal (1983) emphasizes multiple means of structuring, and properties characterized in a manner not restricted to their axiomatic formulation within the basic properties of arithmetic, thereby widening discussions on what constitutes a property.

In this regard, Linchevski and Livneh (1999) have drawn our attention to young students’ difficulties with using knowledge of arithmetic structures at the early stages of learning algebra. They suggest that instruction in arithmetic be designed to foster the development of structural thinking by providing experience with equivalent structures of expressions and with their decomposition and recomposition. For example, a number such as 989 can be decomposed structurally as 9 × 109 + 8 according to the division algorithm, or as 900 + 80 + 9 in accordance with the order and addition structures, or in several other ways. Our broadening of perspective on structure and structuring leads us to consider the decomposability of number as an inherent property. While it can be argued that the symmetric property of equality underpins the rewriting of the addition fact of, say, 5 + 7 = 12 as 12 = 5 + 7, we hold that the term decomposing has a much more dynamic feel to it than the more static terminology of symmetry, and thus has a certain appropriateness for activity involving primary school students and their work with transforming equalities.

2.3 On primary school students’ views of sameness within equalities

A perusal of the large body of research literature on young students’ views of the equals sign casts some light on how they see sameness in this domain. For example, Sáenz-Ludlow and Walgamuth (1998) report that the 3rd grade students whom they studied had notions of sameness that tended to vary from quantitative sameness (i.e., the same result after adding the numbers on each side of the equality) to nominal sameness (i.e., the same numbers used on both sides of the equality). They note that when the teacher asked: “What if I said six plus six equals six plus six; is this a true statement?”, one of the students, Ka, stated in response: “Six times [sic] six equals six times six because they are the same/they are the same thing … they both equal the same amount” (p. 170)—blending both the identical similarity of the two numerical expressions and the fact that they yield the same result. However, many of the other young students in that study could not accept verbal or written equality statements with the same numbers on both sides.

Research conducted by Molina et al. (2008) states that Miguel, an 8-year-old, responded (albeit incorrectly) that the equality 18–7 = 7–18 is true because “18 minus 7 and the other is the same, and if it is the same, they are equal” (p. 80)—noticing sameness between the numbers of the sentence, just as he had done for the equality 75 + 23 = 23 + 75. He also used the term, same, for the equality 7 + 7 + 9 = 14 + 9 when he said: “[It’s] true; I did it by adding seven and seven … which is fourteen, the same than there [right side]” (p. 81)—using a number fact related to the given equality sentence, so as to establish mentally the sameness of both sides. And from Carpenter and Franke (2001), a 2nd grade student remarked that 78–49 + 49 = 78 was the same on both sides because “you took the 49 away, and it’s just like getting it back” (p. 157)—implicitly referring to the zero property of addition.

Jones (2009), however, claims that a distinction ought to be made between a “sameness” meaning for the equals sign and one that he refers to as a “can be exchanged for” meaning. He argues that “the sameness view promotes distinguishing statements by truthfulness; the exchanging view promotes distinguishing statements by form in terms of the notation they transform” (p. 175), and that one does not necessarily imply the other—a point of view that we will return to later in the paper. In contrast, Sfard (2008) emphasizes that “we speak about different-looking things as ‘the same’ if we can transform one into the other” (p. 187)—just as the student above, Miguel, exchanged the 7 + 7 for 14 so as to justify the sameness/truthfulness of both sides of the equality 7 + 7 + 9 = 14 + 9.

At this juncture, although we have only cited a limited number of studies related to the equals sign and numerical equalities, they are representative of the wider literature base. They allow us to synthesize the principal ways in which “same in what way” has been expressed by young students in the face of numerical equality statements:

  • Same computed total on each side of the equality (e.g., 246 + 14 = 260 or 7 + 7 = 13 + 1).

  • Same numbers on both sides of the equal sign, yielding the same total (e.g., 6 + 6 = 6 + 6).

  • Same numbers on both sides by using a number fact (e.g., that 7 plus 7 is the same as 14 in 7 + 7 + 9 = 14 + 9).

  • Same numbers on both sides by invoking properties (e.g., 78–49 + 49 = 78).

The point we wish to emphasize here is that the majority of these views that have been documented in studies with primary school students involve different types of sameness between left and right sides of an equality. Absent from this research is the element of sameness with respect to top–down equivalence in the successive transformation of numerical equalities—an aspect that is central to transforming algebraic equations.

3 Conceptual framework: a mathematical frame involving sameness and top–down and left–right types of equivalence

Our conceptual framework, which will be used to support the analysis of our data, is largely mathematical in nature. It involves the interplay between left–right and top–down types of equivalence in transformational activity with numerical equalities and a novel perspective regarding the sometimes visible, but usually invisible, characteristics of these equivalences.

Within both the numeric and algebraic worlds of equalities and equations, equivalence activity—be it computational or structural—can also be characterized according to its form and its transformational aspects, for example, that of left–right and top–down. As has been mentioned, left–right equivalence can be established either computationally by totalling each side or structurally by invoking properties. Top–down equivalence, in contrast, relies largely on the structural dimension. Because of the centrality of top–down and left–right equivalence to our study, and our already-stated position that early algebraic activity with primary students ought to foreshadow aspects of their later algebraic activity at the secondary level, we elaborate with examples from the algebraic and numeric domains.

Within the algebraic domain, we consider two types of equations, those that are solvable for a particular value (or values) of the unknown and those that are identities. We look first at an equation where it is assumed from the start that the left-hand expression is equal to the right-hand expression for a particular value of x:

$$ \begin{gathered} {3}\left( {{3}x + {4}} \right) - {2}\left( {x - {6}} \right) = {6}\left( {x + {3}} \right) \hfill \\ {9}x + {12} - {2}x + {12} = {6}x + {18}\hfill \\{7}x + {24} = {6}x + {18} \hfill \\ {7}x + {24} - {18} = {6}x + {18} - {18} \hfill \\ {7}x + {6} = {6}x \hfill \\ {7}x - {6}x + {6} - {6} = {6}x - {6}x - {6} \hfill \\ x = - {6}. \hfill \\ \end{gathered} $$

Within the above example, we note first of all two different types of top–down equivalence transformations: subexpressions of each side are operated upon by applying the distributive property along with the property of composability/decomposability; but so too is the equation itself operated upon by means of performing the same operation on both sides of the equation. We stress that such transformations, whether applied to subexpressions within the equation or to equations themselves, are both considered as top–down equivalence transformations, each type being equally vital to the equation-solving process. Second, we use the term top–down to mean that the equivalences that are so formed result from a transformation carried out on the subexpression or equation that is usually directly above, while maintaining at the same time the left–right equivalence across the equals sign at each step. We note further that this top–down equivalence between a/an subexpression/equation and its transformed version is of the invisible sort in that each of the transformed objects no longer looks the same, vertically speaking.

We next examine an equation that, once transformed, shows itself to be an identity when the third step is reached:

$$ \begin{gathered} \left( {x - {3}} \right)\left( {{4}x - {3}} \right) = \left( { - x + {3}} \right)^{{2}} + x\left( {{3}x - {9}} \right) \hfill \\ {4}x^{{2}} - {3}x - {12}x + {9} = x^{{2}} - {3}x - {3}x + {9} + {3}x^{{2}} - {9}x \hfill \\ {4}x^{{2}} - {15}x + {9} = {4}x^{{2}} - {15}x + {9} \hfill \\ \end{gathered} $$

The top–down equivalence of the various subexpressions of the above equation with their respective transformed versions yields an equation equivalent to the original and one that in this case is an identity—true for all values of the variable x—thereby establishing the truth-value of the original equation. Once again, we note that there is still the invisible sameness with respect to the top–down equivalence transformations, but now there is also a visible equivalence, horizontally speaking, seen in the third line—the left and right sides are now visibly the same.

The combination of invisible top–down equivalence with visible left–right equivalence seen in the example just above is also encountered in numerical equalities where the structural transformations are aimed specifically at showing the truth-value of the initial equality. We illustrate with the following example:

$$ \begin{gathered} {765} + {237} = {967} + {35} \hfill \\ {7}00 + {65} + {2}00 + {37} = {7}00 + {265} + {2} + {35} \hfill \\ {7}00 + {65} + {2}00 + {2} + {35} = {7}00 + {65} + {2}00 + {2} + {35} \hfill \\ \end{gathered} $$

The central property at play in the transformations used to generate this numerical identity is that of decomposability of whole numbers within the addition structure, along with that of the reflexive property of equality. We choose to rely on these two properties to justify the truth-value of the initial equality, rather than the transitive property of equality (i.e., if A = C and B = C, then A = B), for a couple of reasons. Transitivity, by definition, attends to the expressions per se and ignores that they might be part of an existing equality. Furthermore, this property obscures the crucial role played by top–down transformations in generating equivalent equalities/equations and merely highlights the final form of each transformed expression. In a nutshell, transitivity tends to emphasize the aspect of form rather than that of transforming. And so, our choice to justify the result of the top–down transformations—a result that yields a left–right identity—by the properties of decomposability and reflexivity rather than transitivity adds a measure of didactical recognition to both the aspect of transformation and the context of equality/equation where the expressions reside.

As has just been demonstrated, the process of maintaining top–down equivalence for numerical equalities has some clear parallels with that for algebraic equations. To summarize, both involve invisible sameness between initial and final equalities/equations—this invisibility due to the application of various properties. And the visible sameness of the horizontal left–right equivalence of the final, transformed, numerical equality is a mirror of the reflexive result obtained in the last line of the transformed algebraic identity seen above.

We conclude this section with a statement of our research question: What is the manner in which 10- to 12-year-olds write and talk about sameness when transforming numerical equalities into equivalent equalities that explicitly show their truth-value, within a didactical approach that encourages the movement from computational to structural ways of thinking?

4 Methodology

The research presented in this paper is part of a larger project designed to foster the development of structural thinking on equivalence among Grade 6 primary school students. A description of the students’ evolution from a computational to a structural perspective, and on the nature of the interviewer’s interventions that were especially conducive to spurring the growth of the students’ structure sense, is reported in Kieran and Martínez-Hernández (2022). The present paper deals with an aspect of the larger project that was not reported therein, but that occurred within the structural evolution of the students’ thinking. Details of the methodology given below are those that pertain to the present paper.

4.1 Participants

The research study, which was qualitative in nature, involved three students from the 6th grade (10- to 12-year-olds) of a public community school in Mexico. These students were a subset of the six original students who had participated in the larger project; they were the three who had been the most verbal of the six and who had tended to participate more fully during the earlier interview sessions, on tasks that did not involve the equals sign and where issues related to sameness did not arise. The facet of sameness in the context of numerical equalities, which is reported herein, involved two sessions—the first when the students were halfway through their last year of primary school, and the second when they were just finishing the year and on the verge of completing the arithmetic curriculum for Mexican public education (SEP 2016). They had not had any prior experience with tasks dealing with the equivalence of numerical equalities; they had, however, seen and worked with equalities containing several terms on each side of the equals sign in their regular mathematics class.

4.2 Data collection

The data collection technique was that of the Group Interview, a method that involved the students first working individually on a given question or two. This was followed by an interviewer-orchestrated, discussion segment where the students shared their responses with the rest of the group—a cycle that was repeated for each question or pair of questions. During the group sharing, the interviewer (i.e., the 2nd author of this paper) probed the students’ thinking by asking for clarification or posed additional questions. The interviewer’s interventions were not planned in advance; they were of an ad-hoc nature in reaction to the students’ verbalizations about their own thinking and work.

Data were obtained during sessions that lasted about 60 min each in one of the rooms of the school. The data sources include the individual students’ worksheets, videotaped footage of the interviewer interacting with the group of students and the recording of all their verbalizations and board work, the researcher’s field notes, and follow-up transcriptions and translation of selected parts of the videotaped footage. All interactions and task-sets were in the Spanish language.

4.3 Design of the tasks

Two task-sets were designed, one per session. Task-set A consisted of four numerical equalities and one non-equality (i.e., 4 + 5 = 4 + 3 + 2, 480 + 6 + 123 = 486 + 123, 172 + 10 + 75 = 182 + 50 + 25, 150–70 = 125 + 25–70, and 2 + 8 = 1 + 1 + 5). Some equalities had small numbers and others larger numbers so as to discern whether number size had an impact on students’ approaches. For each equality, there were two types of questions: one asking students whether the given equality statement was true and to explain why, and the other to rewrite the true equalities in another way so as to show they were true.

While Task-set A did not involve any constraints on the way in which students might go about rewriting the equalities so as to show their truth-value, Task-set B asked students not to compute the value of each side in order to determine their truth-value. Three true equalities comprised Task-set B (i.e., 10 + 7 = 5 + 12, 530 + 200 = 300 + 430, and 8 + 2 + 16 = 10 + 12 + 4). As with the previous task-set, students were to rewrite the equalities in a different way that showed that they were true, explaining the reasoning that underpinned their rewriting. The last question of this task-set asked for a generalization of the main strategy that the students had been using throughout the task-set.

Additionally, prior to the unfolding of the designed tasks, the classroom teacher of these students reviewed the tasks. In her opinion, the students had never solved similar tasks; they had only worked with the use of the equals sign in a computational manner.

4.4 Data analysis

Using the above-described mathematical conceptual framework as a support for examining our data, we analyzed the ways in which sameness was a central part of students’ interpretations of their work in transforming the given equalities into other equivalent forms that indicated explicitly their truth-value. All instances in which students wrote or verbalized the term “same,” or some similar term, were noted. As we searched for and documented these instances within our data, we asked ourselves: what kinds of language were the students using to justify the equivalence transformations they carried out in order to indicate the truth-value of their rewritten numerical equalities? What kinds of properties were they mentioning, either explicitly or implicitly, in their justifications? Were they expressing that the resulting equivalence between the left and right sides of the transformed equality was different from the top–down equivalence in that the former was clearly a visible form of equivalence? Was there an evolution throughout the study with respect to their ways of transforming the equalities and of justifying these transformations in terms of sameness? All the worksheets and videotaped-transcripts were coded for language-use bearing on sameness. Both researchers coded the data independently. For those very few cases where there was initial lack of agreement, the researchers discussed the differences and reached consensus.

5 Results

The samples of representative student work and talk that are offered herein respect the chronological order of the group activity and thus allow for inferring the evolutionary nature of the students’ thinking about sameness with respect to equivalence transformations of numerical equalities. We acknowledge, nonetheless, that the results we present are not independent of the background experience of the students, nor of the tasks we designed, nor of the interventions on the part of the interviewer, nor of the various individual students’ input to the group discourse. In fact, all of these contributed to the ways in which the students came to think, write, and speak about sameness. The occasions during which students expressed their ideas about sameness were principally those when they were justifying that their manner of rewriting a given equality was maintaining its truth-value. In that their rewriting of equivalent equalities was evolving from computational to more structural approaches, their views of sameness, and how they achieved what they construed as sameness, are to be interpreted within that context.

5.1 Sameness by calculating the total of each side of the equality

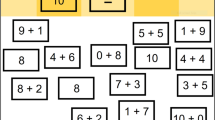
At the outset of Task-set A, sameness was visibly achieved by computing the total of each side of a given equality—as seen by the student S3’s two additions to the right of the worksheet questions (see Fig. 1). Note that this student justified the truth of the given equality with the words, “Because we get the same result.” For each of the other given equalities of this task-set and the question as to whether the equality was true or not, the students continued with the approach of computing both sides and justified the truth (or falsity) of each with the statement that they obtained (or not) the same result.

Fig. 1
figure 1

Showing and expressing sameness by computing the total of each side [NB: English translation that is provided here was not part of the students’ worksheets]

Full size image

5.2 Top–down, invisible sameness between numbers of initial equality and their transformed versions, but without relating the two sides of the transformed equality

When the second part of the same task-set then requested that the students rewrite the given equalities, but in a different way, they transformed one side in one way and the other side in another way (see Fig. 2). However, the justification remained the same: “Because I get the same result”—possibly because they had already computed the total of each side and knew that the results were the same. But take note of the properties used to decompose each side, which favored the use of place-value, halving, and addends involving 10s and 5s.

Fig. 2
figure 2

S1’s rewritten equality, but without any visible sameness between the two sides

Full size image

Also of interest in the approach used by the students in response to this question is that it suggests that vertical, but invisible, sameness for equivalence of numerical equalities may come more spontaneously than the idea of working toward a common form that would achieve horizontal, visible sameness between left and right sides of an equality, and thereby demonstrate the truth-value of the equality. However, the fact that the students had already computed the total of each side for the given equalities may have prompted them instead to demonstrate their prowess at representing the left- and right-side expressions in a variety of ways. In any case, the left–right sameness of the two transformed sides remained invisible.

This same invisibility of the left–right equivalence occurred when students were presented with the non-equality of the task-set, 2 + 8 = 1 + 1 + 5—for which they once again computed both sides and declared the “equality” to not be true. When they were asked how they might transform it so that it would be true, S1 rewrote it as 8 + 1 + 1 = 5 + 5; but she was the only one to do so in this form with a numerical expression on each side. In contrast, S2 and S3 chose to rewrite in the form of a numerical expression on the left side and the total on the right side—attesting to the strength of their computational view of equivalence.

5.3 Left–right visible sameness of the transformed equality

The tasks of the next set (Task-set B) asked explicitly that the students not calculate the total of each side in order to show that the given equalities were true and then to justify their approach. When presented with this constraint, the students were clearly puzzled: “What other way is there?”, they wondered. And, so, the interviewer asked if they could rewrite the equalities, but using the same numbers that were in the equalities—hinting with this comment that one side might be transformed into the form of the other side. But the follow-up suggestion by one of the students, S3, prompted the discussion to move instead toward generating a third common form.

When the first equality statement of this set was then presented, 10 + 7 = 5 + 12, S3 suggested that each of the numbers of the left side could be rewritten in such a way that the left side became 5 + 5 + 5 + 2, and justified it by stating that “the 5 + 5 came from the 10 and the 5 + 2 from the 7”, that is, that the 10 could be decomposed into 5 + 5, and the 7 into 5 + 2. And, so, the interviewer then asked: “What about the right side? Could it be expressed in this way?” S3 responded that it could, stating how the decomposed expression fit with each number of the initial right side. While this was for the students a novel way of rewriting an equality, all three agreed that the right side of the initial equality could be rewritten in the same way as the transformed left side. Each student in the group stated that the initial equality could be rewritten as 5 + 5 + 5 + 2 = 5 + 5 + 5 + 2 because “it gives the same,” which we interpreted as “the same total on each side.”

With the identical pair of expressions that had been suggested for each side of the newly transformed equality, the seed for a visible form of left–right equivalence had now been planted. The computational dimension of equivalence that the students had been relying upon was on the way to being augmented by a more structural approach that would not require totaling each side once the same form had been obtained for each side—as the following verbatim extract suggests (I is the interviewer; S1, S2, and S3 are the students):

figure a

As can be seen from the second-to-last line of the above extract, S3 who had earlier related the decomposed equality to both sides of the initial equality still considered that computing the total of each side was the means to validate it (although he may have misunderstood the intended meaning of the interviewer’s question). In any case, S2 and S1 now supported the idea that the decomposed equality proposed by S3 was all that was needed for validation, due to the common form in which both sides had been rewritten. We note at this moment how S2’s speech and thinking have changed. At the beginning of the task, S2 stated that the total “is” the same, which means that she had calculated each side. But now S2 justified with another tense: it “will” give the same, which suggests that she was aware that she would get the same total, but that it was not necessary to calculate it. It would be enough to observe that both sides of the equality were written in the same form in order to establish the truth-value.

5.4 Coordinating and justifying top–down sameness with left–right sameness

The interviewer subsequently asked the students to rewrite differently (i.e., different from 5 + 5 + 5 + 2 = 5 + 5 + 5 + 2) the initial equality 10 + 7 = 5 + 12, but again in such a way that both sides would look alike. In response, S1 genuinely decomposed only the left side (10 + 7) of the initial equality to obtain 2 + 2 + 2 + 2 + 2 + 2 + 2 + 3, and then copied this expression onto the right side. This became obvious when S1 was asked to relate the copied expression 2 + 2 + 2 + 2 + 2 + 2 + 2 + 3 with the right side (5 + 12) of the initial equality—which she could not do. After a few futile attempts at relating the two, S1 started anew. She proposed a different expression, this time starting with the right side, 2 + 2 + 1 + 5 + 5 + 2, which she could relate to the right side of the initial equality. And then she rewrote the left side of the initial equality in the same way—but this time she was able to relate the decomposed expression, number by number, with the left side of the initial equality.

Not only was S1 now able to express the invisible top–down sameness between the expressions of the initial equality and their equivalent versions in terms of the transformations she had carried out, but she was also now referring to the left–right sameness that resulted from her transformations—as evidenced from her written justification (see Fig. 3): “Because if I look and compare each number, they are the same and obviously it is the same.”

Fig. 3
figure 3

S1 referring to the visible left–right sameness of the rewritten equality

Full size image

We inferred that the “it” in S1’s statement, “obviously it is the same,” refers to the total of each side. It seemed that all the students now no longer needed to compute the total. This was confirmed with the next equality involving larger numbers, 530 + 200 = 300 + 430. Their aim had become that of achieving sameness by decomposing each side in exactly the same way—and for some students even in the same order—as illustrated in the following extract:

figure b

As can be discerned from the last line of the excerpt just above, S2’s first idea for rewriting the right side began with the number 100, but she immediately realized that it would give not only a different starting number from that of her left-hand decomposed expression 200+ 300 + 30 + 200, but also a different decomposition. After this first attempt, S2 changed her proposal: she transformed the left side 530 + 200 into 300 + 200 + 30 + 200, and then used the same expression for the right side (see Fig. 4), which she could justify with the right side of the initial equality.

Fig. 4
figure 4

S2’s successful second attempt at transforming 530 + 200 = 300 + 430 into 300 + 200 + 30 + 200 = 300 + 200 + 30 + 200

Full size image

But not everyone in the group needed to have both sides decomposed in exactly the same order. As illustrated by the work shown in Fig. 5, S3 initially started to decompose the right-hand side in the same way that he had transformed the left-hand side, but then crossed it out and switched to a different strategy. We hypothesize that, when he noticed the 4 on the far-right side of the initial equality (8 + 2 + 16 = 10 + 12 + 4), he realized that this 4 would not fit with the last 8 of his proposed decomposition 4 + 4 + 1 + 1 + 8 + 8. So, he decomposed the right side with a different ordering, but using the same numbers—his decomposition of the left side clearly guiding his alternative decomposition of the not-yet-decomposed right side of the initial equality. This resulted in the same numbers on both sides of the transformed equality, even if the order of the terms was different.

Fig. 5
figure 5

S3’s transformation of 8 + 2 + 16 = 10 + 12 + 4 into 4 + 4 + 1 + 1 + 8 + 8 = 8 + 1 + 1 + 8 + 4 + 4

Full size image

At the end of the session, the students were presented with a question of a more general nature: “What should be done, regardless of the numbers involved in the equality and without calculating the total of each side, to show that the equality is true?”:

figure c

The comments of S2 and S3 synthesize the double aspect of (1) decomposing in such a way that the numerical subexpression that replaces a number of the initial equality has the same value as the number it replaces, and (2) ensuring that the transformation of both sides results in the same left-hand and right-hand expressions so as to demonstrate the equality of the two sides—with S1 seeming to emphasize from her prior comment (before S3 and S2 spoke up) that the uppermost aim was to “convert the numbers in a different way [from the initial equality], but that they should be the same [on both sides of the transformed equality].” This combination of top–down equivalence of the given numbers with their transformed versions, along with clearly demonstrating at the same time the aspect of left–right equivalence, involved a way of thinking that was new to these students. Nevertheless, the justification for both the invisible sameness of the top–down equivalence, and the resulting visible sameness of left–right equivalence, was in terms of the uncalculated results that were being maintained for each numerical transformation and for the final form of the equality.

6 Discussion

The first part of this Discussion focuses on the language that students relied upon, both in their written work and in their oral contributions to the group discussions, and thereby serves to synthesize our main results. In that the structurally-oriented equivalence transformations that students described were characterized by both invisibility and visibility, yet in a manner that tended to collapse both in terms of hidden numerical values, the second part of the Discussion looks more deeply at this aspect—one which suggests not only the tight connection between the numerical and the structural in students’ algebraic thinking but also some implications for transitioning to letter-symbolic algebra. The third and last part of the Discussion returns to an issue that arose in the literature review and, in view of our results, casts new light on the question regarding the separation of the exchanging and sameness components of equality in students’ approaches to generating and justifying equivalences.

6.1 The language that students used to express sameness, properties, and equivalence

We asked ourselves several questions at the outset of our analysis, questions focusing on the kind of language that students might use to express sameness, properties, and equivalence and the role of this language in developing their thinking. Our approach in this regard is in line with Sfard (2008) who argues that “linguistic communication is the primary source of sustainable, accumulable changes in human forms of doing” (p. 123), and where thinking and communicating, whether the communicating be intra- or inter-personal, are united. In our study, both the student-sharing of their written work within the group and of their justifications of the approaches taken, as well as the interviewer-probing of students’ responses or his hinting at alternative ways of tackling a given task, were all conducive to expanding students’ mathematical discourse.

With respect to the kinds of language the students used to justify the equivalence transformations that they carried out in order to indicate the truth-value of their rewritten numerical equalities, it was seen that they initially computed the total of each side of the equalities and justified the truth-value with a language that centered on the results they had obtained: “We get the same result.” Even after rewriting those given equalities with a different decomposition of each side, their written and oral justifications continued with the same language.

When subsequently presented with a new set of equalities and being asked explicitly to not calculate the total of each side before rewriting the equalities, their thinking began to evolve. Instead of first calculating the total for the left side, they began by decomposing it. Their language shifted to explaining where each of the numbers of the decomposed left side of their partially rewritten equality came from with respect to the initial equality. It is noted, however, that the search for appropriate transformations for the numbers of the initial left side sometimes required a few tries, and several back-and-forth comparisons with the initial right side, before arriving at a decomposition that would work equally well for the right side. As seen in the schematic representation of the process of transforming an initial equality, illustrated in Fig. 6, the students came to reason that, since the various numbers of A can be decomposed in a top–down manner into A1 and since B can be equivalently decomposed in the same way, the resulting equality A1 = A1 which is obviously true demonstrates that the initial equality A = B is also true.

Fig. 6
figure 6

The transformation process whereby A = B is transformed to A1 = A1 (illustrated by the sequencing of arrows starting at A; the curved arrow represents the comparing of A1 with B, which then served to guide the transformation of B into A1)

Full size image

After completing the rewriting of the decomposed equality, the students’ oral justification of its truth-value was expressed as: “It will give the same”—suggesting that they no longer needed to compute the result of each side, but that it would surely be the same. In fact, the language they used to justify both the numerical transformation of a number into a decomposed, equivalent numerical subexpression, and the final form of the rewritten equality with its two identical sides, involved the word same in both cases, but the former top–down transformation had a qualification attached to it: “It looks different but gives the same result.” Thus, the term same applied to both the value of the decomposed numerical subexpressions in relation to the corresponding numbers of the initial sides and the appearance of the final expressions on both sides of the resulting equality.

With respect to the properties that the students mentioned, it was noted that, in transforming a number into its decomposed form and in comparing the decomposed side with the not-yet-decomposed side of the initial equality, there was an implicit reference to the order and addition structures for whole numbers and a more explicit reference to the decomposability of these numbers. There was also a written, but implicit, reference to the reflexive property of equality when describing the final decomposed equality: “Because if I look and compare each number, they are the same and obviously it is the same.” However, the students’ lack of precise names for these properties of number and of equality led to their using more informal, everyday language to describe them. This was seen in their manner of referring to decomposing in the last question of Task-set B—a question on describing in a general way their approaches to rewriting the equalities so as to show their truth-value—with terms such as simplifying, making the numbers smaller, and converting the numbers. This was, however, not unexpected. These students had not had any prior experience with explicit statements of properties, or structure-based approaches to equivalence, in their earlier classroom mathematical experience. Even that part of the question that included the phrase, “regardless of the numbers involved in the equality,” seemed somewhat unusual to them. Nevertheless, their ability to transplant familiar words to unfamiliar situations was a first step for them in the building of new mathematical discourses related to equivalence. We hasten to add however that, while students never used the term equivalence (and only very rarely, the term equal), the language that they did use provided evidence of their growing sense of how equalities could be transformed to show equivalence (i.e., sameness), and this by means of top–down structural decompositions that relied on number properties related to the addition structure, such as place-value, halving, doubling, and so on.

6.2 The collapsing of invisible and visible sameness in justifying their structure-based equivalence transformations

In our conceptual framework, we introduced a distinction between invisible and visible sameness, and used this distinction in analyzing students’ talk about the vertical transformations carried out in the generating of equivalent numerical subexpressions and the resulting horizontally-displayed common form for each side of the equality. We expected that the students would differentiate between the invisible and visible aspects of sameness in their justifications, but they did not use these exact terms. As highlighted above, they referred to the vertical, invisible sameness as: “it looks different but gives the same result”; and for the horizontal, visible sameness as: “Because if I look and compare each number, they are the same and obviously it is the same.” While the term, same, was used for both cases of “it”, for the former they were referring to particular numerical values that were safeguarded by the top–down, vertical transformations; and for the latter, to the invisible total of each side as represented by the same numbers on each side. So, in fact, there were really two types of invisible sameness inherent to the students’ statements—both of them referring to unseen computational totals. In other words, both the initial untransformed numbers and their corresponding transformed equivalents, vertically speaking, and the identical transformed numbers on each side of the equal sign, horizontally speaking, shared something in common: some same, but hidden, numerical results. This brings us back to an earlier statement made by Melhuish and Czocher (2020) that “we often mean that the two objects are the same in some (meaningful) respect but are not the same in every respect” (p. 39, italics in original). The form into which the numbers of the initial equality had been transformed was clearly different from their original version; however, their numerical values remained the same, albeit hidden from view.

What is of particular interest is that it was the aspect of the “same numerical result”—a computational underpinning to their structural approach—that allowed the students to collapse the vertical invisibility and horizontal visibility of equivalence into a single type of sameness justification. This suggests that computational underpinnings may be more central to structure-based transformational work than previously acknowledged, not only at primary school, but also later on in secondary school algebra. Examples to support this point of view can be found in several past research studies as, for instance, in a study with eighth-grade students (13- and 14-year-olds) on the introduction of various transformations involved in algebraic equation-solving (Kieran, 1987). When the students were presented at the end of that eight-session study with questions related to the equivalence of pairs of equations, numerical thinking clearly provided a basis for their decisions about sameness in what was patently an algebraic context. In fact, the kind of control afforded by the numerical in algebraic activity has recently led some researchers to conceive of algebraic thinking more broadly and to rename it as numerico-algebraic (Pilet & Grugeon-Allys, 2021) and as arithmetico-algebraic thinking (Fernando Hitt, personal communication, May 2021).

We emphasize, moreover, that the numerical is not only useful but also necessary for certain kinds of algebraic activity. When students encounter algebraic expressions that represent rational functions, a property-based structural perspective will not be sufficient. A numeric perspective will also be required—that is, the domain needs to be accounted for when considering equivalence between, for example, the expression (x − 2)/(x2 − 2x) and its structurally-transformed version 1/x (Solares & Kieran, 2013; see also Kieran et al., 2013). It may seem ironic that the development of a structural view of equivalence at the primary school level tends to involve some dampening down of the usage of the computational, but that both the computational and the structural dimensions of equivalence will be needed within the later study of algebra.

An additional aspect that is suggested by our finding related to the students’ notion of the hidden numerical values that characterize both the invisible top–down equivalences of initial and final equalities and the visible left–right equivalence of the resulting transformed numerical equality concerns the question of making transitions to letter-symbolic algebra—a world that also comprises hidden numerical values. Herscovics and Kieran (1980) have described a didactical approach to introducing algebraic equations with 7th and 8th graders that begins with numerical equalities that are identities and that involves hiding one of the numbers with a literal symbol. While space constraints do not permit a detailed description of the approach and how it is extended to include the top–down equivalences entailed in equation-solving, suffice it to say that the meaning given by the students to equation-solving was that of uncovering the hidden number by means of successive transformations that were oriented toward maintaining the truth-value of each step. In view of the way in which our 6th graders came to justify the equivalence transformations that they carried out in order to indicate the truth-value of their rewritten numerical equalities, we suggest that an avenue for future research would be to design a study that brings together the didactic approaches of the prior research reported by Herscovics and Kieran and of the research presented in this paper within an integrated sequence that transitions from numerical to algebraic transformational equivalences.

6.3 The linking of exchanging and sameness in students’ structural approaches to generating and justifying equivalences

We now return to a point that arose earlier within the literature review: the claim by Jones (2009) that a distinction ought to be made between the “sameness” meaning for the equals sign, which promotes distinguishing statements by truthfulness, and the “can be exchanged for” meaning, and his follow-up argument that one meaning does not imply the other. Jones’s claim is based on his research involving the Sum Puzzles digital environment that promoted a substitutive meaning for the equals sign and where students were presented with tasks such as that of transforming 31 + 40 into a single result by selecting from among the various equality tiles that were offered (e.g., 31 = 30 + 1, 30 + 1 = 1 + 30, etc.). These tiles allowed the students to make the following repeated substitutions: 31 + 40, 30 + 1 + 40, 1 + 30 + 40, 1 + 70, leading to the desired single result of 71 (see also Jones & Pratt, 2012). With such tasks, some of the students made substitutive exchanges without considering truthfulness, which was deemed to provide support for the notion that sameness and exchanging/substitution are two distinct facets of equivalence. However, aspects of our study prompt an alternative interpretation with respect to the sameness versus exchanging debate.

If one takes into account the properties underlying those very substitutive acts—such as, when 30 + 1 is substituted in Sum Puzzles for 31—then it would seem reasonable to suggest that it is precisely because 30 + 1 is the “same” as 31 (i.e., by the addition structure, 30 + 1 = 31, and by symmetry 31 = 30 + 1) that the substitution is tenable. From this perspective, exchanging depends on the support of sameness. In all fairness, however, it should be stated that Jones’s tasks were quite different from the ones used in our study. Tasks requiring students to choose from among a set of given exchanging options in order to solve sum puzzles are markedly not the same as tasks that involve generating equivalent numerical equalities. As our results suggest, tasks that explicitly ask students to rewrite an equality in such a way that demonstrates its truth-value can lead them to become sensitive to value-preserving transformations/substitutions. In such tasks, possible distinctions between sameness and exchanging would seem to fade completely.

To the same extent that exchanging depends on the support of sameness, it is also suggested that sameness can be demonstrated by exchanging. Recall from the literature review the case of Miguel who signaled the truthfulness of the equality 7 + 7 + 9 = 14 + 9 by mentally exchanging the 7 + 7 for 14 (Molina et al., 2008). More generally, determining the truthfulness of simple equalities—even when done by means of computing each side and substituting the computed totals for the given addition operation(s)—can be said to involve exchanging. Thus, we suggest that determining truthfulness/sameness within equivalence tasks of the kind used in our study, as well as within some of the tasks of the earlier empirical research on young students’ views of equality, will usually always involve some exchanging action, just as exchanging usually always involves the support of sameness. And this argument extends beyond the numerical domain to that of the algebraic.

To conclude this discussion, we come back to our construct of invisible sameness and propose that this construct encapsulates an essential characteristic of the duality of exchanging and sameness within equivalence transformations. Even if the students participating in our study did not use the exact terminology of invisibility when referring to the sameness of the equivalent expressions they were generating, we would argue nonetheless that the notion of invisible sameness captures the spirit of the truth-maintaining exchange process whereby the result of an equivalence transformation is invisibly the same as its pre-transformed version—such sameness being justifiable by properties, broadly speaking. Taking a cue from our students who referred to both truth-value and exchanging with the singular term of “sameness,” we suggest that invisible sameness is integral to activity related to the top–down equivalence transformations of numerical equalities, and at times to left–right equivalence too.

7 Concluding remarks

Sameness has been the fundamental theme of this paper. We have described the ways in which students expressed sameness as they came to rewrite numerical equalities in a structural way that showed that the equalities were true, but without computing the totals of each side. To supplement their computational way of indicating that an equality was true, they came to transform each side of the given equalities in such a way that produced a third common form—a common form that explicitly revealed the identity of the equality. That is, the invisible sameness of their top–down transformations yielded a visible horizontal sameness to the resulting rewritten equality. However, for the students, both the transformed vertical and horizontal equivalences had a certain invisible aspect, a hidden sameness with respect to uncalculated numerical values.

With the sensitizing that was aimed at in our study with respect to the role played by value-preserving, top–down equivalence transformations in the rewriting of equalities, we conjecture that these students will be better prepared for algebra. Moreover, we dare to add that the students’ numerical and computational knowledge, which underpinned their interpretations of the sameness of the structurally-based equivalence transformations that they carried out with numerical equalities, may also be key to controlling the equivalence transformations that they will be called upon to engage with in their later introduction to the alpha-numeric expressions and equations of algebra.

In closing, we point to one of the limitations of our study in that the equalities involved only addition operations. Previous research, for example, that of Herscovics and Linchevski (1991), has shown how equalities that contain both additions and subtractions are more difficult to parse for students of this age. In their study, students were seen to compose part of the expression 237 + 89–89 + 67–92 + 92 into 237 + 89–89 + 67–184. Had we included equalities containing expressions such as these in our study, it could have been much more difficult for the students to relate the (possibly incorrect) transformations they carried out on one side of the equality with equivalent transformations to be carried out on the other side so as to yield an equality that was an identity. So, what might be considered a limitation was really a blessing. It helped with the students’ coming to develop a structural orientation to equality transformations that they justified in terms of sameness, as well as leading to insights on the part of the researchers regarding one small facet of students’ early algebraic thinking.