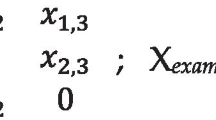How a liquidity saving mechanism affects bank behavior in interconnected payment networks

Abstract

A surge in banks’ liquidity needs increases settlement costs that could burden the functioning of the real economy through its impact on banks’ lending behavior. A liquidity saving mechanism (LSM) can help reduce banks’ liquidity needs, but it could also affect banks’ strategic behavior. To understand how an LSM affects banks’ behavior in a real-time gross settlement system, this study models settlements in a day as a timing game in which banks decide when to make payments, thereby trading off the cost of delaying payments against the cost of borrowing liquidity. An LSM provides a partial offsetting service, whose direct effect is to decrease the cost of liquidity associated with payments that are offset. The study’s stylized analyses reveal that an LSM indirectly affects banks’ strategic behavior in a network context. Without an LSM, a positive strategic spillover effect can arise through the network of payments, which an LSM can dismiss by cutting off the underlying payment network. To demonstrate its welfare impact along with the network structures, this study theoretically analyzes a class of core-periphery networks. The density of the network is shown to have implications on the welfare consequence of adding an LSM. From a policy perspective, the implications on a policy mix between an LSM and the fee setting for intraday lending are discussed.

1 Introduction

This study theoretically examines the effect of a liquidity saving mechanism (LSM) that operates in an interbank settlement system or a large-value payment system. Ongoing shift to real-time processing of settlements underlies the recent introduction of LSMs in several interbank settlement systems. There is also a debate in the effectiveness of LSMs in distributed ledger environments, which might be adopted in future interbank settlement systems.¹ The stylized analyses in this work provide basic implications applicable to both the currently adopted centralized environments and distributed ledger environments.

LSMs have been equipped to mitigate undesirable consequences associated with real-time processing of settlements. Over the last 30 years, real-time processing of settlements called real-time gross settlement (RTGS) systems largely replaced the traditional designated-time net settlement (DTNS) systems.² An RTGS system enables fund settlements on a gross basis while a DTNS system executes settlements typically at several designated times a day. A merit of an RTGS system is that it helps reduce banks’ credit risk associated with unsettled payments that could be voluminous in DTNS systems. However, banks’ liquidity needs for their settlements tend to be much larger than those under DTNS systems. Under conventional arrangements, there are typically no interbank markets for intraday credit; instead, a central bank provides intraday lending against the banks’ liquidity needs. By providing intraday lending, a central bank would be prone to credit risk associated with its daylight overdraft; thus, intraday lending is typically provided with fee or collateral. A surge in banks’ liquidity needs for their settlements increases settlement costs overall, which could burden the functioning of the real economy through its effects on banks’ lending behavior. Thus, reducing banks’ liquidity needs is an important policy concern under an RTGS system.

An LSM is developed to equip an RTGS system with a function of offsetting payments. A typical functioning of LSM is to provide a central queue, to which banks can choose to send their payments instead of making payments to their creditors, and the payments within the queue are offset bilaterally or multilaterally when the corresponding payments are found within the queue.

Thus, LSMs are primarily expected to reduce banks’ liquidity needs, but they could affect banks’ behaviors in the relevant strategic context. In daily settlements, banks can decide when to make their payments considering the costs of financing liquidity and of delaying payments. Since using incoming payments made by other banks effectively means zero liquidity financing cost, each bank would choose to time its payments, thus avoiding such costs. The addition of an LSM might influence banks’ timing decisions and consequently affect realized costs. The welfare consequence in terms of the costs of liquidity and delay is not necessarily apparent until the underlying strategic interactions are explicitly investigated.

This study provides theoretical analyses in this direction, based on the setting in Martin and McAndrews (2008, 2010) but departs from their works by revealing a different aspect of the effects of an LSM. Martin and McAndrews (2008, 2010) focus on the implications of an LSM’s queuing arrangements, by implicitly assuming the availability of offsetting even without an LSM. By contrast, this study focuses on the implications of an LSM’s offsetting by ruling out the possibility of offsetting for cases without an LSM.

The key observation in examining the effects of LSMs’ offsetting service is that an LSM, in reality, is able to offset at best only part of all outstanding payments in a day. This is understandable since offsetting mechanisms essentially reintroduce credit risk, and the risk becomes larger when an LSM holds a larger number of payments in a queue so that larger cycles of payments can be offset.

Based on this observation, this study investigates the implications of a partial offsetting service provided by LSMs. In this respect, Hayakawa (2018) analyzes the effects of a partial offsetting service in the context of a central clearing counterparty. Hayakawa (2018) utilizes a graph theoretic framework invented by Hayakawa (2020), in which banks’ timing decisions are completely abstracted, and the total required funds are derived in a non-strategic manner along with each scenario. The most relevant finding in his work, a bit surprisingly, is that partial offsetting could increase the total required funds. This negative effect can arise when partial offsetting cuts off the underlying network of payments.

In contrast to the finding in Hayakawa (2018), our main finding is that partial offsetting can further increase the total required funds through its effect on banks’ strategic behavior. In particular, we find a potential positive spillover effect through the connection of a payment network that serves to economize the funds used for the settlements. The addition of partial offsetting in the form of an LSM could dismiss the positive spillover effect by cutting off the underlying network. Thus, adding an LSM could increase liquidity costs. The total welfare consequences need to be examined together with the associated delay costs.

To demonstrate the welfare consequences, our investigation is based on a tractable class of core-periphery payment networks.³ In each core-periphery payment network, participant banks are classified into two groups: core and periphery banks, wherein the former serves as a hub to the latter. An LSM provides partial offsetting, such that only the payments among the core banks are offset. The effect is examined with the density of the network. To state informally, a network is considered more dense when periphery banks are more tightly connected with each other. The analyses show that the density has a nonlinear impact on the welfare consequence of an LSM, particularly in relation to the spillover effect arising through the payment network.

From a policy perspective, the optimal policy of a central bank on the fee level for intraday lending is incorporated to examine the effect of an LSM. Implications on the policy mix between an LSM and the fee setting are discussed.

1.1 Relevant literature

The behavior of banks under RTGS systems have been theoretically examined in several papers. Angelini (1998) points out that banks might excessively delay payments to avoid borrowing costs. Bech and Garratt (2003) provide a game-theoretic framework discussing the implications of several credit regimes, and show that intraday liquidity costs tend to deter efficient coordination. Mills Jr. and Nesmith (2008) provide similar results by extending the model of Bech and Garratt (2003).

Several studies theoretically examine the welfare implications of LSMs in view of the strategic interactions between banks. Martin and McAndrews (2008, 2010) show that queuing availability can have a positive effect by mitigating coordination problems. Our study departs from their setting to focus on the effects of offsetting instead of queuing. Willison (2005) analyzes the implications of offsetting in a setting different from that adopted in this study. He examines complete network structures of payments, for which an LSM can offset all outstanding payments. He shows that an appropriately equipped LSM tends to improve welfare. By contrast, our work shows a possible negative effect when an LSM can offset only part of all outstanding payments.

The effects of an LSM are also analyzed in Kobayakawa (1997); Roberds (1999); Kahn and Roberds (2001); Galbiati and Soramäki (2010), and Jurgilas and Martin (2013). Our work is different especially in that it examines the implications of the structure of the underlying payment network.

The rest of this paper is organized as follows. Section 2 introduces the setting. Section 3 provides the analysis. Section 4 presents the conclusions. The Appendix includes some of the proofs and complementary analyses.

2 The setting

We consider an interbank settlement system with the set of banks $I=I_{core}\cup I_{per}$ with $|I|=N$ and one settlement institution, where $I_{core}$ and $I_{per}$ denote the sets of “core" and “periphery" banks, respectively. Each bank is either a core or periphery bank. The banks are risk-neutral strategic agents, while the settlement institution is a non-strategic agent.⁴

We examine settlements within a day, which consists of four periods: opening, morning, afternoon, and closing periods. Periods are simply referred to as 1, 2, 3, and 4. There are two types of payments in a day; payments among the banks themselves and payments between the banks and the settlement institution. The former type of payments are specified with a payment network $\psi =\{p_{i,j}\in \{0,1\} \}_{i,j\in I}$, where $p_{i,j}$ indicates the size of the payment made by bank i to j. Each core bank makes and receives two payments, while each periphery bank makes and receives one payment. Each of the payments is settled in one of the periods, or delayed to the next day. A bank incurs unit delay cost $\gamma _{t-1}$ when a payment made by the bank to another bank is settled in period t, for $t=2,3,4$, where $\gamma _4$ is for the delay to the next day.

For payments between banks and the institution, each bank either makes and receives no payment, or makes and receives one unit of payment. These payments occur between periods 2 and 3, such that a bank must first send a payment to the institution before receiving a payment.⁵ Each bank learns whether it has a payment to make and receive against the institution only at the time of the payment to the institution; thus, these payments work as temporary liquidity shocks for banks. Concerning the shocks, the type of bank $i\in I$ is denoted with $\theta _i\in \{high, low\}$, where the banks of the high type face the shock with probability $\sigma ^h$, while the banks of the low type with probability $\sigma ^l$, with $0< \sigma ^l<\sigma ^h<1$. We let $\sigma ^h$ and $\sigma ^l$ also denote the actual ratio of the banks that face the shocks within the banks of the high- and low-shock types, respectively.

Each bank can borrow funds⁶ from the central bank (CB) in periods 1 and 4 (opening and closing periods) and also at the middle of the day when making payments to the institution.⁷ The borrowed funds must be returned at the end of the day. The unit cost of fund from the CB is x. There is no market for banks to borrow intraday funds.

Remarks

Some remarks are warranted concerning the period structure and the assumption on the availability of intraday lending adopted in this study. The period structure in this study is highly stylized in that potentially much more frequent decisions of banks in the real-world interbank settlements are reduced into four periods; however, it is interpreted as a generalization of the standard two period structure, as adopted in Martin and McAndrews (2008, 2010).⁸ Combined with the assumption of non-availability of intraday lending in periods 2 and 3—which could be interpreted as a consequence of an optimal policy of the CB in serving intraday lending, as mentioned in Footnote 7—, the setting in this study successfully reveals the possibility that payments made in a day are settled in a coordinated manner with circulating funds, thereby economizing the funds for the settlements considerably even without an LSM. From this perspective, the purpose of these settings is to analytically highlight the negative effects of an LSM, particularly when settlements are possible to be made in such a coordinated manner.

2.1 The setting without an LSM

The setting without an LSM is described below. The setting with an LSM is described separately in Sect. 2.3.

Before entering period 1, a payment network between the banks $\psi$ is given. Each bank initially has zero reserve. Let $\psi _t$ denote the remaining payments at the beginning of period $t=1,2,3,4$, where $\psi _1=\psi$. Moreover, let $\{F_t^i\}_{i\in I}$ denote the amount of funds held by bank i at the beginning of period $t=1,2,3,4$, where $F_1^i=0$ for every $i\in I$. We assume that $\psi _t$ and $\{F_t^i\}_{i\in I}$ are common knowledge at the beginning of period t. At any point in a day before the liquidity shocks occur, every bank $i\in I$ knows the own type with regard to the liquidity shocks $\theta _i$ and the probability of facing the shock of each type $\{\sigma ^l, \sigma ^h\}$.

In period 1, the banks simultaneously decide whether to make each of their payments to other banks or delay it to later periods. The payments made are settled by the settlement system in a sequential manner. Formally, let $P_1\subseteq \psi$ denote the set of payments that banks have decided to make in period 1. Let $Seq(P_1)$ denote the set of the sequences (i.e., permutations) of $P_1$. In the process of the settlements, one of the sequences $s\in Seq(P_1)$ is realized by the settlement system. When indexing $s=(p^1, p^2,.., p^k)$ (for $|P_1|=k$), the payments are settled one by one from the head $p^1$ to the tail $p^j$. When a payment $p_{i,j}$ that has been made by bank i to j is settled, the specified amount of funds $p_{i,j}$ is transferred from bank i to j. We assume that every sequence $s'\in Seq(P_1)$ is realized with equal probability. The sequential settlement process intends to express settlements on a purely gross basis, excluding simultaneous settlements wherein payments are offset or settled without any fund transfer. Whenever banks face shortage of funds, the CB promptly provides the necessary amount of funds in the form of intraday lending.⁹

In period 2, it is assumed that banks cannot borrow funds. The banks simultaneously decide whether to make each of their remaining payments to other banks “responsively" or delay the payment. At the same time, a core bank that chooses to make multiple payments “responsively" additionally prioritizes the payments as to which is to be paid earlier. Formally, let $P_2$ denote the set of payments that are chosen to be paid responsively in period 2, and $P_2^i\subseteq P_2$ denote the payments made by bank $i\in I$ within $P_2$. Specifically, for $P_2^i=\{p_{i,j'}, p_{i,j''}\}$ with some $j',j''\in I$, core bank i further chooses the order of the payments, such that $(p_{i,j'}, p_{i,j''})$, which indicates that the former payment is endowed with a higher priority. When a bank chooses to make one of its payments “responsively," the payment is settled either with funds held at the beginning of the period or funds received in the course of the settlements within the period. When a bank has decided to make multiple payments, the one with the higher priority is settled first. The settlement system settles the given payments in a sequential manner as formally described below. Payments that are not settled at the end of the period are all delayed to later periods.

Settlement procedure in period 2¹⁰

Step (0) Set initially $\hat{F}_2^i:= F_2^i$; $\hat{P}_2^i:= P_2^i$ for every $i\in I$, and set $i_{current}:=\emptyset$.

Step (1) The settlement system arbitrarily chooses bank $i\in I$ for which $\hat{P}_2^i\ne \emptyset$ and $\hat{F}_2^i\ne 0$ are satisfied, and set $i_{current}:=i$. If there is no such bank, the process terminates.

Step (2) Suppose that $p_{i_{current},j}$ is endowed with the highest priority within $\hat{P}_2^{i_{current}}$. Then, the system settles $p_{i_{current},j}$ by transferring one unit of funds held by bank $i_{current}$ to j. Set $\hat{P}_2^{i_{current}}:= \hat{P}^{i_{current}}_2{\setminus } p_{i_{current},j}$, $\hat{F}_2^{i_{current}}:=\hat{F}_2^{i_{current}}-1$, $\hat{F}_2^j:=\hat{F}_2^j +1$. If $\hat{P}_2^j\ne \emptyset$, set $i_{current}:=j$, then repeat Step 2. Otherwise, go back to Step 1.

At the time of the liquidity shocks between periods 2 and 3, the CB promptly provides intraday funds whenever banks are short of funds in making payments to the institution. In period 3, the decisions of the banks and the settlement process are all the same as in period 2. Moreover, those in period 4 are the same as in period 1.¹¹ At the end of the day, the funds borrowed from the CB are returned. Banks incur costs of borrowing and also costs of delaying payments.

2.2 Core-periphery network

For the structures of the payments among the banks, we define a class of core-periphery network with the intention of capturing two observations in reality, summarized as:

• (1) Payments among core banks are more interconnected than those among periphery banks, and

• (2) Core banks tend to make larger payments than periphery banks.

Definition 2.1

Core-periphery payment networks

${\hat{\Psi }} _{(K,W)}$ with $1< K <W$ denotes a class of core-periphery payment networks among banks I such that:

• (1) Banks are divided into K core banks $I_{core}=\left\{ i_{core, k}\right\} _{k=1,2,..K}$ and K groups of periphery banks $I_{per}=\left\{ I_{per, k}\right\} _{k=1,2,..,K}$ where $|I_{per,k}|=W$ for every $k=1,2,...,K$;

• (2) Each core bank is given two units of payments to make such that one is to a core bank and the other is to a periphery bank. Moreover, it has two units of payments to receive, where one is from a core bank, and the other is from a periphery bank;

• (3) Payments among the core banks form a cycle;

• (4) Each periphery bank has one unit of payment to make and one unit to receive; and

• (5) For each group of periphery banks indexed with $k=1,2,..K$, the payments among $I_{per,k}$ constitute a path, in which the starting node has a payment to receive from a core bank, while the ending node has a payment to make to a core bank.

Given $\psi \in {\hat{\Psi }}_{K,W}$ with $1<K<W$, index the banks with $I=\{1,2,...,N\}$, such that $j'=j+1$ for periphery banks $j,j'\in I_{per}$ whenever $p_{j,j'}=1$.

Throughout this study, we focus our analyses on $\Psi _{(K,W)} \subset {\hat{\Psi }}_{(K,W)}$ for each (K, W) to avoid certain technical complication, by adding a constraint that is introduced in the latter part of this subsection. Figure 1 shows examples of core-periphery networks within $\Psi _{(3,4)}$.

Fig. 1

Examples of core-periphery networks $\Psi _{(3,4)}$. Note In each network, there are $K=3$ core banks $I_{core}=\left\{ a,b,c\right\}$, and $K=3$ groups of periphery banks. The density for each network is 1, 2, and 3 from the left to the right. Thus, the network on the left is the most dense

Note that $K< W$ intends to describe that payments among core banks are more interconnected than those among periphery banks.

We proceed to define density for a core-periphery network. In Fig. 1, the network on the left (right) side is the most (least) dense among the three networks. Density is shown to be a crucial parameter in analyzing the effects of an LSM.

Definition 2.2

Density

For a core-periphery network $\psi \in {\hat{\Psi }} _{(K,W)}$, suppose that the cycle of payments among core banks is removed. The rest of the payments now form several numbers of disconnected cycles of payments, and we call each cycle a core-separated cycle. Let $d(\psi )$ denote the density for $\psi$, which indicates the number of core-separated cycles. Further, for $\psi , \psi ' \in \Psi _{(K,W)}$, we say $\psi$ is more dense than $\psi '$ if $d(\psi )<d(\psi ')$.

2.2.1 Eulerian property and aligned networks

There are two properties of networks, Euler property and alignment property, which help simplify our analysis.

Eulerian property holds for the class of core-periphery networks ${\hat{\Psi }} _{(K,W)}$.

Observation 2.1

Eulerian property¹²

For arbitrary core-periphery network $\psi \in {\hat{\Psi }} _{(K,W)}$ with $1<K<W$, the directed graph $\psi$ constitutes a Euler graph.

Now, we restrict our attention to core-periphery networks that are aligned.

Definition 2.3

Alignment property

A core-periphery network is aligned if any combination of non-punctured cycles¹³ within the network is not mutually vertex-twisted.

The definition of vertex-twisted is provided in Appendix A.1, together with the necessary preparation of graph-relevant terminologies. Informally, we say that a network is aligned when there is no inconsistency in the directions among any combination of the non-punctured cycles. We let the aligned networks within ${\hat{\Psi }} _{(K,W)}$ constitute $\Psi _{(K,W)}$. Note that the networks shown in Fig. 1 are all aligned networks.¹⁴ For our analysis, observe that there exist sufficiently many aligned core-periphery networks in the following sense.

Observation 2.2

(Sufficiently many aligned networks)

For each $\Psi _{(K,W)}$ with $1<K<W$, there is at least one aligned network $\psi \in \Psi _{(K,W)}$ that attains each $d(\psi )=1,2,...,K$.

When calculating borrowing costs, we will see that the Eulerian property simplifies the derivation of the minimum possible total borrowed funds, while the alignment property does so concerning the maximum possible total borrowed funds. These simplifications allow us to focus on the implications of density in discussing welfare consequences.

2.2.2 Distribution of the types concerning the liquidity shocks

We focus on a specific class of distribution concerning the heterogeneity of liquidity shocks, which has a segregated property. For a given core-periphery network $\psi \in \Psi _{(K,W)}$, we assume that every core bank is of the high type. For the periphery banks, there exists $L\in \left\{ 1,2,..,K\right\}$ where every bank $i\in I_{per,L}$ is of the low type, while all the other periphery banks are of the high type.

Note that the positive correlation between the volume of the payments and the probability of facing a liquidity shock intends to capture reality, while the small portion of the low type is to analytically highlight the negative effect of an LSM. For the sake of our subsequent analyses, denote the average probability of liquidity shocks as ${\tilde{\sigma }}=\frac{1}{N}(N^{h}\sigma ^{h}+N^{l}\sigma ^{l})$, where $N^{l}=|I_{per,L}|, N^{h}=N-N^{l}$.

2.3 The setting with an LSM

An LSM is added in period 1. The LSM provides a queue, and each bank now has an option to put each payment into the queue. For queued payments, the LSM conducts partial offsetting. Specifically, for given $\Psi _{(K,W)}$, the LSM offsets all queued payments when they constitute cycles with less than or equal to K number of payments.¹⁵ Thus, when a bank queues a payment and it is offset, the bank does not incur any borrowing cost. If a payment is queued but turns out not being offset, it is released to the original bank at the end of period 1. From period 2 onward, all previous settings are maintained.

3 Analysis

We focus on subgame-perfect Nash equilibria in pure strategies. Let $S_i$ denote the set of available strategies for bank $i\in I$. Let $(s_1,s_2,..., s_N)$ denote a strategy profile, and $\pi _i(s_i\in S_{i}, S_{-i})$ denote the expected payoff of bank i under its own strategy $s_i$ and the strategies of the other banks $S_{-i}=(s_1,s_2,.., s_{i-1},s_{i+1},,,..s_N)$.

3.1 Real-time gross settlement without a liquidity saving mechanism

3.1.1 Strategies

A bank’s strategy consists of the decisions in period 1, 2, 3, and 4. For the decisions in period 4, we assume that $\gamma _4$ is sufficiently large so that any bank chooses to make all the remaining payments instead of delaying any of them. Concerning the decisions in period 3, it is always better for a bank to choose to make all the remaining payments responsively instead of delaying any of them. The remaining decision that a bank must make in period 3 concerns prioritizing the outstanding payments. For analytical tractability, we focus on the situations in which the banks successfully coordinate such that the largest amount of payments is settled.¹⁶¹⁷

For the decisions in period 2, we make the same assumption as in period 3 concerning the decisions on the priorities of making payments responsively. In period 2, a bank potentially has an incentive to delay payments, especially when it has funds at the beginning of the period, since the funds insure a bank from the risk of temporary liquidity needs that can arise between periods 2 and 3. In the analysis, we focus on situations where every bank chooses to make all the remaining payments responsively, by making the following assumption.

Assumption 3.1

$\frac{\gamma _{1}}{x} < \frac{\gamma _2}{x}-\sigma ^h$, where x is defined as the unit cost of intraday borrowing from the CB.

This assumption serves the following purpose. Suppose that a bank holds an outstanding payment but does not have funds at the beginning of period 2. Suppose further that the bank receives funds within period 2 through payments made by other banks. In this case, making the payment in period 2 costs $\gamma _1 + \sigma ^h x$ in expectation, which is smaller than the cost of delaying, $\gamma _2$, that is incurred by the bank when delaying the payment to period 3.

In period 1, each bank faces a non-trivial choice of whether to make each of its payments or delay it. Thus, given our focus on the types of equilibria (which is stated later), it is sufficient to examine the following strategies. The strategy for each periphery bank j is $s_j\in \{P,D\}$, where P is for making the payment in period 1, while D states that the bank delays the payment in period 1, makes the outstanding payment responsively in periods 2 and 3, and makes the outstanding payment in period 4. The strategy for each core bank i becomes $s_i\in \{ (P,P), (P,D), (D,P), (D,D) \}$, where P and D specifies the decisions for each payment in the same manner, and in each parenthesis the former shows the decision on the payment to a core bank, while the latter is to a periphery bank. Note that the choices on the priority when making multiple payments in periods 2 and 3 are implicit. It is assumed that the choices on the priorities are formed through coordination.

We focus on two types of strategy profiles as the candidates of equilibrium in our analysis.

• 1. All-pay: $s_{j}=P$ and $s_{i}=(P,P)$, for every $j\in I_{per}$ and $i\in I_{core}$.

• 2. Sole-pay: There exists $j\in I_{per}$ such that $s_{j}=P$, while $s_{j'}=D$ for every $j'\ne j\in I_{per}$, and $s_{i}= (D,D)$ for every $i\in I_{core}$.

We refer to the former type of strategy profile as $S^1$, and the latter type as $S^2$. The payments in periods 2 and 3 are assumed to be made in the best coordinated manner.¹⁸ Thus, combined with the Eulerian property, observe that all the payments made in period 2 are successfully settled for any $S^2$ type strategy profile.

Note that under our core-periphery networks, there are various other types of strategy profiles that can achieve equilibria. Nonetheless, our limited focus is rationalized, such that each of the $S^1$ and $S^2$ type strategy profiles could become the first best equilibrium, as shown in Sect. 3.1.3.

3.1.2 Equilibrium and spillover effect

To examine the conditions for each of $S^1$ and $S^2$ to be an equilibrium, we assess the relevant payoffs. First, we examine the payoff of core bank $i\in I_{core}$ under the others’ strategies $S_{-i}$, in which there is a periphery bank $j\in I_{per}$ with $s_j=P$. Observe that for each core bank, there are two payers–one core bank and one periphery bank. Let $s^p_{i}\in \{ \{P,P\}, \{P,D\}, \{D,D\} \}$ denote the unordered pair of the strategies of the payers to bank i.¹⁹

The payoffs of core bank $i\in I_{core}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=\{P,P\}$ and there exists a periphery bank $j\in I_{per}$ that takes $s_j=P$, are derived as follows:

$$\pi _{i}((P,P),S_{-i})= -\left(\frac{1}{6}(2x)+\frac{1}{2}x+\frac{1}{3}\sigma ^{h}x\right)=-\frac{5}{6}x-\frac{1}{3}\sigma ^{h}x,$$

(1)

$$\pi _{i}((P,D),S_{-i})= \pi _{i}((D,P),S_{-i})= -\frac{1}{3}x-\frac{2}{3}\sigma ^{h}x-\gamma _{1},$$

(2)

$$\pi _{i}((D,D),S_{-i})= -\sigma ^{h}x-2\gamma _{1}.$$

(3)

For Eq. (1), bank i borrows two units of funds in period 1 with probability $\frac{1}{6}$, one unit with $\frac{1}{2}$, and none with $\frac{1}{3}$.²⁰ The insurance effect of early payment prevents bank i from borrowing when a liquidity shock arises. For Eq. (2), bank i borrows one unit in period 1 with probability $\frac{1}{3}$ and none with $\frac{2}{3}$. At the end of period 1, the bank holds two units of funds in the former case, and one unit for the latter. Note that one unit of funds held at the end of period 1 is always used to settle the bank’s own remaining payment. Thus, the insurance effect of early payment similarly works. For Eq. (3), observe that the payments are surely settled in period 2.

The payoffs of core bank $i\in I_{core}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=\{P,D\}$ and there exists a periphery bank $j\in I_{per}$ that takes $s_j=P$, are derived as follows:

$$\pi _{i}((P,P),S_{-i})=-\frac{1}{3}(2x)-\frac{2}{3}x =-\frac{4}{3}x,$$

(4)

$$\pi _{i}((P,D),S_{-i})= \pi _{i}((D,P),S_{-i})= -\frac{1}{2}x-\frac{1}{2}\sigma ^{h}x-\gamma _{1},$$

(5)

$$\pi _{i}((D,D),S_{-i})= -\sigma ^{h}x-2\gamma _{1}.$$

(6)

For Eq. (4), bank i surely receives one unit of payment in period 2, which insures the bank from a liquidity shock. For Eq. (5), when bank i does not borrow funds in period 1, which occurs with probability $\frac{1}{2}$, the bank has no funds at the end of the period. In period 2, the remaining payment is settled with an incoming fund. Thus, again, there is no fund for insurance against a liquidity shock when the bank has not borrowed in period 1.

The payoffs of core bank $i\in I_{core}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=\{D,D\}$ and there exists a periphery bank $j\in I_{per}$ that takes $s_j=P$, are derived as follows:

$$\begin{aligned}&\pi _{i}((P,P),S_{-i})= -2x,\end{aligned}$$

(7)

$$\begin{aligned}&\pi _{i}((P,D),S_{-i})=\pi _{i}((D,P),S_{-i})= -x-\gamma _{1}, \end{aligned}$$

(8)

$$\begin{aligned}&\pi _{i}((D,D),S_{-i})= -2\gamma _{1}-\sigma ^{h}x. \end{aligned}$$

(9)

Next, for periphery bank i, we similarly denote the strategy of the payer as $s_{i}^{p}\in \{P,D\}$.

The payoffs of periphery bank $i\in I_{per}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=P$ are derived as follows:

$$\begin{aligned}&\pi _{i}(P,S_{-i})=-\frac{1}{2}x - \frac{1}{2}\sigma _{i} x, \end{aligned}$$

(10)

$$\begin{aligned}&\pi _{i}(D,S_{-i})=-\gamma _{1}-\sigma _{i} x. \end{aligned}$$

(11)

The payoffs of periphery bank $i\in I_{per}$ with respect to $S_{-i}$ in which $s^p_i=D$ are derived as follows:

$$\begin{aligned}&\pi _{i}(P,S_{-i})= -x,\end{aligned}$$

(12)

$$\begin{aligned}&\pi _{i}(D,S_{-i})= \left\{ \begin{array}{ll} -\gamma _1 -\sigma _i x &{} \text { if } \exists i'\in I, s_{i'}\in \{P, (P,P), (P,D), (D,P)\} \\ -\gamma _2 -\sigma _i x &{} \text { otherwise. } \end{array} \right. \end{aligned}$$

(13)

The following lemma shows the conditions for each $S^1$ and $S^2$ strategy profile to be equilibria.

Lemma 3.1

Equilibrium $S^1$ and $S^2$

1. All-pay: Strategy profile $S^{1}$ is an equilibrium if the following Condition (A) is satisfied.

Condition (A)

• (i) $\frac{\gamma _{1}}{x} < \frac{\gamma _{2}}{x}- \sigma ^{h}$,

• (ii) $\frac{1}{2}(1-\frac{2}{3}\sigma ^{h})\le \frac{\gamma _{1}}{x}$, and

• (iii) $\frac{1}{2}(1-\sigma ^{l})\le \frac{\gamma _{1}}{x}$.

2. Sole-pay: There exists a strategy profile $S^{2}$ that constitutes an equilibrium if the following Condition (B) is satisfied.

Condition (B)

• (i) $\frac{\gamma _{1}}{x} < \frac{\gamma _{2}}{x}- \sigma ^{h}$,

• (ii) $1 -\sigma ^{l} \le \frac{\gamma _{2}}{x}$,

• (iii) $\frac{\gamma _{1}}{x} < \frac{1}{2}(1-\sigma ^{l})$, and

• (iv) $\frac{\gamma _{1}}{x}< 1-\sigma ^{h}$.

Proof

See Appendix A.2. $\square$

A crucial observation regarding the negative effects of an LSM in our later analysis is the possibility of a spillover effect without an LSM that is formally described below.

Proposition 3.1

Spillover effect

Under Condition (B) and when $\frac{1}{2}(1-\sigma ^{h})\le \frac{\gamma _{1}}{x}$,

• (i) If there were no bank of the low-shock type, that is, $\theta ^i=high$ for every $i\in I$, then, any $S^2$ type strategy profile no longer constitutes an equilibrium, but $S^1$ does.

• (ii) A strategy profile within $S^{2}$ wherein $s_j =P$ for $j\in I_{per}$ and $j+1\in I_{per}$ constitutes an equilibrium if and only if $\sigma _{j+1}=\sigma ^l$.

Proof

This is immediate from the best responses of the banks shown in Appendix A.5. $\square$

Part (i) of the proposition states that there is a spillover effect from a bank of the low-shock type to those of the high-shock type for the specified parameter values. Considering the banks’ best responses, which are elaborated in Appendix A.5, for the given parameter values in the proposition, high shock type banks would choose to make payments in period 1 if the payers make payments, while delay payments if the payers delay payments. High-shock type banks adopt such conditional choice on making payments because the merit of the insurance effect of early payment is relatively large. By contrast, low-shock type banks choose to delay payments in period 1 even when the payers choose to make payments, since the merit of the insurance effect of early payment is relatively smaller. Thus, the existence of a low-shock type bank prevents the receiver from making payments in period 1, which further affects similarly the next receiver, and so on. The effects are spilled over along with the payment relation to the whole payment network. Part (ii) of the proposition clarifies that the spillover is sourced from a low-shock type bank that receives a payment but chooses to delay its payment in period 1.

3.1.3 Welfare

Social welfare consists of the social delay costs associated with the delay of payments and the social liquidity costs associated with the funds lent intraday. For the former, we assume that social delay costs are internalized through the costs of delaying payments incurred by the banks. For the latter, we let r express the social cost associated with one unit of intraday lending.²¹

When evaluating social liquidity costs, observe that there is uncertainty on the size of the intraday lending, particularly under the $S^1$ strategy profile where all banks pay in period 1. Social liquidity cost is evaluated under the worst-case scenario.²² Note that the focus of the worst-case scenario is to highlight the positive effect of offsetting through an LSM. The formal expression below summarizes our definition of social welfare $W(S,\psi )$ under a strategy profile S and a network structure $\psi \in \Psi$.²³

$$\begin{aligned} W(S, \psi )= - max\biggl [\sum _{i\in I}r_i(S,\psi )\biggr ] - \sum _{i\in I}\sum _{t=2}^{4}\gamma _{i,t}(S, \psi ) \end{aligned}$$

(14)

where $r_i(S,\psi )$ shows the amount of funds borrowed by bank i multiplied by r, and $\sum _{t=2}^{4}\gamma _{i,t}(S, \psi )$ is the realized delay cost incurred by bank i. max[.] operator indicates the evaluation in the worst-case scenario.

We now introduce the following definition.

Definition 3.1

The first best strategy profile

A strategy profile S under a network structure $\psi \in \Psi$ attains the first best if and only if $W(S,\psi )\ge W(S',\psi )$ for any other available strategy profile $S'$.

The following assumptions are maintained for the rest of the analysis. The assumptions as a whole ensure that either $S^1$ or $S^2$ attains the first best as shown in Proposition 3.2, as well as allow the welfare for each of $S^1$ and $S^2$ to be derived in a simple manner as shown in Lemma 3.2.

Assumption 3.2

$\frac{\gamma _{1}}{r} < 1$.

Assumption 3.3

$1 < (N+K)\frac{\gamma _2}{r}- (N+K+1)\frac{\gamma _1}{r}$.

Assumption 3.4

$\frac{\gamma _{1}}{r} < \frac{\gamma _2}{r}-\sigma ^h$.

Assumption 3.5

$\frac{2K}{N}<{\tilde{\sigma }}<\frac{N-K}{N}$.

Assumption 3.2 serves to limit our focus on a particularly interesting situation where each of $S^1$ and $S^2$ is possible to be the first best. In fact, for the opposite case ($\frac{\gamma _{1}}{r} > 1$), $S^2$ is no more a candidate of the first best while $S^1$ is, as is apparent by the statement of Proposition 3.2.²⁴ Assumptions 3.3 and 3.4 concern the relative sizes of delay costs $\gamma _1$ and $\gamma _2$. Assumptions 3.3 is to focus on realistic situations wherein delaying payments by all banks is not the first best. Assumptions 3.4 is also to focus on realistic situations wherein payments are better to be made in period 2 rather than to be delayed further. Assumption 3.5 is to avoid certain inessential complication in calculating social welfare.

The welfare under types $S^1$ and $S^2$ strategy profiles is derived as below.

Lemma 3.2

For arbitrary $\psi \in \Psi$, we have;

$W(S^{1}, \psi ) = -(N+K)r$, and

$W(S^{2}, \psi ) = -(1+ N {\tilde{\sigma }})r -(N+K-1)\gamma _{1}$.

Proof

See Appendix A.3. $\square$

As Lemma 3.2 shows that the welfare under each of $S^1$ and $S^2$ does not depend on $\psi$, we denote the welfare as $W(S^1)$ and $W(S^2)$, respectively. The following lemma helps to rationalize our focus on the $S^1$ and $S^2$ equilibria, as further elaborated in Sect. 3.1.4.

Proposition 3.2

The first best strategy profiles

• (i) Strategy profile $S^{1}$ attains the first best if $\frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})\le {\tilde{\sigma }}<\frac{N-K}{N}$.

• (ii) Strategy profile $S^{2}$ attains the first best if $\frac{2K}{N}<{\tilde{\sigma }}\le \frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})$.

Proof

See Appendix A.4. $\square$

3.1.4 Regimes

To analyze the functioning of an LSM, we classify the relevant parameter values into two sets, called “liquidity non-precious regime" and “liquidity precious regime."

Definition 3.2

Regimes

(i) Liquidity non-precious regime refers to the parameter values that satisfy Conditions (A) and $\frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})< \tilde{\sigma }<\frac{N-K}{N}$, for which strategy profile $S^{1}$ is an equilibrium and attains the first best.

(ii) Liquidity precious Regime refers to the parameter values that satisfy Conditions (B) and $\frac{2K}{N}<{\tilde{\sigma }}\le \frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})$, for which there exists a strategy profile $S^{2}$ that is an equilibrium and attains the first best.

In each regime, the CB is assumed to optimally choose the fee level x so that the first best strategy profile is an equilibrium. The welfare effect of the addition of an LSM is analyzed given the fee level x for each regime, which would amount to examining a rather short-term effect of the addition of an LSM. The next lemma ensures the existence of each regime.

Lemma 3.3

Existence of the regimes

(i) When strategy profile $S^{1}$ attains the first best, $S^1$ constitutes an equilibrium with sufficiently low fee level x.

(ii) When arbitrary strategy profile $S^2$ attains the first best, there exists a strategy profile $S^{2}$ that constitutes an equilibrium with an appropriate fee level for a sufficiently large $\gamma _2$, such that $\max (2,\frac{1-\sigma ^{l}}{1-\sigma ^{h}}, 1 + \frac{2\sigma ^{h}}{1-\sigma ^{l}}, \frac{1}{1-\sigma ^{h}})<\frac{\gamma _{2}}{\gamma _{1}}$.

Proof

Part (i) is immediate from Condition (A). For Part (ii), Condition (B) is rewritten as:

• (B1) $x<\frac{\gamma _{2}-\gamma _{1}}{\sigma ^{h}}$,

• (B2) $x<\frac{\gamma _2}{1-\sigma ^{l}}$,

• (B3) $\frac{2\gamma _{1}}{1-\sigma ^{l}}<x$, and

• (B4) $\frac{\gamma _{1}}{1-\sigma ^{h}}<x$.

The condition reduces to $\max (\frac{2\gamma _{1}}{1-\sigma ^{l}}, \frac{\gamma _{1}}{1-\sigma ^{h}} )$ $<x$ $<\min (\frac{\gamma _{2}-\gamma _{1}}{\sigma ^{h}}, \frac{\gamma _2}{1-\sigma ^{l}})$. This reduces to $\max (2,\frac{1-\sigma ^{l}}{1-\sigma ^{h}}, 1 + \frac{2\sigma ^{h}}{1-\sigma ^{l}}, \frac{1}{1-\sigma ^{h}})<\frac{\gamma _{2}}{\gamma _{1}}$. The condition is consistent with Assumption 3.1: $\gamma _1 + \sigma ^h r < \gamma _2$, and it is also consistent with the condition for $S^2$ being the first best: $\frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})< {\tilde{\sigma }}<\frac{N-K}{N}$. $\square$

3.2 Real-time gross settlement with a liquidity saving mechanism

3.2.1 Strategies and welfare

Let Q denote the decisions of a bank with respect to one of its payments to another bank, such that the bank puts the payment into the queue in period 1, makes the outstanding payment responsively in periods 2 and 3, and makes the outstanding payment in period 4. Let P and D denote the same decisions as in the setting without an LSM. Observe that for a payment that is made to a periphery bank, putting the payment into the queue will never be settled in period 1. Thus, it is sufficient to examine the following strategies: for periphery bank $j\in I_{per}$, $s_j\in \{P,D\}$, while for core bank $i\in I_{core}$, $s_i\in \{(P,P),(P,D),(D,P), (Q,P),(Q,D),(D,D)\}$.

Under the LSM, we focus on the following three types of strategy profiles, which are shown to constitute equilibria.

1. All-pay: $s_i=(Q,P)$ for every core bank $i\in I_{core}$, and $s_{j}=P$ for every periphery bank $j\in I_{per}$.

2. $d(\psi )$-pay: $s_{i}=(Q,D)$ for every core bank $i\in I_{core}$, and there exists one periphery bank $j\in I_{per}$ with $s_{j}=P$ within each core-separated cycle, and all other periphery bank $j'$ takes $s_{j'}=D$.

3. High-pay: Separate I into $I=\cup _{n=1,2,..,d(\psi )}I^{n}$ so that each constitutes a core-separated cycle of payments. Denote $I^{L}\in \{ I^n \}_{n=1,2,..,d(\psi )}$ when it satisfies $I_{per,L}\subset I^{L}$.²⁵ With these notations, the strategies are specified as follows: $s_{i}=(Q,D)$ for every core bank i that belongs to $I^L$, while $s_{i'}=(Q,P)$ for every core bank $i'$ that does not belong to $I^L$. In addition, $s_j=P$ for every periphery bank j that does not belong to $I^L$. Among periphery banks that belong to $I^L$, there exists a periphery bank $j'$ with $s_{j'}=P$, and all other periphery bank $j''\ne j'$ takes $s_{j''}=D$.

Let $S^{1}_{net},S^{2}_{net}$ and $S^{3}_{net}$ denote each type of the strategy profile, respectively. To perceive $S^3_{net}$, consider core-periphery networks within $\Psi _{(3,4)}$ shown in Fig. 2, where $I_{per,L}$ shows the set of periphery banks with low liquidity shock. Figure 3 shows the payment network after the payments among the core banks are offset and eliminated from the network.

On the left side of the figure, $I^{L}=I$ since all banks belong to one connected payment network. In this case, $S^3_{net}$ states that any one periphery bank takes P, while all the other periphery banks take D. For the network shown in the middle of the figure, $I^{L}$ refers to the banks that belong to a cycle of payments including core banks b and c. $S^3_{net}$ specifies strategies such that one periphery bank $j\in I^{L}$ takes P, while the other $j'\in I^{L}$ takes D, and the core banks b and c take (Q, D). For the other banks that belong to another cycle of payments including core bank a, all the periphery banks take P, and core bank a takes (Q, P). For the network shown on the right side of the figure, $I^{L}$ refers to the banks that belong to a cycle of payments including core bank b. The strategies are similarly specified.

Fig. 2

Examples of core-periphery networks $\Psi _{(3,4)}$ before offsetting

Fig. 3

Examples of core-periphery networks $\Psi _{(3,4)}$ after offsetting. Note For the original networks shown in Fig. 2, this figure shows only the payments that cannot be offset by the LSM

The social welfare under each $S^1_{net}$, $S^2_{net}$, and $S^3_{net}$ is derived as follows²⁶:

$$W(S^{1}_{net})=-Nr,$$

$$W(S^{2}_{net})=-(d(\psi )+{\tilde{\sigma }}N)r-(N-d(\psi ))\gamma _{1},$$

and

$$W(S^{3}_{net})=-(1+\sigma ^{l}N^{l}+\min (N^{h}, n^{h}(\psi )+\sigma ^{h}N^{h}))r - (N-1-n^{h}(\psi ))\gamma _{1},$$

where $N^l = |I_{per, L}|$, $N^h= N-N^l$, and $n^{h}(\psi )=|I{\setminus } I^L|$.

Note that $n^{h}(\psi )$ is later shown to be interpreted as the number of high shock type banks that had enjoyed the spillover effect under RTGS without the LSM but no longer do after the LSM is introduced. Since $n^h(\psi )$ depends on the location of $I_{per,L}$ in general, the relevance of the density is not simply derived. The following lemma shows the relevance for each polar case.

Lemma 3.4

$n^h(\psi )$ and density

For $\psi \in \Psi _{(K,W)}$,

• i) $n^h(\psi ) = 0$ if and only if $d(\psi )=1$, and

• ii) $n^h(\psi ) \le N^h -1$ for arbitrary $\psi \in \Psi _{(K,W)}$, and the equality holds if $d(\psi )=K$ regardless of the location of $I_{per,L}$ in the network $\psi$.

3.2.2 Equilibrium and the effects of an LSM

We first observe that the strategies (P, P), (P, D), (D, P), (D, D) can be ignored for our analysis. Consider deviations of a core bank from each strategy profile $S^1_{net}$, $S^2_{net}$, and $S^3_{net}$, in which core banks take either (Q, P) or (Q, D). The deviation to (P, P) from (Q, P) is worse because it merely increases the cost of borrowing in expectation. Furthermore, the deviation to (P, P) from (Q, D) is worse than the deviation to (Q, P). Thus, (P, P) is excluded from our analysis. For the same reason, (P, D), (D, P), and (D, D) are excluded. Thus, for core bank $i\in I_{core}$, it is sufficient to focus on $s_i\in \{(Q,P),(Q,D)\}$. To examine the relevant payoffs of core banks, observe that under strategy profiles $S^1_{net}$, $S^2_{net}$, and $S^3_{net}$, the strategies of the payers for a core bank $i\in I_{core}$ is $s^P_i\in \{ \{Q,P\}, \{Q,D\} \}$.

The payoffs of core bank $i\in I_{core}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=\{Q,P\}$ and there exists a periphery bank $j\in I_{per}$ that takes $s_j=P$, are derived as follows:

$$\begin{aligned}&\pi _{i}((Q,P),S_{-i})&=-\frac{1}{2}x-\frac{1}{2}\sigma ^{h}x,\end{aligned}$$

(15)

$$\begin{aligned}&\pi _{i}((Q,D),S_{-i})&= -\sigma ^{h}x-\gamma _{1}. \end{aligned}$$

(16)

The payoffs of core bank $i\in I_{core}$ with respect to arbitrary $S_{-i}$ in which $s^p_i=\{Q,D\}$ and there exists a periphery bank $j\in I_{per}$ that takes $s_j=P$, are derived as follows:

$$\begin{aligned}&\pi _{i}((Q,P),S_{-i})&=-x,\end{aligned}$$

(17)

$$\begin{aligned}&\pi _{i}((Q,D),S_{-i})&= -\sigma ^{h}x - \gamma _{1}. \end{aligned}$$

(18)

All the payoffs for the periphery banks are derived in the same manner as the case without an LSM. The next lemma confirms that each type of strategy profile constitutes an equilibrium.

Lemma 3.5

Netting equilibrium

• 1. All-pay: Strategy profile $S^{1}_{net}$ is an equilibrium if $\frac{\gamma _{1}}{x} + \sigma ^{h}< \frac{\gamma _{2}}{x}$, and $\frac{1}{2}(1-\sigma ^{l})\le \frac{\gamma _{1}}{x}$.

• 2. $d(\psi )$-pay: Strategy profile $S^{2}_{net}$ is an equilibrium if $\frac{\gamma _{1}}{x} + \sigma ^{h} < \frac{\gamma _{2}}{x}$, $\frac{\gamma _{1}}{x}< \frac{1}{2}(1-\sigma ^{h})$, and $1-\sigma ^{l}\le \frac{\gamma _{2}}{x}$. 3. High-pay: Strategy profile $S^{3}_{net}$ is an equilibrium if $\frac{\gamma _{1}}{x} + \sigma ^{h}< \frac{\gamma _{2}}{x}$, $\frac{1}{2}(1-\sigma ^{h}) \le \frac{\gamma _{1}}{x}< \frac{1}{2}(1-\sigma ^{l})$, $\frac{\gamma _{1}}{x}< 1-\sigma ^{h}$, and $1-\sigma ^{l}\le \frac{\gamma _{2}}{x}$.

Proof

As the proof is almost the same as that for Lemma 3.1, it is omitted. $\square$

Note that the difference in equilibrium conditions between $S^2_{net}$ and $S^3_{net}$ comes from the incentive of periphery banks with high shock. Observe that under the parameter values in which the spillover effect exists without an LSM, the addition of the LSM lets an $S^3_{net}$ type equilibrium emerge. We proceed to state the effects of the LSM for each regime.

Proposition 3.3

Effects of an LSM: liquidity non-precious regime

Under the liquidity non-precious regime,

(a) $S^{1}_{net}$ is an equilibrium under RTGS with the LSM, but $S^2 _{net}$ and $S^3_{net}$ are not, and

(b) $W(S^{1})<W(S^{1}_{net})$.

Proof

The proof for part a) is evident by comparing the two sets of conditions. For part b), $W(S^{1}_{net})-W(S^{1})=-Nr- (-(N+K)r)=Kr>0$. $\square$

Proposition 3.3 states that under the liquidity non-precious regime, the use of an LSM improves welfare.

Proposition 3.4

Effects of an LSM: Liquidity precious regime (no spillover)

Under the liquidity precious regime with $\frac{\gamma _{1}}{x}< \frac{1}{2}(1-\sigma ^{h})$,

• (a) $S^{2}_{net}$ is an equilibrium under RTGS with the LSM, and

• (b) $W(S^{2})\le W(S^{2}_{net})$ if and only if $\frac{d(\psi )-1}{K+d(\psi )-1} \le \frac{\gamma _{1}}{r}$.

Proof

The proof of part (a) is immediate and omitted. For part (b), $W(S^{2}_{net})\ge W(S^{2})\Leftrightarrow -(d(\psi )+\tilde{\sigma }N)r - (N-d(\psi ))\gamma _{1}\ge -(1+\sigma ^{l}N^{l}+\sigma ^{h}N^{h})r- (N+K-1)\gamma _{1}\Leftrightarrow (d(\psi )-1)r \le (K+d(\psi )-1)\gamma _{1}$. $\square$

Proposition 3.4 states that under the liquidity precious regime, introducing an LSM does not always improve welfare. Furthermore, welfare is more likely to decrease when the payment network is less dense, that is, the number of core-separated cycles is larger. It is simply understood by observing that the activation of offsetting among the core banks ends up in an additional unit liquidity cost for each core-separated cycle.

Proposition 3.5

Effects of an LSM: Liquidity precious regime (spillover)

Under the liquidity precious regime with $\frac{\gamma _{1}}{x}\ge \frac{1}{2}( 1-\sigma ^{h})$,

(a) $S^{3}_{net}$ is an equilibrium under RTGS with the LSM, and

(b) $W(S^{2})\le W(S^{3}_{net})$ if and only if $\frac{\min ((1-\sigma ^h)N^h, n^{h}(\psi ))}{K+ n^{h}(\psi )}\le \frac{\gamma _{1}}{r}$.

Proof

The proof of part (a) is immediate and thus, omitted. For part (b), $W(S^{3}_{net})\ge W(S^{2})\Leftrightarrow -(1+\sigma ^{l}N^{l}+min(N^{h}, n^{h}(\psi )+\sigma ^{h}N^{h}))r - (N-1-n^{h}(\psi ))\gamma _{1} \ge -(1+\sigma ^{l}N^{l}+\sigma ^{h}N^{h})r- (N+K-1)\gamma _{1} \Leftrightarrow min( (1-\sigma ^h)N^h, n^h(\psi )) r\le (K+ n^h (\psi ))\gamma _1$. $\square$

Proposition 3.5 states that for the liquidity precious regime, the partial offsetting might dismiss the positive spillover effect that is possible to arise under RTGS without an LSM. To clarify the welfare impact shown in part b), define a function as $f(n^h(\psi ) )= \frac{\min ((1-\sigma ^h)N^h, n^{h}(\psi ))}{K+ n^{h}(\psi )}$. Note that $(1-\sigma ^h)N^h$ can be interpreted as the number of banks with high liquidity shock that are not hit by the liquidity shocks.

We observe a nonlinear welfare impact of $n^h(\psi )$ as; $f'(n^h(\psi ))>0$ for $0<n^h(\psi )<(1-\sigma ^h)N^h$, and $f'(n^h(\psi ))<0$ for $(1-\sigma ^h)N^h< n^h(\psi )$. Intuitively, when $n^h(\psi )$ is not too large, the increase of $n^h(\psi )$ has a larger negative welfare impact since it brings additional liquidity cost. By contrast, when $n^h(\psi )$ is sufficiently large, the increase of $n^h(\psi )$ no longer increases liquidity cost since the liquidity costs associated with the banks with high liquidity shock have already reached the maximum, and the effect of economizing delay cost dominates.

The density has a welfare impact through its effects on $n^h(\psi )$, as indicated in Lemma 3.4. For the most dense network, there is no dismissal of the spillover effect as $n^h(\psi )=0$; thus, adding an LSM always improves welfare. For the least dense network, though the dismissal of the spillover effect is largest as $n^h(\psi )$ is the maximum possible, whether the addition of an LSM improves welfare or not depends on the parameter values. Lastly, the nonlinear welfare impact of $n^h(\psi )$ indicates that adding an LSM tends to have the largest negative welfare impact for networks with middle range density as $1<d(\psi )<K$.

3.2.3 Policy implications

The analyses bring insights for policies concerning fee control in terms of the relative cost of liquidity against the cost of delay. When the cost of liquidity is evaluated as relatively smaller, reserve should be lent at a sufficiently small fee to encourage earlier payments. Furthermore, adding an LSM tends to be socially better in that it reduces liquidity costs. By contrast, when the cost of delay, particularly short delay within a day, is evaluated as relatively smaller, reserve should be lent at a sufficiently high fee to promote efficient circulation of funds. Once funds circulate efficiently without offsetting, introducing an LSM could have a negative effect by dismissing the efficient circulation. In particular, the negative effect tends to be large if a spillover effect had worked, as shown in the analyses.

Overall, the baseline policy implication is that the use of an LSM should be examined together with the policies that promote efficient circulation of funds. In this respect, controlling the fee level would not be the sole method. Banks can be classified into groups considering the underlying payment network or bank characteristics, and each group may be allowed to make payments in each time zone.²⁷ The effects of an LSM should be contemplated in combination with these policies.

4 Concluding remarks

This paper analyzed the effect of introducing an LSM in a strategic context. The addition of an LSM could have a negative welfare effect when it serves partial offsetting. Particularly, the negative effect is pronounced under situations in which funds are circulated in a coordinated manner for the relevant settlements. The negative effect can arise when the LSM separates the underlying payment network into disconnected subnetworks. The disconnection itself has a possible negative effect since each cycle requires at least one unit of liquidity input. Furthermore, the disconnection could also dismiss a spillover effect among the connected payment networks. The fact that an LSM could have a negative effect suggests the need for an effective policy mix, in which the addition of an LSM is examined together with the adoption of other policies that promote efficient circulation of funds.

The analyses focused on the qualitative aspect of the effects of LSM. The negative aspect is elaborated with the class of core-periphery networks, particularly in view of the density of the network. Although the concept of density is defined on a limited class, the key insights hold for other types of networks. Still, many questions about the implementation of an LSM remain unanswered. A possible extension of this study could be to investigate the effect of setting a minimum proportion of the value of payments sent through the LSM. For this purpose, the effect would be clearly examined under situations where the demonstrated negative effects arising from partial offsetting are not relevant. To quantitatively assess the effects of an LSM, the analyses need to be reframed considerably in various dimensions, for example, concerning the way of incorporating the sequential nature of the interbank settlements, the realistic level of coordination concerning funds circulation, or the dynamic nature of the underlying network structure. These challenges remain for future work.

Appendix

1.1 Definition of alignment property

In Definition 2.3, alignment property is defined through the vertex-twisted concept that was originally introduced in Hayakawa (2020). Below, we show the definition. Let $G=<V,A>$ denote a directed graph, where V denotes a set of vertices and $A=\{(v,w)|v,w\in V \}$ specifies a set of arcs.²⁸ A cycle $c\subseteq A$ with $c=\{(v_1,v_2),(v_2,v_3),...,(v_{n-1},v_n)\}$ is simply denoted with $(v_1,v_2,.., v_{n+1})$ by letting $v_{n+1}=v_1$. We prepare to define the vertex-reverse number.

Definition A.1

(Vertex-reverse number) For $<V,A>$, define the vertex-sequence $s_{v}:V\rightarrow \left\{ 1,2,..,|V|\right\}$ as a one-to-one mapping. For a given $<V,A>$ together with a vertex-sequence $s_{v}$ on V, let cycle $c\in A$ consist of $(v_{1},v_{2},...,v_{n},v_{n+1})$, where $v_{n+1}=v_{1}$. Then, the vertex-reverse number is defined as $r^{vtwi}(c,s_{v})=\sum _{k=1}^{n}1_{\left\{ s_{v}(v_{k})>s_{v}(v_{k+1})\right\} }$. When there are multiple ways to index the vertices for a cycle and accordingly multiple values of the vertex-reverse number (which is possible when the cycle is punctured), we set the vertex-reverse number for the cycle as the minimum among them.

Definition A.2

(Vertex-twisted) Let $C_G$ denote the set of cycles for $G=<V,A>$. Cycles in $C\subseteq C_{G}$ are in vertex-twisted relation, or simply they are vertex-twisted if there is no vertex-sequence $s_{v}$ such that $r^{vtwi}(c,s_v)=1$ for every $c\in C$.

The focus on alignment networks in this study avoids complication concerning the calculation of the maximum borrowed funds under strategy $S^1$. The relevant result is shown in Theorem 6 in Hayakawa (2020).

1.2 Proof of Lemma 3.1

Case for $S^1$

• (a). Periphery bank i has no incentive to deviate from $s_{i}=P$ if

  $\pi _i(P,S_{-i})\ge \pi _i(D,S_{-i})$ $\Leftrightarrow$ $\frac{1}{2}x+\frac{1}{2}\sigma _{i}x\le \gamma _{1}+\sigma _{i}x$.

  The condition is rearranged as $\frac{1}{2}(1-\sigma ^{l})\le \frac{\gamma _{1}}{x}$.

• (b). Core bank i has no incentive to deviate from $s_{i}=(P,P)$ if

• (1) $\pi _i((P,P),S_{-i})\ge \pi _i((P,D),S_{-i})$ $\Leftrightarrow$ $\frac{5}{6}x+\frac{1}{3}\sigma ^{h}x \le \frac{1}{3}x+\frac{2}{3}\sigma ^{h}x+\gamma _{1}$, and

• (2) $\pi _i((P,D),S_{-i})\ge \pi _i((D,D),S_{-i})$ $\Leftrightarrow$ $\frac{1}{3}x+\frac{2}{3}\sigma ^{h}x+\gamma _{1} \le \sigma ^{h}x+2\gamma _{1}$.

Conditions (1) and (2) are rearranged as $\frac{1}{2}-\frac{1}{3}\sigma ^h \le \frac{\gamma _1}{x}$ and $\frac{1}{3}(1-\sigma ^h) \le \frac{\gamma _1}{x}$. Here, the latter condition is satisfied by the condition derived in (a), since $\frac{1}{3}(1-\sigma ^h)< \frac{1}{2}(1-\sigma ^h) < \frac{1}{2}(1-\sigma ^{l})$.

Thus, when combined with Assumption 3.1, $S^1$ is an equilibrium if $\frac{\gamma _{1}}{x}+\sigma ^{h} \le \frac{\gamma _{2}}{x}$, $\frac{1}{2}(1-\sigma ^{l})\le \frac{\gamma _{1}}{x}$, and $\frac{1}{2}-\frac{1}{3}\sigma ^{h}\le \frac{\gamma _{1}}{x}$.

Case for $S^2$ Within $S^2$, we focus on a strategy profile where $s_i = P$ for periphery bank $i\in I_{per}$ with $\sigma _l$. Moreover, the bank that receives a payment from bank i is a periphery bank $j\in I_{per}$ with $\sigma _l$.

(a). For periphery bank i with $s_{i}=D$, there is no incentive to deviate if

(1) $\pi _i(D,S_{-i})> \pi _i(P,S_{-i})$ with $s^P_i=P$ $\Leftrightarrow$ $\sigma _i x + \gamma _1< \frac{1}{2}x+ \frac{1}{2}\sigma _i x$,

(2) $\pi _i(D,S_{-i})> \pi _i(P,S_{-i})$ with $s^P_i=D$ $\Leftrightarrow$ $\sigma _i x + \gamma _1< x$.

Condition (1) and (2) are rearranged as $\frac{\gamma _{1}}{x} < \frac{1}{2}(1-\sigma ^{l})$ and $\frac{\gamma _{1}}{x}< 1-\sigma ^{h}$.

(b). For periphery bank i with $s_{i}=P$, there is no incentive to deviate if

(1) $\pi _i(P,S_{-i})\ge \pi _i(D,S_{-i})$ $\Leftrightarrow$ $x\le \sigma _i x + \gamma _2$.

The condition is rearranged as $1-\sigma ^l \le \frac{\gamma _2}{x}$ for bank i with $\sigma _i = \sigma ^l$.

(c). Core bank i has no incentive to deviate from $s_{i}=(D,D)$ when $s_{i}^{p}=(D,D)$ if

(1) $\pi _i((D,D),S_{-i})> \pi _i((P,P),S_{-i})$ $\Leftrightarrow$ $\sigma ^h x + 2\gamma _1 < 2x$,

(2) $\pi _i((D,D),S_{-i})> \pi _i((P,D),S_{-i})$ $\Leftrightarrow$ $\sigma ^h x + 2\gamma _1 < x + \gamma _{1}$.

Condition (1) and (2) are rearranged as $\frac{\gamma _1}{x}< 1- \frac{1}{2}\sigma ^h$ and $\frac{\gamma _1}{x}< 1-\sigma ^h$. The latter condition is sufficient for the former condition.

Thus, when combined with Assumption 3.1, $S^2$ is an equilibrium if $\frac{\gamma _{1}}{x} < \frac{1}{2}(1-\sigma ^{l})$, $\frac{\gamma _{1}}{x}< 1-\sigma ^{h}$, $\frac{\gamma _{1}}{x}+\sigma ^{h} < \frac{\gamma _{2}}{x}$, and $1-\sigma ^l \le \frac{\gamma _2}{x}$.

1.3 Proof of Lemma 3.2

Under strategy profile $S^{1}$, every bank makes payments in period 1. For the networks within class $\Psi$, observe that there are at most $K+1$ non-punctured cycles. The alignment property indicates that for each non-punctured cycle, there exists at most one payment that is settled in period 1 without borrowing.²⁹ Thus, there are at most $K+1$ payments that are made in period 1 without borrowing funds. Since we focus on the worst-case scenario, the liquidity shocks must first hit the banks without holding funds. Since the former part of Assumption 3.5 ensures $K+1 \le N^h \sigma ^h$, every payment requires intraday borrowing in the end. Since there are $|I|=N$ banks with K core banks, the total borrowed amount becomes $N+K$. Thus, we have $W(S^1)=-(N+K)r$.

Under an $S^2$ strategy profiles, only one periphery bank makes a payment in period 1. All payments are settled successfully before the liquidity shock at the end of period 2. In the worst-case scenario, the liquidity shock increases the borrowing amounts by $N^h\sigma ^h + N^l \sigma ^l$. The latter part of Assumption 3.5 states that $N {\tilde{\sigma }} <N-K$, which ensures that every bank that is hit by the liquidity shock does not hold funds. Thus, the total borrowed amount is $1+ N{\tilde{\sigma }}$. Since the total delay costs are $(N+K-1) \gamma _1$, we have $W(S^2)= -( (1+ {\tilde{\sigma }}N)r + (N+K-1)\gamma _{1})$.

1.4 Proof of proposition 3.2

Referring to Lemma A.1, it is sufficient to compare $W(S^{1})$ and $W(S^{2})$. $W(S^{1}) \le W(S^{2})$ if $(N+K)r- (1+N{\tilde{\sigma }})r - (N+K-1)\gamma _{1}\ge 0$, or, $(N+K-1-N{\tilde{\sigma }})r\ge (N+K-1)\gamma _{1}$. We have, ${\tilde{\sigma }}\le \frac{N+K-1}{N}(1-\frac{\gamma _{1}}{r})$. Combining this with Assumption 3.5, we complete our proof.

Lemma A.1

Under Assumption 3.2, 3.3, and 3.4, either $S^{1}$ or $S^{2}$ attains first best.

Proof

Suppose some of the payments $0\le n < N+K$ are made in period 1, and all the other payments are not made in period 2 but held instead. Now, we change the strategy profile so that one payment is made instead of being held in period 2. This improves welfare by at least $\gamma _2 - \gamma _1 - \sigma ^hr$, which is positive based on Assumption 3.4. Since each additional replacement done in the same manner improves the welfare similarly, it is sufficient to examine strategy profiles where the outstanding payments are always made in period 2.

For the remaining alternative strategy profiles, the number of payments made in period 1 essentially differ. Consider a strategy profile where every bank does not make any payment in period 1. The total cost is $N{\tilde{\sigma }}r + (N+K)\gamma _2$. Against this strategy profiles, Assumption 3.3 ensures that $S^2$ attains larger welfare.

Now, replace $S^2$ such that $k>1$ payments are made in period 1. When moving from the case with k to the case with $k+1$, it decreases welfare by $r- \gamma _1$ as long as it generates additional liquidity cost in the worst-case scenario. Assumption 3.2 ensures that $r- \gamma _1 >0$. Note that an additional liquidity cost emerges as long as the liquidity shock is sufficiently small. Suppose this additional liquidity cost emerges for the case with $k'$ but not in the case with $k'+1$. Then, moving from the case with $k'$ to the case with $k'+1$ let social welfare increase by $\gamma _1$. Observe that moving from the case with $k'+1$ to the case with $k'+2$, to the case with $k'+3$,..., the welfare always increases by the same amount. Since this procedure reaches $S^1$, either $S^1$ or $S^2$ attains the first best. $\square$

1.5 Best responses and spillover effect

For periphery banks, we focus on the following three types of reactions as candidates for the best responses.

For periphery bank $i\in I_{per}$;

(B1): $s_i = P$ for any $S_{-i}$;

(B2): $s_i=P$ only if $s_{i-1}=P$ or no other bank $i'\ne i$ takes $s_{i'}\in \{P, (P,P), (P,D),(D,P)\}$; and

(B3): $s_i=P$ only if no other bank $i'\ne i$ takes $s_{i'}\in \{P, (P,P), (P,D),(D,P)\}$.

For core banks, the relevant reactions are classified into five types. Below, the reactions of core bank $i\in I_{core}$ are stated in relation to the payer’s strategies $s_i^p\in \{\{P,P\}, \{D,D\}\}$:

$(B_c 0)$: $s_{i}=(P,P)$ for $s_i^p\in \{ \{P,P\},\{D,D\} \}$.

$(B_c 1)$: $s_{i}=(P,P)$ if $s_{i}^p = \{P,P\}$, while $s_i = (P,D)$ if $s_i^p= \{D,D\}$.

$(B_c 2a)$: $s_{i}=(P,P)$ if $s_{i}^p=\{P,P\}$, while $s_i = (D,D)$ if $s_{i}^p =\{D,D\}$.

$(B_c 2b)$: $s_{i} =(P,D)$ for $s_{i}^p\in \{\{P,P\}, \{D,D\}\}$.

$(B_c 3)$: $s_{i} =(P,D)$ if $s_{i}^p=\{P,P\}$, while $s_i = (D,D)$ if $s_{i}^p =\{D,D\}$.

$(B_c 4)$: $s_{i} =(D,D)$ for $s_{i}^p\in \{\{P,P\},\{D,D\}\}$.

Observation A.1

(Best response)

(i). With sufficiently large $\gamma _2$, the best response for periphery bank i with $\sigma _{i}\in \left\{ \sigma ^{l},\sigma ^{h}\right\}$ is:

(B1) if $1-\sigma _{i}\le \frac{\gamma _{1}}{x}$.

(B2) if $\frac{1}{2}(1-\sigma _{i})\le \frac{\gamma _{1}}{x}< 1-\sigma _{i}$.

(B3) if $\frac{\gamma _{1}}{x} < \frac{1}{2}(1-\sigma _{i})$.

(ii). The best responses against strategy profiles $S^{1}$ and $S^{2}$ are examined. For $S^{2}$, let $s_{j}=P$ for $j\in I_{per}$, which is not a payer to any core bank. Then, with sufficiently large $\gamma _2$, the best response for core bank i with $\sigma _{i} =\sigma ^{h}$ is:

$(B_c 0)$ if $1 \le \frac{\gamma _1}{x}$.

$(B_c 1)$ if $1-\sigma ^{h} \le \frac{\gamma _{1}}{x}$ and $\frac{1}{2}(1-\frac{1}{3}\sigma _h x) \le \frac{\gamma _{1}}{x}$.

$(B_c 2a)$ if $\frac{\gamma _{1}}{x} < 1-\sigma ^{h}$ and $\frac{1}{2}(1-\frac{1}{3}\sigma _h x) \le \frac{\gamma _{1}}{x}$.

$(B_c 2b)$ if $1-\sigma ^{h} \le \frac{\gamma _{1}}{x}$ and $\frac{\gamma _{1}}{x} \le \frac{1}{2}(1-\frac{1}{3}\sigma _h x)$.

$(B_c 3)$ if $\frac{\gamma _{1}}{x}\le \frac{1}{2}(1-\frac{2}{3}\sigma ^{h})$, $\frac{\gamma _{1}}{x} < 1-\sigma ^h$, and $\frac{1}{3}(1-\sigma ^h ) \le \frac{\gamma _{1}}{x}$.

$(B_c 4)$ if $\frac{\gamma _{1}}{x} < \frac{1}{3}(1-\sigma ^h )$.

Figure 4 shows the best responses for periphery banks in a two-dimensional presentation regarding parameters $\frac{\gamma _1}{x}$ and $\sigma ^l, \sigma ^h$.

Fig. 4

Relevant best responses for periphery banks. Note Relevant best responses of periphery banks are presented with sufficiently large $\gamma _2$

Figure 5 similarly shows the relevant best responses for core banks in the same two dimensional representation. For core banks with two payments, the insurance for liquidity shock matters only for making one payment. Thus, whether making both payments $(B_c0)$ constitutes a best response or not does not depend on $\sigma ^l, \sigma ^h$. It is the best response when the relative cost of delay is sufficiently large, that is, $\frac{\gamma _1}{x}>1$. In the case of $S^2$ in which $s_{i}^p=(D,D)$ for core bank $i\in I_{core}$, the logic of the insurance is evident: a payment is made when a larger shock is experienced ($(B_c1)$ and $(B_c2b)$), and not otherwise ($(B_c2a)$, $(B_c3)$, and $(B_c4)$). In the case of $S^1$, where $s_i^p = (P,P)$, the insurance is less attractive for making additional payments since there is a smaller increase in the probability of borrowing a reserve. Thus, the marginal increase of the liquidity shock leads to lower marginal increase in the attractiveness of the insurance, as confirmed in the figure wherein the dividing lines $\frac{\gamma _{1}}{x} = \frac{1}{3}(1-\sigma ^h )$ and $\frac{\gamma _{1}}{x} = \frac{1}{2}(1-\frac{1}{3}\sigma _h x)$ gets steeper. We obtain the latter line by a parallel shift of the former line to the right since making the second additional payment is possibly accompanied by an ex-post “excessive" insurance when the bank borrows two units of funds. Note that the occurrence of $(B_c2b)$ or $(B_c2a)$ depends on the level of $\sigma ^h$.

Fig. 5

Relevant best responses for core banks. Note Relevant best responses of core banks are presented with sufficiently large $\gamma _2$

Fig. 6

Equilibrium, best response, and spillover effect. Note The figure shows equilibria, best responses(BRs) of periphery banks (peri) and core banks (core), and the existence of “spillover effect" (Spillover) for $0<\frac{\gamma _{1}}{x}\le 1$, with $(a)=\frac{1}{3}(1-\sigma ^{h})$, $(b)=\frac{1}{2}(1-\sigma ^{h})$, $(c)= \frac{1}{2}(1-\frac{2}{3}\sigma ^{h})$, $(d)=\frac{1}{2}(1-\sigma ^{l})$, $(e)=1-\sigma ^{h}$, and $(f)=1-\sigma ^{l}$. Specifically, the consistent parameter values are: $\sigma ^{h}=0.45$, $\sigma ^{l}=0.2$, and $\frac{\gamma _{2}}{x}=1.5$. Under the values, $(a)=0.18\dot{3}$, $(b)=0.275$, $(c)=0.35$, $(d)=0.4$, $(e)=0.55$, and $(f)=0.8$

Figure 6 summarizes the relationship between equilibria and relevant best responses in a one-dimensional figure with varying parameter $\frac{\gamma _1}{x}$ given specific values for $\sigma ^h (<\frac{3}{4})$, $\sigma ^l$, and $\gamma _2$. Observe that the dividing line between $S^1$ or $S^2$ is determined by the line between (B2) and (B3) for the periphery banks with low liquidity shock. The dark area shows an equilibrium for $S^2$, wherein a spillover effect arises.