Micro-amplitude vibration suppression of a bistable nonlinear energy sink constructed by a buckling beam

Abstract

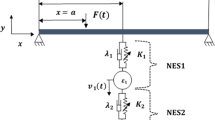
Effective reduction of micro-amplitude vibration has always been a serious challenge. The nonlinear energy sink (NES) has been proven to be able to reduce vibration at a wide frequency. However, the energy threshold of the NES prevents it from suppressing micro-vibrations. In this paper, a bistable nonlinear energy sink (BNES) based on a buckling beam oscillator is constructed. The threshold of the NES is lowered by the nonlinear dynamic behavior of jumping between wells of the bistable oscillator. The motion equations of the discrete–continuous system are derived by using Hamilton's principle. The approximate analytical solution is obtained and verified numerically. The results show that even when the primary system has a micro-amplitude resonance, the nonlinear cross-well vibration of the BNES can still reduce the vibration. The robustness of the BNES is stronger than that of the tuned mass damper (TMD). The optimal parameters of the BNES are obtained with particle swarm optimization (PSO) algorithm. The result of parameter optimization shows that the energy threshold of the nonlinear energy sink can be effectively lowered. In short, the method based on nonlinear dynamics in this paper provides an effective reduction strategy for micro-vibration in engineering.

Graphical abstract

Appendix A. Derivation of differential equations of motion

According to the generalized Hamilton’s principle, the governing equation of the coupling system can be written as

$$ \delta \int_{{t_{1} }}^{{t_{2} }} {\left( {T - V} \right)} {\text{d}}t + \delta \int_{{t_{1} }}^{{t_{2} }} {W_{{\text{F}}} } {\text{d}}t = 0 $$

(A.1)

where $\delta$ is the variation taken within the specified time interval.

The kinetic energy and the potential energy of the LO can be expressed as

$$ T_{1} = \frac{1}{2}m_{1} \dot{x}^{2} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} V_{1} = \frac{1}{2}k_{1} (x - x_{0} )^{2} $$

(A.2)

where m₁ and k₁ are mass and stiffness of LO, respectively.

The kinetic energy of m₂ can be expressed as

$$ T_{2} = \frac{1}{2}m_{2} \left[ {\frac{{\partial \overline{w}(L/2)}}{\partial t}} \right]^{2} $$

(A.3)

where m₂ is the concentrated mass fixed at the midpoint of the beam.

The kinetic energy of the beam can be expressed as

$$ T_{{\text{b}}} = \frac{1}{2}\int_{0}^{L} {\rho S\left[ {\left( {\frac{\partial u}{{\partial t}}} \right)^{2} + \left( {\frac{{\partial \overline{w}}}{\partial t}} \right)^{2} } \right]} {\text{d}}y $$

(A.4)

where L is the initial length of the beam, $\rho$ is mass per unit volume, and S is the cross-sectional area of the beam.

The bending potential energy of the beam is expressed as

$$ V_{{{\text{b1}}}} = \frac{1}{2}\int_{0}^{L} {EI\left[ {\frac{{\partial^{2} (\overline{w} - x)}}{{\partial y^{2} }}} \right]}^{2} {\text{d}}y $$

(A.5)

where E and I are the modulus of elasticity and the moment of inertia of the beam, respectively.

The axial tensile potential energy is denoted as

$$ V_{{{\text{b2}}}} = \frac{1}{2}\int_{0}^{L} {P\left[ {\frac{\partial u}{{\partial y}} + \frac{{\partial (\overline{w} - x)}}{\partial y}} \right]}^{2} {\text{d}}y $$

(A.6)

The potential energy generated by the stretching of the neutral surface can be expressed as

$$ V_{{{\text{b3}}}} = \frac{1}{2}\int_{0}^{L} {ES\left[ {\frac{\partial u}{{\partial y}} + \frac{1}{2}\left( {\frac{{\partial (\overline{w} - x)}}{\partial y}} \right)^{2} } \right]}^{2} {\text{d}}y $$

(A.7)

The total kinetic energy and total potential energy of the system, respectively, are

$$ T = T_{1} + T_{2} + T_{{\text{b}}} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} V = V_{1} + V_{{{\text{b1}}}} + V_{{{\text{b2}}}} + V_{{{\text{b3}}}} $$

(A.8)

The work done by the external force can be written as

$$ W_{{\text{C}}} = c_{1} \left( {\dot{x} - \dot{x}_{0} } \right)\left( {x - x_{0} } \right) + \mu \int_{0}^{L} {\left( {\overline{w} - x} \right)} \left( {\dot{\overline{w}} - \dot{x}} \right){\text{ d}}y $$

(A.9)

where c₁ is the damping coefficient of the LO; $\mu$ is the viscous damping coefficient.

Substituting Eqs. (A.2)–(A.9) into Eq. (A.1), the governing equations of the beam can be obtained by using the variation principle as

$$ m_{1} \ddot{x} + c_{1} \left( {\dot{x} - \dot{x}_{0} } \right) + k_{1} \left( {x - x_{0} } \right){ + }\left[ {m_{2} \delta \left( {y - L/2} \right){ + }\rho S} \right]\frac{{\partial^{2} \left( {w{ + }x} \right)}}{{\partial t^{2} }} = 0 $$

(A.10)

$$ \begin{gathered} \left[ {m_{2} \delta \left( {y - \frac{L}{2}} \right){ + }\rho S} \right]\frac{{\partial^{2} \left( {w{ + }x} \right)}}{{\partial t^{2} }} + EI\frac{{\partial^{4} w}}{{\partial y^{4} }}{ + }P\frac{{\partial^{2} w}}{{\partial y^{2} }} \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} - ES\left[ {\frac{3}{2}\left( {\frac{\partial w}{{\partial y}}} \right)^{2} \frac{{\partial^{2} w}}{{\partial y^{2} }} + \frac{{\partial^{2} u}}{{\partial y^{2} }}\frac{\partial w}{{\partial y}} + \frac{\partial u}{{\partial y}}\frac{{\partial^{2} w}}{{\partial y^{2} }}} \right] + \mu \frac{\partial w}{{\partial t}} = 0 \hfill \\ \end{gathered} $$

(A.11)

$$ \rho S\frac{{\partial^{2} u}}{{\partial t^{2} }} - ES\left( {\frac{{\partial^{2} u}}{{\partial y^{2} }} + \frac{\partial w}{{\partial y}}\frac{{\partial^{2} w}}{{\partial y^{2} }}} \right) = 0 $$

(A.12)

where w is also the relative displacement, which is the transverse vibration displacement of the beam and is defined as $\overline{w} - x$. $\delta$ is the Dirac function.

Because the longitudinal displacement is much smaller than the transverse displacement and ρS <  < ES, Eq. (A.12) can be written as

$$ \frac{{\partial^{2} u}}{{\partial y^{2} }} + \frac{\partial w}{{\partial y}}\frac{{\partial^{2} w}}{{\partial y^{2} }}{ = }0 $$

(A.13)

The solution to Eq. (A.13) is obtained

$$ u = - \frac{1}{2}\int_{0}^{y} {\left( {\frac{\partial w}{{\partial y}}} \right)}^{2} {\text{d}}y + yC_{1} \left( t \right) + C_{2} \left( t \right) $$

(A.14)

where C₁(t) and C₂(t) are the functions of time t.

The longitudinal boundary condition of the beam is fixed at both ends.

$$ u\left( {0,t} \right) = 0,u\left( {L,t} \right) = 0 $$

(A.15)

Substituting Eq. (A.15) into Eq. (A.14) yields

$$ u = \frac{y}{2L}\int_{0}^{L} {\left( {\frac{\partial w}{{\partial y}}} \right)}^{2} {\text{d}}y - \frac{1}{2}\int_{0}^{y} {\left( {\frac{\partial w}{{\partial y}}} \right)}^{2} {\text{d}}y $$

(A.16)

Substituting Eq. (A.16) into Eq. (A.11), the coupling nonlinear equation of the discrete–continuous system is as follows.

$$ m_{1} \ddot{x} + c_{1} \left( {\dot{x} - \dot{x}_{0} } \right) + k_{1} \left( {x - x_{0} } \right){ + }\left[ {m_{2} \delta \left( {y - L/2} \right)} \right]\frac{{\partial^{2} \left( {w{ + }x} \right)}}{{\partial t^{2} }} = 0 $$

(A.17)

$$ \left[ {m_{2} \delta \left( {y - \frac{L}{2}} \right){ + }\rho S} \right]\frac{{\partial^{2} \left( {w{ + }x} \right)}}{{\partial t^{2} }} + EI\frac{{\partial^{4} w}}{{\partial y^{4} }}{ + }\left[ {P - \frac{ES}{{2L}}\int_{0}^{L} {\left( {\frac{\partial w}{{\partial y}}} \right)^{2} {\text{d}}y} } \right]\frac{{\partial^{2} w}}{{\partial y^{2} }} + \mu \frac{\partial w}{{\partial t}} = 0 $$

(A.18)

Ignore the time term of Eq. (A.18), and the static governing equations of the beam are obtained as follows.

$$ EI\frac{{\partial^{4} w}}{{\partial y^{4} }} + \left[ {P - \frac{ES}{{2L}}\int_{0}^{L} {\left( {\frac{\partial w}{{\partial y}}} \right)^{2} {\text{d}}y} } \right]\frac{{\partial^{2} w}}{{\partial y^{2} }} = 0 $$

(A.19)

The transverse boundary conditions of the beam are fixed at both ends.

$$ w\left( {0,t} \right) = 0, \, \frac{{\partial w\left( {0,t} \right)}}{\partial y} = 0, \, w\left( {L,t} \right) = 0, \, \frac{{\partial w\left( {L,t} \right)}}{\partial y} = 0 $$

(A.20)

An equilibrium configuration $w_{{\text{p}}}$ of post-buckling is obtained as

$$ w_{{\text{p}}} { = }d_{0} \phi \left( y \right) $$

(A.21)

where $\phi \left( y \right){ = }\frac{1}{2}\left[ {1 - \cos \left( {\frac{2\pi y}{L}} \right)} \right],{\kern 1pt} {\kern 1pt} {\kern 1pt} d_{0} { = }\frac{2}{E\pi S}\sqrt {ES\left( {PL^{2} - 4EI\pi^{2} } \right)}$, $\phi \left( y \right)$ is the first buckling mode shape, and d₀ is the midpoint deflection.

In order to study the dynamic problem around the buckling configuration, the total deflection can be defined as the sum of the post-buckling static deflection and the time-dependent displacement r(x,t) around the initial equilibrium configuration [43],

$$ w\left( {y,t} \right) = d_{0} \phi \left( y \right) + r\left( {y,t} \right) $$

(A.22)

According to Galerkin's truncation method, r(y,t) can be expanded into a superposition of N orthonormal base functions $\phi_{g} \left( y \right)$ as follows.

$$ r\left( {y,t} \right){ = }\sum\nolimits_{{{\text{g}} = 1}}^{N} {z_{{\text{g}}} \left( t \right)\phi_{{\text{g}}} \left( y \right)} $$

(A.23)

where $z_{{\text{g}}} \left( t \right)$ are the generalized coordinates. If only the first mode is preserved, and $\phi_{1} \left( y \right){ = }\phi \left( y \right)$, then the total deflection becomes

$$ w\left( {y,t} \right) = d_{0} \phi \left( y \right){ + }z_{1} \left( t \right)\phi_{1} \left( y \right){ = }q\left( t \right)\phi \left( y \right) $$

(A.24)

where $q\left( t \right) = d_{0} + z_{1} \left( t \right)$ represents the time-dependent displacement of the fixed–fixed beam relative to the axis passing through the supports. Substitute Eq. (A.24) into Eq. (A.18), and then multiply by $\phi \left( y \right)$ and integrate over the length of the beam. It should be stated that the formula derivation of the mechanical model in this paper mainly refers to reference [43].