Design criteria of bistable nonlinear energy sink in steady-state dynamics of beams and plates

Abstract

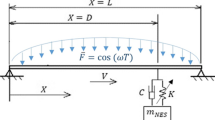
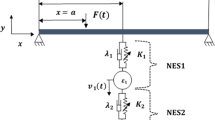
A bistable nonlinear energy sink (BNES) conceived for the passive vibration control of beam and plate structures under harmonic excitation is investigated. By applying an Incremental Harmonic Balance (IHB) method together with an adjusted arc-length continuation technique, the frequency and amplitude responses are obtained, and their respective trends are discussed in detail from three aspects. The simplest single-mode dynamics is first considered with a special focus on the coupled effect of the cubic nonlinear stiffness and the negative linear stiffness, where an analytical treatment using complex-averaging method is also applied to obtain the slow invariant manifold for understanding the underlying dynamics. Then the multi-mode dynamics of the beam are discussed in variation of each parameter. As a result, a simple step-by-step design rule for the BNES is summarized. Finally, the obtained results and design criteria of the BNES in the beam case are extended to a 2D plate, realizing a broadband control for multi-mode plate vibration. It is found that compared to a traditional cubic one, a BNES can have a better performance both on the frequency and amplitude point of view.

Appendices

Appendix A: Matrices in Eq. (11)

The matrices in Eq. (11) are calculated as

$$\begin{aligned} \begin{aligned}&{\mathbf {N}} = {\left[ {\begin{array}{*{20}{c}} {{\mathbf {N}}_1^T,\;{\mathbf {N}}_2^T,\; \cdots ,\;{\mathbf {N}}_{{N_m}}^T} \end{array}} \right] ^T}, \\&{{\mathbf {N}}_k} = \left[ {{a_0},{a_{k1}},{a_{k2}} \cdots ,{a_{kn}},{b_{k1}},{b_{k2}}, \cdots {b_{kn}}} \right] , \\&{{\mathbf {K}}_{mc}} = \omega _0^2{\mathbf {M}} + {\omega _0}{\mathbf {C}} + {\mathbf {K}} + 3{{\mathbf {K}}_{n0}}, \\&{\mathbf {R}} = {\mathbf {F}} - \left( {\omega _0^2{\mathbf {M}} + {\omega _0}{{\mathbf {C}}_{n0}} + {\mathbf {K}} + {{\mathbf {K}}_{n0}}} \right) {\mathbf {N}}, \\&{{\mathbf {R}}_{mc}} = - \left( {2{\omega _0}{\mathbf {M}} + {\mathbf {C}}} \right) {\mathbf {N}}, \\&{\mathbf {M}} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}{{\bar{\mathbf {M}}\ddot{{\varLambda }} }}} d\tau , {\mathbf {K}} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}{{\bar{\mathbf {K}}{\varLambda } }}} d\tau , \\&{{\mathbf {K}}_{n0}} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}{{{{\bar{\mathbf {K}}}}}_{n0}}{\varvec{\Lambda }}} d\tau , {\mathbf {C}} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}{{\bar{\mathbf {C}}{\dot{{\varLambda }}} }}} d\tau , \\&{{\mathbf {R}}_f} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}\cos \tau } d\tau , {\mathbf {F}} = \int _0^{2\pi } {{{\varvec{\Lambda }}^T}{{{{\bar{\mathbf {F}}}}}_0}\cos \tau } d\tau . \\&{\varvec{\Lambda }} = diag\left[ {{\mathbf {D}},{\mathbf {D}}, \cdots {\mathbf {D}}} \right] , \\&{\mathbf {D}} = \left( {1,\cos \tau ,\cos 2\tau , \cdots ,\sin \tau ,sin2\tau , \cdots } \right) \end{aligned} \end{aligned}$$

(A.1)

Appendix B: Stability analysis using Floquet theory

The stabilities of the periodic solutions can be analyzed by means of the Floquet theory. Let ${\mathbf{X }} = {{\mathbf{X }}_0} + {\varDelta } {\mathbf{X }}$ and consider the perturbation motion of Eq. (7) near the fixed point ${{\mathbf{X }}_0}$ yields

$$\begin{aligned} {\omega ^2}{\bar{\mathbf{M }}}{\varDelta } {{\ddot{\varvec{X}}}} + \omega {{\bar{\mathbf{C }}}}{\varDelta } {\dot{\mathbf{X }}} + \left( {{\bar{\mathbf{K }}} + 3{{{\bar{\mathbf{K }}}}_{n0}}} \right) {\varDelta } {\mathbf{X }} = 0, \end{aligned}$$

(B.2)

or equivalently,

$$\begin{aligned} {\dot{\mathbf{Y }}} = {\mathbf{Q }}\left( \tau \right) {\mathbf{Y }}, \end{aligned}$$

(B.3)

with

$$\begin{aligned}&{\mathbf{Q }}\left( \tau \right) = \left[ {\begin{array}{*{20}{c}} 0&{}{\mathbf{I }}_n\\ { - \frac{{{\bar{\mathbf{M }}}}^{ - 1}}{{{\omega ^2}}}\left( {{\bar{\mathbf{K }}} + 3{{{\bar{\mathbf{K }}}}_{n0}}} \right) }&{}{ - \frac{{{\bar{\mathbf{M }}}}^{ - 1}}{\omega }{{{\bar{\mathbf{C }}}}}} \end{array}} \right] ,\\&{\mathbf{Y }} = \left[ {\begin{array}{*{20}{c}} {{\varDelta } {\mathbf{X }}}\\ {{\varDelta } {\dot{\mathbf{X }}}} \end{array}} \right] . \end{aligned}$$

Since the solution $\mathbf{X }_0$ is periodic with period $T = 2\pi$, the associated matrix ${\mathbf{Q }}\left( \tau \right)$ must also have the same property, which in turn implies ${\mathbf{Q }}\left( {\tau + T} \right) = {\mathbf{Q }}\left( \tau \right)$ and ${\mathbf{Y }}\left( {\tau + T} \right)$ solves Eq. (B.3) as well. The relationship between ${\mathbf{Y }}\left( {\tau + T} \right)$ and ${\mathbf{Y }}\left( {\tau } \right)$ can be expressed as

$$\begin{aligned} {\mathbf{Y }}\left( {\tau + T} \right) = {\mathbf{P }}{\mathbf{Y }}\left( \tau \right) , \end{aligned}$$

(B.4)

where ${\mathbf{P }}$ is usually termed as the transition matrix in the literature. Many researches have been devoted to evaluate the transition matrix ${\mathbf{P }}$. In this paper a precise Hsu’s method is applied following [49, 57], in which the period T is divided equally into n intervals with time step h, such that for the kth interval $[{\tau _k},{\tau _{k + 1}}]$, ${\mathbf{Q }}\left( \tau \right) ={{\mathbf{Q }}_k}$ is assumed to be constant, then the local transition matrix ${{\mathbf{P }}_k}$ from ${\tau _k}$ to ${\tau _{k+1}}$ writes,

$$\begin{aligned} {{\mathbf{P }}_k} = {e^{h{{\mathbf{Q }}_k}}} = {\mathbf{I }} + \sum \limits _{j = 1}^n {\frac{{{{\left( {h{{\mathbf{Q }}_k}} \right) }^j}}}{{j!}}}, \end{aligned}$$

(B.5)

thus the transition matrix P can be approximated by multiplying all the local matrices ${{\mathbf{P }}_k}$ together as

$$\begin{aligned} {\mathbf{P }} = \mathop {\varPi }\limits _{i = 1}^n {{\mathbf{P }}_k}. \end{aligned}$$

(B.6)

In the framework of the Floquet theory, the stability of the periodic response is then determined by checking the eigenvalues of P. If the spectral radius of P is less than 1, then the periodic solution is asymptotic stable, otherwise, it is unstable.