Skip to main content
SpringerLink
Log in

An improved transfer-matrix method on steady-state response analysis of the complex rotor-bearing system

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

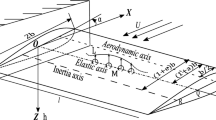
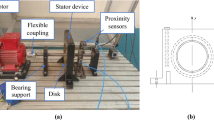
Abstract

This paper proposes an improved transfer-matrix method (TMM) for investigating the steady-state response of complex rotor-bearing systems. The internal damping of the shafts is considered to enhance the accuracy of the method. A transfer matrix of ball bearings with clearance and Hertzian contact is established. In addition, the incremental harmonic balance method is combined with the TMM to obtain the steady-state response of the rotor systems and simplify the investigation processes. The simulation results verify the superiority of this method in programming, reducing the order of system matrix, and determining stability. Thus, this method is efficient in investigating the nonlinear dynamic characteristics of large-scale rotor-bearing systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Daniel Maraini, C.: Nataraj: nonlinear analysis of a rotor-bearing system using describing functions. J. Sound Vib. 420(28), 227–241 (2018)

    Article  Google Scholar 

  2. Kappaganthu, K., Nataraj, C.: Nonlinear modeling and analysis of a rolling element bearing with a clearance. Commun. Nonlinear Sci. Numer. Sim. 16(10), 4134–4145 (2011)

    Article  MATH  Google Scholar 

  3. Kurvinen, E., Sopanen, J., Mikkola, A.: Ball bearing model performance on various sized rotors with and without centrifugal and gyroscopic forces. Mech. Mach. Theory 90(8), 240–260 (2015)

    Article  Google Scholar 

  4. Hou, L., Chen, Y., Yiqiang, F., Chen, H., Zhenyong, L., Liu, Z.: Application of the HB–AFT method to the primary resonance analysis of a dual-rotor system. Nonlinear Dyn. 88(4), 1–21 (2017)

    Article  Google Scholar 

  5. Bonello, P., Hai, P.M.: A receptance harmonic balance technique for the computation of the vibration of a whole aero-engine model with nonlinear bearings. J. Sound Vib. 324(1–2), 221–242 (2009)

    Article  Google Scholar 

  6. Zhenyong, L., Hou, L., Chen, Y., Sun, C.: Nonlinear response analysis for a dual-rotor system with a breathing transverse crack in the hollow shaft. Nonlinear Dyn. 83(1–2), 169–185 (2016)

    MathSciNet  MATH  Google Scholar 

  7. Sinou, J.-J.: Effects of a crack on the stability of a non-linear rotor system. Int. J. Non-linear Mech. 42(7), 959–972 (2007)

    Article  Google Scholar 

  8. AL-Shudeifat, M.A., Butcher, E.A., Stern, C.R.: General harmonic balance solution of a cracked rotor-bearing-disk system for harmonic and sub-harmonic analysis: analytical and experimental approach. Int. J. Eng. Sci. 48(10), 921–935 (2010)

    Article  Google Scholar 

  9. von Groll, G., Ewins, D.J.: The harmonic balance method with arc-length continuation in rotor/stator contact problems. J. Sound Vib. 241(2), 223–233 (2001)

    Article  Google Scholar 

  10. Bai, C., Qingyu, X., Wang, J.: Effects of flexible support stiffness on the nonlinear dynamic characteristics and stability of a turbopump rotor system. Nonlinear Dyn. 64(3), 237–252 (2011)

    Article  Google Scholar 

  11. Villa, C., Sinou, J.-J., Thouverez, F.: Stability and vibration analysis of a complex flexible rotor bearing system. Commun. Nonlinear Sci. Numer. Simul. 13(4), 804–821 (2008)

    Article  Google Scholar 

  12. Sinou, J.-J.: Non-linear dynamics and contacts of an unbalanced flexible rotor supported on ball bearings. Mech. Mach. Theory 44(9), 1713–1732 (2009)

    Article  MATH  Google Scholar 

  13. Sinou, J.-J., Didier, J., Faverjon, B.: Stochastic non-linear response of a flexible rotor with local non-linearities. Int. J. Non-linear Mech. 74(9), 92–99 (2015)

    Article  Google Scholar 

  14. Zu-Qing, Q.: Model reduction for dynamical systems with local nonlinearities. AIAA J. 40(2), 327–333 (2002)

    Article  Google Scholar 

  15. Nataraj, C., Nelson, H.D.: Periodic solutions in rotor dynamic systems with nonlinear supports: a general approach. J. Vib. Acoust. 111(2), 187–193 (1989)

    Article  Google Scholar 

  16. Rouch, K.E., McMains, T.H., Stephenson, R.W., Emerick, M.F.: Modeling of complex rotor systems by combining rotor and substructure models. Finite Element Anal. Des. 10(1), 89–100 (1991)

    Article  Google Scholar 

  17. Papadimitriou, C., Papadioti, D.-C.: Component mode synthesis techniques for finite element model updating. Comput. Struct. 126(15), 15–28 (2013)

    Article  Google Scholar 

  18. Bathe, K.-J., Dong, J.: Component mode synthesis with subspace iterations for controlled accuracy of frequency and mode shape solutions. Comput. Struct. 139(15), 28–32 (2014)

    Article  Google Scholar 

  19. Theodosiou, C., Sikelis, K., Natsiavas, S.: Periodic steady state response of large scale mechanical models with local nonlinearities. Int. J. Solids Struct. 46(20), 3565–3576 (2009)

    Article  MATH  Google Scholar 

  20. Das, A.S., Dutt, J.K.: Reduced model of a rotor-shaft system using modified SEREP. Mech. Res. Commun. 35(6), 398–407 (2008)

    Article  MATH  Google Scholar 

  21. Sun, C., Chen, Y., Hou, L.: Steady-state response characteristics of a dual-rotor system induced by rub-impact. Nonlinear Dyn. 86(1), 1–15 (2016)

    Article  Google Scholar 

  22. Kuan, L., Zhenyong, L., Chen, Y.: Comparative study of two order reduction methods for high-dimensional rotor systems. Int. J. Non-linear Mech. 106(11), 330–334 (2018)

    Google Scholar 

  23. He, F., Dousti, S., Allaire, P., Dousti, M.: Squeeze film damper effect on vibration of an unbalanced flexible rotor using harmonic balance method. J. Eng. Sci. Technol. XX(Y(3)), 1–19 (2017)

    Google Scholar 

  24. David, J.W., Mitchell, L.D., Daws, J.W.: Using transfer matrices for parametric system forced response. J. Vib. Acoust. 109(4), 356–360 (1987)

    Article  Google Scholar 

  25. Lee, A.-C., Kang, Y., Liu, S.-L.: Steady-state analysis of a rotor mounted on nonlinear bearings by the transfer matrix method. Int. J. Mech. Sci. 35(6), 479–490 (1993)

    Article  MATH  Google Scholar 

  26. Liew, A., Feng, N.S., Hahn, E.J.: A non-linear transfer matrix technique for statically indeterminate rotor bearing systems. Proc. Inst. Mech. Eng. Part C-J. Mech. Eng. Sci. 215(11), 1343–1355 (2001)

    Article  Google Scholar 

  27. Hsieh, S.-C., Chen, J.-H., Lee, A.-C.: A modified transfer matrix method for the coupling lateral and torsional vibrations of symmetric rotor-bearing systems. J. Sound Vib. 289(1–2), 294–333 (2006)

    Article  Google Scholar 

  28. Cheung, Y.K., Chen, S.H., Lau, S.L.: Application of the incremental harmonic balance method to cubic non-linearity systems. J. Sound Vib. 140(2), 273–286 (1990)

    Article  Google Scholar 

  29. Jin, Y., Yang, R., Hou, L., Chen, Y., Zhang, Z.: Experiments and numerical results for varying compliance vibrations in a rigid-rotor ball bearing system. J. Tribol. 139(4), 041103 (2017)

    Article  Google Scholar 

  30. Yasuda, K., Torii, T., Kasahara, M.: Proposition of an incremental transfer matrix method for nonlinear vibration analysis. Trans. Jpn. Soc. Mech. Eng. C. 34(1), 12–18 (1991)

    Google Scholar 

  31. Zhang, Z., Chen, Y., Cao, Q.: Bifurcations and hysteresis of varying compliance vibrations in the primary parametric resonance for a ball bearing. J. Sound Vib. 350, 171–184 (2015)

    Article  Google Scholar 

  32. Chen, G.: Vibration modeling and verifications for whole aero-engine. J. Sound Vib. 349(4), 163–176 (2015)

    Article  Google Scholar 

  33. Vijayasree, N.K., Munjal, M.L.: On an integrated transfer matrix method for multiply connected mufflers. Journal of Sound and Vibration. 350, 171–184 (2015)

    Article  Google Scholar 

  34. Parrinello, A., Ghiringhelli, G.L.: Transfer matrix representation for periodic planar media. J. Sound Vib. 317(9), 196–209 (2016)

    Article  Google Scholar 

Download references

Acknowledgement

This work was supported by the National Science Foundation of China [Grant Numbers 11872148, 11572082]; the Fundamental Research Funds for the Central Universities of China [Grant Numbers N170308028, N160312001]; and the Excellent Talents Support Program in Institutions of Higher Learning in Liaoning Province of China [Grant Number LJQ2015038].

Author information

Authors and Affiliations

  1. School of Mechanical Engineering and Automation, Northeastern University, Shenyang, 110819, People’s Republic of China

    Zhong Luo & Haopeng Liu

  2. Key Laboratory of Vibration and Control of Aero-Propulsion System Ministry of Education, Northeastern University, Shenyang, 110819, China

    Zhong Luo & Haopeng Liu

  3. College of Intelligence Science and Technology, National University of Defense Technology, Changsha, 410073, China

    Zifang Bian

  4. Department of Automatic Control and System Engineering, University of Sheffield, Sheffield, S13JD, UK

    Yunpeng Zhu

Authors
  1. Zhong Luo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zifang Bian
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yunpeng Zhu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Haopeng Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Zhong Luo or Yunpeng Zhu.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix 1

Appendix 1

$$ {\user2{T}}_{o} = \left| {\begin{array}{*{20}l} {\begin{array}{*{20}l} {\frac{{\partial F_{x0} }}{{\partial a_{0} }}} & {\frac{{\partial F_{x0} }}{{\partial c_{0} }}} & 0 & 0 \\ {\frac{{\partial F_{y0} }}{{\partial a_{0} }}} & {\frac{{\partial F_{y0} }}{{\partial c_{0} }}} & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \\ \end{array} } & \cdots & {\begin{array}{*{20}l} {\frac{{\partial F_{x0} }}{{\partial a_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial c_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial b_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{y0} }}{{\partial a_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial c_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial b_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial d_{j} }}} \\ 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ \end{array} } & {{\user2{O}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 2} } \\ \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ {\begin{array}{*{20}l} {\begin{array}{*{20}l} {\frac{{\partial F_{xck} }}{{\partial a_{0} }}} & {\frac{{\partial F_{xck} }}{{\partial a_{0} }}} \\ {\frac{{\partial F_{yck} }}{{\partial a_{0} }}} & {\frac{{\partial F_{yck} }}{{\partial c_{0} }}} \\ {\frac{{\partial F_{xsk} }}{{\partial a_{0} }}} & {\frac{{\partial F_{xsk} }}{{\partial c_{0} }}} \\ {\frac{{\partial F_{ysk} }}{{\partial a_{0} }}} & {\frac{{\partial F_{ysk} }}{{\partial c_{0} }}} \\ \end{array} } & {{\user2{O}}_{4 \times 2} } \\ \end{array} } & \cdots & {\begin{array}{*{20}l} {\frac{{\partial F_{xck} }}{{\partial a_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial c_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial b_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{yck} }}{{\partial a_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial c_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial b_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{xsk} }}{{\partial a_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial c_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial b_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{ysk} }}{{\partial a_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial c_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial b_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial d_{j} }}} \\ \end{array} } & {{\user2{O}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 2} } \\ {{\user2{O}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 4} } & {{\user2{I}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 2} } \\ \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ {{\user2{O}}_{2 \times 4} } & \cdots & {{\user2{O}}_{2 \times 4} } & {{\user2{O}}_{2 \times 4} } & \cdots & {{\user2{I}}_{2 \times 2} } \\ \end{array} } \right| $$
(A-1)

R and P in Sect. 2.2 can be expressed into Fourier series, as follows:

$$ R = R_{0} + \sum\limits_{i = 1}^{N} {R_{ic} \cos (i\varOmega t) + R_{is} \sin (i\varOmega t)} $$
(A-2)
$$ P = P_{0} + \sum\limits_{i = 1}^{N} {P_{ic} \cos (i\varOmega t) + P_{is} \sin (i\varOmega t)} $$
(A-3)
$$ {\user2{T}}_{i} = \left| {\begin{array}{*{20}l} {\begin{array}{*{20}l} {\frac{{\partial F_{x0} }}{{\partial a_{0} }}} & {\frac{{\partial F_{x0} }}{{\partial c_{0} }}} & { - 1} & 0 \\ {\frac{{\partial F_{y0} }}{{\partial a_{0} }}} & {\frac{{\partial F_{y0} }}{{\partial c_{0} }}} & 0 & { - 1} \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \\ \end{array} } & \cdots & {\begin{array}{*{20}l} {\frac{{\partial F_{x0} }}{{\partial a_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial c_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial b_{j} }}} & {\frac{{\partial F_{x0} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{y0} }}{{\partial a_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial c_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial b_{j} }}} & {\frac{{\partial F_{y0} }}{{\partial d_{j} }}} \\ 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ \end{array} } & {{\user2{O}}_{4 \times 4} } & \cdots & {\begin{array}{*{20}l} 0{R_{0} } \\ 0{P_{0} } \\ 00 \\ 00 \\ \end{array} } \\ \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ {\begin{array}{*{20}l} {\begin{array}{*{20}l} {\frac{{\partial F_{xck} }}{{\partial a_{0} }}} & {\frac{{\partial F_{xck} }}{{\partial a_{0} }}} \\ {\frac{{\partial F_{yck} }}{{\partial a_{0} }}} & {\frac{{\partial F_{yck} }}{{\partial c_{0} }}} \\ {\frac{{\partial F_{xsk} }}{{\partial a_{0} }}} & {\frac{{\partial F_{xsk} }}{{\partial c_{0} }}} \\ {\frac{{\partial F_{ysk} }}{{\partial a_{0} }}} & {\frac{{\partial F_{ysk} }}{{\partial c_{0} }}} \\ \end{array} } & {{\user2{O}}_{4 \times 2} } \\ \end{array} } & \cdots & {\begin{array}{*{20}l} {\frac{{\partial F_{xck} }}{{\partial a_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial c_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial b_{j} }}} & {\frac{{\partial F_{xck} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{yck} }}{{\partial a_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial c_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial b_{j} }}} & {\frac{{\partial F_{yck} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{xsk} }}{{\partial a_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial c_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial b_{j} }}} & {\frac{{\partial F_{xsk} }}{{\partial d_{j} }}} \\ {\frac{{\partial F_{ysk} }}{{\partial a_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial c_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial b_{j} }}} & {\frac{{\partial F_{ysk} }}{{\partial d_{j} }}} \\ \end{array} } & { - {\user2{I}}_{4 \times 4} } & \cdots & {\begin{array}{*{20}l} 0{R_{ic} } \\ 0{P_{ic} } \\ 0{R_{is} } \\ 0{P_{is} } \\ \end{array} } \\ {{\user2{O}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 4} } & {{\user2{I}}_{4 \times 4} } & \cdots & {{\user2{O}}_{4 \times 2} } \\ \vdots & \vdots & \vdots & \vdots & \vdots & \vdots \\ {{\user2{O}}_{2 \times 4} } & \cdots & {{\user2{O}}_{2 \times 4} } & {{\user2{O}}_{2 \times 4} } & \cdots & {{\user2{I}}_{2 \times 2} } \\ \end{array} } \right| $$
(A-4)

The state vector of the ball-bearing transfer matrix is presented as follows:

$$ \left[ {\begin{array}{*{20}l} {\Delta x_{0} }{\Delta y_{0} }{\Delta Q_{x,0} }{\Delta Q_{y,0} } \cdots {\Delta x_{ic} }{\Delta y_{ic} }{\Delta x_{is} }{\Delta y_{is} }{\Delta Q_{x,ic} }{\Delta Q_{y,ic} }{\Delta Q_{x,is} }{\Delta Q_{y,is} } \cdots {\Delta \varOmega }1 \\ \end{array} } \right]^{\text{T}} $$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Luo, Z., Bian, Z., Zhu, Y. et al. An improved transfer-matrix method on steady-state response analysis of the complex rotor-bearing system. Nonlinear Dyn 102, 101–113 (2020). https://doi.org/10.1007/s11071-020-05952-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-05952-5

Keywords

Search

Navigation