On Determination of the Linear Viscoelastic Compliance and Relaxation Functions for Polymers in One Tensile Test

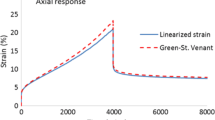
Usually, the viscoelastic (VE) response of polymers for applications in composites is obtained in uniaxial strainor stress-controlled tests. However, analyzing multimaterial structures by the Finite Element Method (FEM) or by other numerical or analytical tools, a material model in terms of a complete set of compliance functions and/or relaxation functions is required. In this paper, a methodology and exact analytical expressions for calculating the whole set of VE functions is presented based on the relaxation modulus E(t)and Poisson’s ratio v (t) determined in strain-controlled tests. The method is based on Laplace transforms, where an exact inversion is possible if a linear VE model with functions in Prony series is used. Results of the analytical model are compared with the FEM simulation, where specific boundary conditions to determine each particular VE function are used. Finally, the applicability of the so-called quasi-elastic method is investigated, where the expressions of elasticity theory are used to calculate a given viscoelastic function at an instant of time t_k using the instant values of E(t_k) and v(t_k). For isotropic materials, the three approaches render almost coinciding results.

Appendices

Appendix 1. Relaxation Functions C ₁₁ (t) and C ₁₂(t)

In the Laplace domain, the relationships between the compliance functions ${\overline{S}}_{ij}$ and relaxation functions ${\overline{C}}_{ij},i,j=1,2,$ for isotropic material can be obtained from Eqs. (40) and (42):

$$ {\overline{C}}_{11}={\overline{C}}_{22}={\overline{C}}_{33}=\frac{1}{p^2}\frac{{\overline{S}}_{11}+{\overline{S}}_{12}}{\left({\overline{S}}_{11}-{\overline{S}}_{12}\right)\left({\overline{S}}_{11}-2{\overline{S}}_{12}\right)}, $$

(A1.1)

$$ {\overline{C}}_{12}={\overline{C}}_{13}={\overline{C}}_{23}=\frac{1}{p^2}\frac{{\overline{S}}_{12}}{\left({\overline{S}}_{11}-{\overline{S}}_{12}\right)\left({\overline{S}}_{11}-2{\overline{S}}_{12}\right)}. $$

(A1.2)

Substituting in (A1.1) and (A1.2) expressions (54) for ${\overline{S}}_{11}$ and ${\overline{S}}_{12}$, gives

$$ {\overline{C}}_{11}=\frac{\left[{\varPi}_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]\left({\sum}_{k=0}^M{E}_1^k{S}_k^q(p)\right)}{p\left[{\varPi}_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]\left[{\varPi}_{m=1}^M\left(p+\frac{1}{q_m}\right)-2{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]}, $$

(A1.3)

$$ {\overline{C}}_{12}=\frac{\left({\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right)\left({\sum}_{k=0}^M{E}_1^k{S}_k^q(p)\right)}{p\left[{\varPi}_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]\left[{\varPi}_{m=1}^M\left(p+\frac{1}{q_m}\right)-2{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]}. $$

(A1.4)

The 2M +1 singular points of (A1.3) needed for inverse transformation are found numerically as roots of polynomials in the denominator $\left({p}_k=0,-\frac{1}{r_1},-\frac{1}{r_2},-\frac{1}{r_3},\dots, -\frac{1}{r_{2M}}\right).$ Then, using the partial fraction decomposition, the constants ${C}_{11}^k$ and ${C}_{12}^k$ are found similarly as described in Sect. 5.2. The expressions in the time domain are

$$ {C}_{ij}(t)={C}_{ij}^0+\sum_{m=1}^{2M}{C}_{ij}^me\left(-\frac{t}{r_m}\right), $$

(A1.5)

$$ {C}_{11}^k=\frac{1}{\left(1+{v}_{12}(0)\right)\left(1-2{v}_{12}(0)\right)}\frac{\left[{\varPi}_{m=1}^M\left(-\frac{1}{r_k}+\frac{1}{q_m}\right)-{\sum}_{k=0}^M{v}_{12}^m{S}_m^q\left(-\frac{1}{r_k}\right)\right]\left({\sum}_{m=0}^M{E}_1^m{S}_m^q\left(-\frac{1}{r_k}\right)\right)}{S_k^r\left(-\frac{1}{r_k}\right)}, $$

(A1.6)

$$ {C}_{12}^k=\frac{1}{\left(1+{v}_{12}(0)\right)\left(1-2{v}_{12}(0)\right)}\frac{\left({\sum}_{m=0}^M{v}_{12}^m{S}_m^q\left(-\frac{1}{r_k}\right)\right)\left({\sum}_{m=0}^M{E}_1^m{S}_m^q\left(-\frac{1}{r_k}\right)\right)}{S_k^r\left(-\frac{1}{r_k}\right)}, $$

(A1.7)

In (A1.6) and (A1.7),

$$ {S}_k^r(p)={\prod}_{\underset{i\ne k}{i=0}}^{2M}\left(p+{z}_i\right),\textrm{where}\ {z}_i=0,\frac{1}{r_1},\frac{1}{r_2},\frac{1}{r_3},\dots, \frac{1}{r_{2M}}. $$

(A1.8)

Appendix 2. Viscoelastic Shear Functions C ₆₆ ( t ) = Q ₆₆ (t) = G ₁₂ ( t ) and S ₆₆ ( t )

In the Laplace domain, the relations between shear stress and strain for isotropic materials can be written as

$$ {\overline{C}}_{66}=\frac{1}{p^2}\frac{1}{{\overline{S}}_{66}}=\frac{1}{2{p}^2}\frac{1}{{\overline{S}}_{11}-{\overline{S}}_{12}}. $$

(A2.1)

Inserting (54) into (A2.1), it is obtained that

$$ {\overline{C}}_{66}=\frac{1}{2{p}^2}\frac{\sum_{k=0}^M{E}_1^k{S}_k^q(p)}{\prod_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)}. $$

(A2.2)

The creep compliance in shear is

$$ {\overline{S}}_{66}=\frac{2}{p}\frac{\prod_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)}{\sum_{k=0}^M{E}_1^k{S}_k^q(p)}. $$

(A2.3)

The singular points of (A2.2) are found numerically and denoted ${p}_k=-\frac{1}{\rho_k},k=1,\dots, M.$ The singular points of (A2.3) are the same as for ${\overline{S}}_{11},\kern0.5em {p}_k=-\frac{1}{\tau_k},\kern1em k=1,2,\dots, M.$

Expressing, as described above, the rational functions in partial fractions, the following expressions were obtained:

$$ {C}_{66}(t)={C}_{66}^0+\sum \limits_{m=1}^M{C}_{66}^me\left(-\frac{t}{\rho_m}\right),\kern1em {S}_{66}(t)={S}_{66}^0+\sum \limits_{m=1}^M{S}_{66}^me\left(-\frac{t}{\tau_m}\right), $$

(A2.4)

$$ {C}_{66}^k=\frac{1}{2\left(1+{v}_{12}(0)\right)}\frac{\sum_{m=0}^M{E}_1^m{S}_m^q\left(-\frac{1}{\rho_k}\right)}{S_k^{\rho}\left(-\frac{1}{\rho_k}\right)},{S}_{66}^k=\frac{2}{E_1(0)}\frac{\prod_{m=1}^M\left(-\frac{1}{\tau_k}+\frac{1}{q_m}\right)+{\sum}_{m=0}^M{v}_{12}^m{S}_m^q\left(-\frac{1}{\tau_k}\right)}{S_k^{\tau}\left(-\frac{1}{\tau_k}\right)}, $$

(A2.5)

where

$$ {S}_k^{\rho }(p)={\prod}_{\underset{i\ne k}{i=0}}^M\left(p+{w}_i\right),\kern0.5em {w}_i=0,\frac{1}{\rho_1},\frac{1}{\rho_2},\frac{1}{\rho_3},\dots, \frac{1}{\rho_M}. $$

(A2.6)

Appendix 3. Stress Relaxation Functions Q ₁₁ (t) and Q ₁₂ (t)

The relationships between the stress relaxation functions and compliance functions in the Laplace domain are simplified for cases where the out-of-plane normal stress can be neglected (σ₃ = 0 ), as it is assumed in the classical laminate theory (CLT) for composites. For isotropic plies (for example, polymers, aluminum etc.),

$$ {\overline{Q}}_{11}={\overline{Q}}_{22}=\frac{1}{p^2}\frac{{\overline{S}}_{11}}{\left({\overline{S}}_{11}-{\overline{S}}_{12}\right)\left({\overline{S}}_{11}+{\overline{S}}_{12}\right)}, $$

(A3.1)

$$ {\overline{Q}}_{12}=-\frac{1}{p^2}\frac{{\overline{S}}_{12}}{\left({\overline{S}}_{11}-{\overline{S}}_{12}\right)\left({\overline{S}}_{11}+{\overline{S}}_{12}\right)}. $$

(A3.2)

Inserting (54) into (A3.1) and (A3.2) gives

$$ {\overline{Q}}_{11}=\frac{\left({\sum}_{k=0}^M{E}_1^k{S}_k^q(p)\right){\prod}_{m=1}^M\left(p+\frac{1}{q_m}\right)}{p\left[{\prod}_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]\left[{\prod}_{m=1}^M\left(p+\frac{1}{q_m}\right)-{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]}, $$

(A3.3)

$$ {\overline{Q}}_{12}=\frac{\left({\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right)\left({\sum}_{k=0}^M{E}_1^k{S}_k^q(p)\right)}{p\left[{\prod}_{m=1}^M\left(p+\frac{1}{q_m}\right)+{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]\left[{\prod}_{m=1}^M\left(p+\frac{1}{q_m}\right)-{\sum}_{k=0}^M{v}_{12}^k{S}_k^q(p)\right]}. $$

(A3.4)

The singular points of ${\overline{Q}}_{11}$ and ${\overline{Q}}_{11}$ are found numerically as roots of polynomials in the denominator $\left({p}_k=0,-\frac{1}{\theta_1},-\frac{1}{\theta_2},-\frac{1}{\theta_3},\dots, -\frac{1}{\theta_{2M}}\right).$ Then, using the partial fraction decomposition, the constants ${Q}_{11}^k$ and ${Q}_{1k}^k$ are found as described in Sect 5.2. The expressions after inversion are

$$ {Q}_{ij}(t)={Q}_{ij}^0+\sum \limits_{m=1}^{2M}{Q}_{ij}^me\left(-\frac{t}{\theta_m}\right), $$

(A3.5)

$$ {Q}_{11}^k=\frac{1}{\left(1+{v}_{12}(0)\right)\left(1-{v}_{12}(0)\right)}\frac{\prod_{m=1}^M\left(-\frac{1}{\theta_k}+\frac{1}{q_m}\right)\left({\sum}_{m=0}^M{E}_1^m{S}_m^q\left(-\frac{1}{\theta_k}\right)\right)}{S_k^{\theta}\left(-\frac{1}{\theta_k}\right)}, $$

(A3.6)

$$ {Q}_{12}^k=\frac{1}{\left(1+{v}_{12}(0)\right)\left(1-{v}_{12}(0)\right)}\frac{\left({\sum}_{m=0}^M{v}_{12}^m{S}_m^q\left(-\frac{1}{\theta_k}\right)\right)\left({\sum}_{m=0}^M{E}_1^m{S}_m^q\left(-\frac{1}{\theta_k}\right)\right)}{S_k^{\theta}\left(-\frac{1}{\theta_k}\right)}, $$

(A3.7)

In (A3.6) and (A3.7),

$$ {S}_k^{\theta }(p)={\prod}_{\underset{i\ne k}{i=0}}^{2M}\left(p+{v}_i\right),\textrm{where}\ {v}_i=0,\frac{1}{\theta_1},\frac{1}{\theta_2},\frac{1}{\theta_3},\dots, \frac{1}{\theta_{2M}}. $$

(A3.8)