Skip to main content
SpringerLink
Log in

Forced vibration of an axially moving laminated composite cylindrical shallow shell

  • Published:
Meccanica Aims and scope Submit manuscript

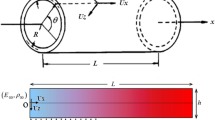
Abstract

An axially moving composite cylindrical shallow shell is presented in a wide class of engineering problem, such as the space sailors drag sail mechanism and the deployable antennas. It is very important to have a better understanding of the vibrational characteristics of an axially moving composite cylindrical shallow shell. Nonlinear forced vibrations of an axially moving thin laminated composite cylindrical shallow shell are investigated in the present work. The governing equation and the compatibility equation are derived based on the von Kármán plate theory and discretized by the Galerkin method in a system of ordinary differential equations. The ordinary differential equations are solved by employing the harmonic balance method to obtain the approximate analytical response. Moreover, the stability of the analytical response is also determined. The model is validated by comparing with the natural frequencies available in the literature and the dynamic response obtained through the Runge–Kutta method. Natural frequencies and frequency–response characteristics are discussed in detail. The effects of the movement speed, curvature radius on the natural frequencies are examined. The system has a strong hardening nonlinear behavior and complex dynamic behavior. The frequency–response curve possesses three peaks, due to the strong nonlinear modal coupling. It is further found that the effects of the movement speed, curvature radius, transverse excitation amplitude, damping coefficient and initial tension on the nonlinear dynamic response of an axially moving thin laminated composite cylindrical shallow shell are examined.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Liu Y, Qin Z, Chu F (2022) Nonlinear forced vibrations of rotating cylindrical shells under multi-harmonic excitations in thermal environment. Nonlinear Dynam 108(4):2977–2991. https://doi.org/10.1007/s11071-022-07449-9

    Article  Google Scholar 

  2. Toorani MH (2003) Dynamics of the geometrically non-linear analysis of anisotropic laminated cylindrical shells. Int J Nonlinear Mech 38:1315–1335. https://doi.org/10.1016/S0020-7462(02)00073-2

    Article  MATH  Google Scholar 

  3. Zhao X, Ng TY, Liew KM (2004) Free vibration of two-side simply-supported laminated cylindrical panels via the mesh-free kp-Ritz method. Int J Mech Sci 46(1):123–142. https://doi.org/10.1016/j.ijmecsci.2004.02.010

    Article  MATH  Google Scholar 

  4. Shakeri M, Alibeigloo A (2005) Dynamic analysis of orthotropic laminated cylindrical panels. Mech Adv Mater Struct 12(1):67–75. https://doi.org/10.1080/15376490490493925

    Article  Google Scholar 

  5. Naidu NVS, Sinha PK (2007) Nonlinear free vibration analysis of laminated composite shells in hygrothermal environments. Compos Struct 77:475–483. https://doi.org/10.1016/j.compstruct.2005.08.002

    Article  Google Scholar 

  6. Nanda N, Bandyopadhyay JN (2007) Nonlinear free vibration analysis of laminated composite cylindrical shells with cutouts. J Reinf Plast Comp 26:1413–1427. https://doi.org/10.1177/0731684407079776

    Article  Google Scholar 

  7. Nanda N, Bandyopadhyay JN (2008) Nonlinear transient response of laminated composite shells. J Eng Mech 134:983–990. https://doi.org/10.1061/(ASCE)0733-9399(2008)134:11(983)

    Article  Google Scholar 

  8. Qing G, Liu Y, Guo Q, Zhang D (2008) Dynamic analysis for three-dimensional laminated plates and panels with damping. Int J Mech Sci 50(1):83–91. https://doi.org/10.1016/j.ijmecsci.2007.05.002

    Article  MATH  Google Scholar 

  9. Ribeiro P, Jansen E (2008) Non-linear vibrations of laminated cylindrical shallow shells under thermomechanical loading. J Sound Vib 315:626–640. https://doi.org/10.1016/j.jsv.2008.01.017

    Article  Google Scholar 

  10. Ribeiro P (2009) On the influence of membrane inertia and shear deformation on the geometrically non-linear vibrations of open, cylindrical, laminated clamped shells. Compos Sci Technol 69:176–185. https://doi.org/10.1016/j.compscitech.2008.09.038

    Article  Google Scholar 

  11. Hashemian AH, Jam JE (2010) Nonlinear free dynamic response of laminated compressible cylindrical shell panels. Mech Compos Mater 46:15–28. https://doi.org/10.1007/s11029-010-9122-5

    Article  Google Scholar 

  12. Nanda N, Pradyumna S (2011) Nonlinear dynamic response of laminated shells with imperfections in hygrothermal environments. J Compos Mater 45:2103–2112. https://doi.org/10.1177/0021998311401061

    Article  Google Scholar 

  13. Mohammadi F, Sedaghati R (2012) Nonlinear free vibration analysis of sandwich shell structures with a constrained electrorheological fluid layer. Smart Mater Struct 21(7):416–422. https://doi.org/10.1088/0964-1726/21/7/075035

    Article  Google Scholar 

  14. Bodaghi M, Shakeri M (2012) An analytical approach for free vibration and transient response of functionally graded piezoelectric cylindrical panels subjected to impulsive loads. Compos Struct 94(5):1721–1735. https://doi.org/10.1016/j.compstruct.2012.01.009

    Article  Google Scholar 

  15. Alijani F, Amabili M (2013) Nonlinear vibrations of thick laminated circular cylindrical panels. Compos Struct 96:643–660. https://doi.org/10.1016/j.compstruct.2012.09.016

    Article  Google Scholar 

  16. Zhang LW, Lei ZX, Liew KM, Yu JL (2014) Static and dynamic of carbon nanotube reinforced functionally graded cylindrical panels. Compos Struct 111(3):205–212. https://doi.org/10.1016/j.compstruct.2013.12.035

    Article  Google Scholar 

  17. Tornabene F, Brischetto S, Fantuzzi N, Viola E (2015) Numerical and exact models for free vibration analysis of cylindrical and spherical shell panels. Compos Part B Eng 81:231–250. https://doi.org/10.1016/j.compositesb.2015.07.015

    Article  Google Scholar 

  18. Kiani Y (2017) Dynamics of FG-CNT reinforced composite cylindrical panel subjected to moving load. Thin Wall Struct 111:48–57. https://doi.org/10.1016/j.tws.2016.11.011.10.1016/j.tws.2016.11.011

    Article  Google Scholar 

  19. Alijani F, Amabili M, Balasubramanian P, Carra S, Ferrari G, Garziera R (2016) Damping for large-amplitude vibrations of plates and curved panels, Part 1: modeling and experiments. Int J Nonlinear Mech 85(10):23–40. https://doi.org/10.1016/j.ijnonlinmec.2016.05.003

    Article  Google Scholar 

  20. Shen HS, Xiang Y, Fan Y, Hui D (2018) Nonlinear vibration of functionally graded graphene-reinforced composite laminated cylindrical panels resting on elastic foundations in thermal environments. Compos Part B Eng 136:177–186. https://doi.org/10.1016/j.compositesb.2017.10.032

    Article  Google Scholar 

  21. Zhai Y, Chai M, Su J, Liang S (2018) Dynamics properties of composite sandwich open circular cylindrical shells. Compos Struct 189(4):148–159. https://doi.org/10.1016/j.compstruct.2018.01.076

    Article  Google Scholar 

  22. Wang Q, Shao D, Qin B (2018) A simple first-order shear deformation shell theory for vibration analysis of composite laminated open cylindrical shells with general boundary conditions. Compos Struct 184:211–232. https://doi.org/10.1016/j.compstruct.2017.09.070

    Article  Google Scholar 

  23. Chakraborty S, Dey T, Kumar R (2019) Stability and vibration analysis of CNT-Reinforced functionally graded laminated composite cylindrical shell panels using semi-analytical approach. Compos Part B Eng 168(7):1–14. https://doi.org/10.1016/j.compositesb.2018.12.051

    Article  Google Scholar 

  24. Bisheh H, Civalek O (2020) Vibration of smart laminated carbon nanotube-reinforced composite cylindrical panels on elastic foundations in hygrothermal environments. Thin Wall Struct. https://doi.org/10.1016/j.tws.2020.106945

    Article  Google Scholar 

  25. Farsadi T, Rahmanian M, Kurtaran H (2021) Nonlinear lay-up optimization of variable stiffness composite skew and taper cylindrical panels in free vibration. Compos Struct. https://doi.org/10.1016/j.compstruct.2021.113629

    Article  Google Scholar 

  26. Wang YQ, Huang XB, Li J (2016) Hydroelastic dynamic analysis of axially moving plates in continuous hot-dip galvanizing process. Int J Mech Sci 110:201–216. https://doi.org/10.1016/j.ijmecsci.2016.03.010

    Article  Google Scholar 

  27. Wolff N, Seefeldt P, Bauer W, Fiebig C, Gerding P, Parow-Souchon K, Pongs A, Reiffenrath M, Ziemann T (2014) Alternative application of solar sail technology. In: Macdonald M (ed) Advances in solar sailing, springer praxis books. Springer, Berlin, Heidelberg, pp 351–365. https://doi.org/10.1007/978-3-642-34907-2_23

    Chapter  Google Scholar 

  28. Costantine J, Tawk Y, Christodoulou CG, Banik J, Lane S (2012) CubeSat deployable antenna using bistable composite tape-springs. IEEE Antenn Wirel Propag 11:285–288. https://doi.org/10.1109/LAWP.2012.2189544

    Article  Google Scholar 

  29. Hu J, Lin S, Dai F (2017) Pattern reconfigurable antenna based on morphing bistable composite laminates. IEEE T Antenn Propag. https://doi.org/10.1109/TAP.2017.2677258

    Article  Google Scholar 

  30. Mohamadi A, Shahgholi M, Ghasemi FA (2020) Nonlinear vibration of axially moving simply-supported circular cylindrical shell. Thin Wall Struct. https://doi.org/10.1016/j.tws.2020.107026

    Article  Google Scholar 

  31. Lin CC (1997) Stability and vibration characteristics of axially moving plates. Int J Solids Struct 34(24):3179–3190. https://doi.org/10.1016/S0020-7683(96)00181-3

    Article  MATH  Google Scholar 

  32. Wang X (1999) Numerical analysis of moving orthotropic thin plates. Comput Struct 70(4):467–486. https://doi.org/10.1016/S0045-7949(98)00161-8

    Article  MATH  Google Scholar 

  33. Luo Z, Hutton SG (2002) Formulation of a three-node traveling triangular plate element subjected to gyroscopic and in-plane forces. Comput Struct 80(26):1935–1944. https://doi.org/10.1016/S0045-7949(02)00291-2

    Article  Google Scholar 

  34. Luo ACJ, Hamidzadeh HR (2004) Equilibrium and buckling stability for axially traveling plates. Commun Nonlinear Sci 9(3):343–360. https://doi.org/10.1016/S1007-5704(02)00132-6

    Article  MATH  Google Scholar 

  35. Hatami S, Azhari M, Saadatpour MM (2007) Free vibration of moving laminated composite plates. Compos Struct 80(4):609–620. https://doi.org/10.1016/j.compstruct.2006.07.009

    Article  MATH  Google Scholar 

  36. Hatami S, Ronagh HR, Azharia M (2008) Exact free vibration analysis of axially moving viscoelastic plates. Comput Struct 86(17–18):1738–1746. https://doi.org/10.1016/j.compstruc.2008.02.002

    Article  Google Scholar 

  37. Zhou YF, Wang ZM (2008) Vibrations of axially moving viscoelastic plate with parabolically varying thickness. J Sound Vib 316(1–5):198–210. https://doi.org/10.1016/j.jsv.2008.02.040

    Article  Google Scholar 

  38. Banichuk N, Jeronen J, Neittaanmäki P, Tuovinen T (2010) On the instability of an axially moving elastic plate. Int J Solids Struct 47(1):91–99. https://doi.org/10.1016/j.ijsolstr.2009.09.020

    Article  MATH  Google Scholar 

  39. Marynowski K (2010) Free vibration analysis of the axially moving Levy-type viscoelastic plate. Eur J Mech A Solid 29(5):879–886. https://doi.org/10.1016/j.eurchsol.2010.03.010

    Article  MATH  Google Scholar 

  40. Yang XD, Chen LQ, Zu JW (2011) Vibrations and stability of an axially moving rectangular composite plate. J Appl Mech T ASME 78(1):1–26. https://doi.org/10.1115/1.4002002

    Article  Google Scholar 

  41. Tang YQ, Chen LQ (2011) Nonlinear free transverse vibrations of in-plane moving plates: Without and with internal resonances. J Sound Vib 330(1):110–126. https://doi.org/10.1016/j.jsv.2010.07.005

    Article  Google Scholar 

  42. Tang YQ, Chen LQ (2012) Primary resonance in forced vibrations of in-plane translating viscoelastic plates with 3:1 internal resonance. Nonlinear Dyn 69(1–2):159–172. https://doi.org/10.1007/s11071-011-0253-6

    Article  MathSciNet  MATH  Google Scholar 

  43. Yang XD, Zhang W, Chen LQ, Yao MH (2012) Dynamical analysis of axially moving plate by finite difference method. Nonlinear Dyn 67:997–1006. https://doi.org/10.1007/s11071-011-0042-2

    Article  MathSciNet  MATH  Google Scholar 

  44. Ghayesh MH, Amabili M (2013) Non-linear global dynamics of an axially moving plate. Int J Nonlin Mech 57(4):16–30. https://doi.org/10.1016/j.ijnonlinmec.2013.06.005

    Article  Google Scholar 

  45. Wang YQ, Liang L, Guo XH (2013) Internal resonance of axially moving laminated circular cylindrical shells. J Sound Vib 332(24):6434–6450. https://doi.org/10.1016/j.jsv.2013.07.007

    Article  Google Scholar 

  46. Wang YQ, Zu JW (2018) Vibration characteristics of moving sigmoid functionally graded plates containing porosities. Int J Mech Mater Des 14:473–489. https://doi.org/10.1007/s10999-017-9385-2

    Article  Google Scholar 

  47. Wang YQ, Wan YH, Zhang YF (2017) Vibrations of longitudinally traveling functionally graded material plates with porosities. Eur J Mech A Solid 66:55–68. https://doi.org/10.1016/j.euromechsol.2017.06.006

    Article  MathSciNet  MATH  Google Scholar 

  48. Wang YQ, Yang ZB (2017) Nonlinear vibrations of moving functionally graded plates containing porosities and contacting with liquid: internal resonance. Nonlinear Dyn 90:1461–1480. https://doi.org/10.1007/s11071-017-3739-z

    Article  Google Scholar 

  49. Wang YQ, Zu JW (2017) Nonlinear dynamic thermoelastic response of rectangular FGM plates with longitudinal velocity. Compos Part B Eng 117:74–88. https://doi.org/10.1016/j.compositesb.2017.02.037

    Article  Google Scholar 

  50. Li C, Liu JJ, Cheng M, Fan XL (2017) Nonlocal vibrations and stabilities in parametric resonance of axially moving viscoelastic piezoelectric nanoplate subjected to thermo-electro-mechanical forces. Compos Part B Eng 116:153–169. https://doi.org/10.1016/j.compositesb.2017.01.071

    Article  Google Scholar 

  51. Zhang DB, Tang YQ, Ding H, Chen LQ (2019) Parametric and internal resonance of a transporting plate with a varying tension. Nonlinear Dyn 98(4):2491–2508. https://doi.org/10.1007/s11071-019-04981-z

    Article  MATH  Google Scholar 

  52. Zhang DB, Tang YQ, Chen LQ (2019) Internal resonance in parametric vibrations of axially accelerating viscoelastic plates. Eur J Mech A Solid 75:142–155. https://doi.org/10.1016/j.euromechsol.2019.01.021

    Article  MathSciNet  MATH  Google Scholar 

  53. Mohamadi A, Shahgholi M, Ghasemi FA (2019) Free vibration and stability of an axially moving thin circular cylindrical shell using multiple scales method. Meccanica 54(14):2227–2246. https://doi.org/10.1007/s11012-019-01062-8

    Article  MathSciNet  Google Scholar 

  54. Wang YQ, Wu H, Yang FL, Wang Q (2021) An efficient method for vibration and stability analysis of rectangular plates axially moving in fluid. Appl Math Mech 42:291–308. https://doi.org/10.1007/s10483-021-2701-5

    Article  MathSciNet  MATH  Google Scholar 

  55. Mohamadi A, Shahgholi M, Ghasemi FA (2021) Nonlinear dynamic and bifurcations analysis of an axially moving circular cylindrical nanocomposite shell. Int J Mech Mater Des. https://doi.org/10.1007/s10999-021-09571-9

    Article  Google Scholar 

  56. Lotfan S, Anamagh MR, Bediz B (2021) A general higher-order model for vibration analysis of axially moving doubly-curved panels/shells. Thin Wall Struct 164:107813. https://doi.org/10.1016/j.tws.2021.107813

    Article  Google Scholar 

  57. Li M, Jiang WH, Li YQ, Dai FH (2022) Steady-state response of an axially moving circular cylindrical panel with internal resonance. Eur J Mech A Solid 92:104464. https://doi.org/10.1016/j.euromechsol.2021.104464

    Article  MathSciNet  MATH  Google Scholar 

  58. Li M, Li YQ, Liu XH, Dai FH (2022) A quasi-zero-stiffness vibration isolator using bi-stable hybrid symmetric laminate. Compos Struct 299:116047. https://doi.org/10.1016/j.compstruct.2022.116047

    Article  Google Scholar 

  59. Amabili M, Paidoussis MP (2003) Review of studies on geometrically nonlinear vibrations and dynamics of circular cylindrical shells and panels, with and without fluid-structure interaction. Appl Mech Rev 56(4):511–520. https://doi.org/10.1115/1.1565084

    Article  Google Scholar 

  60. Amabili M (2003) Nonlinear vibrations of circular cylindrical panels. J Sound Vib 281(3–5):509–535. https://doi.org/10.1016/j.jsv.2004.01.021

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of China [grant No. 11772107].

Author information

Authors and Affiliations

  1. National Key Laboratory of Science and Technology on Advanced Composites in Special Environment, Harbin Institute of Technology, Harbin, 150001, China

    Ming Li, Yanqi Li, Xiaohui Liu & Fuhong Dai

  2. School of Mechatronics Engineering, Harbin Institute of Technology, Harbin, 150001, China

    Dong Yu

Authors
  1. Ming Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yanqi Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xiaohui Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Fuhong Dai
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Dong Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Fuhong Dai or Dong Yu.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

The coefficients in Eq. (12) and Eq. (13) are given by

$$\begin{gathered} p_{1} = \frac{{2\xi^{2} \left( {D_{12} + 2D_{66} + 4B_{16} B_{26} P_{12} - B_{16} B_{26} P_{33} - 2B_{16}^{2} P_{11} - 2B_{26}^{2} P_{22} } \right)}}{{D_{11} - B_{16}^{2} P_{33} }},p_{2} = \frac{{\xi^{4} \left( {D_{22} - B_{26}^{2} P_{33} } \right)}}{{D_{11} - B_{16}^{2} P_{33} }}, \hfill \\ p_{3} = \frac{{h^{2} \xi^{4} }}{{P_{22} \left( {D_{11} - B_{16}^{2} P_{33} } \right)}},p_{4} = \frac{\xi }{h}\left( {2B_{16} P_{11} - 2B_{26} P_{12} - B_{26} P_{33} } \right),p_{5} = \frac{1}{\xi h}\left( {2B_{26} P_{22} - 2B_{16} P_{12} - B_{16} P_{33} } \right), \hfill \\ p_{6} = \frac{{\xi^{2} \left( {P_{33} - 2P_{12} } \right)}}{{P_{22} }},p_{7} = \frac{{\xi^{4} P_{11} }}{{P_{22} }} \hfill \\ \end{gathered}$$

The coefficients in Eq. (19) are given by

$$\begin{gathered} K_{1} { = }1,K_{2} { = }c,K_{3} { = } - \frac{16}{3}\gamma ,K_{4} { = } - \pi^{2} \gamma^{2} + \frac{{\kappa^{2} p_{3} }}{{1 + p_{6} + p_{7} }}{ + }\pi^{2} N_{0}^{{}} + \pi^{4} \left( {1 + p_{1} + p_{2} } \right),K_{5} { = } - \frac{8}{3}c\gamma , \hfill \\ K_{6} { = }\frac{{16\kappa p_{3} }}{{1 + p_{6} + p_{7} }},K_{7} { = }\frac{{64\kappa p_{3} }}{{5\left( {1 + p_{6} + p_{7} } \right)}} + \frac{{512\kappa p_{3} }}{{5\left( {16 + 4p_{6} + p_{7} } \right)}},K_{8} { = }\frac{{512p_{3} }}{{9\left( {1 + p_{6} + p_{7} } \right)}},K_{9} { = }\frac{{2048p_{3} }}{{15\left( {1 + p_{6} + p_{7} } \right)}} + \frac{{16384p_{3} }}{{25\left( {16 + 4p_{6} + p_{7} } \right)}}, \hfill \\ K_{10} { = } - 4F_{0} \sin \left( {\pi x_{0} } \right)\sin \left( {\pi \theta_{0} } \right) \hfill \\ \end{gathered}$$

The coefficients in Eq. (20) are given by

$$\begin{gathered} T_{1} { = }1,T_{2} { = }\frac{16}{3}\gamma ,T_{3} { = }c,T_{4} { = }\frac{8}{3}c\gamma ,T_{5} { = } - 4\pi^{2} \gamma^{2} + \frac{{16\kappa^{2} p_{3} }}{{16 + 4p_{6} + p_{7} }}{ + 4}\pi^{2} N_{0}^{{}} + \pi^{4} \left( {16 + 4p_{1} + p_{2} } \right), \hfill \\ T_{6} { = }\frac{{128\kappa p_{3} }}{{5\left( {1 + p_{6} + p_{7} } \right)}} + \frac{{1024\kappa p_{3} }}{{5\left( {16 + 4p_{6} + p_{7} } \right)}},T_{7} { = }\frac{{2048p_{3} }}{{15\left( {1 + p_{6} + p_{7} } \right)}} + \frac{{16384p_{3} }}{{25\left( {16 + 4p_{6} + p_{7} } \right)}},T_{8} { = }\frac{{8192p_{3} }}{{25\left( {1 + p_{6} + p_{7} } \right)}}, \hfill \\ T_{9} { = } - 4F_{0} \sin \left( {2\pi x_{0} } \right)\sin \left( {\pi \theta_{0} } \right) \hfill \\ \end{gathered}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, M., Li, Y., Liu, X. et al. Forced vibration of an axially moving laminated composite cylindrical shallow shell. Meccanica 58, 1581–1598 (2023). https://doi.org/10.1007/s11012-023-01693-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-023-01693-y

Keywords

Search

Navigation