Indentation analysis of coating structure based on parabolic concave indenter contact

Abstract

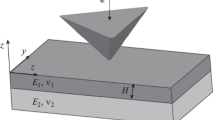
The development trend of functional materials and devices from block to thin film is inevitable. The elastic field of coating structure loaded with concave indenter is studied in this paper. Based on the mirror method and potential theory method, the analytical solutions of the elastic field of coating structure loaded with parabolic concave indenter are obtained. The convergence problem caused by the mirror image method is analyzed. The displacements and stresses with explicit formulation in the coating/substrate are obtained. The stress distribution inside the coating and at the interface is explored. The influence of coating thickness on interfacial stresses and the problem of the interface failure are discussed. The solution is degraded to the existing theory by numerical method for verifying its accuracy, where let the contact radius approach zero for approximating to Green's function of coated structure. The analytical solution given in this paper is expressed as elemental functions, which is convenient for further application. It can used as a benchmark for other solution for indentation contact problem of coating structure.

Appendix A

1.1 The basic notations

The following equations are introduced to simplify notations:

$$\begin{aligned} z_{j} \,=\, & s_{j} z,\;z_{j}^{\prime } \,=\, s_{j}^{\prime } z,\;h_{j}^{\prime } \,=\, s_{j}^{\prime } h,\; \\ \overline{z}_{njk} \,=\, & z_{j} - h_{nk} ,\;\overline{R}_{njk} \,=\, \sqrt {r^{2} + r_{0}^{2} - 2rr_{0} \cos \left( {\varphi - \varphi_{0} } \right) + \overline{z}_{njk}^{2} } , \\ z_{njk} \,=\, & z_{j} - h_{nk} ,\;R_{njk} \,=\, \sqrt {r^{2} + r_{0}^{2} - 2rr_{0} \cos \left( {\varphi - \varphi_{0} } \right) + z_{njk}^{2} } \\ z_{njk}^{\prime } \,=\, & z_{i}^{\prime } - h_{nk} ,\;R^{\prime}_{njk} \,=\, \sqrt {r^{2} + r_{0}^{2} - 2rr_{0} \cos \left( {\varphi - \varphi_{0} } \right) + z_{njk}^{\prime 2} } \\ \overline{R}_{njk}^{*} \,=\, & \overline{R}_{njk} - \overline{z}_{njk} ,\;R_{njk}^{*} \,=\, R_{njk} - z_{njk} ,\;\overline{R}_{njk}^{\prime *} \,=\, \overline{R}_{njk}^{\prime } - z_{njk}^{\prime } \\ l_{1njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + z_{njk}^{2} } - \sqrt {(r - a)^{2} + z_{njk}^{2} } } \right],\; \\ l_{2njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + z_{njk}^{2} } + \sqrt {(r - a)^{2} + z_{njk}^{2} } } \right],\; \\ \overline{l}_{1njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + \overline{z}_{njk}^{2} } - \sqrt {(r - a)^{2} + \overline{z}_{njk}^{2} } } \right],\; \\ \overline{l}_{2njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + \overline{z}_{njk}^{2} } + \sqrt {(r - a)^{2} + \overline{z}_{njk}^{2} } } \right],\; \\ l^{\prime}_{1njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + z_{njk}^{\prime 2} } - \sqrt {(r - a)^{2} + z_{njk}^{\prime 2} } } \right]{\kern 1pt} ,\; \\ l^{\prime}_{2njk} \,=\, & \frac{1}{2}\left[ {\sqrt {(r + a)^{2} + z_{njk}^{\prime 2} } + \sqrt {(r - a)^{2} + z_{njk}^{\prime 2} } } \right]{\kern 1pt} \\ \end{aligned}$$

(43a)

Equivalent relationships of $z_{njk}$, $\overline{z}_{njk}$, and $z^{\prime}_{njk}$ when $z = h$

$$\begin{aligned} \overline{z}_{(n + 1)21} (h) = & h_{2} - (2n + 1)h_{1} ,\; \\ \overline{z}_{(n + 1)1(2n + 2)} (h) = & h_{1} - (2n + 1)h_{2} ,\; \\ z_{n11} (h) = & - \overline{z}_{(n + 1)11} (h) = - \overline{z}_{(n + 1)22} (h) = 2nh_{1} ,\; \\ z_{n2(2n)} (h) = & - \overline{z}_{(n + 1)1(2n + 1)} (h) = - \overline{z}_{(n + 1)2(2n + 2)} (h) = 2nh_{2} \\ z_{n1(m + 1)} (h) = & z_{n2m} (h) = - \overline{z}_{(n + 1)1(m + 1)} (h) = - \overline{z}_{(n + 1)2(m + 2)} (h) = (2n - m)h_{1} + mh_{2} \\ (\;n = & 1,2,\; \cdots ,\infty \;;\;m = 1,2, \cdots 2n - 1) \\ \end{aligned}$$

(43b)

$$\overline{z}_{njk} \left( 0 \right) = - z_{njk} \left( 0 \right) = z^{\prime}_{njk} \left( 0 \right) = - h_{nk} ,\,({\kern 1pt} {\kern 1pt} {\kern 1pt} n = 1,2, \cdots \;,\infty {\kern 1pt} ;{\kern 1pt} \;j = 1,2;\;{\kern 1pt} k = 1,2{\kern 1pt} ,{\kern 1pt} {\kern 1pt} \cdots \;,2n).$$

(43c)

1.2 The expressions for the solutions (21)(22)

The expressions $f_{1i}$, $\overline{f}_{1i}$, $f^{\prime}_{1i}$ $\left( {i = 1,2,3,4,5} \right)$:

$$\begin{aligned} f_{11} \left( {z_{njk} } \right) & = \frac{{2\pi e^{i\varphi } }}{r}\left( {a - \sqrt {a^{2} - l_{1njk}^{2} } } \right), \\ \overline{f}_{11} \left( {\overline{z}_{njk} } \right) & = \frac{{2\pi e^{i\varphi } }}{r}\left( {a - \sqrt {a^{2} - \overline{l}_{1njk}^{2} } } \right), \\ f_{11}^{\prime } \left( {z_{njk} } \right) & = \frac{{2\pi e^{i\varphi } }}{r}\left( {a - \sqrt {a^{2} - l_{1njk}^{\prime 2} } } \right), \\ f_{12} \left( {z_{njk} } \right) & = 2\pi \sin^{ - 1} \frac{a}{{l_{2njk} }}, \\ \overline{f}_{12} \left( {\overline{z}_{njk} } \right) & = - 2\pi \sin^{ - 1} \frac{a}{{\overline{l}_{2njk} }}, \\ f_{12}^{\prime } \left( {z_{njk} } \right) & = - 2\pi \sin^{ - 1} \frac{a}{{l_{2njk}^{\prime } }}, \\ f_{13} \left( {z_{njk} } \right) & = - 2\pi \;\frac{{\sqrt {a^{2} - l_{1njk}^{2} } }}{{l_{2njk}^{2} - l_{1njk}^{2} }}, \\ \overline{f}_{13} \left( {\overline{z}_{njk} } \right) & = - 2\pi \;\frac{{\sqrt {a^{2} - \overline{l}_{1njk}^{2} } }}{{\overline{l}_{2njk}^{2} - \overline{l}_{1njk}^{2} }}, \\ f_{13}^{\prime } \left( {z_{njk}^{\prime } } \right) & = - 2\pi \;\frac{{\sqrt {a^{2} - l_{1njk}^{\prime 2} } }}{{l_{2njk}^{\prime 2} - l_{1njk}^{\prime 2} }}, \\ f_{14} \left( {z_{njk} } \right) & = - 2\pi \;e^{i\varphi } \frac{{l_{1njk} \sqrt {l_{2njk}^{2} - a^{2} } }}{{l_{2njk} \left( {l_{2njk}^{2} - l_{1njk}^{2} } \right)}}, \\ \overline{f}_{14} \left( {\overline{z}_{njk} } \right) & = 2\pi \;e^{i\varphi } \frac{{\overline{l}_{1njk} \sqrt {\overline{l}_{2njk}^{2} - a^{2} } }}{{\overline{l}_{2njk} \left( {\overline{l}_{2njk}^{2} - \overline{l}_{1njk}^{2} } \right)}}, \\ f_{14}^{\prime } \left( {z_{njk}^{\prime } } \right) & = 2\pi \;e^{i\varphi } \frac{{l_{1njk}^{\prime } \sqrt {l_{2njk}^{\prime 2} - a^{2} } }}{{l_{2njk}^{\prime } \left( {l_{2njk}^{\prime 2} - l_{1njk}^{\prime 2} } \right)}}, \\ f_{15} \left( {z_{njk} } \right) & = 2\pi \;e^{2i\varphi } \left[ {\frac{{\sqrt {a^{2} - l_{1njk}^{2} } }}{{l_{2njk}^{2} - l_{1njk}^{2} }} - \frac{2a}{{r^{2} }}\left( {1 - \frac{{\sqrt {a^{2} - l_{1njk}^{2} } }}{a}} \right)} \right], \\ \overline{f}_{15} \left( {\overline{z}_{njk} } \right) & = 2\pi \;e^{2i\varphi } \left[ {\frac{{\sqrt {a^{2} - \overline{l}_{1njk}^{2} } }}{{\overline{l}_{2njk}^{2} - \overline{l}_{1njk}^{2} }} - \frac{2a}{{r^{2} }}\left( {1 - \frac{{\sqrt {a^{2} - \overline{l}_{1njk}^{2} } }}{a}} \right)} \right], \\ f_{15}^{\prime } \left( {z_{njk}^{\prime } } \right) & = 2\pi \;e^{2i\varphi } \left[ {\frac{{\sqrt {a^{2} - l_{1njk}^{\prime 2} } }}{{l_{2njk}^{\prime 2} - l_{1njk}^{\prime 2} }} - \frac{2a}{{r^{2} }}\left( {1 - \frac{{\sqrt {a^{2} - l_{1njk}^{\prime 2} } }}{a}} \right)} \right]. \\ \end{aligned}$$

(44a)

The expressions $f_{2i}$, $\overline{f}_{2i}$, $f^{\prime}_{2i}$$\left( {i = 1,2,3,4,5} \right)$:

$$\begin{aligned} f_{21} \left( {z_{njk} } \right) = & \pi e^{i\varphi } \left[ {r\ln \left( {l_{2njk} + \sqrt {l_{2njk}^{2} - r^{2} } } \right) - r\ln \left( {z_{njk} + \sqrt {z_{njk}^{2} + r^{2} } } \right)} \right] \\ & \quad + \frac{{\left( {a^{2} - z_{njk} \sqrt {r^{2} + z_{njk}^{2} } } \right)}}{r} + \frac{{\left( {rl_{2njk} - 2al_{1njk} } \right)\sqrt {l_{2njk}^{2} - r^{2} } }}{{r^{2} }}, \\ \overline{f}_{21} \left( {\overline{z}_{njk} } \right) = & \pi e^{i\varphi } \left[ {r\ln \left( {\overline{l}_{2njk} + \sqrt {\overline{l}_{2njk}^{2} - r^{2} } } \right) - r\ln \left( { - \overline{z}_{njk} + \sqrt {\overline{z}_{njk}^{2} + r^{2} } } \right)} \right] \\ & \quad + \frac{{\left( {a^{2} + \overline{z}_{njk} \sqrt {r^{2} + \overline{z}_{njk}^{2} } } \right)}}{r} + \frac{{\left( {r\overline{l}_{2njk} - 2a\overline{l}_{1njk} } \right)\sqrt {\overline{l}_{2njk}^{2} - r^{2} } }}{{r^{2} }}, \\ f^{\prime}_{21} \left( {z^{\prime}_{njk} } \right) = & \pi e^{i\varphi } \left[ {r\ln \left( {l^{\prime}_{2njk} + \sqrt {l_{2njk}^{\prime 2} - r^{2} } } \right) - r\ln \left( { - z^{\prime}_{njk} + \sqrt {z_{njk}^{\prime 2} + r^{2} } } \right)} \right] \\ & \quad + \frac{{\left( {a^{2} + z^{\prime}_{njk} \sqrt {r^{2} + z_{njk}^{\prime 2} } } \right)}}{r} + \frac{{\left( {rl^{\prime}_{2njk} - 2al^{\prime}_{1njk} } \right)\sqrt {l_{2njk}^{\prime 2} - r^{2} } }}{{r^{2} }}, \\ f_{22} \left( {z_{njk} } \right) = & 2\pi \left[ {a\sin^{ - 1} \frac{{l_{1njk} }}{r} + \sqrt {l_{2njk}^{2} - a^{2} } - z_{njk} \ln \left( {l_{2njk} + \sqrt {l_{2njk}^{2} - r^{2} } } \right)} \right. \\ & \quad \left. { - \sqrt {r^{2} + z_{njk}^{2} } + z_{njk} \ln \left( {z_{njk} + \sqrt {z_{njk}^{2} + r^{2} } } \right)} \right], \\ \overline{f}_{22} \left( {\overline{z}_{njk} } \right) = & - 2\pi \left[ {a\sin^{ - 1} \frac{{\overline{l}_{1njk} }}{r} + \sqrt {\overline{l}_{2njk}^{2} - a^{2} } + \overline{z}_{njk} \ln \left( {\overline{l}_{2njk} + \sqrt {\overline{l}_{2njk}^{2} - r^{2} } } \right)} \right. \\ & \quad \left. { - \sqrt {r^{2} + \overline{z}_{njk}^{2} } - \overline{z}_{njk} \ln \left( { - \overline{z}_{njk} + \sqrt {\overline{z}_{njk}^{2} + r^{2} } } \right)} \right] \\ f^{\prime}_{22} \left( {z^{\prime}_{njk} } \right) = & - 2\pi \left[ {a\sin^{ - 1} \frac{{l^{\prime}_{1njk} }}{r} + \sqrt {l_{2njk}^{\prime 2} - a^{2} } + z^{\prime}_{njk} \ln \left( {l_{2njk}^{\prime } + \sqrt {l_{2njk}^{\prime 2} - r^{2} } } \right)} \right. \\ & \quad \left. { - \sqrt {r^{2} + z_{njk}^{\prime 2} } - z^{\prime}_{njk} \ln \left( { - z^{\prime}_{njk} + \sqrt {z_{njk}^{\prime 2} + r^{2} } } \right)} \right] \\ f_{23} \left( {z_{njk} } \right) = & - 2\pi \left[ {\ln \left( {l_{2njk} + \sqrt {l_{2njk}^{2} - r^{2} } } \right) - \ln \left( {z_{njk} + \sqrt {z_{njk}^{2} + r^{2} } } \right)} \right], \\ \overline{f}_{23} \left( {\overline{z}_{njk} } \right) = & - 2\pi \left[ {\ln \left( {\overline{l}_{2njk} + \sqrt {\overline{l}_{2njk}^{2} - r^{2} } } \right) - \ln \left( { - \overline{z}_{njk} + \sqrt {\overline{z}_{njk}^{2} + r^{2} } } \right)} \right], \\ f^{\prime}_{23} \left( {z^{\prime}_{njk} } \right) = & - 2\pi \left[ {\ln \left( {l_{2njk}^{\prime } + \sqrt {l_{2njk}^{\prime 2} - r^{2} } } \right) - \ln \left( { - z^{\prime}_{njk} + \sqrt {z_{njk}^{\prime 2} + r^{2} } } \right)} \right], \\ f_{24} \left( {z_{njk} } \right) = & 2\pi e^{i\varphi } \frac{1}{r}\left[ {\sqrt {l_{2njk}^{2} - a^{2} } - \sqrt {r^{2} + z_{njk}^{2} } } \right], \\ \overline{f}_{24} \left( {\overline{z}_{njk} } \right) = & - 2\pi e^{i\varphi } \frac{1}{r}\left[ {\sqrt {\overline{l}_{2njk}^{2} - a^{2} } - \sqrt {r^{2} + \overline{z}_{njk}^{2} } } \right], \\ f^{\prime}_{24} \left( {z_{njk} } \right) = & - 2\pi e^{i\varphi } \frac{1}{r}\left[ {\sqrt {l_{2njk}^{\prime 2} - a^{2} } - \sqrt {r^{2} + z_{njk}^{\prime 2} } } \right], \\ f_{25} \left( {z_{njk} } \right) = & 2\pi e^{2i\varphi } \left[ {\frac{{\left( {2a^{2} - l_{2njk}^{2} } \right)\sqrt {a^{2} - l_{1njk}^{2} } }}{{ar^{2} }} + \frac{{z_{njk} \sqrt {r^{2} + z_{njk}^{2} } - a^{2} }}{{r^{2} }}} \right], \\ \overline{f}_{25} \left( {\overline{z}_{njk} } \right) = & 2\pi e^{2i\varphi } \left[ {\frac{{\left( {2a^{2} - \overline{l}_{2njk}^{2} } \right)\sqrt {a^{2} - \overline{l}_{1njk}^{2} } }}{{ar^{2} }} - \frac{{\overline{z}_{njk} \sqrt {r^{2} + \overline{z}_{njk}^{2} } - a^{2} }}{{r^{2} }}} \right], \\ f^{\prime}_{25} \left( {z^{\prime}_{njk} } \right) = & 2\pi e^{2i\varphi } \left[ {\frac{{\left( {2a^{2} - l_{2njk}^{\prime 2} } \right)\sqrt {a^{2} - l_{1njk}^{\prime 2} } }}{{ar^{2} }} - \frac{{z^{\prime}_{njk} \sqrt {r^{2} + z_{njk}^{\prime 2} } - a^{2} }}{{r^{2} }}} \right]. \\ \end{aligned}$$

(44b)

The expressions $f_{3i}$, $\overline{f}_{3i}$, $f^{\prime}_{3i}$$\left( {i = 1,2,3,4,5} \right)$:

$$\begin{aligned} f_{31} \left( {z_{njk} } \right) & = \pi re^{i\varphi } {\kern 1pt} \left[ { - z_{njk} \sin^{ - 1} \left( {\frac{{l_{1njk} }}{r}} \right) + \sqrt {a^{2} - l_{1njk}^{2} } \left( {1 - \frac{{l_{1njk}^{2} + 2a^{2} }}{{3r^{2} }}} \right) + \frac{{2a^{3} }}{{3r^{2} }}} \right], \\ \overline{f}_{31} \left( {\overline{z}_{njk} } \right) & = \pi re^{i\varphi } \left[ {\overline{z}_{njk} \sin^{ - 1} \left( {\frac{{\overline{l}_{1njk} }}{r}} \right) + \sqrt {a^{2} - \overline{l}_{1njk}^{2} } \left( {1 - \frac{{\overline{l}_{1njk}^{2} + 2a^{2} }}{{3r^{2} }}} \right) + \frac{{2a^{3} }}{{3r^{2} }}} \right], \\ f^{\prime}_{31} \left( {z^{\prime}_{njk} } \right) & = \pi re^{i\varphi } \left[ {z^{\prime}_{njk} \sin^{ - 1} \left( {\frac{{l^{\prime}_{1njk} }}{r}} \right) + \sqrt {a^{2} - l_{1njk}^{\prime 2} } \left( {1 - \frac{{l_{1njk}^{\prime 2} + 2a^{2} }}{{3r^{2} }}} \right) + \frac{{2a^{3} }}{{3r^{2} }}} \right], \\ f_{32} \left( {z_{njk} } \right) & = \frac{\pi }{2}\left[ {\left( {2a^{2} + 2z_{njk}^{2} - r^{2} } \right)\sin^{ - 1} \left( {\frac{{l_{1njk} }}{r}} \right) + \frac{{3l_{1njk}^{2} - 2a^{2} }}{a}\sqrt {l_{2njk}^{2} - a^{2} } } \right], \\ \overline{f}_{32} \left( {\overline{z}_{njk} } \right) & = - \frac{\pi }{2}\left[ {\left( {2a^{2} + 2\overline{z}_{njk}^{2} - r^{2} } \right)\sin^{ - 1} \left( {\frac{{\overline{l}_{1njk} }}{r}} \right) + \frac{{3\overline{l}_{1njk}^{2} - 2a^{2} }}{a}\sqrt {\overline{l}_{2njk}^{2} - a^{2} } } \right], \\ f^{\prime}_{32} \left( {z^{\prime}_{njk} } \right) & = - \frac{\pi }{2}\left[ {\left( {2a^{2} + 2z_{njk}^{\prime 2} - r^{2} } \right)\sin^{ - 1} \left( {\frac{{l^{\prime}_{1njk} }}{r}} \right) + \frac{{3l_{1njk}^{\prime 2} - 2a^{2} }}{a}\sqrt {l_{2njk}^{\prime 2} - a^{2} } } \right], \\ f_{33} \left( {z_{njk} } \right) & = 2\pi \left[ {z_{njk} \sin^{ - 1} \left( {\frac{{l_{1njk} }}{r}} \right) - \sqrt {a^{2} - l_{1njk}^{2} } } \right], \\ \overline{f}_{33} \left( {\overline{z}_{njk} } \right) & = 2\pi \left[ { - \overline{z}_{njk} \sin^{ - 1} \left( {\frac{{\overline{l}_{1njk} }}{r}} \right) - \sqrt {a^{2} - \overline{l}_{1njk}^{2} } } \right], \\ f^{\prime}_{33} \left( {z^{\prime}_{njk} } \right) & = 2\pi \left[ { - z^{\prime}_{njk} \sin^{ - 1} \left( {\frac{{l^{\prime}_{1njk} }}{r}} \right) - \sqrt {a^{2} - l_{1njk}^{\prime 2} } } \right], \\ f_{34} \left( {z_{njk} } \right) & = \pi re^{i\varphi } \left[ { - \sin^{ - 1} \left( {\frac{{l_{1njk} }}{r}} \right) + \frac{{a\sqrt {l_{2njk}^{2} - a^{2} } }}{{l_{2njk}^{2} }}} \right], \\ \overline{f}_{34} \left( {\overline{z}_{njk} } \right) & = - \pi re^{i\varphi } \left[ { - \sin^{ - 1} \left( {\frac{{\overline{l}_{1njk} }}{r}} \right) + \frac{{a\sqrt {\overline{l}_{2njk}^{2} - a^{2} } }}{{\overline{l}_{2njk}^{2} }}} \right], \\ f^{\prime}_{34} \left( {z^{\prime}_{njk} } \right) & = - \pi re^{i\varphi } \left[ { - \sin^{ - 1} \left( {\frac{{l^{\prime}_{1njk} }}{r}} \right) + \frac{{a\sqrt {l_{2njk}^{\prime 2} - a^{2} } }}{{l_{2njk}^{\prime 2} }}} \right], \\ f_{35} \left( {z_{njk} } \right) & = - 2\pi e^{2i\varphi } \frac{{2a^{3} - \left( {l_{1njk}^{2} + 2a^{2} } \right)\sqrt {a^{2} - l_{1njk}^{2} } }}{{r^{2} }}, \\ \overline{f}_{35} \left( {\overline{z}_{njk} } \right) & = - 2\pi e^{2i\varphi } \frac{{2a^{3} - \left( {\overline{l}_{1njk}^{2} + 2a^{2} } \right)\sqrt {a^{2} - \overline{l}_{1njk}^{2} } }}{{r^{2} }}, \\ f^{\prime}_{35} \left( {z^{\prime}_{njk} } \right) & = - 2\pi e^{2i\varphi } \frac{{2a^{3} - \left( {l_{1njk}^{\prime 2} + 2a^{2} } \right)\sqrt {a^{2} - l_{1njk}^{\prime 2} } }}{{r^{2} }}{\kern 1pt} \\ \end{aligned}$$

(44c)

1.3 General solution

In the cylinder coordinates $(r,\varphi ,z)$, when the plane $rO\varphi$ is parallel to the isotropic plane, the constitutive equations of the three-dimensional transversely isotropic material are

$$\begin{aligned} \sigma_{r} & = c_{11} \frac{{\partial u_{r} }}{\partial r} + c_{12} \left( {\frac{{u_{r} }}{r} + \frac{1}{r}\frac{{\partial u_{\varphi } }}{\partial \varphi }} \right) + c_{13} \frac{{\partial u_{z} }}{\partial z}{\kern 1pt} ,\;\tau_{r\varphi } = c_{66} \left( {\frac{1}{r}\frac{{\partial u_{r} }}{\partial \varphi } + \frac{{\partial u_{\varphi } }}{\partial r} - \frac{{u_{\varphi } }}{r}} \right){\kern 1pt} \\ \sigma_{\varphi } & = c_{12} \frac{{\partial u_{r} }}{\partial r} + c_{11} \left( {\frac{{u_{r} }}{r} + \frac{1}{r}\frac{{\partial u_{\varphi } }}{\partial \varphi }} \right) + c_{13} \frac{{\partial u_{z} }}{\partial z}{\kern 1pt} ,\;\tau_{\varphi z} = c_{44} \left( {\frac{{\partial u_{\varphi } }}{\partial z} + \frac{1}{r}\frac{{\partial u_{z} }}{\partial \varphi }} \right){\kern 1pt} \\ \sigma_{z} & = c_{13} \left( {\frac{{\partial u_{r} }}{\partial r} + \frac{{u_{r} }}{r} + \frac{1}{r}\frac{{\partial u_{\varphi } }}{\partial \phi }} \right) + c_{33} \frac{{\partial u_{z} }}{\partial z}{\kern 1pt} ,\;\tau_{zr} = c_{44} \left( {\frac{{\partial u_{z} }}{\partial r} + \frac{{\partial u_{r} }}{\partial z}} \right){\kern 1pt} \\ \end{aligned}$$

(45a)

Regardless of body forces, the mechanical equilibrium differential equations are

$$\begin{gathered} \frac{{\partial \sigma_{r} }}{\partial r} + \frac{1}{r}\frac{{\partial \tau_{r\varphi } }}{\partial \varphi } + \frac{{\partial \tau_{rz} }}{\partial z} + \frac{{\sigma_{r} - \sigma_{\varphi } }}{r} = 0,\; \hfill \\ \frac{{\partial \tau_{r\varphi } }}{\partial r} + \frac{1}{r}\frac{{\partial \sigma_{\varphi } }}{\partial \phi } + \frac{{\partial \tau_{\varphi z} }}{\partial z} + \frac{{2\tau_{r\varphi } }}{r} = 0{\kern 1pt} , \hfill \\ \frac{{\partial \tau_{rz} }}{\partial r} + \frac{1}{r}\frac{{\partial \tau_{\varphi z} }}{\partial \varphi } + \frac{{\partial \sigma_{z} }}{\partial z} + \frac{{\tau_{rz} }}{r} = 0{\kern 1pt} \hfill \\ \end{gathered}$$

(45b)

where $u_{m} {\kern 1pt}$ and $\sigma_{m} {\kern 1pt}$$(\tau_{mn} {\kern 1pt} )$$(m,n{\kern 1pt} = r,\varphi ,z)$ represent the components of the mechanical displacements and stresses, respectively; $c_{ij} {\kern 1pt}$$(i,j = 1,2, \cdots ,6){\kern 1pt}$ are the elastic modulus. For transversely isotropic materials, there is $c_{66} = (c_{11} - c_{12} )/2{\kern 1pt}$.

The following symbols (combinatorial components) for the notational convenience are presented:

$$\begin{aligned} \;U & = u + iv = e^{i\varphi } (u_{r} + iu_{\varphi } ){\kern 1pt} ,\;\sigma_{1} \\ & = \sigma_{x} + \sigma_{y} = \sigma_{r} + \sigma_{\varphi } {\kern 1pt} ,\;\sigma_{2} = \sigma_{x} - \sigma_{y} + 2i\tau_{xy} \\ & = e^{2i\varphi } (\sigma_{r} - \sigma_{\varphi } + 2i\tau_{r\varphi } ){\kern 1pt} ,\;\tau_{z} \\ & = \tau_{xz} + i\tau_{yz} = e^{i\varphi } (\tau_{rz} + i\tau_{\varphi z} ){\kern 1pt} \\ \end{aligned}$$

(45c)

where the following compact general solutions ( [56], 2005)

$$\begin{aligned} U & = \Delta \left( {i\psi_{0} + \sum\limits_{j = 1}^{2} {\psi_{j} } } \right),\;u_{z} = \sum\limits_{j = 1}^{2} {s_{j} k_{j} \frac{{\partial \psi_{j} }}{{\partial z_{j} }}} \\ \sigma_{1} & = 2\sum\limits_{j = 1}^{2} {\left( {m_{j} - c_{66} } \right)\frac{{\partial^{2} \psi_{j} }}{{\partial z_{j}^{2} }}} = - 2\sum\limits_{j = 1}^{2} {\left( {m_{j} - c_{66} } \right)\Lambda \psi_{j} } \,, \\ \sigma_{2} & = 2c_{66} \Delta^{2} \left( {i\psi_{0} + \sum\limits_{j = 1}^{2} {\psi_{j} } } \right){\kern 1pt} ,\;\sigma_{z} = \sum\limits_{j = 1}^{2} {\omega_{j} \frac{{\partial^{2} \psi_{j} }}{{\partial z_{j}^{2} }}} = - \sum\limits_{j = 1}^{2} {\omega_{j} \Lambda \psi_{j} } ,\;{\kern 1pt} \\ \tau_{z} & = \Delta \left( {s_{0} c_{44} i\frac{{\partial \psi_{0} }}{{\partial z_{0} }} + \sum\limits_{j = 1}^{2} {s_{j} \omega_{j} \frac{{\partial \psi_{j} }}{{\partial z_{j} }}} } \right) \\ \end{aligned}$$

(45d)

where

$$\begin{aligned} k_{j} & = \frac{{c_{11} - c_{44} s_{j}^{2} }}{{(c_{13} + c_{44} )s_{j}^{2} }}{\kern 1pt} ,\;\omega_{j} = c_{33} s_{j}^{2} k_{j} - c_{13} {\kern 1pt} ,\;m_{j} = 2c_{66} - \omega_{1j} s_{j}^{2} {\kern 1pt} \\ \Delta & = \partial /\partial x + i\partial /\partial y{\kern 1pt} ,\;\Delta^{2} = \partial^{2} /\partial x^{2} - \partial^{2} /\partial y^{2} + 2i\partial^{2} /\partial x\partial y{\kern 1pt} \\ \end{aligned}$$

(45e)

$\psi_{j}$ satisfies the following harmonic equation

$$\left( {\Lambda + \frac{{\partial^{2} }}{{\partial z_{j}^{2} }}} \right)\psi_{j} = 0,\;\left( {j = 0,1,2} \right)$$

(45f)

and

$$\begin{aligned} \Lambda & = \partial^{2} /\partial x^{2} + \partial^{2} /\partial y^{2} = \partial^{2} /\partial r^{2} + \partial /(r\partial r) + \partial^{2} /(r^{2} \partial \varphi^{2} ){\kern 1pt} ,\; \\ z_{j} & = s_{j} z{\kern 1pt} ,\;(j = 0,1,2){\kern 1pt} ,\;s_{0} = \sqrt {c_{66} /c_{44} } {\kern 1pt} \\ \end{aligned}$$

(45g)

where the eigenvalues $s_{j} \left( {j = 1,2} \right){\kern 1pt}$ should be satisfied with ${\text{Re}} \;(s_{j} ) > 0{\kern 1pt}$ and the following equation:

$$as^{4} - bs^{2} + c = 0{\kern 1pt} ,$$

(45h)

and

$$a = c_{33} c_{44} {\kern 1pt} ,\;b = c_{11} c_{33} + c_{44}^{2} - \left( {c_{13} + c_{44} } \right)^{2} {\kern 1pt} ,\;c = c_{11} c_{44} {\kern 1pt} .$$

(45i)

1.4 Definition of the Notations

See Table

Table 4 Notations

4.