Ginzburg-Landau Analysis of the Critical Temperature and the Upper Critical Field for Three-Band Superconductors

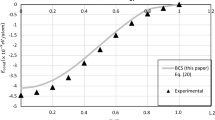
Abstract

Using the microscopic formalism of Eilenberger equations, a three-band Ginzburg-Landau theory for the intraband dirty limit and clean interband scattering case is derived. Within the framework of this three-band Ginzburg-Landau theory, expressions for the critical temperature T _c and the temperature dependence of the upper critical field H _c2 are obtained. Based on some special cases of the matrix of interaction constants, we demonstrate the influence of the sign of the interband interaction on the critical temperature and the upper critical field as compared with a two-band superconductor where it plays no role. We study also analytically and numerically the effect of its magnitude.

Appendices

Appendix A: Derivation of Ginzburg-Landau Equations

For the anomalous Green functions f _i we get:

$$ f_{i} = \frac{D_{i}}{2\omega^{2}}\nabla^{2}\varDelta _{_{i}} - \frac{\vert \varDelta _{i} \vert ^{2}\varDelta _{i}}{2\omega^{3}} + \frac{\varDelta _{i}}{\omega}. $$

(A.1)

Substituting f _i into the self-consistency Eqs. (4) after the summation over the Matsubara frequencies we have finally

$$ \left\{ \begin{array}{ll} \varDelta _{1}N_{1} &= \lambda_{11}N_{1} \bigl[ \frac{\pi D_{1}}{8T_{c}}\varPi^{2}\varDelta _{1} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{1} \vert ^{2}\varDelta _{1} + l\varDelta _{1} \bigr]\\ &\quad + \lambda_{12}N_{2} \bigl[ \frac{\pi D_{2}}{8T_{c}}\varPi^{2}\varDelta _{2} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{2} \vert ^{2}\varDelta _{2} + l\varDelta _{2} \bigr]\\ &\quad + \lambda_{13}N_{3} \bigl[ \frac{\pi D_{3}}{8T_{c}}\varPi^{2}\varDelta _{3} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{3} \vert ^{2}\varDelta _{3} + l\varDelta _{3} \bigr], \\ \varDelta _{2}N_{2} &= \lambda_{21}N_{1} \bigl[ \frac{\pi D_{1}}{8T_{c}}\varPi^{2}\varDelta _{1} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{1} \vert ^{2}\varDelta _{1} + l\varDelta _{1} \bigr] \\ &\quad + \lambda_{22}N_{2} \bigl[ \frac{\pi D_{2}}{8T_{c}}\varPi^{2}\varDelta _{2} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{2} \vert ^{2}\varDelta _{2} + l\varDelta _{2} \bigr] \\ &\quad + \lambda_{23}N_{3} \bigl[ \frac{\pi D_{3}}{8T_{c}}\varPi^{2}\varDelta _{3} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{3} \vert ^{2}\varDelta _{3} + l\varDelta _{3} \bigr], \\ \varDelta _{3}N_{3} &= \lambda_{31}N_{1} \bigl[ \frac{\pi D_{1}}{8T_{c}}\varPi^{2}\varDelta _{1} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{1} \vert ^{2}\varDelta _{1} + l\varDelta _{1} \bigr]\\ &\quad + \lambda_{32}N_{2} \bigl[ \frac{\pi D_{2}}{8T_{c}}\varPi^{2}\varDelta _{2} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{2} \vert ^{2}\varDelta _{2} + l\varDelta _{2} \bigr]\\ &\quad + \lambda_{33}N_{3} \bigl[ \frac{\pi D_{3}}{8T_{c}}\varPi^{2}\varDelta _{3} - \frac{7\zeta ( 3 )}{8\pi^{2}T_{c}^{2}}\vert \varDelta _{3} \vert ^{2}\varDelta _{3} + l\varDelta _{3} \bigr]. \end{array} \right. $$

(A.2)

In order to obtain the GL equations, we multiply the first equation by λ ₂₂ λ ₃₃−λ ₂₃ λ ₃₂, the second equation by −(λ ₁₂ λ ₃₃−λ ₁₃ λ ₃₂) and the third equation by λ ₁₂ λ ₂₃−λ ₁₃ λ ₂₂. Then we sum these three expressions over Matsubara frequencies and finally obtain the GL equations (6) for Δ ₁, Δ ₂, and Δ ₃.

Appendix B: The Determination of the Critical Temperature

T _c can be found from the linearization of the GL system (6):

$$ \left\{ \begin{array}{l} \alpha_{1}\varDelta _{1} + \gamma_{12}\varDelta _{2} + \gamma_{13}\varDelta _{3} = 0, \\ \alpha_{2}\varDelta _{2} + \gamma_{21}\varDelta _{1} + \gamma_{23}\varDelta _{3} = 0, \\ \alpha_{3}\varDelta _{3} + \gamma_{31}\varDelta _{1} + \gamma_{32}\varDelta _{2} = 0. \end{array} \right. $$

(B.1)

which leads to the cubic equation:

$$ \alpha_{1}\alpha_{2}\alpha_{3} - \gamma_{32}\gamma_{23}\alpha_{1} - \gamma_{31}\gamma_{13}\alpha_{2} - \gamma_{12}\gamma_{21}\alpha_{3} + \gamma_{12}\gamma_{31}\gamma_{23} + \gamma_{13}\gamma_{21}\gamma_{32} = 0, $$

(B.2)

or using the phenomenological constants γ _ij introduced in the main paper:

$$\begin{aligned} &( l - a_{1} ) ( l - a_{2} ) ( l - a_{3} ) - \tilde{\gamma}_{32}\tilde{\gamma}_{23} ( l - a_{1} ) - \tilde{\gamma}_{31}\tilde{\gamma}_{13} ( l - a_{2} ) - \tilde{\gamma}_{12}\tilde{\gamma}_{21} ( l - a_{3} ) \\ &\quad + \tilde{\gamma}_{12}\tilde{\gamma}_{31} \tilde{\gamma}_{23} + \tilde{\gamma}_{13}\tilde{ \gamma}_{21}\tilde{\gamma}_{32} = 0. \end{aligned}$$

(B.3)

Equation (B.3) can be rewritten as:

$$ \begin{aligned}[b] &l^{3} - ( a_{1} + a_{2} + a_{3} )l^{2} + ( a_{1}a_{2} + a_{1}a_{3} + a_{2}a_{3} - \tilde{\gamma}_{12}\tilde{\gamma}_{21} - \tilde{\gamma}_{31}\tilde{\gamma}_{13} - \tilde{\gamma}_{32}\tilde{\gamma}_{23} )l\\ &\quad + \tilde{\gamma}_{32}\tilde{\gamma}_{23}a_{1} + \tilde{\gamma}_{31}\tilde{\gamma}_{13}a_{2} + \tilde{\gamma}_{12}\tilde{\gamma}_{21}a_{3} + \tilde{\gamma}_{12}\tilde{\gamma}_{31}\tilde{\gamma}_{23} + \tilde{\gamma}_{13}\tilde{\gamma}_{21}\tilde{\gamma}_{32} = 0. \end{aligned} $$

(B.4)

Comparing Eq. (B.3) with the general form of a cubic equation and bearing in mind the representation of the coefficients a _i and γ _ij , we get

$$ l^{3} + Bl^{2} + Cl + D = 0, $$

(B.5)

where

$$\begin{aligned} B &\equiv - \frac{1}{\det ( \lambda )}\sum_{i} M_{ii}, \\ C &\equiv \frac{M_{11}M_{22} + M_{11}M_{33} + M_{22}M_{33} - M_{12}M_{21} - M_{13}M_{31} - M_{23}M_{32}}{\det^{2} ( \lambda )}, \\ D &\equiv \frac{M_{11}M_{23}M_{32} + M_{13}M_{22}M_{31} + M_{12}M_{21}M_{33} - M_{13}M_{21}M_{32} - M_{12}M_{23}M_{31} - M_{11}M_{22}M_{33}}{\det^{3} ( \lambda )}. \end{aligned}$$

Depending on the sign of the discriminant Z=18BCD−4B ³ D+B ² C ²−4C ³−27D ² we have three distinct real roots, if Z>0, one real root and two complex conjugate roots, if Z<0 and three real roots, if Z=0.

If we expand the coefficients B, C and D in terms of the coupling constants λ _ij and simplify the obtained expressions, we reveal that Eq. (B.5) for determination of the critical temperature is equivalent to the secular equation for the coupling matrix (7).

Appendix C: The Curvature of the Upper Critical Field

In the case (i) the upper critical field is determined by the equation:

$$ A^{ ( h )}h_{c2}^{3} + B^{ ( h )}h_{c2}^{2} + C^{ ( h )}h_{c2} + D^{ ( h )} = 0, $$

(C.1)

where

$$\begin{aligned} A^{ ( h )} &= - d_{12}d_{13}, \end{aligned}$$

(C.2)

$$\begin{aligned} B^{ ( h )} &= ( d_{12} + d_{13} + d_{12}d_{13} ) ( \eta - a - t ), \end{aligned}$$

(C.3)

$$\begin{aligned} C^{ ( h )} &= - ( 1 + d_{12} + d_{13} ) ( \eta - a - \tilde{\gamma} - t ) ( \eta - a + \tilde{\gamma} - t ), \end{aligned}$$

(C.4)

$$\begin{aligned} D^{ ( h )} &= ( \eta - a + 2\tilde{\gamma} - t ) ( \eta - a - \tilde{ \gamma} - t )^{2}. \end{aligned}$$

(C.5)

Let’s consider the simple case when d ₁₂=d ₁₃=d and $a = \frac{k_{0} + k_{1}}{k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2}}$, $\tilde{\gamma} = \frac{k_{1}}{k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2}}$ and $\eta_{a} = 1 + \frac{1}{k_{0} + 2k_{1}}$ (attractive interband interaction).

Then the second derivative yields

$$\begin{aligned} &\frac{d^{2}h_{c2}}{dt^{2}} = \frac{1}{2}\frac{1}{d ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} )} \\ &\quad \times \frac{8d ( d - 1 )^{2}k_{1}^{2} ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} )^{2}}{ \Bigl( \Bigl( t - \frac{ ( d - 1 ) ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) - 2dk_{1} + k_{1} - 2\sqrt{2d} ik_{1}}{ ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) ( d - 1 )} \Bigr) \Bigl( t - \frac{ ( d - 1 ) ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) - 2dk_{1} + k_{1} + 2\sqrt{2d} ik_{1}}{ ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) ( d - 1 )} \Bigr) \Bigr)^{\frac{3}{2}}} \end{aligned}$$

(C.6)

After further simplifications we obtain

$$\begin{aligned} \frac{d^{2}h_{c2}}{dt^{2}} &= \frac{1}{2}\frac{1}{d ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} )} \\ &\quad \times \frac{8d ( d - 1 )^{2}k_{1}^{2} ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} )^{5}}{ ( ( ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) ( d - 1 )^{2}t - ( d - 1 ) ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) - 2dk_{1} + k_{1} )^{2} + 8dk_{1}^{2} )^{\frac{3}{2}}} \\ & = \frac{4 ( d - 1 )^{2}k_{1}^{2} ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} )^{4}}{ ( ( ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) ( d - 1 )t - ( d - 1 ) ( k_{0}^{2} + k_{0}k_{1} - 2k_{1}^{2} ) - 2dk_{1} + k_{1} )^{2} + 8dk_{1}^{2} )^{\frac{3}{2}}} \ge 0. \end{aligned}$$

(C.7)

Analogously it can be shown for repulsive interband interactions, that $\frac{d^{2}h_{c2}}{dt^{2}}$ is non-negative, too.