Abstract
Recent developments in cognitive and educational science highlight the role of the body in learning. Novel digital technologies increasingly facilitate bodily interaction. Aiming for understanding of the body’s role in learning mathematics with technology, we reconsider the instrumental approach from a radical embodied cognitive science perspective. We highlight the complexity of any action regulation, which is performed by a complex dynamic functional system of the body and brain in perception-action loops driven by multilevel intentionality. Unlike mental schemes, functional systems are decentralized and can be extended by artifacts. We introduce the notion of a body-artifact functional system, pointing to the fact that artifacts are included in the perception-action loops of instrumented actions. The theoretical statements of this radical embodied reconsideration of the instrumental approach are illustrated by an empirical example, in which embodied activities led a student to the development of instrumented actions with a unit circle as an instrument to construct a sine graph. Supplementing videography of the student’s embodied actions and gestures with eye-tracking data, we show how new functional systems can be formed. Educational means to facilitate the development of body-artifact functional systems are discussed.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 The need for reconsidering instrumental genesis from an embodied perspective
When using technology in education, one of the central challenges is that the cultural ways of using it are not self-evident for novice users and learners. If one encounters a piano for the first time, it is not obvious what it is designed for or how to play it. Speaking in general, artifacts preserve practices of former generations in forms that are described as crystallized (Radford, 2003), reified (Wenger, 1998), or sedimented (Goodwin, 2003). The challenge for novice users is to develop practices of using the artifact in a culturally intended way, and, perhaps, further contribute to new practices. For mathematics education, the differences in how novices and experts approach artifacts have been noted for many cases, such as visual inscriptions (Presmeg, 2006; Radford, 2010), formulas (Jansen et al., 2003; Susac et al., 2014), and 3D shapes (Roth, 2018). The question at stake now is how to address theoretically the process of novices appropriating cultural practices of using advanced digital technologies for learning mathematics.
The general case has been addressed in cognitive ergonomics by the instrumental approach (Rabardel, 1995, 2002; Verillon & Rabardel, 1995), which introduces the notion of instrument as a mixed entity that is “formed from two sub-systems” (Verillon & Rabardel, 1995, p. 87), namely, an artifact and “one or more associated utilization schemes” (p. 87). These schemes are cognitive structures that regulate action with artifacts and represent operational invariants, which are often associated with theorems-in-action and concepts-in-action (Vergnaud, 1998). Over the past decades, the instrumental approach has been widely applied to the integration of digital technology into mathematics education (Artigue, 2002; Drijvers & Gravemeijer, 2005; Trouche, 2004). Even though this application was helpful in fostering such integration, the instrumental approach has been criticized for different reasons, such as being a cognitivist perspective that focuses on schemes (Nemirovsky et al., 2013; Noble et al., 2006), approaching mathematics as “a cerebral activity” by some interpreters (Drijvers, 2019), paying a limited attention to the ontological role of bodies and machines in constituting mathematics (Sinclair & de Freitas, 2019).
Indeed, contemporary developments in cognitive and educational science highlight the importance of bodily experience. Both body and artifacts are considered indispensable parts of embodied and extended cognition (Kiverstein & Clark, 2009). A radical embodied approach, which joins enactivist and ecological ideas (Baggs & Chemero, 2018; Kiverstein & Rietveld, 2018), stresses the non-representational character of knowledge. Knowledge emerges as part of a complex dynamic system of behavior, which is constituted through multiple perception-action loops. Cognition, as well as the body, co-evolves with cultural tools (Malafouris, 2018) in the course of and across activities. In application to mathematics education, this approach is consonant with pivotal work by Radford and colleagues (Radford et al., 2005) who highlight embodied processes of social practice with signs and artifacts; by Nemirovsky and colleagues (Nemirovsky et al., 2013), who point to perceptuomotor integration as new emerging capacities of students enactment; and by Abrahamson and colleagues (Abrahamson & Sánchez-García, 2016), who develop a radical embodied approach and highlight the non-linear character of learning.
Alongside theoretical advancement towards the body, digital technology is becoming more body-oriented as well: unlike keyboard-oriented devices, multi-touch screens, augmented and virtual reality platforms, motion sensors, and gesture-recognition systems provide students with rich opportunities for embodied interactions. In mathematics education, these new technological artifacts have shown potential for enhancing students’ number sense (Baccaglini-Frank & Maracci, 2015; Sinclair & Heyd-Metzuyanim, 2014), graph comprehension (Duijzer et al., 2019; Nemirovsky et al., 2013), and conceptual understanding (Duijzer et al., 2017; Ladel & Kortenkamp, 2014). Appropriation of such embodied interactive platforms, just like all other types of artifacts, requires instrumental genesis to become a part of students’ mathematical activity and to contribute to students’ understanding of mathematical concepts. In the light of these developments, the theory of instrumental genesis might benefit from considering embodied interactions in these situations.
To summarize, in line with work done by others (Nemirovsky et al., 2013; Radford et al., 2005), we feel the need to reconsider the instrumental approach from an embodied perspective to highlight the role of the body in regulating instrumented actions and to acknowledge new developments in educational technology and cognitive science. As such, our analysis is driven by the research question of how to reconsider instrumental genesis in mathematics education, taking into account contemporary findings of radical embodied cognitive science on action regulation and knowledge constitution.
To address this question, we develop a dynamic functional systems approach to embodied instrumentation in four steps. First, we introduce the instrumental approach (Section 2) and a notion of body-artifact functional system as radical embodied clarification of instrumented actions constitution (Section 3). Then, we compare these two views on action regulation (Section 4). Next, we revisit instrumental genesis from an embodied perspective (Section 5). Finally, we provide an empirical illustration of the developed approach in an interactive embodied design for trigonometry (Section 6). We claim that understanding instrumented action as constituted by a body-artifact dynamic functional system leads to a coherent theoretical framework that takes seriously the role of the body and of the artifact in instrumented actions. Focusing on embodied processes between a student, an artifact, and a teacher, our theoretical framework provides guidelines for facilitating students’ actions through the development of new technologies and corresponding teaching strategies.
2 Instrumental genesis: from cognitive ergonomics to mathematics education
The instrumental approach originated from cognitive ergonomics (Rabardel, 1995, 2002; Verillon & Rabardel, 1995) and was later appropriated by mathematics education (Artigue, 2002). The instrumented approach distinguishes an artifact, as independent from a user entity, from an instrument, which is a mixed entity that includes an artifact and ways of using it for a particular set of tasks in a form of utilization schemes. The process of instrument formation is called instrumental genesis. This process includes mutual transformations on the side of the learner and on the side of the artifact, namely, instrumentation and instrumentalization. The instrumentation side concerns “the emergence and evolution of utilization schemes and instrument-mediated action” (Rabardel, 2002, p. 103); the instrumentalization side concerns “the emergence and evolution of artifact components of the instrument” (p. 103), such as selection or transformation of the artifacts to a particular task or attribution of the properties to it. A scheme is an “invariant organization of behavior for a certain class of situations” (Vergnaud, 1998, p. 168).
The instrumental approach intends to join genetic epistemology, in which knowledge is built from action, and cognitive approaches, according to which knowledge is put to action (Rabardel, 2002). Epistemic and pragmatics aspects of actions are considered two dimensions of artifact use that “are in constant interaction within... activity” (p. 63). This means that on the one hand, following Piaget, knowledge emerges from action being captured in a scheme, and on the other hand, knowledge is pragmatically applied in instrumental activity.
In mathematics education, researchers, designers, and educators care about conceptual understanding and stress epistemic aspects in the dialectics of epistemic and pragmatic values of an instrument (Artigue, 2002). For artifacts such as a spoon or a teapot, users are happy to forget about the functions outsourced to the material artifact, such as how the tall spout on a teapot prevents spilling the liquid. However, in teaching mathematics, we wish students not only to use artifacts but also to understand the mathematics involved. The expectations that the pragmatic use of the artifacts would automatically lead to or facilitate conceptual understanding in mathematics are mostly not met (Artigue, 2002). To facilitate conceptual understanding in practice, careful orchestration of instrumented actions by a teacher is promoted (Drijvers et al., 2010), as well as the iterative co-development of digital technology techniques together with paper and pen techniques (Artigue, 2002).
Building on Vergnaud’s (1998) definition, the schemes are described as integrating “machine techniques and mental concepts” (Drijvers & Gravemeijer, 2005, p. 168) and a scheme’s “mental part consists of the mathematical objects involved, and of the mental image of the problem solving process and the machine actions” (p. 168), so the conceptual aspect of schemes is often considered including an internal image of a mathematical concept. This theorization of conceptual understanding as mental encapsulation might be explained by centuries of considering mathematics as something independent from sensory experience and incorporated in mind, as Descartes and Kant stated it. However, post-constructivist and radical embodied theories reconsider mathematical concepts as distributed in material activities (Coles et al., 2017), and mathematical cognition as serving and emerging from actions with artifacts (Menary, 2015). This focus on constitutive power of activity was present in the initial instrumental approach as it emerged in cognitive ergonomics: the instrument-mediated action schemes “consist of wholes deriving their meaning from the global action which aims at operating transformations on the object of activity” (Rabardel, 2002, p. 83). We highlight the transformation of meaning as arising from acquiring instrumented actions and aim to clarify better the epistemic value of instrumented actions as constitutive for conceptual understanding.
3 Instrumented actions from a functional dynamic system approach
3.1 A functional system of action regulation
There is a variety of theoretical contributions that point at embodied processes in technologically enhanced mathematics learning (Nemirovsky et al., 2013; Noble et al., 2006; Radford, 2005, 2014; Sinclair & de Freitas, 2014; Swidan et al., 2020; White, 2019). Agreeing with these diverse approaches in their radical departure from a representational view on knowledge, we highlight the need to theorize embodied cognition based on the scientific insights on the constitution of bodily movements. We base our theoretical claims on the literature from ecological psychology and coordination dynamics (Bernstein, 1967; Gibson, 1986; Kelso, 1982; Turvey, 1977); we follow ideas of ecological dynamics (Abrahamson & Sánchez-García, 2016) and develop them towards the use of tools. We claim that enactment is intentionality-driven and regulated by a functional body-brain system in the process of permanent anticipation of and adjustment to the environment through feed-forward and feedback processes of multiple perception-action loops (Turvey, 1977). Next, we unpack the meaning of these terms, see also Fig. 1.
Originally, a functional system was defined in physiology by Anokhin as “a complex of neural elements and corresponding executive organs that are coupled in performing defined and specific functions of an organism” (quoted from Kazansky, 2015, p. 105) and characterized as “self-organizing non-linear systems composed of synchronized distributed elements” (Kazansky, 2015, p. 106). A functional system is responsible for a stable, and yet creative, behavior within partially repetitive environmental constraints. Expanding the notion of a functional system (Fig. 1) beyond physiology, we consider it to be a complex dynamic system that develops as the organism couples with the environment in its “tendency towards a grip on multiple affordances” (Rietveld et al., 2018, p. 3). Affordance—a neologism proposed by Gibson (1986)—“implies the complementarity of the animal and the environment” (p. 127) and means the possibilities for actions, which the environment “offers to an animal” (p. 127). A complex, nested system of affordances enables and at the same time constrains possible enactment by restricting the dynamics that might develop within the system (Rietveld et al., 2018). Complementary to affordances, body potentialities—needed to recognize and act with affordances—develop in the dynamics of functional systems and stored as body structures. Body potentialities and affordances frame perception and action—the two entangled processes in the continuous organism–environment coupling and interaction. These processes mark two directions of this interaction: an influence of the environment on the organism and an influence of the organism on the environment.
A driving force of a functional system is multilevel intentionality; it is understood as a general quality of future directedness and anticipation, which is inherent to the living world—not only of a conscious human. It can be described at multiple levels of action-regulation, such as a primitive Ur-intentionality without a content (Hutto & Satne, 2015), motor and operative intentionality (Merleau-Ponty, 1945/2002), and enactive intentionality of coordinating behavior of others without mind reading (Gallagher & Miyahara, 2012). Fulfilling such intentionalities as organic needs—for example eating or breathing—is interwoven together with finding comfortable moves or solving a mathematical task. At the same time, society plays an essential role in framing the intentionality of personal functional systems through guiding students towards social requests. Students and a teacher form intercorporeal functional systems, for which a bodily system of one student is just a sub-system (Newman, Griffin, & Cole, 1989; Shvarts & Abrahamson, in press). Thus, within nested system of affordances, driven by multiple levels of intentionality, a functional system emerges as a complex of body potentialities, such as an eye–head–body system engaged in visual control (Gibson, 1986) or a complex of physiological adjustments of internal organs, spinal reflexes, telereceptors (eyes and ears), and finally cortical structures engaged in motor control (Bernstein, 1967).
Critically, a functional system is not ruled by a central mechanism. Complex systems of perception-action loops in environment-organism interaction “exhibit self-organization and emergent processes at multiple levels” (Thompson & Varela, 2001, p. 421); each level driven by their own intentionality, and express freedom and creativity, as “emergence involves both upward and downward causation” (p. 421).
What processes take place in a functional system of solving a mathematical task? A complex system of multiple perception-action loops is involved in recognizing mathematical affordances in cultural artifacts and acting upon them. This system includes—and is not limited to—physiological processes, maintenance of a posture, drawing and holding a pen in the space of a notebook, or manipulating digital objects in the space of a screen, or multimodal expressions in an interpersonal space. Of course, many of these processes do not require attention from a secondary school student; think of breathing, holding a pen or adding numbers under ten. However, solving a mathematical task requires all these processes to assemble in one functional system that constitutes an action towards the target outcome. Educational interventions, therefore, need to acknowledge and facilitate the assemblance of all these processes into one system that regulates enactment. For mathematical actions, not only body potentialities but also affordances of cultural artifacts take part in the constitution of an action, as we elaborate in the next section.
3.2 Body-artifact functional system
A bodily functional system regulates intentionality-driven actions by perception-action loops in strong anticipation of environmental dynamics. How can we include a digital artifact, such as dynamic geometry software with a point running on a unit circle, in this embodied process? Gibson notices “When in use, a tool is a sort of extension of the hand... This capacity to attach something to the body suggests that the boundary between the animal and the environment is not fixed at the surface of the skin, but can shift” (Gibson, 1986, p. 41, emphasis original). This idea is consonant with an extended mind proposal, where an extended cognitive system is addressed as “a coupling of biological organism and external resources” (Clark & Chalmers, 1998, p. 18). Tools become incorporated into perception-action loops (Lockman, 2000) in fulfillment of functional requests (Bril, 2015) and perception is now directed towards the point where a tool meets the environment. Indeed, famous experiments on monkeys demonstrate that holding a stick changes the neuronal activity of the monkey’s brain, as visual-motor neurons now respond not only to objects at the distance reachable by a hand, but also at the distance reachable by the stick (Iriki et al., 1996). The same holds for humans: when a patient with space dissociation disorder holds a stick, the distortion of far space disappears as it becomes reachable by a stick (Berti & Frassinetti, 2000). From a functional system approach, a shift of an agential boundary means that an artifact becomes a part of a functional system. In mathematics learning, visual inscriptions, formulas, verbal definitions, and terms are the artifacts that become a functional system’s part. A functional system of a human’s body is now enhanced by the history of cultural practices, crystallized in the artifacts (e.g., Radford, 2003; Wenger, 1998). We suggest calling a system that is formed by an artifact and the body (including brain) a body-artifact functional system (Fig. 2).
Functioning of a body-artifact functional system in instrumented activity leads to a quick transformation of body-potentialities. For example, after a short tryout of a wheelchair, persons are capable to anticipate the height of a path which they need to pass on a wheelchair quite precisely (Stoffregen et al., 2009). Such integration is not determined by the development of an instrumented action scheme somewhere in a head but by direct coupling between body-artifact potentialities and environment. This type of anticipation—strong anticipation (Stepp & Turvey, 2010)—does not require any inference, but allows for direct perception of affordances for a task fulfillment. The plasticity of brain structures enable retaining of new body potentialities: “Once we learn how to recognize, understand, and manipulate mathematical symbols our brains undergo a profound transformation” (Menary, 2015, p. 15), and artifacts serve as extra-cerebral levels in a new functional system (Vygotsky, 1965).
The notion of a body-artifact functional system resonates with the neo-materialist proposal of the artifact having an agency in instrumental activity (White, 2019). Indeed, “learners” bodies are always in the process of becoming assemblages of diverse and dynamic materialities, including physical objects such as pencils, compasses, and calculators (de Freitas & Sinclair, 2013, p. 454). However, we consider digital artifacts as participating in a functional system by their affordances for the learner, rather than as equally agential co-actors in assembling mathematical concepts. The artifacts expose their affordances in response to the learner’s complex intentionalities that develop within social practice, and also at the levels of a biological organism and personality.
Our view is also aligned with the idea of a body incorporating a tool (Noble et al., 2006, p. 389) and perceptuomotor integration as a mark of fluent use of a tool (Nemirovsky et al., 2013). Nemirovsky and colleagues showed how students’ lived experience with motion detectors grounded new perception of the graphs. We interpret this experience as developing body-potentialities for perceiving new affordances of the graphs. Nemirovsky and colleagues altered the instrumental approach that is at risk of mentalism with a non-dualist approach which “locate(s) both tool fluency and mathematical thinking and learning in the evolving relationships between action and perception when learners engage with a physical artifact” (p. 378). We share this view on conceptual aspects of understanding the mathematics of the machine as being born from the students’ actions.
4 Body-artifact functional system’s view on an instrumented action scheme
Both schemes and functional systems are constructs that describe constitution of stable actions in particular circumstances. However, while scheme-regulated behavior is usually seen as determined by a central mechanism (see exception in the works of Cellérier, cited by Rabardel, 2002), a functional system is principally decentralized. There are no central mechanisms of taking decisions and determining actions. The structural materiality of body potentialities and environmental affordances both contribute to the constitution of actions, which are driven by a web of intentionality. The artifacts, thus, come to shape the dynamics of the system, as well the students’ bodies and their potentialities come to recognize artifacts’ affordances.
These two theoretical conceptualizations of stable action constitution differ on how stability is achieved. To understand this difference, we focus on how a person predicts that action leads to the desired outcome and distinguish weak and strong anticipation (Stepp & Turvey, 2010). Let us consider a simplified example of a classic baseball outfielder problem (Chapman, 1968). Imagine a person throws a ball ahead, and another person nearby starts to run aiming to catch the ball. Two strategies can be distinguished in achieving a reliable result: The runner can mentally calculate the parabolic movement of the ball and where it will reach the ground, and then run to that point. Such anticipation of the events in reality is called weak anticipation, as it is an anticipation by one system—a cognitive system of the runner—of the behavior of another system—the ball in the field (Stepp & Turvey, 2010). Another possibility is that the runner eventually runs all the time directly under the ball, and naturally catches the ball when it approaches the ground. The runner and the ball couple in a new system and continuously anticipate and coordinate positions within the system. This type of anticipation is called strong anticipation.
A scheme is usually meant to regulate behavior by representing the situation and possible outcomes of actions, thus ruling the choice of appropriate moves (Vergnaud, 1998). In this way, a scheme becomes a central place to keep invariants of actions and to rule the performance. The predictive power of a scheme is based on possibility to compute an action outcome, which is separate from the executing system, so a scheme regulates behavior through weak anticipation between two systems.
In a functional system, stability emerges in the tight coupling between an organism and an environment within intentionality. There is no scheme that would compute, predict, and control invariant behavior; instead, the interaction self-organizes in a way that leads to stability. Just like a runner, having discovered the strategy of running under the ball, would not miss the ball anymore due to the strong anticipation of the situation in recurrent acts of interaction. Aiming for the fulfillment of intentionality—not to following an invariant—a functional system of central and peripheral bodily parts dynamically and creatively adapts to the changes in environment. Scheme-like voluntary control of the invariant behavior (something like “I should run and check if the ball is ahead”) might emerge or do not emerge in the functional system, depending on the circumstances and request for a deliberate effort in describing the strategy.
Given the functional systems perspective, we need to reconsider our understanding of the conceptual knowledge, which was attributed to the mental scheme by some researchers in mathematics education (Drijvers & Gravemeijer, 2005). In a body-artifact functional system, knowledge is distributed across the body and the artifacts, which are both in use. We hypothesize that conceptual understanding in mathematics is related to active recognition of artifacts’ affordances or re-creating of the artifacts in the course of instrumentalization within fulfilling students’ intentionality. Such artifacts come to substitute a part of the functional system, which initially develops to fulfill target intentionality without an artifact.
5 Instrumental genesis from a body-artifact functional system perspective
While complex dynamic system theory stresses the self-organization of the dynamic systems, from an educational perspective, we want to identify conditions that promote such a self-organization and foster the genesis of body-artifact functional systems for regulating instrumented actions. First of all, we stress the role of a task in assembling a new functional system. “What is learnt is not the movement but the capacity to satisfy the functional parameters of the task” (Bril, 2015, p. 109). It is a target task, not an invariant of action that determines the way of acting in a mathematically meaningful use of an artifact.
As a new functional system emerges, the new coupling between a student and a mathematical environment is established. Thus, both the student and the environment are transformed to accomplish the target action: a student develops new body potentialities and recognizes and/or creates new affordances in the environment. In the case of an instrumented action, bi-directional transformation of a student’s body and of an environment includes transition of the artifact from the side of environment into a body-artifact functional system (Fig. 3). In the instrumental approach, this process of instrumental genesis is explained as instrumentalization and instrumentation, respectively, the transformation of an artifact and the students’ schemes of action. From a radical embodied approach, we propose to see instrumentalization as recognition and/or creation of affordances and instrumentation as development of body potentialities. Note however that this distinction is only analytic; we consider these as two sides of the same process.
5.1 Instrumentation as development of body potentialities
Body-potentialities are an inherently developing structures of body and brain (neurons grow, muscles become stronger and ligaments become more flexible), which enable new functionalities. Unlike a mental scheme, body potentiality does not represent invariance of the action, but enables the relevant enactment per se. How can the teaching-learning process contribute to such a development before the target action has been established?
In accordance with the radical embodied approach, ways of teaching new actions have been theorized as establishing fields of promoted actions (Reed & Bril, 1996). Body potentialities develop within multiple levels of a functional system. Given that, we can distinguish two approaches to education. For example, a functional system of cycling fulfills balancing, pedaling, steering, which are all organized in a united system of action. One approach is a scaffolding strategy, where an adult temporarily and contingently complements a functional system by holding a child’s bike at the luggage rack (or helps an infant in her first steps, see Luria & Vygotsky, 1930/1992; Shvarts & Bakker, 2019). In a comparable way, a teacher facilitates the establishment of a new functional system by reducing degrees of freedom in students’ behavior and maintaining the focus on accomplishing the task (Bernstein, 1967; Shvarts & Bakker, 2019; Wood et al., 1976).
Another approach lies in designing special devices that target the oldest grounding levels of coordinations within the functional system. Such an approach led to the development of a balance bike, which is now a prominent tool in learning to ride a bike. When applied to mathematics education, this approach has been promoted as an “instrumented field of promoted actions” (Abrahamson & Trninic, 2015) within an embodied design genre (Abrahamson, 2014; Bakker et al., 2019). Designing special environments that facilitate sensory-motor coordinations, researchers contribute to the development of body potentialities, which are later involved in conceptual understanding of mathematics.
5.2 Instrumentalization as recognition and creation of affordances
A complementary side of developing body potentialities is the recognition of affordances. Appealing to the concept of affordance in the interpretation of instrumental genesis is not new. Trouche (2020) proposes affordance as a critical term for the analysis of instrumental genesis, and uses it as a methodological means to unpack what technology affords to a learner. Monaghan (2016) stresses the importance of affordances for the analysis of the tool’s use as well. He distinguishes physical and cultural affordances and stresses the learning process is needed to highlight cultural affordances.
However, one cannot teach recognition of affordances directly. Instead, it is through exploratory attempts to perform an action that affordances become apparent to a learner: “exploratory activity is the primary behavioral mechanism for generating perceptual information and for differentiating information from the flux” (Adolph & Kretch, 2015, p. 130). Such exploratory activity with the tools might be facilitated by fields of promoted actions, namely, the situations where the use of the tools is well constrained and supported (Bril, 2015).
With respect to the reconsideration of mathematics as being developed in material practice, we point out that recognition and creation of an affordance become two instances of the same process. When intending to eat, one can take a spoon, thus recognizing its particular affordances, or create affordances by cutting a spoon from wood, if no spoon is available. The same way, wanting to estimate the sine of a particular angle, one might use a unit circle if available, or draw it on a piece of paper, or just refer to an imaginary unit circle which can be activatedFootnote 1. Thus, creation of particular artifacts (such as inscriptions or emergent models, see Gravemeijer, 1999) might be particularly helpful in teaching affordances for instrumented actions in mathematics. An epistemic value of instrumented actions lies in recognition and creation of new affordances within broad and flexible functional systems.
6 Empirical illustration of the genesis of a body-artifact functional system
We illustrate the genesis of the body-artifact functional system with an empirical case study from trigonometry in which a student uses a unit circle to build and understand a sine graph (see also Alberto et al., 2019). As a methodological means to illustrate our theoretical claims, we use an embodied action-based design genre (Abrahamson, 2014; Abrahamson et al., 2011; Bakker et al., 2019) that provides an opportunity to observe and facilitate development of mathematical concepts as students progress from establishing new sensory-motor coordinations to multimodal expressions of their reflections on the performance. Each mathematical relation is presented at first in the form of a sensory-motor task, as the student is asked to find and maintain continuous green feedback from the screen. Later, a tutor changes the task by asking the students to reflect on their sensory-motor strategies. As the tutors support the transition of sensory-motor experiences into mathematical discourse (Flood, 2018), they use a variety of multimodal tactics in eliciting students’ verbal and gestural expressions of their sensory-motor coordinations and bridging these expressions with scientific discursive norms (Flood, 2018; Flood et al., 2020)Footnote 2. Aiming to facilitate inclusion of sensory-motor coordinations into further mathematical reasoning in a course of embodied instrumentation (Drijvers, 2019), we supplemented embodied action-based design ideas by mathematical tasks that require instrumented actions. We offered the students the tools that incorporate previously developed coordinations and could be helpful in solving mathematical tasks. For example, once the student established the sensory-motor coordination between an arc on the unit circle and an x-coordinate on the Cartesian plane (section 6.1), a task of transitioning from degrees to radians was given (section 6.2)Footnote 3.
Below, we summarize an empirical tryout of our design with a first-grade college student, whom we call Dmitry. Dmitry studied trigonometry at secondary school approximately three years before this research and did not have any experience of interacting with multi-touch learning technologies. As the data have shown, his prior knowledge was insufficient to establish the connection between the unit circle and the sine graph, although he did anticipate a general wavy form of the sine. The teaching/learning session was audio and video recorded and the student’s eye-movements were recorded to reveal the sensory-motor processes, such as overt-attention to relevant—for affordances’ recognition—aspects of the artifacts. The first author acted as the tutor.
6.1 Developing multilevel functional system and body-potentialities
The first task concerns the coordination of the distances along the circumference of the unit circle and along the x-axis. A student is moving point C around the circle and point X on x-axis (Fig. 4). The feedback-frame turns green when these two target distances are equal. Note, the origin on the Cartesian plane is deliberately aligned with the counting origin on the unit circle. The student is required to find green locations and then maintain—while moving both hands—continuous green feedback from the screen without knowing ahead of the enactment the rule that determines the feedback.
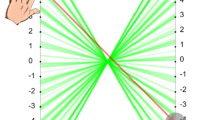
Dmitry explored the embodied task. He iteratively started from the origin and his right hand was delayed compared to the left hand, thus making the frame red. His eyes concentrated on the frame most of the time (Fig. 5a). After about 2 min, he managed to sustain green feedback. The constant feedback from the interactive design facilitated the development of a new functional system of body regulation in the digital environment.
At the next stage, the tutor then triggered reflection, thus establishing a new level of intentionality and a new lever of the functional system, namely, a level of verbal description.
T: Let us reflect. How are you achieving this feedback, how are you keeping it green all the time?
D: <…> I am looking at the color. But I would not be so certain were I should be. I can approximately suggest where I have to be.
T: How would you do such an estimation?
D: <…> the further I am on the circle [gesture around the circle, Fig. 5b] … the further I am here [gesture along the x-axis, Fig. 5c]. So obviously these two correspond. But this one… if I am moving, this one [gesture to the point on the circle] moves slightly faster….. as this like here [as point on x-axis moves].
Dmitry’s reflection uncovers perception of a one-to-one correspondence between the points. The particular relations between the points as moving the right point “slightly faster” are determined by his iterative attempts to move his right hand faster and overcome symmetrical moves, which body potentialities naturally initiated.
While regulation of the action by a scheme would suppose that sensory-motor performance grasps “the same speed” relation from the very beginning (in a form of theorem-in-action, according to Vergnaud, 1998), in this example, we see that the performance is regulated at multiple levels. Sensory-motor performance was also possible without verbal regulation—as we can see from the difficulties that the student experienced once asked to reflect—and stood out for the student as a “slightly faster” strategy.
The conventional expression “same speed” emerged in negotiation with the tutor, thus bringing sensory-motor experience in correspondence with scientific discourse (Flood, 2018). Furthermore, Dmitry expressed his strategy through a metaphor: “It is a kind of a wire. Just wired on [he is performing a gesture that expresses the metaphor, Fig. 6]”. These gestural-verbal expressions emerged as an additional level of regulation which played a role in further conceptual understanding (Section 6.4). We theorize it as a functional system of Dmitry's embodied experience being stored as body-potentialites, which makes Dmitry ready for further recognition of other artifacts' affordances.
6.2 An artifact as a part of a perception-action loop
The next episode represents a more conventional mathematical task, in which Dmitry is asked to find the corresponding angles and radians using an artifact in which the previously enacted coordination was reified: only the point C on the circle was manipulatable and the sum of green arc and green segment was equal to the entire circle (see Fig. 7).
The items in this task did not require much effort from Dmitry. Figure 8 shows his solution of the first item: 1 radian = x degrees.
Dmitry moved the point around the circle and at the same time looked at the x-axis. He looked at the location of 1 radian before it was actually reached by the green segment (Fig. 8a), thus anticipating the enactment of the artifact within body-artifact functional system.Footnote 4
As soon as Dmitry understood the task, he immediately recognized the artifact’s affordances for aligning two different measurements of the arc: a possibility to measure arch length in degrees on the unit circle and to receive the length in radians on the x-axis. Previously elaborated coordination is preserved in Dmitry’s body potentialities and now was activated within the new body-artifact functional system and helped in anticipation of the automatically moving point along the x-axis. New instrumented action was regulated by the system of the body (including eye-movements) and the artifact: the perception-action loop was mediated—in a very physical, not semiotic sense—by the green automatically moved strip which physically connects arm movement and gaze. Digital artifact, Dmitry’s motor action and focus of visual perception are physically interlocked into a loop, thus contributing to the solution of the target task.
6.3 Facilitation and self-organization in affordance recognition
In the last task in the learning sequence, green feedback facilitated drawing a sine graph by manipulating two points (see Fig. 9). Point C moved along the circle, and a new point G moved on the coordinate plane horizontally and vertically. The target performance included alignment of the y-coordinates of two points and the alignment of the x-coordinate of the point G and the arc that point C passed. When the points are moved correctly, the frame becomes green and the dots of the sine graph emerge. To help the student in comparison of the arc’s length and the x-coordinate of the point G, two numbers, each reporting the length of the target distance, were added to the digital environment.
By that moment in the learning sequence, Dmitry had already half-automatically generated a line of a sine graph: he manipulated only point G, while point C would be automatically adjusted based on the x-coordinate of G. However, once Dmitry was asked to draw the line again with full freedom in manipulating the x and y coordinates of G and C, he got lost. In this task, the student needed to integrate two previously explored coordinations in one functional system: vertical alignment (not reported in this paper) and correspondence between arc length and x-coordinate.
He started by enacting a wavy movement based on the resemblance with the sine graph. His eye-movements demonstrated that he was taking care of the y-coordinates of two points by keeping them vertically aligned. However, he never directly gazed at the correspondence between the arc length and x-coordinate: He gazed neither at the numbers nor at the arc. As a result, the frame never became green and the graph did not emerge.
The tutor provided a hint by pointing to the numbers, which had been present on the screen all the time.
T: Should these numbers perhaps help you? (Fig. 10)
In response, the student’s behavior changed dramatically (cf. Abrahamson et al., 2011): Dmitry looked iteratively at two numbers, moved both points to the maximum position at π/2, iteratively checked the numbers again, and moved the points back to the origin maintaining green, checking the numbers and repeating with a low-level voice:
D: Wait… These are the same… These are the same…
Then Dmitry easily found the green position in the second quadrant by his own initiative (Fig. 11).
D: I know that. It is also the prediction that I had. [...] but it did not appear at first.
Now, as the strategy of keeping two numbers the same had surfaced, it appeared to Dmitry as fully corresponding with his anticipations. He tested this new strategy at a few more points by his own initiative.
The tutor facilitated the emergence of the target functional system by pointing at two numbers and thus helping the student to notice the affordance of the artifact and to establish the target perception-action loop for coupling with the artifact. One pointing gesture triggered essential re-organization of the functional system in the task performance. Although the tutor did not provide any explanation of the meaning of these numbers, Dmitry immediately recognized the target affordance based on his previous experience. The numbers afforded for him an action of finding the equivalence between an arc and x-coordinate. The student spontaneously practiced the performance, thus solidifying the assembled functional system. The phrase “these are the same” regulated behavior at the verbal level and the eye-movements between two numbers regulated the target coordination at the embodied level. Body and digital artifact (including two numbers) came to be joined in one functional system of drawing the sine graph, making use of the body potentialities developed in the previous tasks. This instrumented action was regulated at multiple levels, such as verbal expression and sensory-motor coordination. Initial coordination of two equal distances was easily transformed into maintaining the same values of two numbers, thus demonstrating the creativity and flexibility of the functional system, which went beyond repeating previous experience and assembled towards target instrumented action with the available artifact.
6.4 Epistemic value within the functional system of instrumented action
In a short while, the tutor questioned the student’s understanding of this strategy, thus exploring the epistemic value of the emerged functional system:
T: Why? What does this mean? These same values? [the tutor gestures between two numbers]
D: They again, they are kind of amount that I traveled over there [gestures along the circle], so to say, from the tape that I have that does it as much as it have got … So, it is a roller tape [multiple gestures around the circle, Fig. 12a], it is just moved more and more [a gesture as if unrolling a tape, Fig. 12b]. And it is straightened out [a stretching gesture, Fig. 12c]. Just as far.
T: So, this distance [a gesture along the x-axis]?
D: It is a rolled up, from the whole thing.
Dmitry’s understanding of maintaining two values the same appeared to be grounded in the previous embodied experience of moving around the circle (compare Fig. 12 with Figs. 5 and 6, section 6.1). The functional system served not only pragmatic performance but also epistemic explanation. The metaphorical expression had reified embodied experience, thus becoming a new artifact (a word) that constituted epistemic value in response to the tutor’s request. The metaphor spontaneously traveled from “a wired wire” to “a roller tape,” as the student gestures at first on the screen (Fig. 12a) and then separately, repeating his gestures of the previous activity. We see an iterative constitution of the verbal expression that tends to grasp embodied experiences of the functional system in the best way.
6.5 Joining empirical episodes
Our empirical illustration demonstrated the tight coupling of an artifact’s affordances and student’s body potentialities in one functional system of instrumented actions. Episode 1 (Section 6.1) showed the development of body potentialities, which were later involved into body-artifact functional systems. At first (Section 6.2), a point that traveled around the unit circle was attached to a point on the x-axis by a green line; these points were used to translate degrees to radians. Secondly (Section 6.3), a complex artifact with a point on the unit circle and the numbers to align arc length and x-coordinate were used to draw a sign graph. In both cases, hand and eye movements demonstrated that artifact and body were jointly interlocked into perception-action loops, thus forming the body-artifact functional systems.
As we saw in the second case (Section 6.3), the body-artifact functional system regulated behavior at multiple levels: a verbal expression “these are the same” and perceptually checking the numbers by the eye-movements maintained coordination of two distances. Note that despite the availability of the required body-potentialities, the student did not notice the numbers on the artifact at first. However, once prompted towards the numbers, the student immediately recognized their affordances. The numbers substituted the part of the functional system that he had already developed: he was maintaining the equal arc length and a segment on the x-axis in the previous task (see Section 6.1).
Despite the initial difficulty in assembling a functional system, Dmitry could easily explain its functioning through a metaphor grounded in his previous embodied experience (Section 6.4). Thus, the functional system did not only help in the pragmatic achievement of the target solution but provided an opportunity for conceptual understanding.
7 Conclusion
The question guiding this study was how to reconsider instrumental genesis in mathematics education taking into account contemporary findings of radical embodied cognitive science on action regulation and knowledge constitution. The radical embodied perspective led us to introduce the notion of a body-artifact functional system regulating instrumented actions to clarify the notion of instrument. With respect to the science of coordination dynamics (Bernstein, 1967; Kelso, 1982; Turvey, 1977), a functional system emerges as a synergy of many perception-action loops. When a functional system is extended by an artifact, this artifact takes part in perception-action loops. This process is vividly visible in the case where Dmitry manually manipulated one side of the artifact and looked at the other side of the artifact (Section 6.2). We highlight multiple levels of action regulation in this functional system: both verbal expressions and sensory-motor coordinations play a constitutive role in the target performance, as empirical examples in Sections 6.1 and 6.3 illustrate. In this paper, we mostly focused on the digital tools and a sine graph as a visual artifact; other artifacts—such as mathematical terms and definitions—also need to be involved in this functional system as intentionality of communicating, reflecting, or describing calls forth the verbal level of regulation.
From the radical embodied perspective, the process of instrumental genesis consists of the preparation of body potentialities (instrumentation), which contribute to the recognition or creation of the artifact’s affordances (instrumentalization). Our approach stresses the decentralized and multilevel nature of action constitution within a functional system distributed across the body (including the brain) and artifacts. Careful preparation of body potentialities in previous embodied activities can enable recognition of the artifact’s affordances in further mathematical problem solving (Sections 6.1 and 6.2). However, the emergence of a functional system as a self-organization process cannot be guaranteed, only facilitated. This self-organization process takes place within intentionality-driven behavior and it might be triggered by downward causation of a social interaction. It is up to a teacher to flexibly modulate intentionality of a student by introducing sub-tasks and to facilitate the self-organization process by pointing gestures or verbal expressions. In Section 6.1, we illustrated how socially triggered reflection led to establishing a verbal level of regulation. In Section 6.3, we pointed out how the tutor’s subtle prompts directing the student’s attention by a pointing gesture led to assembling a new body-artifact functional system.
In our approach, we want to avoid separation of action and knowledge, and of body and mind. In line with the radical embodied theories cited, we highlight the constitutive role of action in conceptual understanding. An artifact—as it preserves previous actions in a reified, materialized form—ensures the epistemic value of the body-artifact functional system, but efforts are needed for a student to take advantage of this epistemic dimension.
We hypothesize that an artifact gets incorporated into a body-artifact functional system of a student with conceptual understanding in case it substitutes a part of the student’s previously developed body potentialities; thus, the student repeats (in a simplified way) the historical invention of the artifact. Designing the tasks along with this hypothetical claim, before introducing a mathematical artifact, an educator would need to make sure that a student is capable of the target action crystallized in the artifact without the artifact per se. We illustrate this theoretical statement in Section 6.4 where postponing the introduction of a sine graph—as the target artifact—led to a conceptual understanding of relations between a unit circle’s arc and x-coordinate of the point on the emerging sine graph. Embodied activities that precede instrumented actions provide an opportunity to develop body potentialities, which are later involved in the recognition of the artifacts’ affordances. Additional empirical research is needed to corroborate this theoretical statement. However, our theoretical vision explains well the role of pen-and-paper techniques, which has been stressed within the instrumental approach literature: enactment without outsourcing a substantial part of the functional system to an artifact makes students’ bodies capable of the entire action. In this vision, counting without a calculator and coordinating the unit circle’s arc and x-coordinate are needed before outsourcing these actions to a calculator or to dynamic geometry software.
A body-artifact functional system is decentralized and multilevel; different levels of action regulation can cause self-organizing transformations in other levels. These upward and downward causations are not deterministic, but rather act as the triggers for synergetic development. Following Hutto and Sánchez-García (2015) in their call for teaching approaches that “generate more flexible modes of coupling with the environment” (p. 328) in sports training, we call for teaching guidance that leaves freedom for students’ self-organizing processes and facilitates assembling flexible and creative functional systems with the artifacts by careful prompts, guiding questions and tasks.
Notes
Note that an imaginary unit circle is not a mental representation of a previously seen unit circle, but part of prospective control of a possible task of drawing a unit circle (see Kiverstein & Rietveld, 2018).
From the functional systems perspective, a student and a tutor form an intercorporeal functional dynamic system, in which perception and action of two subjects are interwoven in a process of supporting the student’s development of new cultural forms of behavior (Shvarts & Abrahamson, in press), so a body-artifact functional system—the topic of this paper—develops within tight collaboration with a tutor; however, this consideration is beyond the scope of this paper.
A full description of the design sequence is beyond the scope of this theoretical paper. The design sequence can be find at our website https://embodieddesign.sites.uu.nl/activity/. Press on the screenshots for Trigonometry, Activity 1 to access the interactive tasks.
In Fig. 8, the fixation of the student is slightly shifted to the right from 1 due to the calibration issues; we can be sure about anticipatory behavior from analysis of the dynamic record.
References
Abrahamson, D. (2014). Building educational activities for understanding: An elaboration on the embodied-design framework and its epistemic grounds. International Journal of Child-Computer Interaction, 2(1), 1–16. https://doi.org/10.1016/j.ijcci.2014.07.002
Abrahamson, D., & Sánchez-García, R. (2016). Learning is moving in new ways: The ecological dynamics of mathematics education. The Journal of the Learning Sciences, 25, 203–239. https://doi.org/10.1017/CBO9781107415324.004
Abrahamson, D., & Trninic, D. (2015). Bringing forth mathematical concepts: Signifying sensorimotor enactment in fields of promoted action. ZDM-Mathematics Education, 47(2), 295–306. https://doi.org/10.1007/s11858-014-0620-0
Abrahamson, D., Trninic, D., Gutiérrez, J. F., Huth, J., & Lee, R. G. (2011). Hooks and shifts: A dialectical study of mediated discovery. Technology, Knowledge and Learning, 16, 55–85. https://doi.org/10.1007/s10758-011-9177-y
Adolph, K. E., & Kretch, K. S. (2015). Gibson’s theory of perceptual learning. In J. D. Wright (Ed.), International Encyclopedia of the Social & Behavioral Sciences: Second Edition (pp. 127–134). Elsevier. https://doi.org/10.1016/B978-0-08-097086-8.23096-1
Alberto, R., Bakker, A., Walker-van Aalst, O., Boon, P., & Drijvers, P. (2019). Networking theories in design research: An embodied instrumentation case study in trigonometry. In U. T. Jankvist, M. van den Heuvel-Panhuizen, & M. Veldhuis (Eds.), Proceedings of the 11th Congress of the European Society for Research in Mathematics Education (CERME 11) (Vol. TWG17: Theoretical perspectives and approaches in mathematics education research) (pp. 3088–3095). Utrecht University.
Artigue, M. (2002). Learning mathematics in a CAS environment: The genesis of a reflection about instrumentation and the dialectics between technical and conceptual work. International Journal of Computers for Mathematical Learning, 7(3), 245–274. https://doi.org/10.1023/A:1022103903080
Baccaglini-Frank, A., & Maracci, M. (2015). Multi-touch technology and preschoolers’ development of number-sense. Digital Experiences in Mathematics Education, 1(1), 7–27. https://doi.org/10.1007/s40751-015-0002-4
Baggs, E., & Chemero, A. (2018). Radical embodiment in two directions. Synthese. https://doi.org/10.1007/s11229-018-02020-9
Bakker, A., Shvarts, A., & Abrahamson, D. (2019). Generativity in design research: The case of developing a genre of action-based mathematics learning activities. In U. T. Jankvist, M. H. A. M. V. D. Heuvel-Panhuizen, & Veldhuis M. (Eds.), Proceedings of the 11th Congress of the European Society for Research in Mathematics Education (CERME 11) (Vol. TWG17: Theoretical perspectives and approaches in mathematics education research, pp. 3096–3103). Utrecht, the Netherlands: Utrecht University. Retrieved from https://hal.archives-ouvertes.fr/hal-02418078
Bernstein, A. N. (1967). The co-ordination and regulation of movements. Pergamon Press.
Berti, A., & Frassinetti, F. (2000). When far becomes near: Remapping of space by tool use. Journal of Cognitive Neuroscience, 12(3), 415–420. https://doi.org/10.1162/089892900562237
Bril, B. (2015). Learning to use tools: A functional approach to action. In L. Filliettaz & S. Billett (Eds.), Francophone Perspectives of Learning Through Work. Professional and Practice-based Learning, (Vol. 12, pp. 95–118). Springer Nature. https://doi.org/10.1007/978-3-319-18669-6_5
Chapman, S. (1968). Catching a baseball. American Journal of Physics, 36(10), 868–870. https://doi.org/10.1119/1.1974297
Clark, A., & Chalmers, D. J. (1998). The extended mind. Analysis, 58(1), 7–19.
Coles, A., de Freitas, E., & Sinclair, N. (2017). Introduction. In What is a mathematical concept? (pp. 1–16). Cambridge University Press. https://doi.org/10.1017/9781316471128.001
de Freitas, E., & Sinclair, N. (2013). New materialist ontologies in mathematics education: The body in/of mathematics. Educational Studies in Mathematics, 83(3), 453–470. https://doi.org/10.1007/s10649-012-9465-z
Drijvers, P. (2019). Embodied instrumentation: Combining different views on using digital technology in mathematics education. In U. T. Jankvist, M. van den Heuvel-Panhuizen, & M. Veldhuis (Eds.), Eleventh Congress of the European Society for Research in Mathematics Education (pp. 8–28). Utrecht University.
Drijvers, P., Doorman, M., Boon, P., Reed, H., & Gravemeijer, K. (2010). The teacher and the tool: Instrumental orchestrations in the technology-rich mathematics classroom. Educational Studies in Mathematics, 75(2), 213–234. https://doi.org/10.1007/s10649-010-9254-5
Drijvers, P., & Gravemeijer, K. (2005). Computer algebra as an instrument: Examples of algebraic schemes. In D. Guin, K. Ruthven, & L. Trouche (Eds.), The Didactical Challenge of Symbolic Calculators (vol. 36, pp. 163–196). Springer. https://doi.org/10.1007/0-387-23435-7_8
Duijzer, C., Shayan, S., Bakker, A., Van der Schaaf, M. F., & Abrahamson, D. (2017). Touchscreen tablets: Coordinating action and perception for mathematical cognition. Frontiers in Psychology, 8, 144. https://doi.org/10.3389/fpsyg.2017.00144
Duijzer, C., Van den Heuvel-Panhuizen, M., Veldhuis, M., Doorman, M., & Leseman, P. (2019). Embodied learning environments for graphing motion: A systematic literature review. Educational Psychology Review., 31, 597–629. https://doi.org/10.1007/s10648-019-09471-7
Flood, V. J. (2018). Multimodal revoicing as an interactional mechanism for connecting scientific and everyday concepts. Human Development, 61(3), 145–173. https://doi.org/10.1159/000488693
Flood, V. J., Shvarts, A., & Abrahamson, D. (2020). Teaching with embodied learning technologies for mathematics: Responsive teaching for embodied learning. ZDM-Mathematics Education, 52, 1307–1331. https://doi.org/10.1007/s11858-020-01165-7
Gallagher, S., & Miyahara, K. (2012). Neo-pragmatism and enactive intentionality. New directions in philosophy and cognitive science: Adaptation and cephalic expression, 2, 117–146. https://doi.org/10.1057/9780230360792_6
Gibson, J. J. (1986). The ecological approach to visual perception. Psychology Press.
Goodwin, C. (2003). Pointing as situated practice. In K. Sotaro (Ed.), Pointing: Where Language, Culture and Cognition Meet (pp. 217–241). Lawrence Erlbaum.
Gravemeijer, K. (1999). How emergent models may foster the constitution of formal mathematics. Mathematical Thinking and Learning, 1(2), 155–177. https://doi.org/10.1207/s15327833mtl0102_4
Hutto, D. D., & Sánchez-García, R. (2015). Choking RECtified: embodied expertise beyond Dreyfus. Phenomenology and the Cognitive Sciences, 14, 309–331.
Hutto, D. D., & Satne, G. (2015). The natural origins of content. Philosophia, 43(3), 521–536. https://doi.org/10.1007/s11406-015-9644-0
Iriki, A., Tanaka, M., & Iwamura, Y. (1996). Coding of modified body schema during tool use by macaque postcentral neurons. NeuroReport, 7(14), 2325–2330. https://doi.org/10.1097/00001756-199610020-00010
Jansen, A. R., Marriott, K., & Yelland, G. W. (2003). Comprehension of algebraic expressions by experienced users of mathematics. Quarterly Journal of Experimental Psychology Section A: Human Experimental Psychology, 56 A(1), 3–30. https://doi.org/10.1080/02724980244000134
Kazansky, A. B. (2015). Agential anticipation in the central nervous system. In M. Nadin (Ed.), Anticipation: Learning from the Past. Cognitive Systems Monographs (vol. 25, pp. 101–112). Springer. https://doi.org/10.1007/978-3-319-19446-2_6
Kelso, J. A. S. (1982). Human Motor Behavior. Psychology Press. https://doi.org/10.4324/9781315802794
Kiverstein, J. D., & Clark, A. (2009). Introduction: Mind embodied, embedded, enacted: One church or many? Topoi, 28(1), 1–7. https://doi.org/10.1007/s11245-008-9041-4
Kiverstein, J. D., & Rietveld, E. (2018). Reconceiving representation-hungry cognition: An ecological-enactive proposal. Adaptive Behavior, 26(4), 147–163. https://doi.org/10.1177/1059712318772778
Ladel, S., & Kortenkamp, U. (2014). Number concepts—processes of internalization and externalization by the use of multi-touch technology. In U. Kortenkamp, B. Brandt, C. Benz, G. Krummheuer, S. Ladel, & R. Vogel (Eds.), Early Mathematics Learning (pp. 237–253). Springer. https://doi.org/10.1007/978-1-4614-4678-1_15
Lockman, J. J. (2000). A perception-action perspective on tool use development. Child Development, 71(1), 137–144. https://doi.org/10.1111/1467-8624.00127
Luria, A. R., & Vygotsky, L. S. (1930/1992). The child and his behavior. In E. Rossiter (Trans.), Ape, Primitive Man, and Child: Essays in the History of Behaviour. Harvester Wheatsheaf. Retrieved from https://www.marxists.org/archive/vygotsky/works/1930/man/index.htm
Malafouris, L. (2018). Bringing things to mind: 4Es and material engagement. In A. Newen, L. De Bruin, & S. Gallagher (Eds.), The Oxford Handbook of 4E Cognition (pp. 755–772). https://doi.org/10.1093/oxfordhb/9780198735410.013.40
Menary, R. (2015). Mathematical cognition - A case of enculturation. Open MIND, 25, 12–18. https://doi.org/10.15502/9783958570818
Merleau-Ponty, M. (1945/2002). Phenomenology of perception. Routledge.
Monaghan, J. (2016). Developments relevant to the use of tools in mathematics. In Tools and Mathematics. Mathematics Education Library (vol. 110, pp. 163–180). https://doi.org/10.1007/978-3-319-02396-0_7
Nemirovsky, R., Kelton, M. L., & Rhodehamel, B. (2013). Playing mathematical instruments: Emerging perceptuomotor integration with an interactive mathematics exhibit. Journal for Research in Mathematics Education, 44(2), 372–415. https://doi.org/10.5951/jresematheduc.44.2.0372
Newman, D., Griffin, P., & Cole, M. (1989). The construction zone: Working for cognitive change in school. Cambridge University Press.
Noble, T., DiMattia, C., Nemirovsky, R., & Barros, A. (2006). Making a circle: Tool use and the spaces where we live. Cognition and Instruction, 24(4), 387–437. https://doi.org/10.1207/s1532690xci2404_1
Presmeg, N. (2006). Research on visualization in learning and teaching mathematics. In A. Gutiérrez & P. Boero (Eds.), Handbook of Research on the Psychology of Mathematics Education: Past, Present and Future (pp. 205–235). Brill | Sense. https://doi.org/10.1163/9789087901127_009
Rabardel, P. (1995). Les hommes et les technologies. Approche cognitive des instruments contemporains. Paris, France: Armand Colin Retrieved from https://www.persee.fr/doc/stice_1265-1338_1995_num_2_2_1202_t1_0237_0000_1
Rabardel, P. (2002). People and technology: A cognitive approach to contemporary instruments. Université Paris Retrieved from https://hal.archives-ouvertes.fr/hal-01020705
Radford, L. (2003). On the epistemological limits of language: Mathematical knowledge and social practice during the renaissance. Educational Studies in Mathematics, 52(2), 123–150. https://doi.org/10.1023/A:1024029808871
Radford, L. (2005). The semiotics of the schema: Kanty, Piaget, and the calculator. In M. H. Hoffmann, J. Lenhard, & F. Seeger (Eds.), Activity and Sign: Grounding Mathematics Education (pp. 137–152). Springer US. https://doi.org/10.1007/0-387-24270-8_12
Radford, L. (2010). The eye as a theoretician: Seeing structures in generalizing activities. For the Learning of Mathematics, 30(2), 2–7.
Radford, L. (2014). On the role of representations and artefacts in knowing and learning (pp. 405–422). https://doi.org/10.1007/s10649-013-9527-x
Radford, L., Bardini, C., Sabena, C., Diallo, P., & Simbagoye, A. (2005). On embodiment, artifacts, and signs: A semiotic-cultural perspective on mathematical thinking. In H. L. Chick & J. L. Vincent (Eds.), Proceedings of the 29 th Conference of the International Group for the Psychology of Mathematics Education (vol. 4, pp. 113–120). PME.
Reed, E. S., & Bril, B. (1996). The primacy of action in development. In M. L. Latash & M. T. Turvey (Eds.), Dexterity and its Development (pp. 431–451). Lawrence Erlbaum Associates.
Rietveld, E., Denys, D., & Van Westen, M. (2018). Ecological-enactive cognition as engaging with a field of relevant affordances: The skilled intentionality framework (SIF). In A. Newen, L. De Bruin, & S. Gallagher (Eds.), The Oxford Handbook of 4E Cognition. https://doi.org/10.1093/oxfordhb/9780198735410.013.3
Roth, W.-M. (2018). Birth of signs: A (Spinozist-Marxian) materialist approach. In N. Presmeg, L. Radford, W.-M. Roth, & G. Kadunz (Eds.), Signs of signification: Semiotics in mathematics education research (pp. 37–53). Springer International Publishing. https://doi.org/10.1007/978-3-319-70287-2_3
Shvarts, A., & Abrahamson, D. (in press). Intercorporeal dynamic functional system: A dual eye-tracking study of student-tutor collaboration. In L. Edwards & C. Krause (Eds.), The Body in Mathematics. Sense/Brill.
Shvarts, A., & Bakker, A. (2019). The early history of the scaffolding metaphor: Bernstein, Luria, Vygotsky, and before. Mind, Culture, and Activity, 26(1), 4–23. https://doi.org/10.1080/10749039.2019.1574306
Sinclair, N., & de Freitas, E. (2014). The haptic nature of gesture. Gesture, 14(3), 351–374. https://doi.org/10.1075/gest.14.3.04sin
Sinclair, N., & de Freitas, E. (2019). Body studies in mathematics education: diverse scales of mattering. ZDM-Mathematics Education, 51(2), 227–237. https://doi.org/10.1007/s11858-019-01052-w
Sinclair, N., & Heyd-Metzuyanim, E. (2014). Learning number with TouchCounts: The role of emotions and the body in mathematical communication. Technology, Knowledge and Learning, 19(1–2), 81–99. https://doi.org/10.1007/s10758-014-9212-x
Stepp, N., & Turvey, M. T. (2010). On strong anticipation. Cognitive Systems Research, 11(2), 148–164. https://doi.org/10.1016/j.cogsys.2009.03.003
Stoffregen, T. A., Yang, C.-M., Giveans, M. R., Flanagan, M., & Bardy, B. G. (2009). Movement in the perception of an affordance for wheelchair locomotion. Ecological Psychology, 21(1), 1–36. https://doi.org/10.1080/10407410802626001
Susac, A., Bubic, A., Kaponja, J., Planinic, M., & Palmovic, M. (2014). Eye movements reveal students’ strategies in simple equation solving. International Journal of Science and Mathematics Education, 12(3), 555–577. https://doi.org/10.1007/s10763-014-9514-4
Swidan, O., Sabena, C., & Arzarello, F. (2020). Disclosure of mathematical relationships with a digital tool: A three layer-model of meaning. Educational Studies in Mathematics, 103(1), 83–101. https://doi.org/10.1007/s10649-019-09926-2
Thompson, E., & Varela, F. J. (2001). Radical embodiment: Neural dynamics and consciousness. Trends in Cognitive Sciences, 5(10), 418–425.
Trouche, L. (2004). Managing the complexity of human/machine interactions in computerized learning environments: guiding students’ command process through instrumental orchestrations. International Journal of Computers for Mathematical Learning, 9(3), 281–307. https://doi.org/10.1007/s10758-004-3468-5
Trouche, L. (2020). Instrumentation in mathematics education. In S. Lerman (Ed.), Encyclopedia of Mathematics Education. Springer. https://doi.org/10.1007/978-94-007-4978-8_80
Turvey, M. T. (1977). Preliminaries to a theory of action with reference to vision. In R. Shaw & J. Bransford (Eds.), Perceiving, acting, and knowing: Toward an ecological psychology (pp. 211–265). Erlbaum.
Vergnaud, G. (1998). A comprehensive theory of representation for mathematics education. Journal of Mathematical Behavior, 17(2), 167–181. https://doi.org/10.1016/s0364-0213(99)80057-3
Verillon, P., & Rabardel, P. (1995). Cognition and artifacts: A contribution to the study of though in relation to instrumented activity. European Journal of Psychology of Education, 10, 77–101. https://doi.org/10.1007/BF03172796
Vygotsky, L. S. (1965). Psychology and localization of functions. Neuropsychologia, 3(4), 381–386. https://doi.org/10.1016/0028-3932(65)90011-4
Wenger, E. (1998). Communities of practice: Learning, meaning, and identity. Cambridge University Press.
White, T. (2019). Artifacts, agency and classroom activity: Materialist perspectives on mathematics education technology. Cognition and Instruction, 37(2), 169–200. https://doi.org/10.1080/07370008.2019.1578775
Wood, D., Bruner, J. S., & Ross, G. (1976). The role of tutoring in problem-solving. Journal of Child Psychology and Psychiatry, 17(2), 89–100. https://doi.org/10.1111/j.1469-7610.1976.tb00381.x
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Consent to participate
The participant has given his voluntary consent to participate in the study and gave permission to use the data in pseudonymized form in publications.
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Shvarts, A., Alberto, R., Bakker, A. et al. Embodied instrumentation in learning mathematics as the genesis of a body-artifact functional system. Educ Stud Math 107, 447–469 (2021). https://doi.org/10.1007/s10649-021-10053-0
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10649-021-10053-0