Skip to main content
SpringerLink
Log in

Contractivity-preserving explicit Hermite–Obrechkoff ODE solver of order 13

  • Original Article
  • Published:
Celestial Mechanics and Dynamical Astronomy Aims and scope Submit manuscript

Abstract

A new optimal, explicit, Hermite–Obrechkoff method of order 13, denoted by HO(13), that is contractivity-preserving (CP) and has nonnegative coefficients is constructed for solving nonstiff first-order initial value problems. Based on the CP conditions, the new 9-derivative HO(13) has maximum order 13. The new method usually requires significantly fewer function evaluations and significantly less CPU time than the Taylor method of order 13 and the Runge–Kutta method DP(8,7)13M to achieve the same global error when solving standard \(N\)-body problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  • Bancelin, D., Hestroffer, D., Thuillot, W.: Numerical integration of dynamical systems with Lie series. Celest. Mech. Dyn. Astron. 112, 221–234 (2012)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Barrio, R.: Performance of the Taylor series method for ODE/DAEs. Appl. Math. Comput. 163, 525–545 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  • Barrio, R.: Sensitivity analysis of ODEs/DAEs using the Taylor series method. SIAM J. Sci. Comput. 27, 1929–1947 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  • Barrio, R., Blesa, F., Lara, M.: VSVO formulation of the Taylor method for the numerical solution of ODEs. Comput. Math. Appl. 50, 93–111 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  • Binney, J., Tremaine, S.: Galactic Dynamics. Princeton University Press, Princeton (1987)

    MATH  Google Scholar 

  • Broucke, R.A.: Numerical integration of periodic orbits in the main problem of artificial satellite theory. Celest. Mech. Dyn. Astron. 58, 99–123 (1994)

    Article  MathSciNet  ADS  Google Scholar 

  • Butcher, J.C.: The Numerical Analysis of Ordinary Differential Equations: Runge–Kutta and General Linear Methods. Wiley, Chicester (1987)

    MATH  Google Scholar 

  • Chin, S.A.: Multi-product splitting and Runge–Kutta Nyström integrators. Celest. Mech. Dyn. Astron. 106, 391–406 (2010)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Corliss, G.F., Chang, Y.F.: Solving ordinary differential equations using Taylor series. ACM Trans. Math. Softw. 8, 114–144 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  • Deprit, A., Zahar, R.M.W.: Numerical integration of an orbit and its concomitant variations. Z. Angew. Math. Phys. 17, 425–430 (1966)

    Article  Google Scholar 

  • Enright, W.H., Pryce, J.D.: Algorithm 648: NSDTST and STDTST: routines for assessing the performance of IV solvers. ACM TOMS 13, 28–34 (1987)

    Article  Google Scholar 

  • Farr, W.M.: Variational integrators for almost-integrable systems. Celest. Mech. Dyn. Astron. 103, 105–118 (2009)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Gottlieb, S., Ketcheson, D.I., Shu, C.-W.: High order strong stability preserving time discretization. J. Sci. Comput. 38, 251–289 (2009). doi:10.1007/s10915-008-9239-z

    Article  MathSciNet  MATH  Google Scholar 

  • Gottlieb, S., Shu, C.-W., Tadmor, E.: Strong stability-preserving high-order time discretization methods. SIAM Rev. 43, 89–112 (2001)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Hairer, E., Nørsett, S.P., Wanner, G.: Solving Ordinary Differential Equations I. Nonstiff Problems. Springer, Berlin (1993)

    MATH  Google Scholar 

  • Hénon, H., Heiles, C.: The applicability of the third integral of motion. Some numerical examples. Astron. J. 69, 73–79 (1964)

    Article  ADS  Google Scholar 

  • Hoefkens, J., Berz, M., Makino, K.: Computing validated solutions of implicit differential equations. Adv. Comput. Math. 19, 231–253 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  • Huang, C.: Strong stability preserving hybrid methods. Appl. Numer. Math. 59, 891–904 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  • Hull, T.E., Enright, W.H., Fellen, B.M., Sedgwick, A.E.: Comparing numerical methods for ordinary differential equations. SIAM J. Numer. Anal. 9, 603–637 (1972)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Kennedy, C.A., Carpenter, M.K., Lewis, R.M.: Low-storage, explicit Runge–Kutta schemes for the compressible Navier–Stokes equations. Appl. Numer. Math. 35, 177–219 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  • Lambert, J.D.: Numerical Methods for Ordinary Differential Systems: The Initial Value Problem. Wiley, London (1991)

    MATH  Google Scholar 

  • Lara, M., Elipe, A., Palacios, M.: Automatic programming of recurrent power series. Math. Comput. Simul. 49, 351–362 (1999)

    Article  MathSciNet  Google Scholar 

  • Nedialkov, N.S., Jackson, K.R., Corliss, G.F.: Validated solutions of initial value problems for ordinary differential equations. Appl. Math. Comput. 105, 21–68 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  • Nguyen-Ba, T., Kengne, E., Vaillancourt, R.: One-step 4-stage Hermite–Birkhoff–Taylor ODE solver of order 12. Can. Appl. Math. Q. 16, 77–94 (2008)

    MathSciNet  MATH  Google Scholar 

  • Obrechkoff, N.: Neue Quadraturformeln. Abh. Preuss. Akad. Wiss. Math. Nat. Kl. 4, 1–20 (1940)

    Google Scholar 

  • Prince, P.J., Dormand, J.R.: High order embedded Runge–Kutta formulae. J. Comput. Appl. Math. 7, 67–75 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  • Rabe, E.: Determination and survey of periodic Trojan orbits in the restricted problem of three bodies. Astron. J. 66, 500–513 (1961)

    Article  MathSciNet  ADS  Google Scholar 

  • San Miguel, A.: Numerical integration of relativistic equations of motion for Earth satellites. Celest. Mech. Dyn. Astron. 103, 17–30 (2009)

    Google Scholar 

  • Sharp, P.W.: Numerical comparison of explicit Runge–Kutta pairs of orders four through eight. ACM Trans. Math. Softw. 17, 387–409 (1991)

    Article  MATH  Google Scholar 

  • Steffensen, J.F.: On the restricted problem of three bodies. Danske Vid. Selsk., Mat.-fys. Medd. 30, 1–17 (1956)

    MathSciNet  Google Scholar 

  • Szebehely, V.: Theory of Orbits: The Restricted Problem of Three Bodies. Academic Press, New York (1967)

    Google Scholar 

Download references

Acknowledgments

The authors thank the reviewers for their suggestions, particularly those related to the \(N\)-body problems. The authors also thank Martín Lara for supplying the authors with his programs and sharing his experience with it. This work was supported by the Natural Sciences and Engineering Research Council of Canada.

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, University of Ottawa, Ottawa, ON, K1N 6N5, Canada

    Truong Nguyen-Ba, Steven J. Desjardins & Rémi Vaillancourt

  2. Department of Mathematics, University of Auckland, Auckland, New Zealand

    Philip W. Sharp

Authors
  1. Truong Nguyen-Ba
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Steven J. Desjardins
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Philip W. Sharp
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Rémi Vaillancourt
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Philip W. Sharp.

Appendix: The HO(13) formula

Appendix: The HO(13) formula

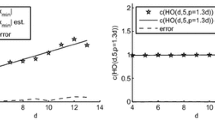
For the new HO(13) method \(c(\text {HO(13)})= 0.687\) (3dp), \(c_{\text {eff}}(\text {HO(13)})= 0.0763\) (4dp), the unscaled stability interval is \((-1.83,\ldots , 0)\) (2dp), and

$$\begin{aligned} y_{n+1}&= 4.5537344707090732 \,\text {e-}01 y_{n-1} + 5.4462655292909279 \,\text {e-}01 y_{n} \\&\quad +\,6.6273842336814770 \,\text {e-}01 \Delta t f_{n-1} + 7.9263502370275962 \,\text {e-}01 \Delta t f_{n} \\&\quad +\,3.6304031487037802 \,\text {e-}01 \Delta t^2 y_{n-1}^{(2)} + 5.7201138496231607 \,\text {e-}01 \Delta t^2 y_{n}^{(2)} \\&\quad +\,1.6194516630180894 \,\text {e-}01 \Delta t^3 y_{n-1}^{(3)} + 1.1228817806297973 \,\text {e-}01 \Delta t^3 y_{n}^{(3)} \\&\quad +\,5.1751955213728010 \,\text {e-}02 \Delta t^4 y_{n-1}^{(4)} + 6.1822230586295386 \,\text {e-}02 \Delta t^4 y_{n}^{(4)} \\&\quad +\,1.5800102293137556 \,\text {e-}02 \Delta t^5 y_{n-1}^{(5)} + 0.0 \, \Delta t^5 y_{n}^{(5)} \\&\quad +\,4.5713674152395474 \,\text {e-}03 \Delta t^6 y_{n-1}^{(6)} + 3.4961844977613780 \,\text {e-}03 \Delta t^6 y_{n}^{(6)} \\&\quad +\,9.3883945698842422 \,\text {e-}04 \Delta t^7 y_{n-1}^{(7)} + 3.7179156905590997 \,\text {e-}06 \Delta t^7 y_{n}^{(7)}\\&\quad +\,1.1501709391358726 \,\text {e-}04 \Delta t^8 y_{n-1}^{(8)} + 5.4812853003565492 \,\text {e-}06 \Delta t^8 y_{n}^{(8)}\\&\quad +\,6.6147276073533409 \,\text {e-}06 \Delta t^9 y_{n-1}^{(9)} + 8.4875255409530395 \,\text {e-}06 \Delta t^9 y_{n}^{(9)}. \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Cite this article

Nguyen-Ba, T., Desjardins, S.J., Sharp, P.W. et al. Contractivity-preserving explicit Hermite–Obrechkoff ODE solver of order 13. Celest Mech Dyn Astr 117, 423–434 (2013). https://doi.org/10.1007/s10569-013-9520-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10569-013-9520-9

Keywords

Search

Navigation