Non-integrability of the dumbbell and point mass problem

Abstract

This paper discusses a constrained gravitational three-body problem with two of the point masses separated by a massless inflexible rod to form a dumbbell. This problem is a simplification of a problem of a symmetric rigid body and a point mass, and has numerous applications in Celestial Mechanics and Astrodynamics. The non-integrability of this system is proven. This was achieved thanks to an analysis of variational equations along a certain particular solution and an investigation of their differential Galois group. Nowadays this approach is the most effective tool for study integrability of Hamiltonian and non-Hamiltonian systems.

1 Equations of motion, symmetries and reduction

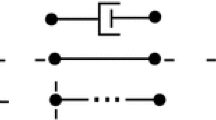
Considered is the gravitational three-body problem with a single constraint. Three point masses, $m_1,\,m_2$ and $m_3$ move in a plane under mutual gravitational interaction. Masses $m_2$ and $m_3$ are connected by a massless inflexible rod of length $l>0$ to form a dumbbell. A pictorial description of the problem is given in Fig. 1. Various celestial objects are considered to possess such bimodal mass distribution because do not have enough gravitational force to form their shape into a spherical object, e.g. some asteroids such as (51) Nemausa and (216) Kleopatra; meteorites, especially large irons or nucleus of some comets e.g. Comet Borrelly (for detailed references see Povenmire 2002).The dumbbell satellite has attracted the attention of scientists since the middle of 20th century because it is suitable for an investigation of the general properties of the rigid body motion in a gravity field and provides important features for tethered satellite systems. See section 5.9 in Celletti (2010) and some original articles and references therein (Celletti and Sidorenko 2008; Guirao et al. 2013; Khan and Goel 2011; Schechter 1964; Vera 2013). Moreover dumbbell can be considered as a simplified model of a large spacecraft or an orbital cable system equipped with an elevator (see e.g. Burov et al. 2012; Okunev 1969; Sanyal et al. 2005). In most of the above examples the restricted version of the problem is considered. That is the mass center of a dumbbell moves on a given orbit and its rotational motion does not disturb the orbital motion. In many cases, e.g., for some double asteroids, such approximation is not well justified. This is why we consider unrestricted problem. An unrestricted problem of a point and axially symmetric body whose gravity field is approximated by a dumbbell field is considered in Goździewski and Maciejewski (1999).

Fig. 1

A dumbbell and a point mass in the plane. Point $O$ denotes the origin of an inertial frame. Point $C$ is the system centre of masses. Axis $OX_1$ of the moving frame is always parallel to the dumbbell

The Lagrangian of the system has the form

$$\begin{aligned} L = \frac{1}{2} m_1\Vert {\dot{{\mathbf{r}}}}_{1}\Vert ^2 + \frac{1}{2} m_2 \Vert {\dot{{\mathbf{r}}}}_{2}\Vert ^2 + \frac{1}{2} m_3\Vert {\dot{{\mathbf{r}}}}_3\Vert ^2 - U({\mathbf{r}}_1,{\mathbf{r}}_2,{\mathbf{r}}_3), \end{aligned}$$

(1.1)

where ${\mathbf{r}}_1,\,{\mathbf{r}}_2$ and ${\mathbf{r}}_3$ are the position vectors of the respective masses, and

$$\begin{aligned} U({\mathbf{r}}_1,{\mathbf{r}}_2,{\mathbf{r}}_3) = - \frac{G m_1 m_2}{\Vert {\mathbf{r}}_1-{\mathbf{r}}_2\Vert } - \frac{G m_1 m_3}{\Vert {\mathbf{r}}_1-{\mathbf{r}}_3\Vert } - \frac{G m_2 m_3}{\Vert {\mathbf{r}}_2-{\mathbf{r}}_3\Vert }, \end{aligned}$$

(1.2)

is the potential. Let ${\mathbf{r}}_\mathrm{d }$ is the radius vector of the centre of mass of the dumbbell, and

$$\begin{aligned} {\mathbf{e}}{:}= \frac{{\mathbf{r}}_3-{\mathbf{r}}_2}{\Vert {\mathbf{r}}_3-{\mathbf{r}}_2 \Vert }, \end{aligned}$$

(1.3)

is a unit vector along the dumbbell. It follows that

$$\begin{aligned} {\mathbf{r}}_2&= {\mathbf{r}}_\mathrm d + \mu _3 l {\mathbf{e}}, \qquad {\dot{\mathbf{r}}}_2 = {\dot{\mathbf{r}}}_\mathrm d + \mu _3 l \dot{\mathbf{e}}, \end{aligned}$$

(1.4)

$$\begin{aligned} {\mathbf{r}}_3&= {\mathbf{r}}_\mathrm d - \mu _2 l {\mathbf{e}}, \qquad {\dot{\mathbf{r}}}_3 = {\dot{\mathbf{r}}}_\mathrm d - \mu _2 l \dot{\mathbf{e}}, \end{aligned}$$

(1.5)

where

$$\begin{aligned} \mu _2{:}= \frac{m_2}{m_\mathrm{d }}, \qquad \mu _3{:}= \frac{m_3}{m_\mathrm{d }}, \qquad m_\mathrm{d }{:}= m_2 + m_3. \end{aligned}$$

(1.6)

The system has five degrees of freedom. The configuration space of the point $m_1$ is $\mathbb R ^2$, and the configuration space of the dumbbell is $\mathbb R ^2\times \mathbb S ^{1}$. A configuration of the system is fully specified by ${\mathbf{r}}_1,\,{\mathbf{r}}_\mathrm{d }$, and ${\mathbf{e}}$. In these coordinates, the Lagrangian is as follows:

$$\begin{aligned} L = \frac{1}{2} m_1 ||{\dot{\mathbf{r}}}_1 ||^2 + \frac{1}{2} m_\mathrm{d }||{\dot{\mathbf{r}}}_\mathrm d ||^2 + \frac{1}{2}I ||{\dot{\mathbf{e}}} ||^{2} -\widetilde{U}({\mathbf{r}}_1, {\mathbf{r}}_\mathrm{d }, {\mathbf{e}}), \end{aligned}$$

(1.7)

where

$$\begin{aligned} I{:}= l^2 \frac{m_2m_3}{m_\mathrm{d }}, \qquad \widetilde{U}({\mathbf{r}}_1, {\mathbf{r}}_\mathrm{d }, {\mathbf{e}})= U({\mathbf{r}}_1, {\mathbf{r}}_\mathrm d + \mu _3 l {\mathbf{e}}, {\mathbf{r}}_\mathrm d - \mu _2 l {\mathbf{e}}). \end{aligned}$$

(1.8)

The system possesses natural symmetries that are exploited to reduce its dimension. Amongst these is translational symmetry. This is manifest by defining ${\mathbf{r}}{:}= {\mathbf{r}}_\mathrm{d }-{\mathbf{r}}_1$ as the vector between the mass $m_1$ and the dumbbell’s centre of mass. A configuration of the system can be thus described by ${\mathbf{r}},\,{\mathbf{e}}$, and ${\mathbf{r}}_\mathrm{s }$ defined by

$$\begin{aligned} m_\mathrm s {\mathbf{r}}_\mathrm{s }=m_1 {\mathbf{r}}_1 + m_\mathrm{d }{\mathbf{r}}_\mathrm{d }, \qquad m_\mathrm s {:}= m_1+m_2+m_3. \end{aligned}$$

(1.9)

As

$$\begin{aligned} {\mathbf{r}}_1= {\mathbf{r}}_\mathrm{s } -\frac{m_\mathrm{d }}{m_\mathrm{s }}{\mathbf{r}}, \quad {\mathbf{r}}_\mathrm{d }={\mathbf{r}}_\mathrm{s } +\frac{m_1}{m_\mathrm{s }}{\mathbf{r}}, \end{aligned}$$

(1.10)

the Lagrangian (1.7) is written in the following form:

$$\begin{aligned} L= \frac{1}{2} m_\mathrm{r } \Vert {\dot{\mathbf{r}}}\Vert ^2 + \frac{1}{2}I\Vert {\dot{\mathbf{e}}}\Vert ^2 + \frac{1}{2} m_\mathrm{s }\Vert {\dot{\mathbf{r}}}_\mathrm s \Vert ^2 - W({\mathbf{r}}, {\mathbf{e}}), \end{aligned}$$

(1.11)

where

$$\begin{aligned} m_\mathrm r {:}= \frac{m_1 m_\mathrm{d }}{m_\mathrm{s }}, \end{aligned}$$

(1.12)

is the reduced mass, and

$$\begin{aligned} W({\mathbf{r}},{\mathbf{e}})= - \frac{G m_1 m_2}{\Vert {\mathbf{r}}+\mu _3 l {\mathbf{e}}\Vert } - \frac{G m_1 m_3}{\Vert {\mathbf{r}}-\mu _2 l {\mathbf{e}}\Vert }. \end{aligned}$$

(1.13)

The components of ${\mathbf{r}}_\mathrm{s }$ are cyclic coordinates, and the motion of the centre of mass separates completely. Therefore, the term $m_\mathrm{s }\Vert {\dot{\mathbf{r}}}_\mathrm s \Vert ^2/2$ is removed from the Lagrangian (1.11).

It is convenient to introduce dimensionless variables, taking $l$ as the unit of length, $m_\mathrm{r }$ as the unit of mass, and

$$\begin{aligned} T{:}=\sqrt{\frac{l^3}{m_\mathrm{s }}}, \end{aligned}$$

(1.14)

as the unit of time. Setting $l=m_\mathrm{r }=T=1$, the Lagrangian (1.11) reduces to

$$\begin{aligned} L= \frac{1}{2} \Vert {\dot{\mathbf{r}}}\Vert ^2 + \frac{1}{2}I \Vert {\dot{\mathbf{e}}}\Vert ^2 - W({\mathbf{r}}, {\mathbf{e}}), \end{aligned}$$

(1.15)

where

$$\begin{aligned} W({\mathbf{r}},{\mathbf{e}})= - \frac{1}{\Vert \left( 1+\mu \right) {\mathbf{r}}+\mu {\mathbf{e}}\Vert } - \frac{\mu }{\Vert \left( 1+\mu \right) {\mathbf{r}}-{\mathbf{e}}\Vert }, \qquad \mu {:}=\frac{m_3}{m_2}. \end{aligned}$$

(1.16)

The reduced system still has a symmetry: it is invariant with respect to the natural action of the group $\mathrm SO (2,\mathbb R )$. This symmetry can be used to reduce the dimension of the configuration space by one. This is achieved by describing the dynamics in a rotating frame in which the dumbbell is at rest. The transformation from the inertial frame to the rotating frame is given by

$$\begin{aligned} {\mathbf{r}}= {\mathbf{A}}{\mathbf{R}}, \qquad {\mathbf{A}}\in \mathrm SO (2,\mathbb R ). \end{aligned}$$

(1.17)

An additional assumption, that ${\mathbf{e}}$ is parallel to $x$-axis of the rotating frame, allows for a coordinate representation of the transformation as follows:

$$\begin{aligned} {\mathbf{A}}= \left[ \begin{array}{ll} \cos \varphi &{} -\sin \varphi \\ \sin \varphi &{}\cos \varphi \end{array}\right] , \quad \text {and}\quad {\mathbf{e}}=[\cos \varphi ,\sin \varphi ]^T. \end{aligned}$$

(1.18)

Then

$$\begin{aligned} {\mathbf{e}}={\mathbf{A}}{\mathbf{E}}, \quad {\mathbf{E}}= [1,0]^T, \end{aligned}$$

(1.19)

and

$$\begin{aligned} \dot{\mathbf{e}}= \dot{\varphi }[-\sin \varphi , \cos \varphi ]^T , \qquad \dot{\mathbf{A}}= \dot{\varphi }{\mathbf{A}}{\mathbf{J}}, \qquad {\mathbf{J}}= \left[ \begin{array}{lr} 0 &{} -1 \\ 1 &{} 0 \end{array}\right] . \end{aligned}$$

(1.20)

The Lagrangian expressed in the coordinates ${\mathbf{R}}=[X_1, X_2]^T$, and $\varphi$ has the form

$$\begin{aligned} L = \frac{1}{2}{\Vert \dot{\mathbf{R}}\Vert }^2 +\dot{\varphi }{\dot{\mathbf{R}}}^T{\mathbf{J}}{\mathbf{R}}+ \frac{1}{2}{\dot{\varphi }}^2 \Vert {\mathbf{R}}\Vert ^{2} +\frac{1}{2}I {\dot{\varphi }}^2 - V({\mathbf{R}}), \end{aligned}$$

(1.21)

where

$$\begin{aligned} V({\mathbf{R}}){:}= - \frac{1}{\Vert \left( 1+\mu \right) {\mathbf{R}}+\mu {\mathbf{E}}\Vert } - \frac{\mu }{\Vert \left( 1+\mu \right) {\mathbf{R}}-{\mathbf{E}}\Vert }. \end{aligned}$$

(1.22)

The generalised momenta ${\mathbf{P}}{:}=\left[ P_1,P_2 \right] ^T$ and $P_{\varphi }$, are given by

$$\begin{aligned} {\mathbf{P}}{:}= \dfrac{\partial L }{\partial \dot{\mathbf{R}}}=\dot{\mathbf{R}}+\dot{\varphi }{\mathbf{J}}{\mathbf{R}}, \qquad P_{\varphi }{:}= \dfrac{\partial L }{\partial \dot{\varphi }}=\dot{\varphi }\left( \Vert {\mathbf{R}}\Vert ^2+ I\right) + {\dot{\mathbf{R}}}^T{\mathbf{J}}{\mathbf{R}}. \end{aligned}$$

(1.23)

Coordinate $\varphi$ is cyclic, so $P_{\varphi }$ is a first integral of the system. Thus, the Hamiltonian of the system is written as

$$\begin{aligned} H=\frac{1}{2}\Vert {\mathbf{P}}\Vert ^{2 }+\frac{1}{2}\alpha \left( \gamma - {\mathbf{P}}^T{\mathbf{J}}{\mathbf{R}}\right) ^2 +V({\mathbf{R}}), \end{aligned}$$

(1.24)

where $\alpha =1/I$, and $\gamma$ is a fixed value of the first integral $P_{\varphi }$ corresponding to the cyclic variable $\varphi$. Hence, the equations of motion have the form

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}{\mathbf{R}}&= {\mathbf{P}}-\alpha \left( \gamma -{\mathbf{P}}^T{\mathbf{J}}{\mathbf{R}}\right) {\mathbf{J}}{\mathbf{R}}, \nonumber \\ \frac{\mathrm{d } }{\mathrm{d }}{\mathbf{P}}&= - \alpha \left( \gamma -{\mathbf{P}}^T{\mathbf{J}}{\mathbf{R}}\right) {\mathbf{J}}{\mathbf{P}}- \left( 1+\mu \right) \frac{\left( 1+\mu \right) {\mathbf{R}}+\mu {\mathbf{E}}}{\Vert \left( 1+\mu \right) {\mathbf{R}}+\mu {\mathbf{E}}\Vert ^3} - \nonumber \\&\mu \left( 1+\mu \right) \frac{\left( 1+\mu \right) {\mathbf{R}}-{\mathbf{E}}}{\Vert \left( 1+\mu \right) {\mathbf{R}}-{\mathbf{E}}\Vert ^3 }. \end{aligned}$$

(1.25)

2 Problem

Numerical experiments suggest that the considered system is not integrable. The complex behaviour of the system is apparent from the Poincaré cross-section. For fixed values of the parameters and the energy the equations (1.25) are integrated numerically by the Bulirsch-Stoer method (Press et al. 1992). The cross-section plane is specified as $X_1=0$, and the cross direction is chosen $\dot{X}_1>0$. The coordinates of the cross-section are $(X_2,P_2)$. The cross-sections presented in the subsequent figures are obtained for parameters $\mu =1$ and $\alpha =\frac{4}{61}$.

Figure 2 is a cross-section corresponding to energy $e= -0.1$ and $\gamma =-1$. Chaotic behaviour is evident.Two cross-sections for $\gamma =0$ are shown in Figs. 3 and 4. Both of them attest to the non-integrability of the system. Let us remark here that all cross sections were done for negative energy values. For non-negative values of energy the energy levels are non-compact. This is why trajectories in such levels usually do not return to the cross section plane. In the best case investigating Poincaré cross-section we observe that a single trajectory intersects the cross plane along a spiral. No useful information about global properties of the system can be deduced.

Fig. 2

Cross-section for energy $e=-0.1$ and $\gamma =-1$

Fig. 3

Cross-section for $e=-0.2$ and $\gamma =0$

Fig. 4

Cross-section for $e=-0.15$ and $\gamma =0$

The problem is to prove the non-integrability of the system analytically. It is attractive to try using the differential Galois approach to the problem of integrability (see e.g. Morales Ruiz 1999). This method was applied successfully to many systems and in particular to various Celestial Mechanics problems such as e.g.: three body problem (Boucher and Weil 2003; Maciejewski and Przybylska 2011; see also Tsygvintsev 2001, 2003, 2007), satellite in geo-magnetic field (Boucher 2006; Maciejewski and Przybylska 2003), two-body problems in constant curvature spaces (Maciejewski and Przybylska 2003), some $n$-body problems (Combot 2012; Simon 2007; Tosel 1998, 1999), Sitnikov’s three-body problem (Morales Ruiz 1999), Hill’s problem (Morales-Ruiz et al. 2005), generalized two-fixed centres problem whose interaction potential is $V = ar^{2n}$ (Maciejewski and Przybylska 2004), generalized anisotropic Kepler problem (Arribas et al. 2003) and many others. However, as is explained in (Combot 2013), application of this theory directly to the system (1.25) is invalid as the Hamiltonian function (1.24) is not single-valued.

Proposed in this paper is a solution addressing this deficiency, based on an extension of the system into a larger phase space. Coordinates of these additional dimensions are denoted by $R_1$ and $R_2$. This approach further requires a definition of the time derivative consistent with system (1.25). Following this, coordinates $R_1$ and $R_2$ are considered as lengths of vectors

$$\begin{aligned} {\mathbf{R}}_1{:}= \left( 1+\mu \right) {\mathbf{R}}+\mu {\mathbf{E}}, \qquad {\mathbf{R}}_2{:}= \left( 1+\mu \right) {\mathbf{R}}-{\mathbf{E}}. \end{aligned}$$

(2.1)

That is, equations $G_1=G_2=0$, where polynomials $G_1, G_2 \in \mathbb C [X_1, X_2, R_1, R_2]$ given by

$$\begin{aligned} \left. \begin{aligned} G_1{:}=&R_1^2-\left[ \left( 1+\mu )X_1 +\mu \right) \right] ^2-\left( 1+\mu \right) ^2X^2_2=R_1^2-{\mathbf{R}}_1^T{\mathbf{R}}_1,\\ G_2{:}=&R_2^2-\left[ \left( 1+\mu )X_1 -1\right) \right] ^2-\left( 1+\mu \right) ^2X^2_2=R_2^2-{\mathbf{R}}_2^T{\mathbf{R}}_2. \end{aligned} \quad \right\} \end{aligned}$$

(2.2)

define $R_1$ and $R_2$ as algebraic functions of $(X_1,X_2)$.

The extended phase space is $\mathbb C ^6$ with coordinates ${\mathbf{Z}}{:}=\left[ X_1,X_2,P_1,P_2,R_1,R_2 \right]$. The Hamiltonian (1.24) expressed in these coordinates reads

$$\begin{aligned} K = K({\mathbf{Z}}){:}= \frac{1}{2}\Vert {\mathbf{P}}\Vert ^{2 }+\frac{1}{2}\alpha \left( \gamma - {\mathbf{P}}^T{\mathbf{J}}{\mathbf{R}}\right) ^2 -\frac{1}{R_1}-\frac{\mu }{R_2}. \end{aligned}$$

(2.3)

As

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}R_i= \frac{{\mathbf{R}}_i^T\dot{\mathbf{R}}_i}{R_i}=(1+\mu ) \frac{{\mathbf{R}}_i^T\dot{\mathbf{R}}}{R_i}, \qquad i=1,2, \end{aligned}$$

(2.4)

system (1.25) can be extended to the following one.

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}{\mathbf{Z}}= {\mathbf{U}}({\mathbf{Z}}), \qquad {\mathbf{U}}({\mathbf{Z}}){:}={\mathbf{J}}({\mathbf{Z}}) K'({\mathbf{Z}}), \qquad {\mathbf{J}}({\mathbf{Z}}){:}= \left[ \begin{array}{r@{\quad }l@{\quad }l} \mathbf{0}_{2} &{} {\mathbf{E}}_2 &{} \mathbf{0}_{2}\\ -{\mathbf{E}}_2 &{} \mathbf{0}_2 &{} -{\mathbf{A}}\\ \mathbf{0}_2 &{} {\mathbf{A}}^T &{} \mathbf{0}_2 \end{array}\right] , \end{aligned}$$

(2.5)

where

$$\begin{aligned} {\mathbf{A}}= (1+\mu )\left[ \frac{{\mathbf{R}}_1}{R_1}, \frac{{\mathbf{R}}_2}{R_2} \right] , \qquad K'({\mathbf{Z}}){:}=\left( \nabla _{{\mathbf{Z}}}K({\mathbf{Z}}) \right) ^T. \end{aligned}$$

(2.6)

It can be shown that matrix ${\mathbf{J}}({\mathbf{Z}})$ defines a Poisson structure on $\mathbb C ^6$. The corresponding Poisson bracket is given by the following formula

$$\begin{aligned} \left\{ F, G \right\} ({\mathbf{Z}})= F'({\mathbf{Z}})^T {\mathbf{J}}({\mathbf{Z}}) G'({\mathbf{Z}}), \end{aligned}$$

(2.7)

where $F$ and $G$ are two smooth functions. The Poisson structure given by ${\mathbf{J}}({\mathbf{Z}})$ is degenerated and has rank 4. It is demonstrable that polynomials $G_1$ and $G_2$ are Casimir functions of this structure, whose common levels are symplectic manifolds. In particular,

$$\begin{aligned} M^4_{\mu } {:}=\left\{ {{\mathbf{Z}}\in \mathbb C ^6}\;\vert \;\; {G_1({\mathbf{Z}})=G_2({\mathbf{Z}})=0} \,\right\} , \qquad \mu \in (0,1/2]. \end{aligned}$$

(2.8)

is a symplectic manifold. System (2.5), when restricted to $M^4_{\mu }$, is equivalent to (1.25).

In the setting thus described the main result of this paper can be formulated in the following theorem.

Theorem 2.1

For $\gamma =0$ and $\mu \in (0,1/2]$, system (2.5), when restricted to the symplectic leaf $M_{\mu }^{4}$, is not integrable in the Liouville sense with meromorphic first integrals.

3 Particular solution and variational equation

If $\gamma =0$, then Eq. (2.5) have an invariant algebraic manifold,

$$\begin{aligned} \mathcal{N }{:}=\left\{ {{\mathbf{Z}}\in \mathbb C ^6}\;\vert \;\; {X_2=P_2=0, \quad G_1({\mathbf{Z}})=G_2({\mathbf{Z}})=0} \,\right\} . \end{aligned}$$

(3.1)

On this manifold it can be taken that

$$\begin{aligned} R_1 = (1+\mu )X_1 +\mu , \qquad R_2 = (1+\mu )X_1 -1, \end{aligned}$$

(3.2)

so the system (2.5), restricted to $\mathcal{N }$, reduces to

$$\begin{aligned} \dot{X}=P,\qquad \dot{P}=-(\mu +1)\left( \dfrac{\mu }{\left[ \left( 1+\mu \right) X -1\right] ^2}+\dfrac{1}{\left[ \left( 1+\mu \right) X+\mu \right] ^{2}}\right) , \end{aligned}$$

(3.3)

where $(X,P){:}=(X_1,P_1)\in \mathbb C ^2$. This is a Hamiltonian system with one degree of freedom. The point and the dumbbell move in one line. Fixing the energy $e$ we have a particular solution $(X(t),P(t))$. Thus the manifold $\mathcal{N }$ is foliated by phase curves $\Gamma _{e}$ on energy levels $K_{|\mathcal{N }}=e$, that is

$$\begin{aligned} \Gamma _e \subset M_e{:}=\left\{ {(X,P)\in \mathbb C ^2}\;\vert \;\; {e=\frac{1}{2}P^2- \dfrac{\mu }{\left( 1+\mu \right) X -1}-\dfrac{1}{\left( 1+\mu \right) X+\mu }} \,\right\} . \end{aligned}$$

(3.4)

These curves are give parametrically $t\longmapsto (X(t),P(t))$ For a generic value of $e$ the level $M_e$ contains three phase curves. If $e$ is real, two or three of these levels have a non-empty intersection with the real part of the phase space. Depending on the real value of $e$, the point can move either between the end masses of the dumbbell or outside them. In further consideration it is assumed that $e\ge 0$, and the chosen phase curve $\Gamma _{e}$ contains the half-line $(1/(1+\mu ),\infty )\times \{0\}\subset \mathbb C ^2$. The other choices are equally good for the proof of non-integrability. We made such a choice because it describes real, physically meaningful solution. In the phase space $\mathbb C ^6$ this curve is given by

$$\begin{aligned} \varvec{\Gamma }_e{:}=\left\{ {{\mathbf{Z}}\in \mathcal{N }}\;\vert \;\; { (X_1,P_1)\in \Gamma _e} \,\right\} . \end{aligned}$$

(3.5)

Application of the Morales-Ramis theory necessitates the linearisation of Eq. (2.5) along $\Gamma _{e}$. It has the form

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}{\mathbf{z}}= {\mathbf{V}}{\mathbf{z}}, \qquad {\mathbf{V}}=\dfrac{\partial {\mathbf{U}} }{\partial {\mathbf{Z}}}({\mathbf{Z}}), \quad {\mathbf{Z}}\in \varvec{\Gamma }_e. \end{aligned}$$

(3.6)

This system has three first integrals

$$\begin{aligned} k({\mathbf{z}}){:}= \nabla K({\mathbf{Z}})\cdot {\mathbf{z}}, \qquad g_1({\mathbf{z}}){:}=\nabla G_1({\mathbf{Z}})\cdot {\mathbf{z}}, \qquad g_2({\mathbf{z}}){:}=\nabla G_2({\mathbf{Z}})\cdot {\mathbf{z}},\quad {\mathbf{Z}}\in \varvec{\Gamma }_e.\qquad \end{aligned}$$

(3.7)

On the level $g_1({\mathbf{z}})=g_2({\mathbf{z}})=0$, the following equalities are satisfied

$$\begin{aligned} R_1 r_1 = (1+\mu )\left[ (1+\mu )X_1 +\mu \right] x_1, \qquad R_2 r_2 = (1+\mu )\left[ (1+\mu )X_1 -1\right] x_1, \end{aligned}$$

(3.8)

where it is assumed that ${\mathbf{z}}=\left[ x_1, x_2, p_1, p_2, r_1, r_2\right] ^T$. On $\varvec{\Gamma }_e$, coordinates $(R_1,R_2)$ can be expressed by $X_1$,

$$\begin{aligned} R_1= (1+\mu )X_1 +\mu , \qquad R_2=(1+\mu )X_1 -1. \end{aligned}$$

(3.9)

Hence, on the level $g_1({\mathbf{z}})=g_2({\mathbf{z}})=0$, variables $(r_1, r_2)$ can be eliminated from the variational equations (3.6). The obtained reduced system of variational equations has the form

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}\left[ \begin{array}{l} u_1\\ v_1\\ u_2\\ v_2 \end{array}\right] = \left[ \begin{array}{llll} 0&{}1&{}0&{}0\\ A &{} 0&{}0&{} 0 \\ 0&{} 0 &{}-\alpha X P &{}1 +\alpha X^2\\ 0&{}0 &{}B&{}\alpha X P \end{array}\right] \left[ \begin{array}{l} u_1\\ v_1\\ u_2\\ v_2 \end{array}\right] , \end{aligned}$$

(3.10)

where

$$\begin{aligned} A&= 2(\mu +1)^2\left( \dfrac{\mu }{\left[ \left( 1+\mu \right) X-1\right] ^3}+\dfrac{1}{\left[ \left( 1+\mu \right) X+\mu \right] ^3}\right) ,\nonumber \\ B&= -\alpha P^2-\frac{1}{2}A, \end{aligned}$$

(3.11)

and $(X,P)\in \Gamma _{e}$. This system splits into two subsystems. Variables $(u_1,v_1)$ describe variations in the invariant plane $\mathcal{N }$. In fact, $(u_1,v_1)$ is a vector tangent to $\mathcal{N }$ at point $(X,P)$, hence the subsystem in variables $(u_1,v_1)$ is called tangential. The second subsystem, corresponding to variables $(u_2,v_2)$, yields the normal variational equations. The latter subsystem can be expressed by a single second-order equation,

$$\begin{aligned} \ddot{u}+a \dot{u}+bu=0, \end{aligned}$$

(3.12)

where $u=u_2$, and

$$\begin{aligned} a{:}=-\dfrac{2 \alpha X P}{1 + \alpha X^2 },\qquad b{:}=\dfrac{2 \alpha P^2}{1 + \alpha X^2 }+ \frac{1}{2} \left( 1+\alpha X^2\right) A. \end{aligned}$$

Changing the independent variable by

$$\begin{aligned} t\longrightarrow z=(1+\mu )X(t) \end{aligned}$$

(3.13)

transforms equation (3.12) into an equation with rational coefficients. This follows from

$$\begin{aligned} \frac{\mathrm{d } }{\mathrm{d }}&= \dot{z}\frac{\mathrm{d } }{ \mathrm{d }z},\qquad \frac{\mathrm{d }^2 }{\mathrm{d } t^2}=(\dot{z})^2\frac{\mathrm{d }^2 }{\mathrm{d }z^2}+\ddot{z} \frac{\mathrm{d } }{ \mathrm{d }z},\\ \dot{z}&= (1\!+\!\mu ) \dot{x}\!=\!(1+\mu ) p_1,\qquad (\dot{z})^2=(1+\mu )^2 p_1^2=2(1+\mu )^2\left( e+\dfrac{\mu }{z-1}+\dfrac{1}{z+\mu } \right) ,\\ \ddot{z}&= (1+\mu )\ddot{p}_1=-(\mu +1)^2\left( \dfrac{\mu }{(z-1)^2}+\dfrac{1}{(z+\mu )^2}\right) , \end{aligned}$$

where the energy integral

$$\begin{aligned} e=\frac{1}{2}{\dot{X}}^2- \dfrac{\mu }{\left( 1+\mu \right) X -1}-\dfrac{1}{\left( 1+\mu \right) X+\mu } \end{aligned}$$

has been used.

Resultantly, Eq. (3.12) transforms into

$$\begin{aligned} u''+p(z)u'+q(z)u=0, \end{aligned}$$

(3.14)

where the prime denotes the derivative with respect to $z$, and

$$\begin{aligned} p&= -\left[ (-1 + z)^2 (1 + z^2 (5 + 4 e z) \alpha ) + (2 + z (-4 + 3 z + (4 + z (-8 + 8 e (-1 + z)^2\right. \\&\left. + 5 z^2)) \alpha )) \mu + (1 + 3 z^2 + 2 z (2 e (-1 + z)^2 + z (-4 + 5 z)) \alpha ) \mu ^2 + (1 + z (4 + z - 4 \alpha \right. \\&\left. + 5 z \alpha )) \mu ^3 \!+\! 2 (1 \!+\! z) \mu ^4 + \mu ^5\right] /\left[ 2 (z-1) (z + \mu ) ((z-1) (1 + e z) + (e (z-1) + z) \mu \right. \\&\left. + \mu ^2) (z^2 \alpha + (1 + \mu )^2)\right] ,\\ q&= \left[ 4 (z-1)^2 \alpha (1 + \mu ) (z + \mu )^2 ((z-1) (1 + e z) + (e (z-1) + z) \mu + \mu ^2) + ((z-1)^3\right. \\&\left. + (1 + 3 (z-1) z) \mu + (3 z-1) \mu ^2 + \mu ^3) (z^2 \alpha + (1 + \mu )^2)^2\right] /\left[ 2 (z-1)^2 (1 + \mu )\right. \\&\left. \cdot (z + \mu )^2 ((z-1) (1 + e z) + (e (z-1) + z) \mu + \mu ^2) (z^2 \alpha + (1 + \mu )^2)\right] . \end{aligned}$$

Making the next change of variables

$$\begin{aligned} u = w \exp \left[ -\frac{1}{2} \int \nolimits _{z_0}^z p(s)\, ds \right] , \end{aligned}$$

(3.15)

(3.14) is transformed to the canonical form

$$\begin{aligned} w'' = r(z) w, \qquad r(z) = -q(z) + \frac{1}{2}p'(z) + \frac{1}{4}p(z)^2, \end{aligned}$$

(3.16)

with coefficients given as

$$\begin{aligned} r&= \dfrac{P}{M},\nonumber \\ M&= 16 (z-1)^2 \beta ^2 (z^2 + \beta ^2)^2 (z + \mu )^2 [(z-1) (1 + e z) + (e (-1 + z) + z) \mu + \mu ^2]^2,\nonumber \\ P&= \sum _{i=0}^{11}p_iz^{11-i}. \end{aligned}$$

(3.17)

Here $\beta ^2=(1+\mu )^2/\alpha$ and coefficients $p_i$ of $P$ are given in the Appendix A. This equation has seven singularities

$$\begin{aligned} z_1&= 0,\qquad z_2=-\mu ,\qquad z_{3,4}=\pm \mathrm{i }\beta ,\\ z_{5,6}&= \dfrac{e-1-(1 + e) \mu \pm \sqrt{(1 + \mu ) ((1 + e)^2 + (e-1)^2 \mu )}}{2e}, \end{aligned}$$

and $z_7=\infty$, providing energy is chosen such that

$$\begin{aligned}&e\not \in \left\{ \dfrac{(1 \pm \mathrm{i }\beta ) (\pm \mathrm{i }+ \beta \mp \mathrm{i }\mu ) (\beta \pm \mathrm{i }\mu ) (1 + \mu )}{(1 + \beta ^2) (\beta ^2 + \mu ^2)},\dfrac{(\sqrt{\mu }\pm \mathrm{i })^2}{\mu +1},\right. \nonumber \\&\qquad \quad \left. \dfrac{-\mu + \mu ^3 \pm \mathrm{i }(1 + \mu ^3 + \beta ^2 (1 + \mu ))|\beta |}{(1 + \beta ^2) (\beta ^2 + \mu ^2)} \right\} . \end{aligned}$$

(3.18)

Points $z_1,\ldots ,z_6$ are regular poles of second order and the order of infinity is 1 provided that $E (1 + \mu )\ne 0$. Here, the order of a singular point is defined as in (Kovacic 1986), see also Appendix B.

The differences of exponents at the singularities in $\mathbb C$ are as follows

$$\begin{aligned} \Delta _1=\dfrac{1}{2}\sqrt{1-\dfrac{8}{\beta ^2}}, \quad \Delta _2=\dfrac{1}{2}\sqrt{1-\dfrac{8\mu ^2}{\beta ^2}},\quad \Delta _3=\Delta _4=2,\quad \Delta _5=\Delta _6=\dfrac{1}{2}. \end{aligned}$$

(3.19)

It is evident that the differences of the exponents at singularities $z_3$ and $z_4$ are integer, and thus, in local solutions around these points, logarithmic terms may appear. Application of the method described in Appendix B verifies the presence of such terms. For singularity $z_3$, solutions of the indicial equation are $\alpha _1=3/2$ and $\alpha _2=-1/2$. The relevant one is $\alpha =\alpha _1=3/2$. The expansion of $r(z-z_3)^2$, according to (B.4), gives coefficients $r_0,r_1$, and $r_2$. Then $f_1,f_2$ and $g_1,g_2$ are determined from (B.6) and (B.11), respectively. Since $s=\alpha _1-\alpha _2=2$, the coefficient $g_2$, which multiplies the logarithm, must be found, and it is given as

$$\begin{aligned} g_2=-\dfrac{\mathrm{i }(1 + \mu ) ((\mathrm{i }+ \beta )^2 + \mu - 2 \mathrm{i }\beta \mu - \mu ^2)}{8 \beta (\mathrm{i }+ \beta ) (\beta - \mathrm{i }\mu ) (1 + e \beta ^2 + (e - \mu ) \mu - \mathrm{i }\beta (1 + e (-1 + \mu ) + \mu ))}. \end{aligned}$$

(3.20)

Examination of the real and imaginary part of $g_2$ yields the following conditions

$$\begin{aligned}&\beta (1 + \mu )[1 + \beta ^4 (1 + \mu ) + 2 \beta ^2 (-1 + \mu ) (-1 - 2 e \mu + \mu ^2) \\&\quad + \mu (-1 - 2 e (-1 + \mu ) (1 + \mu ^2) + \mu (-1 + \mu (-1 + (-1 + \mu ) \mu )))]=0,\\&(1 + \mu )[\mu (1 + 3 \beta ^2 + (-1 + \mu ) \mu ) (-1 + \mu ^2) + e (\beta ^6 + \mu ^2 (-1 + \mu - \mu ^2)\\&\quad + \beta ^4 (2 + \mu (-3 + 2 \mu )) + \beta ^2 (1 + \mu (-1 + \mu (-1 + (-1 + \mu ) \mu ))))]=0. \end{aligned}$$

This system has two solutions satisfying $\mu >0,\,\beta ^2>0$ and $e\in \mathbb R$

$$\begin{aligned} \beta ^2=-1 + \mu - \mu ^2,\,\, e=\dfrac{1 + \mu }{1 - \mu }\quad \text {and}\quad \beta ^2=\mu =1. \end{aligned}$$

(3.21)

The condition precluding a logarithm in a local solution around $z_4$ is $g_2^*=0$, where ${}^*$ denotes complex conjugation. The corresponding solutions are the same as those given previously.

In the first solution in (3.21), condition

$$\begin{aligned} \beta ^2=-1 + \mu - \mu ^2, \end{aligned}$$

(3.22)

gives

$$\begin{aligned} \dfrac{m_0}{m_1}=-\dfrac{\mu (\mu +1)}{\mu ^2+1}\le 0, \end{aligned}$$

with the only non-negative solution $m_1=0$. The second solution in (3.21) also yields $m_1=0$ only.

If conditions (3.21) are not satisfied, the two linearly-independent local solutions $w_1$ and $w_2$ of (3.16) in a neighbourhood of $z_{*}=z_{3}$ or $z_{*}=z_{4}$ have the following forms

$$\begin{aligned} w_1(z) = (z - z_{*})^{\alpha } f(z), \qquad w_2(z)=w_1(z)\ln (z-z_{*})+(z-z_{*})^{\alpha -2} h(z), \end{aligned}$$

(3.23)

where $f(z)$ and $h(z)$ are holomorphic at $z_{*}$ and $f(z_{*})\ne 0$. The local monodromy matrix, which corresponds to the continuation of the matrix of fundamental solutions along a small loop encircling $z_{*}$ counter-clockwise, gives rise to a triangular monodromy matrix,

$$\begin{aligned} \left[ \begin{array}{ll} -1&{}-2\pi \mathrm{i }\\ 0&{}-1 \end{array}\right] , \end{aligned}$$

for details, see Maciejewski and Przybylska (2002). A subgroup of $\mathrm SL (2,\mathbb C )$ generated by a triangular non-diagonalizable matrix is not finite, and thus the differential Galois group is not finite either. Moreover, the differential Galois group $\mathcal{G }$ of this equation is not any subgroup of the dihedral group because such subgroups contain only diagonalizable matrices. Thus, $\mathcal{G }$ is either the full triangular group or $\mathrm SL (2, \mathbb C )$. But since the order of infinity is one, the necessary condition that $\mathcal{G }$ is the full triangular group is not satisfied (see Lemma B.2). This means that the differential Galois group of (3.16) is $\mathrm SL (2,\mathbb C )$, with a non-Abelian identity component equal to the whole group $\mathrm SL (2,\mathbb C )$.

Regarding exceptional values of energies given in (3.18): the condition that the first one is real gives $\beta (1 + \mu ^3 + \beta ^2 (1 + \mu ))=0$, which further suggests the equality (3.22). Its only physical solution is $m_1=0$. The second expression in (3.18) is never real and the third one is real when (3.22) holds only.

Appendices

Appendices

1.1 A. Coefficients of polynomial P in Eq. (3.17)

Explicit form of coefficients $p_i$ in $r$ given by (3.17) are as follows.

$$\begin{aligned} p_0=&-8 e (1 + \mu ),\qquad p_1=-8 (1 + \mu ) (1 + 4 e (-1 + \mu ) + \mu )],\nonumber \\ p_2=&-8 (1 + \mu ) (-4 + 4 \mu ^2 + e (6 + \beta ^2 + 2 \mu (-5 + 3 \mu ))),\nonumber \\ p_3=&-48 e^2 \beta ^4 - 16 e (-1 + \mu ^2) (2 + 2 \beta ^2 + \mu (-3 + 2 \mu )) - (1 + \mu )^2 (11 \beta ^2\nonumber \\&\quad + 24 (2 + \mu (-3 + 2 \mu ))),\nonumber \\ p_4=&-4 (48 e^2 \beta ^4 (-1 + \mu ) + (-1 + \mu ) (1 + \mu )^2 (8 + 11 \beta ^2 - 6 \mu + 8 \mu ^2)\nonumber \\&\quad +2 e (1 + \mu ) (1 + 12 \beta ^4 + \beta ^2 (6 + \mu (-7 + 6 \mu )) + \mu (-5 + \mu (5 + (-5 + \mu ) \mu )))),\nonumber \\ p_5=&-2 (-4 (1 + (e - \mu ) \mu ) (-1 + \mu ^4) + 3 \beta ^4 (9 (1 + \mu )^2 + 64 e (-1 + \mu ^2)\nonumber \\&\quad +16 e^2 (3 \!+ \!\mu (-8 \!+ \!3 \mu ))) \!+\! \beta ^2 (16 e (-1 + \mu ^4) \!+\! 3 (1 \!+\! \mu )^2 (11 \!+\! \mu (-13 \!+\! 11 \mu )))),\nonumber \\ p_6=&-4 \beta ^2 (48 e^2 \beta ^2 (\mu -1) (1 + ( \mu -5) \mu ) + (\mu -1) (1 + \mu )^2 (11 +54 \beta ^2\nonumber \\&\quad + \mu (3 + 11 \mu )) + 2 e (1 + \mu ) (1 + 3 \beta ^2 (24 + \mu (-59 +24 \mu ))\nonumber \\&\quad + \mu (-1 + \mu (-5 + (\mu -1)\mu )))),\nonumber \\ p_7=&-\beta ^2 (11 + 3 \beta ^4 (1 + \mu )^2 + 12 \beta ^2 (4 e (-8 + 33 \mu - 33 \mu ^3 + 8 \mu ^4) + (1 + \mu )^2 (27\nonumber \\&\quad + \mu (-59 + 27 \mu )) + 4 e^2 (1 + (-2 + \mu ) \mu (8 + (-14 + \mu ) \mu ))) + \mu (32 + \mu (-42 \mu \nonumber \\&\quad + \mu ^3 (32 + 11 \mu ) -32 e (-1 + \mu ^2)))),\nonumber \\ p_8=&-4 \beta ^2 (3 \beta ^4 (-1 + \mu ) (1 + \mu )^2 - 2 (1 + (e - \mu ) \mu ) (\mu + \mu ^4)\nonumber \\&\quad + 2 \beta ^2 (-24 e^2 (-1 + \mu ) \mu (1 + (-5 + \mu ) \mu ) + 27 (-1 + \mu ) (1 + \mu )^2 (1 + (-3 + \mu ) \mu )\nonumber \\&\quad + 2 e (1 + \mu ) (6 + \mu (-76 + \mu (157 - 76 \mu + 6 \mu ^2))))),\nonumber \\ p_9=&-2 \beta ^4 (27 +3 \beta ^2 (1 + \mu )^2 (3 + \mu (-5 + 3 \mu )) + \mu (-176 - 140 e (-1 + 4 \mu - 4 \mu ^3\nonumber \\&\quad + \mu ^4) +48 e^2 \mu (3 + \mu (-8 + 3 \mu )) + \mu (4 + \mu (414 + \mu (4 + \mu (-176 + 27 \mu )))))),\nonumber \\ p_{10}=&-4 \beta ^4 (3 \beta ^2 (-1 + \mu ^2 - \mu ^3 + \mu ^5) - 2 \mu (1 + (e - \mu ) \mu ) (-11 + \mu (24 \nonumber \\&\quad + 24 e (\mu -1) + (24 - 11 \mu ) \mu ))),\nonumber \\ p_{11}=&-3 \beta ^4 (16 \mu ^2 (1 + (e - \mu ) \mu )^2 + \beta ^2 (1 + \mu ^3)^2). \end{aligned}$$

(A.1)

B. Second-order differential equation in reduced form with rational coefficients and its differential Galois group

Consider the second-order linear differential equation in reduced form with rational coefficient

$$\begin{aligned} w'' =r(z)w,\qquad r(z)\in \mathbb C (z). \end{aligned}$$

(B.1)

A point $z=c\in \mathbb C$ is a singular point of this equation if it is a pole of $r(z)$. A singular point is a regular point if at this point $(z-c)^2r(z)$ is holomorphic.

Assume that $c$ is a regular point and look for local solutions of this equation in a neighbourhood of this point. For simplicity, assume that $c=0$. Look for a solution of the following form (see e.g. Whittaker and Watson 1935),

$$\begin{aligned} w=z^{\alpha }f(z),\qquad f(z)=1+\sum _{i=1}^{\infty }f_i z^i, \end{aligned}$$

(B.2)

where $\alpha ,f_i$ for $i\in \mathbb N$ are constants to be determined. Substituting in (B.1) yields

$$\begin{aligned} z^{\alpha -2}\left[ \alpha (\alpha -1)+\sum _{i=1}^{\infty } (\alpha +i)(\alpha +i-1)f_iz^i\right] -r(z)z^{\alpha } \left( 1+\sum _{i=1}^{\infty }f_iz^i\right) =0. \end{aligned}$$

(B.3)

Multiplying by $z^2$, apply Taylor’s formula for the analytic function $z^2r(z)$

$$\begin{aligned} z^2r(z)=r_0+\sum _{i=1}^{\infty } r_iz^i, \end{aligned}$$

(B.4)

and equating to zero the coefficients of successive powers of $t$, obtain the sequence of equations determining unknown coefficients in series (B.2). The first of these equations, called the indicial equation and determining $\alpha$, has the following form

$$\begin{aligned} \alpha (\alpha -1)-r_0=0. \end{aligned}$$

(B.5)

From the remaining equations $f_i$ is evaluated.

$$\begin{aligned} f_1=\dfrac{r_1}{2\alpha },\qquad f_i=\dfrac{1}{i(i+2\alpha -1)}\left( \sum _{j=1}^{i-1} r_jf_{i-j}+r_i\right) ,\quad \text {for}\quad i\ge 2. \end{aligned}$$

(B.6)

Let $\alpha _1$ and $\alpha _2$ be the roots of the indical equation. If the difference $s=\alpha _1-\alpha _2$ is not an integer, then Eq. (B.1) has two local solutions of the form

$$\begin{aligned} w_1=z^{\alpha _1}\left( 1+\sum _{i=1}^{\infty }f_i z^i\right) , \qquad w_2=z^{\alpha _2}\left( 1+\sum _{i=1}^{\infty }f_i' z^i\right) . \end{aligned}$$

(B.7)

In the case where $s$ is a positive integer or zero, the series $w_2$ may not exist or coincide with $w_1$, and a second independent solution must be constructed (Whittaker and Watson 1935) as

$$\begin{aligned} w_2(z)=w_1(z)\int ^z\dfrac{\text{ d }x}{w_1(x)^2}= w_1(z)\int ^zz^{-2\alpha _1}\dfrac{\text{ d }x}{f(x)^2}= w_1(z)\int ^zz^{-s-1}\dfrac{\text{ d }x}{f(x)^2}. \end{aligned}$$

(B.8)

In the last equality the relation $2\alpha _1=s+1$ is applied.

Denoting

$$\begin{aligned} \dfrac{1}{f(z)^2}=1+\sum _{i=1}^{\infty }g_iz^i, \end{aligned}$$

(B.9)

the second solution is written as

$$\begin{aligned} w_2(z)=w_1(z)g_s\ln z+z^{\alpha _2}\left( -\dfrac{1}{s}+\sum _{i=1}^{\infty }h_it^i\right) , \end{aligned}$$

(B.10)

where $h_i$ are constants.

Coefficients $g_i$ are given by

$$\begin{aligned} g_1&=-2f_1,\qquad g_2=-2f_2-2f_1g_1-f_1^2=-2f_2+3f_1^2, \end{aligned}$$

(B.11)

and

$$\begin{aligned} g_i&=-2f_i-\sum \limits _{j=1}^{i-1}(2g_{i-j}+ f_{i-j})f_j-\sum \limits _{j=1}^{i-2}f_j\sum \limits _{k=1}^{ i-j-1} f_kg_{i-j-k}, \end{aligned}$$

(B.12)

for $i\ge 3$, while coefficients $h_i$ are defined as follows

$$\begin{aligned} h_i=\left\{ \begin{array}{ll} -\dfrac{f_1}{s}-\dfrac{g_1}{s-1}&{} \quad \text {for}\quad i=1,\\ -\dfrac{f_i}{s}-\dfrac{g_i}{s-i}-\sum \limits _{j=1}^ {i-1}\dfrac{g_j}{s-j}f_{i-j} &{} \quad \text {for}\quad 1<i<s,\\ -\dfrac{f_s}{s}&{} \quad \text {for}\quad i=s,\\ -\dfrac{f_{s+1}}{s}+g_{s+1}-\sum \limits _{j=1}^{s-1} \dfrac{g_j}{s-j}f_{s+1-j}&{} \quad \text {for}\quad i=s+1,\\ -\dfrac{f_i}{s}+\dfrac{g_i}{i-s}-\sum \limits _{j=1}^{s-1} \dfrac{g_j}{s-j}f_{i-j}+ \sum \limits _{j=s+1}^{i-1}\dfrac{g_j}{j-s}f_{i-j}&{} \quad \text {for}\quad i\ge s+2. \end{array}\right. \end{aligned}$$

(B.13)

The differential Galois group of Eq. (B.1) is a subgroup of $\mathrm SL (2,\mathbb C )$. Classification of these subgroups is well known. The following lemma shows that the differential Galois group determines the form of solutions (see e.g. Kovacic 1986; Morales Ruiz 1999).

Lemma B.1

Let $\mathcal{G }$ be the differential Galois group of equation (3.16). Then one of four cases may occur.

• Case I $\mathcal{G }$ is conjugate to a subgroup of the triangular group,

  $$\begin{aligned} \mathcal{T }= \left\{ \left[ \begin{array}{ll} a &{} b\\ 0 &{} a^{-1} \end{array}\right] \; \biggl | \; a\in \mathbb C ^{*}, b\in \mathbb C \right\} \, ; \end{aligned}$$

  in this case equation (3.16) has an exponential solution of the form $y=P\exp \int \omega$, where $P\in \mathbb C [z]$ and $\omega \in \mathbb C (z)$.

• Case II $\mathcal{G }$ is conjugate to a subgroup of

  $$\begin{aligned} \mathcal{D }^\dag = \left\{ \left[ \begin{array}{ll} c &{} 0\\ 0 &{} c^{-1} \end{array}\right] \; \biggl | \; c\in \mathbb C ^*\right\} \cup \left\{ \left[ \begin{array}{ll} 0 &{} c\\ -c^{-1} &{} 0\end{array}\right] \; \biggl | \; c\in \mathbb C ^*\right\} \, ; \end{aligned}$$

  in this case equation (3.16) has a solution of the form $y=\exp \int \omega$, where $\omega$ is algebraic over $\mathbb C (z)$ of degree 2,

• Case III $\mathcal{G }$ is primitive and finite; in this case all solutions of Eq. (3.16) are algebraic, thus $y=\exp \int \omega$, where $\omega$ belongs to an algebraic extension of $\mathbb{C }(z)$ of degree $n=4,6$ or 12.

• Case IV $\mathcal{G }= \mathrm SL (2,\mathbb C )$ and Eq. (3.16) has no Liouvillian solution.

Kovacic (1986) formulated the necessary conditions for the respective cases from Lemma B.1 to hold.

Write $r(z)\in \mathbb C (z)$ in the form

$$\begin{aligned} r(z) = \frac{s(z)}{t(z)}, \qquad s(z),\, t(z) \in \mathbb C [z], \end{aligned}$$

where $s(z)$ and $t(z)$ are relatively prime polynomials and $t(z)$ is monic. The roots of $t(z)$ are poles of $r(z)$. Denote $\Sigma '{:}= \{c\in \mathbb C \,\vert \, t(c) =0 \}$ and $\Sigma {:}=\Sigma '\cup \{\infty \}$. The order $\mathrm ord (c)$ of $c\in \Sigma '$ is equal to the multiplicity of $c$ as a root of $t(z)$, the order of infinity is defined by

$$\begin{aligned} \mathrm ord (\infty ){:}=\deg t - \deg s. \end{aligned}$$

Lemma B.2

The necessary conditions for the respective cases in Lemma B.1 are the following.

• Case I. Every pole of $r$ must have even order or else have order 1. The order of $r$ at $\infty$ must be even or else be greater than 2.

• Case II. $r$ must have at least one pole that either has odd order greater than 2 or else has order 2.

• Case III. The order of a pole of $r$ cannot exceed 2 and the order of $r$ at $\infty$ must be at least 2. If the partial-fraction expansion of $r$ is

  $$\begin{aligned} r(z)=\sum _{i}\dfrac{a_i}{(z-c_i)^2}+ \sum _{j}\dfrac{b_j}{z-d_j}, \end{aligned}$$

  (B.14)

  then $\Delta _i=\sqrt{1+4a_i}\in \mathbb Q$, for each $i,\,\sum _{j}b_j=0$ and if

  $$\begin{aligned} g=\sum _ia_i+\sum _jb_jd_j, \end{aligned}$$

  then $\sqrt{1+4g}\in \mathbb Q$.