Efficient Mesh Updating Scheme for the ALE Corotational Formulation of an Arbitrarily Curved Beam

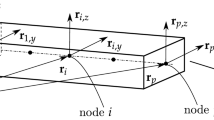
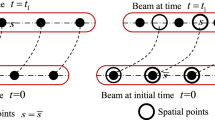
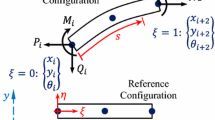
Abstract

This paper presents an efficient mesh updating scheme (MUS) for the arbitrary Lagrangian–Eulerian (ALE) formulation of an arbitrarily curved beam based on the corotational method. By discretizing the beam using both Lagrangian elements and ALE elements, the proposed MUS can take full advantage of the simple expression form of the Lagrangian formulation and the accurate moving-load description of the ALE node. The deleting-node and adding-node procedures of the MUS can avoid the negative influence of the variation of the ALE element length on the element accuracy and stiffness matrix singularity. In contrast to the adding-node procedure for Lagrangian elements, interpolation cannot be used directly. Inserting a Lagrangian node in an ALE element is investigated, and the displacement, velocity, and acceleration of the newly added node are evaluated accurately based on the corotational method. Three examples are investigated to verify the validity, computational accuracy and computational efficiency of the proposed MUS by comparing the results of the MUS with those from literature that utilized traditional ALE formulation. These examples show that the proposed MUS has significant advantages in terms of computational time and computer memory.

Appendix A

This section is devoted to the derivation of ${{\varvec{H}}}_{1}$, ${{\varvec{H}}}_{2}$, ${{\varvec{H}}}_{3}$, ${H}_{4}$, ${\dot{{\varvec{H}}}}_{1}$, ${\dot{{\varvec{H}}}}_{2}$, ${\dot{{\varvec{H}}}}_{3}$ and ${\dot{H}}_{4}$. Investigate first the variation of $\overline{{\varvec{q}} }$. Considering the approximation in Eq. (11) and using Eqs. (2), (3) and (8), the variation of ${l}_{\mathrm{c}}$ can be obtained as

$$\updelta {l}_{\mathrm{c}}={{\varvec{a}}}^{\mathrm{T}}\left({\varvec{E}}\updelta {\varvec{q}}+{{\varvec{a}}}_{0}{\dot{l}}_{s}\updelta t\right), {\varvec{E}}=\left[\begin{array}{cccc}-{\varvec{I}}& {0}_{2\times 1}& {\varvec{I}}& {0}_{2\times 1}\end{array}\right]$$

(A1)

where ${\varvec{I}}$ denotes a $2\times 2$ identity matrix. Then, from Eq. (5), the variation of $\overline{u }$ can be obtained as

$$\updelta \overline{u }={{\varvec{a}}}^{\mathrm{T}}{\varvec{E}}\updelta {\varvec{q}}+\left({{\varvec{a}}}^{\mathrm{T}}-{{\varvec{a}}}_{0}^{\mathrm{T}}\right){{\varvec{a}}}_{0}{\dot{l}}_{s}\updelta t$$

(A2)

Using Eqs. (2), (3) and (A1), the variations of ${\varvec{a}}$ and $\beta$ can be expressed as

$$\updelta {\varvec{a}}={{\varvec{G}}}_{1}\updelta {\varvec{q}}+{{\varvec{G}}}_{2}\updelta t$$

(A3)

$$\updelta \beta ={{\varvec{G}}}_{3}\updelta {\varvec{q}}+{G}_{4}\updelta t$$

(A4)

with

$$\begin{aligned}{{\varvec{G}}}_{1}&=\frac{1}{{l}_{\mathrm{c}}}\left({\varvec{I}}-{\varvec{a}}{{\varvec{a}}}^{\mathrm{T}}\right){\varvec{E}},{{\varvec{G}}}_{2}=\frac{1}{{l}_{\mathrm{c}}}\left({\varvec{I}}-{\varvec{a}}{{\varvec{a}}}^{\mathrm{T}}\right){{\varvec{a}}}_{0}{\dot{l}}_{s},\\ {{\varvec{G}}}_{3}&=\frac{1}{{l}_{\mathrm{c}}}{{\varvec{b}}}^{\mathrm{T}}{\varvec{E}},{G}_{4}=\frac{1}{{l}_{\mathrm{c}}}{{\varvec{b}}}^{\mathrm{T}}{{\varvec{a}}}_{0}{\dot{l}}_{s}\end{aligned}$$

(A5)

Taking the variation of $\overline{{\varvec{q}} }$ and using Eqs. (4), (5), (11) and (A2)–(A4), one obtains

$$\updelta \overline{{\varvec{q}} }={{\varvec{B}}}_{1}\updelta {\varvec{q}}+{{\varvec{B}}}_{2}\updelta t$$

(A6)

with

$$\begin{aligned}{{\varvec{B}}}_{1}&=\left[\begin{array}{cccc}{0}_{1\times 2}& 0& {0}_{1\times 2}& 0\\ {0}_{1\times 2}& 1& {0}_{1\times 2}& 0\\ {0}_{1\times 2}& 0& {0}_{1\times 2}& 1\end{array}\right]-\left[\begin{array}{c}{{\varvec{a}}}^{\mathrm{T}}{\varvec{E}}\\ -{{\varvec{G}}}_{3}\\ -{{\varvec{G}}}_{3}\end{array}\right], \\ {{\varvec{B}}}_{2}&=\left[\begin{array}{c}\left({{\varvec{a}}}^{\mathrm{T}}-{{\varvec{a}}}_{0}^{\mathrm{T}}\right){{\varvec{a}}}_{0}{\dot{l}}_{s}\\ -{G}_{4}\\ -{G}_{4}\end{array}\right]\end{aligned}$$

(A7)

Using Eqs. (2), (10), and (A4), the time derivative of ${{\varvec{R}}}_{\mathrm{r}}$ can be expressed as

$${\dot{{\varvec{R}}}}_{\mathrm{r}}={\varvec{R}}{{\varvec{R}}}_{\mathrm{r}}\left({{\varvec{G}}}_{3}\dot{{\varvec{q}}}+{G}_{4}\right)$$

(A8)

Taking the time derivative of Eq. (9) and using Eqs. (8), (11), (A6) and (A8), the terms ${{\varvec{H}}}_{1}$ and ${{\varvec{H}}}_{2}$ in Eq. (13) can be obtained as

$${{\varvec{H}}}_{1}=\left[\begin{array}{cc}{\varvec{I}}& {0}_{2\times 5}\end{array}\right]+\chi {\varvec{R}}{{\varvec{R}}}_{\mathrm{r}}{{\varvec{e}}}_{1}{{\varvec{G}}}_{3}+{\varvec{R}}{{\varvec{R}}}_{\mathrm{r}}{{\varvec{N}}}_{1}\overline{{\varvec{q}}}{{\varvec{G}} }_{3}+{{\varvec{R}}}_{\mathrm{r}}{{\varvec{N}}}_{1}{{\varvec{B}}}_{1}$$

(A9)

$$\begin{aligned}{{\varvec{H}}}_{2}&={\dot{s}}_{1}{{\varvec{a}}}_{0}-{\dot{s}}_{1}{{\varvec{R}}}_{\mathrm{r}}{{\varvec{e}}}_{1}+\chi {\varvec{R}}{{\varvec{R}}}_{\mathrm{r}}{{\varvec{e}}}_{1}{G}_{4}\\ & \quad +{\varvec{R}}{{\varvec{R}}}_{\mathrm{r}}{{\varvec{N}}}_{1}\overline{{\varvec{q}}}{G }_{4}+{{\varvec{R}}}_{\mathrm{r}}{\dot{{\varvec{N}}}}_{1}\overline{{\varvec{q}} }+{{\varvec{R}}}_{\mathrm{r}}{{\varvec{N}}}_{1}{{\varvec{B}}}_{2}\end{aligned}$$

(A10)

where $\dot{\overline{{\varvec{q}}} }$ can be obtained from (A6). Further, take the time derivative of ${{\varvec{H}}}_{1}$ and ${{\varvec{H}}}_{2}$, one obtains

$$ \begin{aligned} \dot{\user2{H}}_{1} & = - \dot{s}_{1} {\varvec{RR}}_{{\text{r}}} {\varvec{e}}_{1} {\varvec{G}}_{3} + \chi \user2{R\dot{R}}_{{\text{r}}} {\varvec{e}}_{1} {\varvec{G}}_{3} + \chi {\varvec{RR}}_{{\text{r}}} {\varvec{e}}_{1} \dot{\user2{G}}_{3}\\& \quad + \user2{R\dot{R}}_{{\text{r}}} {\varvec{N}}_{1} \overline{\user2{q}}\user2{G}_{3} + {\varvec{RR}}_{{\text{r}}} \dot{\user2{N}}_{1} \overline{\user2{q}}\user2{G}_{3} + {\varvec{RR}}_{{\text{r}}} {\varvec{N}}_{1} \user2{\dot{\overline{q}}G}_{3} \\ & \quad + {\varvec{RR}}_{{\text{r}}} {\varvec{N}}_{1} \user2{\overline{q}\dot{G}}_{3} + \dot{\user2{R}}_{{\text{r}}} {\varvec{N}}_{1} {\varvec{B}}_{1} + {\varvec{R}}_{{\text{r}}} \dot{\user2{N}}_{1} {\varvec{B}}_{1} + {\varvec{R}}_{{\text{r}}} {\varvec{N}}_{1} \\ & \quad \times \left[ {\begin{array}{*{20}c} { - {\varvec{E}}^{{\text{T}}} \dot{\user2{a}}} & {\dot{\user2{G}}_{3}^{{\text{T}}} } & {\dot{\user2{G}}_{3}^{{\text{T}}} } \\ \end{array} } \right]^{{\text{T}}} \\ \end{aligned} $$

(A11)

$$ \begin{aligned} \dot{\user2{H}}_{2} & = \ddot{s}_{1} {\varvec{a}}_{0} - \ddot{s}_{1} {\varvec{R}}_{{\text{r}}} {\varvec{e}}_{1} - \dot{s}_{1} \dot{\user2{R}}_{{\text{r}}} {\varvec{e}}_{1} - \ddot{s}_{1} {\varvec{RR}}_{{\text{r}}} {\varvec{e}}_{1} G_{4}\\&\quad + \chi \user2{R\dot{R}}_{{\text{r}}} {\varvec{e}}_{1} G_{4} + \chi {\varvec{RR}}_{{\text{r}}} {\varvec{e}}_{1} \dot{G}_{4}\\&\quad + \user2{R\dot{R}}_{{\text{r}}} {\varvec{N}}_{1} \overline{\user2{q}}G_{4} + {\varvec{RR}}_{{\text{r}}} \dot{\user2{N}}_{1} \overline{\user2{q}}G_{4} \\ & \quad + {\varvec{RR}}_{{\text{r}}} {\varvec{N}}_{1} \user2{\dot{\overline{q}}}G_{4} + {\varvec{RR}}_{{\text{r}}} {\varvec{N}}_{1} \overline{\user2{q}}\dot{G}_{4} + \dot{\user2{R}}_{{\text{r}}} \dot{\user2{N}}_{1} \overline{\user2{q}} + {\varvec{R}}_{{\text{r}}} \user2{\ddot{N}}_{1} \overline{\user2{q}} \\&\quad+ {\varvec{R}}_{{\text{r}}} \dot{\user2{N}}_{1} \user2{\dot{\overline{q}}} + \dot{\user2{R}}_{{\text{r}}} {\varvec{N}}_{1} {\varvec{B}}_{2} + {\varvec{R}}_{{\text{r}}} \dot{\user2{N}}_{1} {\varvec{B}}_{2} \\&\quad+ {\varvec{R}}_{{\text{r}}} {\varvec{N}}_{1} \left[ {\begin{array}{*{20}c} {\dot{\user2{a}}^{{\text{T}}} {\varvec{a}}_{0} \dot{l}_{s} + \left( {{\varvec{a}}^{{\text{T}}} - {\varvec{a}}_{0}^{{\text{T}}} } \right){\varvec{a}}_{0} \ddot{l}_{s} } & { - \dot{G}_{4} } & { - \dot{G}_{4} } \\ \end{array} } \right]^{{\text{T}}} \\ \end{aligned} $$

(A12)

where $\dot{{\varvec{a}}}$ can be obtained from Eq. (A3). The first-time derivative and second-time derivative of the shape function matrix can be obtained using Eq. (8), i.e.,

$$\begin{aligned}\dot{\boldsymbol{\varPhi }}&=\frac{\partial \boldsymbol{\varPhi }}{\partial \chi }\dot{\chi }+\frac{\partial \boldsymbol{\varPhi }}{\partial {l}_{s}}{\dot{l}}_{s}, \ddot{\boldsymbol{\varPhi }}=\frac{{\partial }^{2}\boldsymbol{\varPhi }}{{\partial }^{2}\chi }{\dot{\chi }}^{2}+\frac{\partial \boldsymbol{\varPhi }}{\partial \chi }\ddot{\chi }++\frac{{\partial }^{2}\boldsymbol{\varPhi }}{\partial {l}_{s}\partial \chi }{\dot{l}}_{s}\dot{\chi }\\ &\quad +\frac{{\partial }^{2}\boldsymbol{\varPhi }}{{\partial }^{2}{l}_{s}}{\dot{l}}_{s}^{2}+\frac{\partial \boldsymbol{\varPhi }}{\partial {l}_{s}}{\ddot{l}}_{s}\end{aligned}$$

(A13)

${\dot{{\varvec{G}}}}_{3}$ and ${\dot{G}}_{4}$ can be obtained using Eq. (A5), i.e.,

$${\dot{{\varvec{G}}}}_{3}=-\frac{{\dot{l}}_{\mathrm{c}}}{{l}_{\mathrm{c}}^{2}}{{\varvec{b}}}^{\mathrm{T}}{\varvec{E}}-\frac{1}{{l}_{\mathrm{c}}}{\dot{{\varvec{a}}}}^{\mathrm{T}}{\varvec{R}}{\varvec{E}}$$

(A14)

$${\dot{G}}_{4}=-\frac{{\dot{l}}_{\mathrm{c}}}{{l}_{\mathrm{c}}^{2}}{{\varvec{b}}}^{\mathrm{T}}{{\varvec{a}}}_{0}{\dot{l}}_{s}-\frac{1}{{l}_{\mathrm{c}}}{\dot{{\varvec{a}}}}^{\mathrm{T}}{\varvec{R}}{{\varvec{a}}}_{0}{\dot{l}}_{s}+\frac{1}{{l}_{\mathrm{c}}}{{\varvec{b}}}^{\mathrm{T}}{{\varvec{a}}}_{0}{\ddot{l}}_{s}$$

(A15)

where ${\dot{l}}_{\mathrm{c}}$ can be obtained from (A1).

Taking the time derivative of Eq. (12) and using Eqs. (A4) and (A6), the terms ${{\varvec{H}}}_{3}$ and ${H}_{4}$ in Eq. (14) can be obtained as

$${{\varvec{H}}}_{3}={{\varvec{N}}}_{2}{{\varvec{B}}}_{1}+{{\varvec{G}}}_{3}, {H}_{4}={\dot{{\varvec{N}}}}_{2}\overline{{\varvec{q}} }+{{\varvec{N}}}_{2}{{\varvec{B}}}_{2}+{G}_{4}$$

(A16)

Further, taking the time derivative of ${{\varvec{H}}}_{3}$ and ${H}_{4}$, one obtains

$${\dot{{\varvec{H}}}}_{3}={\dot{{\varvec{N}}}}_{2}{{\varvec{B}}}_{1}+{{\varvec{N}}}_{2}{\left[\begin{array}{ccc}-{{\varvec{E}}}^{\mathrm{T}}\dot{{\varvec{a}}}& {\dot{{\varvec{G}}}}_{3}^{\mathrm{T}}& {\dot{{\varvec{G}}}}_{3}^{\mathrm{T}}\end{array}\right]}^{\mathrm{T}}+{\dot{{\varvec{G}}}}_{3}$$

(A17)

$$\begin{aligned}{\dot{H}}_{4}&={\ddot{{\varvec{N}}}}_{2}\overline{{\varvec{q}} }+{\dot{{\varvec{N}}}}_{2}\dot{\overline{{\varvec{q}}} }+{\dot{{\varvec{N}}}}_{2}{{\varvec{B}}}_{2}+{{\varvec{N}}}_{2}\\ & \quad \times{\left[\begin{array}{ccc}{\dot{{\varvec{a}}}}^{\mathrm{T}}{{\varvec{a}}}_{0}{\dot{l}}_{s}+\left({{\varvec{a}}}^{\mathrm{T}}-{{\varvec{a}}}_{0}^{\mathrm{T}}\right){{\varvec{a}}}_{0}{\ddot{l}}_{s}& -{\dot{G}}_{4}& -{\dot{G}}_{4}\end{array}\right]}^{\mathrm{T}}+{\dot{G}}_{4}\end{aligned}$$

(A18)