Skip to main content
SpringerLink
Log in

A new beam element for analysis of planar large deflection

  • Technical Paper
  • Published:
Journal of the Brazilian Society of Mechanical Sciences and Engineering Aims and scope Submit manuscript

Abstract

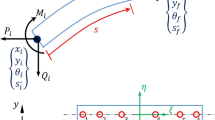
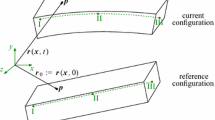
In the present study, a simple and efficient finite element approach is presented for large deflection analysis of both the straight and curved Euler–Bernoulli beams in the planar static problems. The linear stress–strain relationship is assumed for the Euler–Bernoulli beam. In some of finite element methods, displacements together with rotations, and in some others, positions are considered as the main fields of interpolation. However, in the present study, the main idea for the interpolation is using the dimensions of the deformed element instead of the displacements. Therefore, the slope angle (like the previous works) and the length of beam centroidal axis (unlike the previous works) are used as the main field parameters. This treatment creates simplicity in the constitutive equations. Next, using the weighted residual method, the constitutive equations are applied to the element. Using the equilibrium equations and kinematic equations, the position coordinates of the nodes are related to the internal forces and the main field parameters. In the present study, three-node element and, consequently, Simpson’s 1/3 rule is used for integration. For solving nonlinear equations of the beam, the Newton–Raphson method is used. Finally, several numerical examples are presented and compared with the previous works to illustrate the validity and efficiency of the new element.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Reddy JN (1997) Relationships between bending solutions of classical and shear deformation beam theories. Int J Solids Struct 34(26):3373–3384

    Article  MATH  Google Scholar 

  2. Crisfield MA (1990) A consistent co-rotational formulation for non-linear, three-dimensional, beam-elements. Comput Methods Appl Mech Eng 81:131–150

    Article  MATH  Google Scholar 

  3. Simo JC (1985) A finite strain beam formulation. The three-dimensional dynamic problem. Part I. Comput Methods Appl Mech Eng 49:55–70

    Article  MATH  Google Scholar 

  4. Simo JC (1986) A three-dimensional finite-strain rod model. Part II: computational aspects. Comput Methods Appl Mech Eng 58:79–116

    Article  MATH  Google Scholar 

  5. Mattiasson K (1981) Numerical results from large deflection beam and frame problems analysed by means of elliptic integrals. Int J Numer Methods Eng 17:145–153

    Article  MATH  Google Scholar 

  6. Nanakorn P, Vu LN (2006) A 2D field-consistent beam element for large displacement analysis using the total Lagrangian formulation. Finite Elem Anal Des 42:1240–1247

    Article  Google Scholar 

  7. Lee K (1997) Analysis of large displacements and large rotations of three-dimensional beams by using small strains and unit vectors. Commun Numer Methods Eng 13:987–997

    Article  MATH  Google Scholar 

  8. Schwab AL, Meijaard JP (2010) Comparison of three-dimensional flexible beam elements for dynamic analysis: classical finite element formulation and absolute nodal coordinate formulation. J Comput Nonlinear Dyn 5(1):011010

    Article  Google Scholar 

  9. Ryu HS, Sin HC (1996) Curved beam elements based on strain fields. Commun Numer Methods Eng 12:767–773

    Article  MathSciNet  MATH  Google Scholar 

  10. Choi JK, Lim JK (1995) General curved beam elements based on the assumed strain fields. Comput Struct 55(3):379–386

    Article  MATH  Google Scholar 

  11. Dvorkin EN (1988) On a non-linear formulation for curved Timoshenko beam elements considering large displacement/rotation increments. Int J Numer Methods Eng 26:1597–1613

    Article  MATH  Google Scholar 

  12. Schulz M, Filippou FC (2001) Non-linear spatial Timoshenko beam element with curvature interpolation. Int J Numer Methods Eng 50:761–785

    Article  MATH  Google Scholar 

  13. Ganapathi M, Patel BP, Saravanan J, Touratier M (1999) Shear flexible curved spline beam element for static analysis. Finite Elem Anal Des 32:181–202

    Article  MATH  Google Scholar 

  14. Reddy JN (1997) On locking-free shear deformable beam finite elements. Comput Methods Appl Mech Eng 149:113–132

    Article  MATH  Google Scholar 

  15. Calhoun PR, DaDeppo DA (1983) Nonlinear finite element analysis of clamped arches. J Struct Eng 109:599–612

    Article  Google Scholar 

  16. Wen RK, Suhendro B (1991) Nonlinear curved-beam element for arch structures. J Struct Eng 117:3496–3515

    Article  Google Scholar 

  17. DaDeppo DA, Schmidt R (1975) Instability of clamped-hinged circular arches subjected to a point load. Trans ASME 97(3):894–896

    Article  Google Scholar 

  18. Elias M, Chen KL (1988) Nonlinear shallow curved-beam finite element. J Eng Mech 114:1076–1087

    Article  Google Scholar 

  19. DaDeppo DA, Schmidt R (1974) Large deflections and stability of hingeless circular arches under interacting loads. J Appl Mech 41(4):989–994

    Article  Google Scholar 

  20. Surana KS, Sorem RM (1989) Geometrically non-linear formulation for three dimensional curved beam elements with large rotations. Int J Numer Methods Eng 28:43–73

    Article  MATH  Google Scholar 

  21. Meier C, Popp A, Wall WA (2015) A locking-free finite element formulation and reduced models for geometrically exact Kirchhoff rods. Comput Methods Appl Mech Eng 290:314–341

    Article  MathSciNet  Google Scholar 

  22. Meier C, Popp A, Wall WA (2014) An objective 3D large deformation finite element formulation for geometrically exact curved Kirchhoff rods. Comput Methods Appl Mech Eng 278:445–478

    Article  MathSciNet  Google Scholar 

  23. Masjedi PK, Ovesy HR (2015) Chebyshev collocation method for static intrinsic equations of geometrically exact beams. Int J Solids Struct 54:183–191

    Article  MATH  Google Scholar 

  24. Zhang R, Zhong H (2014) Weak form quadrature element analysis of spatial geometrically exact shear-rigid beams. Finite Elem Anal Des 87:22–31

    Article  MathSciNet  Google Scholar 

  25. Gerstmayra J, Irschik H (2008) On the correct representation of bending and axial deformation in the absolute nodal coordinate formulation with an elastic line approach. J Sound Vib 318:461–487

    Article  Google Scholar 

  26. Sugiyama H, Koyama H, Yamashita H (2010) Gradient deficient curved beam element using the absolute nodal coordinate formulation. J Comput Nonlinear Dyn 5(2):021001

    Article  Google Scholar 

  27. Gerstmayr J, Matikainen MK, Mikkola AM (2008) A geometrically exact beam element based on the absolute nodal coordinate formulation. Multibody Syst Dyn 20:359–384

    Article  MathSciNet  MATH  Google Scholar 

  28. Romero I (2008) A comparison of finite elements for nonlinear beams: the absolute nodal coordinate and geometrically exact formulations. Multibody Syst Dyn 20:51–68

    Article  MathSciNet  MATH  Google Scholar 

  29. Bauchau OA, Han S, Mikkola A, Matikainen MK (2014) Comparison of the absolute nodal coordinate and geometrically exact formulations for beams. Multibody Syst Dyn 32(1):67–85

    Article  MathSciNet  Google Scholar 

  30. Shabana AA, Yakoub RA (2001) Three dimensional absolute nodal coordinate formulation for beam elements: theory. J Mech Des 123:606–613

    Article  Google Scholar 

  31. Yakoub RA, Shabana AA (2001) Three dimensional absolute nodal coordinate formulation for beam elements: implementation and applications. J Mech Des 123:614–621

    Article  Google Scholar 

  32. Shabana AA (1998) Dynamics of multibody systems. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  33. Coda HB, Greco M (2004) A simple FEM formulation for large deflection 2D frame analysis based on position description. Comput Methods Appl Mech Eng 193:3541–3557

    Article  MATH  Google Scholar 

  34. Jonker JB, Meijaard JP (2013) A geometrically non-linear formulation of a three-dimensional beam element for solving large deflection multibody system problems. Int J Nonlinear Mech 53:63–74

    Article  Google Scholar 

  35. Saritas A (2009) Modeling of inelastic behavior of curved members with a mixed formulation beam element. Finite Elem Anal Des 45:357–368

    Article  MathSciNet  Google Scholar 

  36. Pulngern T, Sudsanguan T, Athisakul C, Chucheepsakul S (2013) Elastica of a variable-arc-length circular curved beam subjected to an end follower force. Int J Nonlinear Mech 49:129–136

    Article  Google Scholar 

  37. Alkhaldi HS, Alshaikh IA, Mallouh RA, Ghazal O (2014) Closed-form solution of large deflection of a spring-hinged beam subjected to non-conservative force and tip end moment. Eur J Mech A Solid 47:271–279

    Article  MathSciNet  Google Scholar 

  38. Shvartsman BS (2013) Analysis of large deflections of a curved cantilever subjected to a tip-concentrated follower force. Int J Nonlinear Mech 50:75–80

    Article  Google Scholar 

  39. Levyakov SV (2015) Formulation of a geometrically nonlinear 3D beam finite element based on kinematic-group approach. Appl Math Modelling, In Press, Corrected Proof, Available online 7 February 2015

  40. Sharifnia M, Akbarzadeh A (2016) A constrained assumed modes method for solution of a new dynamic equation for an axially moving beam. Comput Math Appl 72(9):2167–2180

    Article  MathSciNet  MATH  Google Scholar 

  41. Sharifnia M, Akbarzadeh A (2014) An analytical model for vibration and control of a PR-PRP parallel robot with flexible platform and prismatic joint. J Vib Control 22(3):632–648

    Article  Google Scholar 

  42. Sharifnia M, Akbarzadeh A (2016) Approximate analytical solution for vibration of a 3-PRP planar parallel robot with flexible moving platform. Robotica 34(1):71–79

    Article  Google Scholar 

  43. Sharifnia M, Akbarzadeh A (2016) Dynamics and vibration of a 3-PSP parallel robot with flexible moving platform. J Vib Control 22(4):1095–1116

    Article  MathSciNet  Google Scholar 

  44. Sharifnia M, Akbarzadeh A (2017) A constrained assumed modes method for dynamics of a flexible planar serial robot with prismatic joints. Multibody Syst Dyn 40(3):261–285

    Article  MathSciNet  MATH  Google Scholar 

  45. Hoffman JD (1992) Numerical methods for engineers and scientists, 2nd edn. McGraw-Hill, Inc., New York

Download references

Acknowledgements

This work was supported by the Office of the Vice Chancellor for Research, Islamic Azad University, Semnan Branch, with Grant no. 6357-05/05/1394. The author would like to express his grateful thanks to Islamic Azad University, Semnan Branch.

Author information

Authors and Affiliations

  1. Mechanical Engineering Department, Semnan Branch, Islamic Azad University, Semnan, Iran

    Mahdi Sharifnia

Authors
  1. Mahdi Sharifnia
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mahdi Sharifnia.

Additional information

Technical Editor: Aline Souza de Paula.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sharifnia, M. A new beam element for analysis of planar large deflection. J Braz. Soc. Mech. Sci. Eng. 40, 92 (2018). https://doi.org/10.1007/s40430-018-0970-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40430-018-0970-6

Keywords

Search

Navigation