Coriolis effects on the thermo-mechanical vibration analysis of the rotating multilayer piezoelectric nanobeam

Abstract

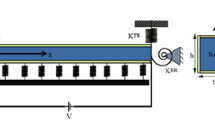
In this study, the Coriolis effects on the vibration behavior of the multilayer rotating piezoelectric nanobeam are investigated. The governing equations are obtained using the nonlocal continuum and surface elasticity theories. The axial and transverse governing equations are influenced by the Coriolis effects. The differential quadrature method (DQM) is applied to obtain the vibration frequencies of the multilayer piezoelectric nanobeams. A good correlation is obtained between the numerical results and the results presented in available literature. The influences of the different boundary conditions and the thermal change in the vibration behavior of multilayer piezoelectric nanobeams are studied. The present work shows that the Coriolis effects strongly affect the vibration behavior of multilayer piezoelectric nanobeams. The numerical results show that the Coriolis effects under the flexible boundary conditions are more significant than rigid boundary conditions. The results of this study can be used to design and manufacture nanosensors, biosensors, atomic force microscopes, nanoelectromechanical systems, and micro-electromechanical systems (NEMS/MEMS) devices.

Appendix

In this section, to clarify more, some extra equations for Eq. (35) are given. To this end, the mass, the damping, and the stiffness matrix are stated as

$$\left[ M \right] = \left[ {\begin{array}{*{20}l} {\begin{array}{*{20}l} {M_{11} } \\ {M_{21} } \\ {M_{31} } \\ \end{array} } & {\begin{array}{*{20}l} {M_{12} } \\ {M_{22} } \\ {M_{32} } \\ \end{array} } & {\begin{array}{*{20}l} {M_{13} } \\ {M_{23} } \\ {M_{33} } \\ \end{array} } \\ \end{array} } \right], \quad \left[ C \right] = \left[ {\begin{array}{*{20}l} {\begin{array}{*{20}l} {C_{11} } \\ {C_{21} } \\ {C_{31} } \\ \end{array} } & {\begin{array}{*{20}l} {C_{12} } \\ {C_{22} } \\ {C_{32} } \\ \end{array} } & {\begin{array}{*{20}l} {C_{13} } \\ {C_{23} } \\ {C_{33} } \\ \end{array} } \\ \end{array} } \right], \quad \left[ K \right] = \left[ {\begin{array}{*{20}l} {\begin{array}{*{20}l} {K_{11} } \\ {K_{21} } \\ {K_{31} } \\ \end{array} } & {\begin{array}{*{20}l} {K_{12} } \\ {K_{22} } \\ {K_{32} } \\ \end{array} } & {\begin{array}{*{20}l} {K_{13} } \\ {K_{23} } \\ {K_{33} } \\ \end{array} } \\ \end{array} } \right] .$$

(40)

The rows of the matrices are related to Eqs. (30a-c–32a-c). The nonzero elements of the matrices are given as

$$K_{{23}} = F_{{31}}^{*} \sum\limits_{{k = 1}}^{N} {C_{{ik}}^{2} } \left( \phi \right)_{{kj}},$$

$$K_{{32}} = - F_{{31}}^{*} \sum\limits_{{k = 3}}^{{N - 2}} {C_{{ik}}^{2} \left( W \right)_{{kj}} },$$

$$K_{33} = X_{11}^{*} \sum\limits_{k = 2}^{N - 1} {C_{ik}^{2} \left({\varvec{\varPhi}}\right)_{kj} } - X_{33}^{*} \left({\varvec{\varPhi}}\right)_{ij},$$

$${\tilde{K}}_{w} = \left\{ {\begin{array}{*{20}c} {K_{w} + K_{w}^{u} {\text{ for }}m = 1 {\text{ or the first layer}}} \\ {K_{w} {\text{ for }}m = 2, \dots, N - 1 {\text{ or the internal layers}}} \\ {K_{w} + K_{w}^{l} {\text{ for }}m = N {\text{ or the last layer}}} \end{array}} \right.$$

$${\tilde{K}}_{s} = \left\{ {\begin{array}{*{20}l}{K_{s} + K_{s}^{u} {\text{ for }}m = 1} \\ {K_{s} {\text{ for }}m = 2,\ldots, N - 1} \\ {K_{s} + K_{s}^{l} {\text{ for }}m = N} \end{array} } \right.$$

(41)

The other elements are zero.

$$\begin{gathered} M_{12} = M_{13} = M_{21} = M_{23} = M_{31} = M_{32} = M_{33} = 0, \hfill \\ C_{11} = C_{13} = C_{22} = C_{23} = C_{31} = C_{32} = C_{33} = 0, \hfill \\ K_{12} = K_{13} = K_{21} = K_{31} = 0 \hfill \\ \end{gathered}$$

(42)