Skip to main content
SpringerLink
Log in

Layer-wise dynamic analysis of a beam with global and local viscoelastic contributions using an FE/Laplace transform approach

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

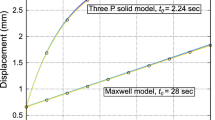
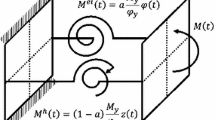
In this research, the mechanism of energy dissipation in viscoelastic materials is presented by extracting time-dependent displacement and stress–strain distribution analysis in a beam, which contains a viscoelastic part. The structure is discretized into finite beam elements, the kinematic theory of which is based on Carrera unified formulation using Lagrange expansion on the cross section. For the viscoelastic contribution, the integral form of viscoelastic constitutive law in which the stress is related to the integral form of the viscous module and the strain rate is executed. The governing equations of the finite element model of the structure are derived using Hamilton’s principle, and to avoid the computational cost of convolution integral, we have exploited the Laplace transform to the three-dimensional displacement, stress, and strain fields. Finally, the results are transformed to the time domain using numerical inversion methods. A numerical model that has been validated with the results in the literature is considered. To highlight the depreciating consequence of the viscoelastic material, simulation of the multi-layered beam that is isotropic and elastic in all parts except for a certain part that contains the viscoelastic contribution is undertaken, and the temporal response and the stress–strain fields are extracted and discussed. As a result, extreme variations in stress distribution in the location of viscoelastic contributions, hysteresis stress–strain, and changes in beam temporal history to viscoelastic contribution displacement have been observed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  1. Nashif, A.D., Jones, D.I.G., Henderson, J.P.: Vibration Damping. Kluwer Academic Publishers, Dordrecht, The Netherlands (1992)

    Google Scholar 

  2. Jones, D.I.G.: Handbook of Viscoelastic Vibration Damping. Wiley, Dordrecht, The Netherlands (2001)

    Google Scholar 

  3. Deleeuw, S.L.: Theory of Viscoelasticity, An Introduction, 2nd edn. By R. M. Christensen, Elsevier, Amsterdam (2012)

  4. Gurtin, M.E., Sternberg, E.: On the linear theory of viscoelasticity. Arch. Rational Mech. Anal. 11, 291–356 (1962)

    Article  MathSciNet  MATH  Google Scholar 

  5. Lockett, F.J.: Nonlinear viscoelastic solids. SIAM Rev. 7(3), 323–340 (1965)

    Article  MathSciNet  Google Scholar 

  6. Lakes, R.S.: Viscoelastic Materials. CRC Press, Boca Raton (1999)

    MATH  Google Scholar 

  7. Golden, J.M., Graham, G.A.C.: Boundary value problems in linear viscoelasticity. Springer Science & Business Media, New York (2013)

    MATH  Google Scholar 

  8. Bertram, A., Reddy, J.N.: An Introduction to Continuum Mechanics. Cambridge University Press, Cambridge (2007)

    Google Scholar 

  9. Flügge, W.: Viscoelasticity. Springer-Verlag, Berlin (1967)

  10. Narayanan, G.V., Beskos, D.E.: Numerical operational methods for time-dependent linear problems. Int. J. Numer. Methods Eng. 18(12), 1829–1854 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  11. Luongo, A., D’Annibale, F.: Invariant subspace reduction for linear dynamic analysis of finite-dimensional viscoelastic structures. Meccanica 52, 3061–3085 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  12. Golla, D.F., Hughes, P.C.: Dynamics of viscoelastic structures–a time-domain, finite element formulation. J. Appl. Mech. 52(4), 897–906 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  13. Sim, W.J., Kwak, B.M.: Linear viscoelastic analysis in time-domain by boundary element method. Comput. Struct. 29(4), 531–539 (1988)

    Article  MATH  Google Scholar 

  14. McTavish, D.J., Hughes, P.C.: Modeling of linear viscoelastic space structures. J. Vibr. Acoust. 115(1), 103–110 (1993)

    Article  Google Scholar 

  15. Schanz, M.: A boundary element formulation in time-domain for viscoelastic solids. Commun. Numer. Methods Eng. 15(11), 799–809 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  16. Hatada, T., Kobori, T., Ishida, M., Niwa, N.: Dynamic analysis of structures with maxwell model. Earthq. Eng. Struct. Dyn. 29(12), 159–176 (2000)

    Article  Google Scholar 

  17. Lewandowski, R., Bartkowiak, A., Maciejewski, H.: Dynamic analysis of frames with viscoelastic dampers: a comparison of damper models. Struct. Eng. Mech. 41(1), 113–137 (2012)

    Article  Google Scholar 

  18. Reddy, J.N.: Introduction to the Finite Element Method. McGraw-Hill Education, New York (2005)

  19. Yagawa, G., Miyazaki, N., Ando, Y.: Superposition method of finite element and analytical solutions for transient creep analysis. Int. J. Numer. Methods Eng. 11(7), 1107–1115 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  20. Yang, T.Y., Lianis, G.: Large displacement analysis of viscoelastic beams and frames by the finite-element method. J. Appl. Mech. 11(7), 635–640 (1974)

    Article  Google Scholar 

  21. Schapery, R.A.: Viscoelastic behavior and analysis of composite materials. Mech. Compos. Mater. (1974)

  22. Coleman, B.D., Noll, W.: Foundations of linear viscoelasticity. Rev. Mod. Phys. 33(2), 113–123 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  23. Chen, T.M.: The hybrid Laplace transform/finite element dynamic analysis of viscoelastic Timoshenko beams method applied to the quasi-static. Int. J. Numer. Methods Eng. 38(3), 509–522 (1955)

    Article  MATH  Google Scholar 

  24. Johnson, A.R., Tessler, A., Dambach, M.: Dynamics of thick viscoelastic beams. J. Eng. Mater. Technol. Trans. ASME 119(3), 273–278 (1997)

    Article  Google Scholar 

  25. Payette, G.S., Reddy, J.N.: Nonlinear quasi-Gtatic finite element formulations for viscoelastic Euler-Bernoulli and Timoshenko beams. Int. J. Numer. Methods Biomed. Eng. 26(12), 1736–1755 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  26. Vallala, V.P., Payette, G.S., Reddy, J.N.: A spectral/hp nonlinear finite element analysis of higher-order beam theory with viscoelasticity. Int. J. Appl. Mech. 4(1), 1250010 (2012)

    Article  Google Scholar 

  27. Vallala, V., Ruimi, A., Reddy, J.N.: Nonlinear viscoelastic analysis of orthotropic beams using a general third-order theory. Compos. Struct. 94(12), 3759–3768 (2012)

    Article  Google Scholar 

  28. Halpin, J.C., Pagano, N.J.: Observations on linear anisotropic viscoelasticity. J. Compos. Mater. 2(1), 68–80 (1968)

    Article  Google Scholar 

  29. Chambers, R.S., Becker, E.B.: Integration error controls for a finite element viscoelastic analysis. Comput. Struct. 24(4), 537–544 (1986)

    Article  MATH  Google Scholar 

  30. Chniewind, A.P., Barrett, J.D.: Wood as a linear orthotropic viscoelastic material. Comput. Struct. 6(1), 43–57 (1972)

  31. Filippi, M., Carrera, E., Valvano, S.: Analysis of multilayered structures embedding viscoelastic layers by higher-order, and zig-zag plate elements. Compos. Part B Eng. 154(12), 77–89 (2018)

    Article  MATH  Google Scholar 

  32. Filippi, M., Carrera, E., Regalli, A.M.: Layerwise analyses of compact and thin-walled beams made of viscoelastic materials. J. Vibr. Acoust., 138(6) (2016)

  33. Filippi, M., Carrera, E.: Various refined theories applied to damped viscoelastic beams and circular rings. Acta Mech. 228(12), 4235–4248 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Filippi, M., Carrera, E.: Advanced zig-zag beam theories for sandwich structures analyses. ASME International Mechanical Engineering Congress and Exposition, 52002:V001T03A02 (2018)

  35. Filippi, M., Carrera, E.: Stress analyses of viscoelastic three-dimensional beam-like structures with low-and high-order one-dimensional finite elements. Meccanica 56(6), 1475–1482 (2021)

    Article  MathSciNet  Google Scholar 

  36. Carrera, E., Cinefra, M., Petrolo, M., Zappino, E.: Finite Element Analysis of Structures Through Unified Formulation. Springer-Verlag, Berlin (2014)

    Book  MATH  Google Scholar 

  37. Bathe, K.: Finite Element Procedures. Springer-Verlag, Berlin (1995)

    MATH  Google Scholar 

  38. Ing, Y.S., Liao, H.F.: Investigation on transient responses of a piezoelectric crack by using Durbin and Zhao methods for numerical inversion of Laplace transforms. J. Mech. 30, 3–10 (2014)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Aerospace Engineering, Amirkabir University of Technology, Tehran, 159163-4311, Iran

    Sh. Kiasat & A. S. Nobari

  2. Mul2 Group, Department of Mechanical and Aerospace Engineering, Politecnico di Torino, 10129, Torino, Italy

    Sh. Kiasat, M. Filippi & E. Carrera

  3. Aeronautics Engineering Department, Imperial College, New York, USA

    A. S. Nobari

Authors
  1. Sh. Kiasat
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. Filippi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. S. Nobari
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. E. Carrera
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sh. Kiasat.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kiasat, S., Filippi, M., Nobari, A.S. et al. Layer-wise dynamic analysis of a beam with global and local viscoelastic contributions using an FE/Laplace transform approach. Acta Mech 233, 4747–4761 (2022). https://doi.org/10.1007/s00707-022-03349-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03349-6

Search

Navigation