Time-dependent behavior of laminated functionally graded beams bonded by viscoelastic interlayer based on the elasticity theory

Abstract

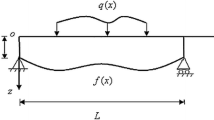
A two-dimensional (2-D) elasticity solution is developed to investigate the time-dependent response of laminated functionally graded beam with viscoelastic interlayer. The elastic modulus in each functionally graded layer varies through the thickness following an exponential function, and the mechanical property of each layer is described by the exact 2-D elasticity equations. Viscoelastic property in the interlayer is simulated by the Maxwell–Wiechert model, in which the memory effect of it is neglected. By virtue of the recursive matrix method, the solution of stress and deformation can be obtained efficiently. The comparison studies indicate that the relative error of the Euler–Bernoulli solution is slight for slender beam with small gradient factor, but it significantly increases as the beam thickness or the gradient factor grows. The finite element solution is close to the present one with large number of sub-layers in FE modeling and small gradient factor of FG layer, while the error of FE solution increases as the number of sub-layers decrease or the gradient factor increases. Besides, the influences of material parameters upon the time-dependent behavior and the optimization for the stress and deformation in the beam are discussed in detail.

Appendix

Functions $n_{m,j}^{i}$ and $g_{m,j}^{i} (j=1, 2, 3, 4)$ involved in Eqs. (14) and (15)

$$\begin{aligned} n_{m,1}^{i}= & {} -\frac{1}{2}\left( k_{i} +\sqrt{k_{i}^{2}+4\alpha _{m}^{2}-4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } \right) , n_{m,2}^{i} =\frac{1}{2}\left( -k_{i} +\sqrt{k_{i}^{2}+4\alpha _{m}^{2}-4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } \right) , \\ n_{m,3}^{i}= & {} -\frac{1}{2}\left( k_{i} +\sqrt{k_{i}^{2}+4\alpha _{m}^{2}+4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } \right) , n_{m,4}^{i} =\frac{1}{2}\left( -k_{i} +\sqrt{k_{i}^{2}+4\alpha _{m}^{2}+4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } \right) , \\ g_{m,1}^{i}= & {} \frac{1}{4\alpha _{m} f_{m,3}^{i} }\left[ -k_{i}^{2} f_{m,1}^{i} +\mu _{i} (f_{m,1}^{i} )^{3}-2\alpha _{m}^{2} k_{i} -6\alpha _{m}^{2} f_{m,1}^{i} +(f_{m,1}^{i} )^{3}+12\mu _{i} k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} -\mu _{i} k_{i}^{2} f_{m,1}^{i} \right. \\&\left. -\,4k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +6\alpha _{m}^{2} \mu _{i}^{2} k_{i} -2\alpha _{m}^{2} \mu _{i}^{2} f_{m,1}^{i} +4\alpha _{m}^{2} \mu _{i} k_{i} -8\alpha _{m}^{2} \mu _{i} f_{m,1}^{i} \right] , \\ g_{m,2}^{i}= & {} \frac{1}{4\alpha _{m} f_{m,3}^{i} }\left[ k_{i}^{2} f_{m,1}^{i} -\mu _{i} (f_{m,1}^{i} )^{3}-2\alpha _{m}^{2} k_{i} +6\alpha _{m}^{2} f_{m,1}^{i} -(f_{m,1}^{i} )^{3}+12\mu _{i} k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +\mu _{i} k_{i}^{2} f_{m,1}^{i}\right. \\&\left. -\,4k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +6\alpha _{m}^{2} \mu _{i}^{2} k_{i} +2\alpha _{m}^{2} \mu _{i}^{2} f_{m,1}^{i} +4\alpha _{m}^{2} \mu _{i} k_{i} +8\alpha _{m}^{2} \mu _{i} f_{m,1}^{i} \right] , \\ g_{m,3}^{i}= & {} \frac{1}{4\alpha _{m} f_{m3}^{i} }\left[ -k_{i}^{2} f_{m,1}^{i} +\mu _{i} (f_{m,1}^{i} )^{3}-2\alpha _{m}^{2} k_{i} -6\alpha _{m}^{2} f_{m,1}^{i} +(f_{m,1}^{i} )^{3}-12\mu _{i} k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} -\mu _{i} k_{i}^{2} f_{m,1}^{i} \right. \\&\left. +\,4k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +6\alpha _{m}^{2} \mu _{i}^{2} k_{i} -2\alpha _{m}^{2} \mu _{i}^{2} f_{m,1}^{i} +4\alpha _{m}^{2} \mu _{i} k_{i} -8\alpha _{m}^{2} \mu _{i} f_{m,1}^{i} \right] , \\ g_{m,4}^{i}= & {} \frac{1}{4\alpha _{m} f_{m,3}^{i} }\left[ k_{i}^{2} f_{m,1}^{i} -\mu _{i} (f_{m,1}^{i} )^{3}-2\alpha _{m}^{2} k_{i} +6\alpha _{m}^{2} f_{m,1}^{i} -(f_{m,1}^{i} )^{3}-12\mu _{i} k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +\mu _{i} k_{i}^{2} f_{m,1}^{i} \right. \\&\left. +4k_{i}^{2} \sqrt{-\mu _{i} } \alpha _{m} +6\alpha _{m}^{2} \mu _{i}^{2} k_{i} +2\alpha _{m}^{2} \mu _{i}^{2} f_{m,1}^{i} +4\alpha _{m}^{2} \mu _{i} k_{i} +8\alpha _{m}^{2} \mu _{i} f_{m,1}^{i} \right] , \\ f_{m,1}^{i}= & {} \sqrt{k_{i}^{2} +4\alpha _{m}^{2} -4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } , \\ f_{m,2}^{i}= & {} \sqrt{k_{i}^{2} +4\alpha _{m}^{2} +4\alpha _{m} k_{i} \sqrt{-\mu _{i} } } , \\ f_{m,3}^{i}= & {} \alpha _{m}^{2} \mu _{i}^{2} +2\alpha _{m}^{2} \mu _{i} +4k_{i}^{2} \mu _{i} +\alpha _{m}^{2} , \quad i =1, 2,\ldots , p, m=1, 2, 3\ldots . \end{aligned}$$