A discrete element and ray framework for rapid simulation of acoustical dispersion of microscale particulate agglomerations

Abstract

In industry, particle-laden fluids, such as particle-functionalized inks, are constructed by adding fine-scale particles to a liquid solution, in order to achieve desired overall properties in both liquid and (cured) solid states. However, oftentimes undesirable particulate agglomerations arise due to some form of mutual-attraction stemming from near-field forces, stray electrostatic charges, process ionization and mechanical adhesion. For proper operation of industrial processes involving particle-laden fluids, it is important to carefully breakup and disperse these agglomerations. One approach is to target high-frequency acoustical pressure-pulses to breakup such agglomerations. The objective of this paper is to develop a computational model and corresponding solution algorithm to enable rapid simulation of the effect of acoustical pulses on an agglomeration composed of a collection of discrete particles. Because of the complex agglomeration microstructure, containing gaps and interfaces, this type of system is extremely difficult to mesh and simulate using continuum-based methods, such as the finite difference time domain or the finite element method. Accordingly, a computationally-amenable discrete element/discrete ray model is developed which captures the primary physical events in this process, such as the reflection and absorption of acoustical energy, and the induced forces on the particulate microstructure. The approach utilizes a staggered, iterative solution scheme to calculate the power transfer from the acoustical pulse to the particles and the subsequent changes (breakup) of the pulse due to the particles. Three-dimensional examples are provided to illustrate the approach.

Appendices

Appendix 1: Basics of acoustics

In our approach, we model the individual particles as being rigid, and the material surrounding the particles as being isotropic and having a relatively low shear modulus, in the zero limit becoming an acoustical medium. Generally, for an isotropic material, one has the classical relationship between the components of infinitesimal strain (${\varvec{\epsilon }}$) to the Cauchy stress (${\varvec{\sigma }}$)

$$\begin{aligned} {\varvec{\sigma }}=\displaystyle {{\mathbb {E}}:{\varvec{\epsilon }}=3\kappa \frac{\mathrm{tr} {\varvec{\epsilon }}}{3}\mathbf{1}+2\mu {\varvec{\epsilon }}^{\prime }}, \end{aligned}$$

(41)

where ${\mathbb {E}}$ is the elasticity tensor and where ${\varvec{\epsilon }}^{\prime }$ is the strain deviator. The corresponding strain energy density is

$$\begin{aligned} \displaystyle {W=\frac{1}{2}{\varvec{\epsilon }}:{{\mathbb {E}}}:{\varvec{\epsilon }}= \frac{1}{2}\left( 9\kappa \left( \frac{\mathrm{tr} {\varvec{\epsilon }}}{3}\right) ^2+2\mu {\varvec{\epsilon }}^{\prime }:{\varvec{\epsilon }}^{\prime }\right) }. \end{aligned}$$

(42)

We focus on the dilatational deformation in the low-shear modulus matrix surrounding the particles. This naturally leads to an idealized “acoustical” material approximation, $\mu \approx 0$. Hence, Eq. 41 collapses to ${\varvec{\sigma }}=-p \mathbf{1}$, where the pressure is $p=-3\, \kappa \,\frac{\mathrm{tr} {{\varvec{\epsilon }}}}{3} \mathbf{1}$ and with a corresponding strain energy of ${W=\frac{1}{2}\frac{p^2}{\kappa }}$. By inserting the simplified expression of the stress ${\varvec{\sigma }}=-p\mathbf{1}$ into the equation of equilibrium, we obtain

$$\begin{aligned} \nabla \cdot {\varvec{\sigma }}=-\nabla p=\rho \ddot{{\varvec{u}}}, \end{aligned}$$

(43)

where ${\varvec{u}}$ is the displacement. By taking the divergence of both sides, and recognizing that $\nabla \cdot {\varvec{u}}=-\frac{p}{\kappa }$, we obtain

$$\begin{aligned} \nabla ^2 p=\frac{\rho }{\kappa }\ddot{p}=\frac{1}{c^2}\ddot{p}. \end{aligned}$$

(44)

If we assume a harmonic solution, we obtain

$$\begin{aligned}&p=Pe^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)} \Rightarrow \dot{p}=Pj\omega e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)} \nonumber \\&\quad \Rightarrow \ddot{p}=-P\omega ^2 e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}, \end{aligned}$$

(45)

and

$$\begin{aligned}&\nabla p=Pj(k_x{\varvec{e}}_x+k_y{\varvec{e}}_y+k_z{\varvec{e}}_z)e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)} \nonumber \\&{} \Rightarrow \quad \nabla \cdot \nabla p= \nabla ^2 p= -P\underbrace{(k^2_x+k^2_y+k^2_z)}_{||{\varvec{k}}||^2} e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}.\nonumber \\ \end{aligned}$$

(46)

We insert these relations into Eq. 44, and obtain an expression for the magnitude of the wave number vector

$$\begin{aligned} -P||{\varvec{k}}||^2e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}=-\frac{\rho }{\kappa } P\omega ^2e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)} \Rightarrow ||{\varvec{k}}||=\frac{\omega }{c}. \end{aligned}$$

(47)

Equation 43 (balance of linear momentum) implies

$$\begin{aligned} \rho \ddot{{\varvec{u}}}=-\nabla p=-Pj(k_x{\varvec{e}}_x+k_y{\varvec{e}}_y+k_z{\varvec{e}}_z)e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}. \end{aligned}$$

(48)

Now we integrate once, which is equivalent to dividing by $-j\omega$, and obtain the velocity

$$\begin{aligned} \dot{{\varvec{u}}}=\frac{Pj}{\rho \omega }(k_x{\varvec{e}}_x+k_y{\varvec{e}}_y+k_z{\varvec{e}}_z)e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}, \end{aligned}$$

(49)

and do so again for the displacement

$$\begin{aligned} {\varvec{u}}=\frac{Pj}{\rho \omega ^2}(k_x{\varvec{e}}_x+k_y{\varvec{e}}_y+k_z{\varvec{e}}_z)e^{j({\varvec{k}}\cdot {\varvec{r}}-\omega t)}. \end{aligned}$$

(50)

Thus, we have

$$\begin{aligned} ||\dot{{\varvec{u}}}||=\frac{P}{c\rho }. \end{aligned}$$

(51)

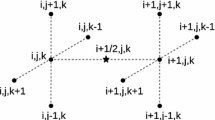
The reflection of a plane harmonic pressure wave at an interface is given by enforcing continuity of the (acoustical) pressure and disturbance velocity at that location; this yields the ratio between the incident and reflected pressures. We use a local coordinate system (Fig. 3), and require that the number of waves per unit length in the $x-\mathrm{direction}$ must be the same for the incident, reflected and refracted (transmitted) waves,

$$\begin{aligned} {\varvec{k}}_i\cdot {\varvec{e}}_x={\varvec{k}}_r\cdot {\varvec{e}}_x= {\varvec{k}}_t\cdot {\varvec{e}}_x. \end{aligned}$$

(52)

From the pressure balance at the interface, we have

$$\begin{aligned} P_ie^{j({\varvec{k}}_i\cdot {\varvec{r}}-\omega t)}+ P_re^{j({\varvec{k}}_r\cdot {\varvec{r}}-\omega t)}= P_te^{j({\varvec{k}}_t\cdot {\varvec{r}}-\omega t)}, \end{aligned}$$

(53)

where $P_i$ is the incident pressure ray, $P_r$ is the reflected pressure ray, and $P_t$ is the transmitted pressure ray. This forces a time-invariant relation to hold at all parts on the boundary, because the arguments of the exponential must be the same. This leads to ($k_i=k_r$)

$$\begin{aligned} k_i sin \theta _i=k_r sin \theta _r\Rightarrow \theta _i=\theta _r, \end{aligned}$$

(54)

and

$$\begin{aligned} k_i sin \theta _i= & {} k_t sin \theta _t\nonumber \\\Rightarrow & {} \frac{k_i}{k_t} =\frac{sin\theta _t}{sin \theta _i}=\frac{\omega /c_t}{\omega /c_i} =\frac{c_i}{c_t} =\frac{v_i}{v_t} =\frac{n_t}{n_i}. \end{aligned}$$

(55)

Equations 52 and  53 imply

$$\begin{aligned} P_ie^{j({\varvec{k}}_i \cdot {\varvec{r}})}+ P_re^{j({\varvec{k}}_r \cdot {\varvec{r}})}= P_te^{j({\varvec{k}}_t \cdot {\varvec{r}})}. \end{aligned}$$

(56)

The continuity of the displacement, and hence the velocity

$$\begin{aligned} {\varvec{v}}_i+{\varvec{v}}_r={\varvec{v}}_t, \end{aligned}$$

(57)

leads to, after use of Eq. 51,

$$\begin{aligned} -\frac{P_i}{\rho _ic_i}cos\theta _i+ \frac{P_r}{\rho _rc_r}cos\theta _r= -\frac{P_t}{\rho _tc_t}cos\theta _t. \end{aligned}$$

(58)

We solve for the ratio of the reflected and incident pressures to obtain

$$\begin{aligned} r=\frac{P_r}{P_i}= \frac{\hat{A}cos\theta _i-cos\theta _t}{\hat{A}cos\theta _i+cos\theta _t}, \end{aligned}$$

(59)

where $\hat{A}\mathop {=}\limits ^\mathrm{def}\frac{A_t}{A_i}=\frac{\rho _tc_t}{\rho _ic_i}$, where $\rho _t$ is the medium which the ray encounters (transmitted), $c_t$ is corresponding sound speed in that medium, $A_t$ is the corresponding acoustical impedance, $\rho _i$ is the medium in which the ray was traveling (incident), $c_i$ is corresponding sound speed in that medium $A_i$ is the corresponding acoustical impedance. The relationship (the law of refraction) between the incident and transmitted angles is $c_tsin\theta _t=c_isin\theta _i$. Thus, we may write the Fresnel relation

$$\begin{aligned} r=\frac{\tilde{c}\hat{A}cos\theta _i-(\tilde{c}^2-sin^2\theta _i)^{\frac{1}{2}}}{\tilde{c}\hat{A}cos\theta _i+(\tilde{c}^2-sin^2\theta _i)^{\frac{1}{2}}}, \end{aligned}$$

(60)

where $\tilde{c}\mathop {=}\limits ^\mathrm{def}\frac{c_i}{c_t}$. The reflectance for the (acoustical) energy $\mathcal{R}=r^2$ is

$$\begin{aligned} \mathcal{R}=\left( \frac{P_r}{P_i}\right) ^2= \left( \frac{\hat{A}cos\theta _i-cos\theta _t}{\hat{A}cos\theta _i+cos\theta _t}\right) ^2=\left( \frac{I_r}{I_i}\right) ^2. \end{aligned}$$

(61)

For the cases where $sin \theta _t=\frac{sin \theta _i}{\tilde{c}}>1$, one may rewrite the reflection relation as

$$\begin{aligned} r= \frac{\tilde{c}\hat{A}cos\theta _i-j(sin^2\theta _i-\tilde{c}^2)^{\frac{1}{2}}}{\tilde{c}\hat{A}cos\theta _i+j(sin^2\theta _i-\tilde{c}^2)^{\frac{1}{2}}}. \end{aligned}$$

(62)

where $j=\sqrt{-1}$. The reflectance is $\mathcal{R} \mathop {=}\limits ^\mathrm{def}r \bar{r}=1$, where $\bar{r}$ is the complex conjugate. Thus, for angles above the critical angle $\theta _i \ge \theta ^*_i$, all of the energy is reflected. We note that when $A_t=A_i$ and $c_i=c_t$, then there is no reflection. Also, when $A_t>>A_i$ or when $A_t<<A_i$, then $r\rightarrow 1$.

Remark

If one considers for a moment an incoming pressure wave (ray), which is incident on an interface between two general elastic media ($\mu \ne 0$), reflected shear waves must be generated in order to satisfy continuity of the traction, This is due to the fact that

(63)

For an idealized acoustical medium, $\mu =0$, no shear waves need to be generated to satisfy Eq. 63.

Appendix 2: Contact area parameter and alternative models

1.1 Contact area parameter

Following Zohdi [80], and referring to Fig. 11, one can solve for an approximation of the common contact radius $a_{ij}$ (and the contact area, $A^c_{ij}=\pi a_{ij}^2$) by solving the following three equations,

$$\begin{aligned} a_{ij}^2+L_i^2=R^2_i, \end{aligned}$$

(64)

and

$$\begin{aligned} a_{ij}^2+L_j^2=R^2_j, \end{aligned}$$

(65)

and

$$\begin{aligned} L_i+L_j=||{\varvec{r}}_i-{\varvec{r}}_j||, \end{aligned}$$

(66)

where $R_i$ is the radius of particle i, $R_j$ is the radius of particle j, $L_i$ is the distance from the center of particle i and the common contact interpenetration line and $L_j$ is the distance from the center of particle j and the common contact interpenetration line, where the extent of interpenetration is

$$\begin{aligned} \delta _{ij}=R_i+R_j-||{\varvec{r}}_i-{\varvec{r}}_j||. \end{aligned}$$

(67)

The above equations yield an expression $a_{ij}$, which yields an expression for the contact area parameter

$$\begin{aligned} A^c_{ij}=\pi a_{ij}^2=\pi (R^2_i-L^2_i), \end{aligned}$$

(68)

where

$$\begin{aligned} L_i=\frac{1}{2}\left( ||{\varvec{r}}_i-{\varvec{r}}_j||-\frac{R^2_j-R^2_i}{||{\varvec{r}}_i-{\varvec{r}}_j||}\right) . \end{aligned}$$

(69)

Fig. 11

An approximation of the contact area parameter for two particles in contact (see Zohdi [80])

1.2 Alternative models

One could easily construct more elaborate relations connecting the relative proximity of the particles and other metrics to the contact force, ${\varvec{\Psi }}^{con,n}_{ij}\propto \mathcal{F}({\varvec{r}}_i, {\varvec{r}}_j, {\varvec{n}}_{ij},R_i,R_j,\ldots )$, building on, for example, Hertzian contact models. This poses no difficulty in the direct numerical method developed. For the remainder of the analysis, we shall use the deformation metric in Eq. 14. For detailed treatments, see Wellman et al. [63–67] and Avci and Wriggers [5]. We note that with the appropriate definition of parameters, one can recover Hertz, Bradley, Johnson–Kendell–Roberts, Derjaguin–Muller–Toporov contact models. For example, Hertzian contact is widely used, with the assumptions being

• frictionless, continuous, surfaces,

• each of contacting bodies are elastic half-spaces, whereby the contact area dimensions are smaller radii of the bodies and,

• the bodies remain elastic (infinitesimal strains),

results in the following contact force:

$$\begin{aligned} {\varvec{\Psi }}^{con,n}_{ij}=\frac{4}{3}(R^*)^{1/2}E^*\delta ^{3/2}_{ij}{\varvec{n}}_{ij}, \end{aligned}$$

(70)

which has the general form of ${\varvec{\Psi }}^{con,n}=K^*_{ij}\delta ^p_{ij}$, where

• $R^*=\left( \frac{1}{R_i}+\frac{1}{R_j}\right) ^{-1}$ and

• $E^*=\left( \frac{1-\nu _i^2}{E_i}+\frac{1-\nu _j^2}{E_j}\right) ^{-1}$,

where E is the Young’s modulus and $\nu$ is the Poisson ratio, The contact area with such a model has already been incorporated in the relation above, and is equal to $A^c_{ij}=\pi a^2$ where $a=\sqrt{R^*\delta _{ij}}$. For more details, we refer the reader to Johnson [40]. It is obvious that for a deeper understanding of the deformation within a particle, it must be treated as a deformable continuum, which would require a highly-resolved spatial discretization, for example using the Finite Element Method for the contacting bodies. This requires a large computational effort. For the state of the art in finite element methods and contact mechanics, see the books of Wriggers [69, 70]. For work specifically focusing on the continuum mechanics of particles, see Zohdi and Wriggers [75].

Appendix 3: Iterative solution method

The set of equations represented by Eq. 34 can be solved recursively. Equation 34 can be solved recursively by recasting the relation as

$$\begin{aligned} {\varvec{r}}^{L+1,K}_i= & {} {\varvec{r}}^L_i+{\varvec{v}}^L_i\Delta t +\frac{(\phi \Delta t)^2}{m_i}{\varvec{\Psi }}^{tot,L+1,K-1}_i \nonumber \\&+\frac{\phi (\Delta t)^2}{m_i}(1-\phi ){\varvec{\Psi }}^{tot,L}_i, \end{aligned}$$

(71)

which is of the form

$$\begin{aligned} {\varvec{r}}_i^{L+1,K}=\mathcal{G}({\varvec{r}}_i^{L+1,K-1})+\mathcal{L}_i, \end{aligned}$$

(72)

where $K=1, 2, 3,\ldots$ is the index of iteration within time step $L+1$ and

• ${\varvec{\Psi }}^{tot,L+1,K-1}_i \mathop {=}\limits ^\mathrm{def}{\varvec{\Psi }}^{tot,L+1,K-1}_i\left( {\varvec{r}}^{L+1,K-1}_1, {\varvec{r}}^{L+1,K-1}_2\ldots {\varvec{r}}^{L+1,K-1}_N\right)$,

• ${\varvec{\Psi }}^{tot,L}_i \mathop {=}\limits ^\mathrm{def}{\varvec{\Psi }}^{tot,L}_i\left( {\varvec{r}}^L_1, {\varvec{r}}^L_2\ldots {\varvec{r}}^L_N\right)$,

• $\mathcal{G}({\varvec{r}}_i^{L+1,K-1})=\frac{(\phi \Delta t)^2}{m_i}{\varvec{\Psi }}^{tot,L+1,K-1}_i$ and

• $\mathcal{L}_i={\varvec{r}}^L_i+{\varvec{v}}^L_i\Delta t+\frac{\phi (\Delta t)^2}{m_i}(1-\phi ){\varvec{\Psi }}^{tot,L}_i$.

The term $\mathcal{L}_i$ is a remainder term that does not depend on the solution. The convergence of such a scheme is dependent on the behavior of $\mathcal{G}$. Namely, a sufficient condition for convergence is that $\mathcal{G}$ is a contraction mapping for all ${\varvec{r}}_i^{L+1,K}$, $K=1, 2, 3\ldots$ In order to investigate this further, we define the iteration error as

$$\begin{aligned} \varpi ^{L+1,K}_i \mathop {=}\limits ^\mathrm{def}{\varvec{r}}^{L+1,K}_i-{\varvec{r}}^{L+1}_i. \end{aligned}$$

(73)

A necessary restriction for convergence is iterative self consistency, i.e. the “exact” (discretized) solution must be represented by the scheme, ${\varvec{r}}^{L+1}_i=\mathcal{G}({\varvec{r}}^{L+1}_i)+\mathcal{L}_i$. Enforcing this restriction, a sufficient condition for convergence is the existence of a contraction mapping

$$\begin{aligned} ||\underbrace{{\varvec{r}}^{L+1,K}_i-{\varvec{r}}^{L+1}_i}_{\varpi ^{L+1,K}_i}||= & {} ||\mathcal{G}({\varvec{r}}^{L+1,K-1}_i)-\mathcal{G}({\varvec{r}}^{L+1}_i)|| \nonumber \\\le & {} \eta ^{L+1,K} ||{\varvec{r}}^{L+1,K-1}_i-{\varvec{r}}^{L+1}_i||, \end{aligned}$$

(74)

where, if $0\le \eta ^{L+1,K}<1$ for each iteration K, then $\varpi ^{L+1,K}_i\rightarrow \mathbf{0}$ for any arbitrary starting value ${\varvec{r}}^{L+1,K\,=\,0}_i$, as $K \rightarrow \infty$, which is a contraction condition that is sufficient, but not necessary, for convergence. The convergence of Eq. 71 is scaled by ${\eta \propto \frac{(\phi \Delta t)^2}{m_i}}$. Therefore, we see that the contraction constant of $\mathcal{G}$ is:

• directly dependent on the magnitude of the interaction forces ($||{\varvec{\Psi }}||$),

• inversely proportional to the masses $m_i$ and

• directly proportional to $(\Delta t)^2$.

Thus, decreasing the time step size improves the convergence. In order to maximize the time-step sizes (to decrease overall computing time) and still meet an error tolerance on the numerical solution’s accuracy, we build on an approach originally developed for continuum thermo-chemical multifield problems (Zohdi [71]), where one assumes: (1) $\eta ^{L+1,K} \approx S(\Delta t)^p$, (S is a constant) and (2) the error within an iteration behaves according to $(S (\Delta t)^p)^K\varpi ^{L+1,0}=\varpi ^{L+1,K}$, $K=1, 2,\ldots$, where $\varpi ^{L+1,0}={\varvec{r}}^{L+1,K=1}-{\varvec{r}}^L$ is the initial norm of the iterative (relative) error and S is intrinsic to the system. For example, for second-order problems, due to the quadratic dependency on $\Delta t$, $p \approx 2$. The objective is to meet an error tolerance in exactly a preset (the analyst sets this) number of iterations. To this end, one writes $(S (\Delta t_\mathrm{tol})^p)^{K_{d}}\varpi ^{L+1,0}=TOL$, where TOL is a tolerance and where $K_{d}$ is the number of desired iterations. If the error tolerance is not met in the desired number of iterations, the contraction constant $\eta ^{L+1,K}$ is too large. Accordingly, one can solve for a new smaller step size, under the assumption that S is constant,

$$\begin{aligned} \displaystyle {\Delta t_\mathrm{tol} =\Delta t \left( \frac{(\frac{TOL}{\varpi ^{L+1,0}})^{\frac{1}{pK_{d}}}}{(\frac{\varpi ^{L+1,K}}{\varpi ^{L+1,0}})^{\frac{1}{pK}}}\right) \mathop {=}\limits ^\mathrm{def}\Delta t \Lambda _K}. \end{aligned}$$

(75)

The assumption that S is constant is not critical, since the time steps are to be recursively refined and unrefined throughout the simulation. Clearly, the expression in Eq. 75 can also be used for time step enlargement, if convergence is met in less than $K_d$ iterations (typically chosen to be between five to ten iterations).