Abstract
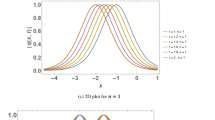
This paper is about the retrieval of highly dispersive optical solitons for Sasa-Satsuma equation with differential group delay in presence of white noise. There are four integration schemes that make this retrieval possible. A full spectrum of optical solitons have been revealed from these schemes. The parametric restrictions for the existence of such solitons are also presented. The displayed surface plots support the analytical findings.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
Sasa-Satsuma equation (SSE) was formulated as the perturbed version of the well-known nonlinear Schrödinger’s equation about three decades ago. This proposed model provided an accurate description of the soliton propagation through optical fibers. The three Hamiltonian perturbative effects are from soliton self-frequency shifts and the self-steepening effects. This model gained popularity and extensive research was conducted with it for decades. While it is only the scalar version of the model that was mostly studied thus far, it is about time to consider SSE further along with a freshly new perspective.
While a plethora of studies have been conducted with regards to stochastic nonlinear evolution equation, the current paper turns the page to give the model an effect of unprecedented novelty [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30]. The model is first considered with higher order dispersions, namely the dispersive effects are from first order to sixth order with the effect of chromatic dispersion (CD) being included. Thus, the dispersive effects stem from inter-model dispersion, CD, third-order dispersion (3OD), fourth-order dispersion (4OD) and fifth-order dispersion (5OD) and finally the sixth-order dispersion (6OD). These dispersive effects together constitute the highly dispersive optical solitons. The self-phase modulation is from Kerr law. Next, this SSE is considered with differential group delay and thus the two-component model is considered. Finally, from a practical perspective, The effect of white noise is included. The resulting coupled stochastic differential equation is studied in fiber optics and its soliton solutions are retrieved.
The coupled model is addressed using a few integration algorithms this led to the retrieval of a full spectrum of optical solitons. It will be observed that the effect of white noise is only present in the phase component of the solitons and not in the amplitude part. The details are all enumerated in the rest of the paper after the model is presented with the technicalities as stated are illustrated.
Governing model
Highly dispersive stochastic in dimensionless form for the first time, the SSE in birefringent fibers with Kerr law nonlinearity, and multiplicative white noise in the Itô sense is expressed as:
and
In the prior system, u(x,t) and v(x,t) are complex-valued functions that reflect the wave profiles & \(i^{2}=-1.\) The first terms in the above system represent the linear temporal evolution. The constants \(\left( a_{lk},l=1,2,k=1,2,\ldots ,6\right)\) correspond to the coefficients of IMD, CD, 3OD, 4OD, 5OD and 6OD respectively. The parameters \(c_{j}\), \(d_{j}\), \(\left( j=1,2\right)\) are the coefficients of SPM and cross-phase modulation respectively. The coefficients of nonlinear dispersion terms are denoted by the constants \(e_{j}\), \(f_{j}\), \(g_{j}\), \(h_{j}\), \(\left( j=1,2\right)\) Finally, \(\sigma _{j}\), \(\left( j=1,2\right)\) represent the noises strength coefficients & \(W_{j}(t)\), \(\left( j=1,2\right)\) give the standard Wiener processes, such that \(dW_{j}(t)/dt\), \(\left( j=1,2\right)\) represent white noise. Also, the terms \(dW_{j}(t)/dt\), \(\left( j=1,2\right)\) are the temporal derivative of the standard Wiener processes.
Mathematical preliminaries
To analyze the stochastic systems (1) and (2), we make the following assumption (3):
and
where \(\kappa _{l},\Omega _{l},\left( l=1,2\right)\) & V are nonzero real-valued constants. The frequencies of the solitons may be calculated from the phase component \(\kappa _{l}\), \(\left( l=1,2\right)\), while \(\Omega _{l},\left( l=1,2\right)\) arise the wave numbers and the velocity soliton is denoted by V. The functions \(P_{1}\left( z\right)\), \(P_{2}\left( z\right)\) & \(\eta _{l}\left( x,t\right)\) are real functions that reflect the amplitude and phase components of solitons, respectively. Inputting (3) into Eqs. (1) and (2) yields the following:
and
Setting
where \(\beta\) is a non zero constant, such that\(\ \beta \ne 1.\) Now, Eqs.(3)–(6) become
and
Equations (10) and (11), when integrated with zero-integration constants, provide the following
When we use Eqs. (12) and (13), which are linearly independent functions, and we set their coefficients to zero, we obtain
also, we gain the velocity of the soliton
and the constraints conditions
where \(a_{15},a_{16},b_{1},b_{2}\) are nonzero constants. Under the constraint conditions, Eqs. (8) and (9) are equal:
From (19), we gain the following:
provided \(a_{16}\ne 0.\) You may rewrite equation (8) as follows:
where
provided \(a_{16}\ne 0.\) The balance number \(N=3\) is obtained by balancing \(P_{1}^{(6)}\left( z\right)\) and \(P_{1}^{3}\left( z\right)\) in Eq. (21). The following methods are implemented in the next sections to discuss Eq. (21).
Integration approaches applied to the model
This section implements the basic mathematical foundations laid down in the previous section to integrate the governing model using four of the integration algorithms that are present in the literature.
Simplest equation method
Equation (21) enables the exact solution:
and \(F\left( z\right)\) fulfil the Bernoulli’s equation
or the Riccati equation
in which \(A_{0},A_{1},A_{2}\), \(A_{3}\), a, b & \(\sigma\) are future-determined constants. Equation (24) has the solutions as:
and
in which \(z_{0}\) is an integration constant, and if \(\sigma < 0\), the following soliton solution structures emerge:
or
which are singular and dark soliton solutions respectively. The remaining cases when \(\sigma > 0\) and \(\sigma = 0\) are excluded since tey do not yield soliton solutions.
Bernoulli’s equation approach
The results are obtained by inserting (24) and (25) into Eq. (21), collecting all the coefficients of each power \(F^{s}\left( z\right) ,\left( s=0,1,..,9\right) ,\)and setting these coefficients to zero:
provided \(\Delta _{3}<0.\)
(I) If \(a>0,\) \(b<0,\) one gains
(II) If \(a<0,\) \(b>0,\) we obtain
For example, if \(a=1,b=-1\) or \(a=-1,b=1,\) the combo dark soliton solutions are available:
Riccati equation scheme
The following results are obtained by inserting (24) and (26) into Eq. (21), accumulating the coefficients of each power \(F^{s}\left( z\right)\), \(\left( s=0,1,2,..,9\right)\), and then setting each of these coefficients to zero:
provided \(\Delta _{3}<0\). Now, one finds the soliton solutions to Equations (1) and (2) outlined below for \(\Delta _{0}<0\), after ignoring the remaining cases when \(\Delta _0 > )\) or \(\Delta _0 = 0\), which do not give way to soliton solutions:
The straddled singular solitons are:
and the straddled dark soliton solutions are:
Extended simplest equation algorithm
Equation (21), which relies on the explicit solution:
where \(\chi _{0},\chi _{1},\chi _{2},\chi _{3},B_{0},B_{1}\) and \(B_{2}\) are constants, \(\chi _{3}^{2}+B_{2}^{2}\ne 0\) and the function \(\phi \left( z\right)\) presumes the auxiliary equation
in where \(\delta\) and \(\upsilon _{0}\) are integers. The case when \(\delta < 0\) is considered here since the other two cases, namely when \(\delta = 0\) or \(\delta >0\) are discarded since they do not yield soliton solutions.
Here, we replace (43) for Eq.(21) and apply Eq.(43) together with the connection
where \(L_{1}=\delta \left( \rho _{1}^{2}-\rho _{2}^{2}\right) -\frac{\upsilon _{0}^{2}}{\delta },\) yields the following solutions even when \(\rho _{1}\) and \(\rho _{2}\) are constants.
Solution–1:
provided \(\Delta _{3}<0\), \(\left( \rho _{2}^{2}+\frac{\upsilon _{0}^{2}}{ \delta ^{2}}\right) >0\) and \(\upsilon _{0}\ne 0\). As a result, the solitary solutions to Eqs.(1) and (2) are as follows:
and
where
Solution–2:
provided \(\left( \rho _{1}^{2}-\rho _{2}^{2}\right) \Delta _{3}>0\). In light of this, we arrive at the subsequent solitary solutions to Eqs. (1) and (2):
and
where
We get the combo-singular soliton solutions if we put \(\rho _{1}=0,\rho _{2}\ne 0,\) in (51) and (52):
and
provided \(\Delta _{3}<0.\) The combo-bright soliton solutions are available if we put \(\rho _{1}\ne 0,\rho _{2}=0:\)
and
provided \(\Delta _{3}>0.\)
Solution–3:
provided \(\Delta _{3}<0\). As a result, we arrive at the solitary solutions of equations (1) and (2) as follows:
and
We get the combo-singular soliton solutions in (59) and (60), specifically if we put \(\rho _{1}=0,\rho _{2}\ne 0:\)
and
while in (59) and (60), the combo-dark soliton solutions are obtained if we set \(\rho _{1}\ne 0,\rho _{2}=0:\)
and
Note that, when \(\Delta _{0}=332\delta ,\) the solutions (61)-(64) are similar to the solutions (39)-(42).
Conclusions
The current paper retrieved a full spectrum of highly dispersive optical solitons for SSE in birefringent fibers in presence of white noise when the SPM is of Kerr type. A wide range of integration algorithms has made this retrieval possible. It has been observed that the effect of white noise stays confined to the phase component of the solitons and never enters the amplitude portion of such pulses. The results are thus overwhelming and stand strong for future activities in this field. The model is to be next considered with additional forms of SPM that would produce further interesting results. Additionally, later the model would be generalized to dispersion-flattened fibers. That’s when the studies are going to get more interesting. The results of such research activities will be disseminated across the board with time after they are colineared with the pre-existing ones [26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54].
References
M.A.E. Abdelrahman, W.W. Mohammed, M. Alesemi, S. Albosaily, The effect of multiplicative noise on the exact solutions of nonlinear Schrodinger equation. AIMS Math. 6, 2970–2980 (2021)
S. Albosaily, W.W. Mohammed, M.A. Aiyashi, A.A.E. Abdelrahman, Exact solutions of the (2+1)-dimensional stochastic chiral nonlinear Schrödinger equation. Symmetry 12, 1874–1886 (2020)
W.W. Mohammed, H. Ahmad, A.E. Hamza, E.S. Aly, M. El-Morshedy, E.M. Elabbasy, The exact solutions of the stochastic Ginzburg-Landau equation. Results Phys. 23, 103988 (2021)
Mohammed, W.W., Ahmad, H. Boulares, H. Kheli, F. & El-Morshedy, M. Exact solutions of HirotaMaccari system forced by multiplicative noise in the Itô sense. J. Low Freq. Noise Vib. Active Control. https://doi.org/10.1177/14613484211028100 (2021)
W.W. Mohammed, N. Iqbal, A. Ali, M. El-Morshedy, Exact solutions of the stochastic new coupled Konno-Oono equation. Results Phys. 21, 103830 (2021)
W.W. Mohammed, M. El-Morshedy, The influence of multiplicative noise on the stochastic exact solutions of the Nizhnik-Novikov-Veselov system. Math. Comput. Simul. 190, 192–202 (2021)
W.W. Mohammed, S. Albosaily, N. Iqbal, M. El-Morshedy, The effect of multiplicative noise on the exact solutions of the stochastic Burger equation. Waves Random Complex Media. https://doi.org/10.1080/17455030.2021.1905914 (2021)
N.A. Kudryashov, E.V. Antonova, Solitary waves of equation for propagation pulse with power nonlinearities. Optik 217, 164881 (2020)
N.A. Kudryashov, A generalized model for description of propagation pulses in optical fiber. Optik 189, 42–52 (2019)
N.A. Kudryashov, Mathematical model of propagation pulse in optical fiber with power nonlinearities. Optik 212, 164750 (2020)
N.A. Kudryashov, Method for finding highly dispersive optical solitons of nonlinear differential equations. Optik 206, 163550 (2020)
N.A. Kudryashov, Highly dispersive optical solitons of the generalized nonlinear eighth-order Schrödinger equation. Optik 206, 164335 (2020)
N.A. Kudryashov, Solitary wave solutions of hierarchy with non-local nonlinearity. Appl. Math. Lett. 103, 106155 (2020)
N.A. Kudryashov, Highly dispersive solitary wave solutions of perturbed nonlinear Schrödinger equations. Appl. Math. Comput. 371, 124972 (2020)
N.A. Kudryashov, Construction of nonlinear differential equations for description of propagation pulses in optical fiber. Optik 192, 162964 (2019)
N.A. Kudryashov, Solitary waves of the generalized Sasa-Satsuma equation with arbitrary refractive index. Optik 232, 166540 (2021)
U. Bandelow, N. Akhmediev, Sasa-Satsuma equation: soliton on a background and its limiting cases. Phys. Rev. E. 86, 026606 (2012)
O.G. Gonzalez, A. Biswas, M. Ekici, A.S. Alshomrani, Optical solitons with Sasa-Satsuma equation by Laplace-Adomian decomposition algorithm. Optik 229, 166262 (2021)
F. Sun, Optical solutions of Sasa-Satsuma equation in optical fibers. Optik 228, 166127 (2021)
J. Xu, E. Fan, The unified transform method for the Sasa-Satsuma equation on the half-line. Proc. Roy. Soc. A. 469, 20130068 (2013)
M. Hayek, Exact and traveling-wave solutions for convection-diffusion-reaction equation with power-law nonlinearity. Appl. Math. Comput. 218, 2407–2420 (2011)
N.A. Kudryashov, Exact solitary waves of the Fisher equation. Phys. Lett. A 342, 99–106 (2005)
N.A. Kudryashov, Simplest equation method to look for exact solutions of nonlinear differential equations. Chaos, Solitons, Fractals 24, 1217–1231 (2005)
S. Bilige, T. Chaolu, An extended simplest equation method and its application to several forms of the fifth-order KdV equation. Appl. Math. Comput. 216, 31463153 (2010)
W. Zhang, X. Ling, B.-B. Wang, S. Li, Solitary and periodic wave solutions of Sasa-Satsuma equation and their relationship with Hamilton energy. Complexity 2020, 8760179 (2020)
Y.P. Zhang, L. Yu, G.M. Wei, Integrable aspects and rogue wave solution of Sasa-Satsuma equation with variable coefficients in the inhomogeneous fiber. Modern Phys. Lett. B. 32, 1850059 (2018)
L.-C. Zhao, S.-C. Li, L. Ling, Rational W-shaped solitons on a continuous-wave background in the Sasa-Satsuma equation. Phys. Rev. E 89, 023210 (2014)
N. Jihad, M.A.A. Almuhsan, Evaluation of impairment mitigations for optical fiber communications using dispersion compensation techniques. Al-Rafidain J. Eng. Sci. 1(1), 81–92 (2023)
Z. Li, E. Zhu, Optical soliton solutions of stochastic Schrödinger-Hirota equation in birefringent fibers with spatiotemporal dispersion and parabolic law nonlinearity. J. Opt. https://doi.org/10.1007/s12596-023-01287-7 (2023)
S. Nandy, V. Lakshminarayanan, Adomian decomposition of scalar and coupled nonlinear Schrödinger equations and dark and bright solitary wave solutions. J. Opt. 44, 397–404 (2015)
Y.S. Ozkan, E. Yasar, Three efficient schemes and highly dispersive optical Solitons of perturbed Fokas-Lenells equation in stochastic form. Ukrainian J. Phys. Opt. 25, S1017–S1038 (2024)
L. Tang, Bifurcations and optical solitons for the coupled nonlinear Schrödinger equation in optical fiber Bragg gratings. To appear in J. Opt. https://doi.org/10.1007/s12596-022-00963-4
L. Tang, Phase portraits and multiple optical solitons perturbation in optical fibers with the nonlinear Fokas-Lenells equation. J. Opt. 52(4), 2214–2223 (2023)
A.J.M. Jawad, M.J. Abu-AlShaeer, Highly dispersive optical solitons with cubic law and cubic-quintic-septic law nonlinearities by two methods. Al-Rafidain J. Eng. Sci. 1(1), 1–8 (2023)
A.R. Adem, T.J. Podile, B. Muatjetjeja, A generalized (3+ 1)-dimensional nonlinear wave equation in liquid with gas bubbles: symmetry reductions; exact solutions; conservation laws. Int. J. Appl. Comput. Math. 9(5), 82 (2023)
I. Humbu, B. Muatjetjeja, T.G. Motsumi, A.R. Adem, Solitary waves solutions and local conserved vectors for extended quantum Zakharov-Kuznetsov equation. Eur. Phys. J. Plus 138(9), 873 (2023)
M.C. Sebogodi, B. Muatjetjeja, A.R. Adem, Exact solutions and conservation laws of a (E +)-dimensional combined potential Kadomtsev-Petviashvili-b-type kadomtsev-petviashvili equation. Int. J. Theor. Phys. 62(8), 165 (2023)
I. Humbu, B. Muatjetjeja, T.G. Motsumi, A.R. Adem, Periodic solutions and symmetry reductions of a generalized Chaffee-Infante equation. Partial Diff. Eq. Appl. Math. 7, 100497 (2023)
A.R. Adem, T.S. Moretlo, B. Muatjetjeja, A generalized dispersive water waves system: conservation laws; symmetry reduction; travelling wave solutions; symbolic computation. Partial Diff. Eq. Appl. Math. 7, 100465 (2023)
A.R. Adem, B. Muatjetjeja, T.S. Moretlo, An extended (2+ 1)-dimensional coupled burgers system in fluid mechanics: symmetry reductions; Kudryashov method; conservation laws. Int. J. Theor. Phys. 62(2), 38 (2023)
A.R. Adem, B. Muatjetjeja, Conservation laws and exact solutions for a 2D Zakharov-Kuznetsov equation. Appl. Math. Lett. 48, 109–117 (2015)
A.R. Adem, The generalized (1+ 1)-dimensional and (2+ 1)-dimensional Ito equations: multiple exp-function algorithm and multiple wave solutions. Comput. Math. Appl. 71(6), 1248–1258 (2016)
A.R. Adem, X. Lü, Travelling wave solutions of a two-dimensional generalized Sawada-Kotera equation. Nonlinear Dyn. 84, 915–922 (2016)
A.R. Adem, Solitary and periodic wave solutions of the Majda-Biello system. Modern Phys. Lett. B 30(15), 1650237 (2016)
A.R. Adem, A (2+ 1)-dimensional Korteweg-de Vries type equation in water waves: lie symmetry analysis; multiple exp-function method; conservation laws. Int. J. Modern Phys. B 30(28n29), 1640001 (2016)
S.O. Mbusi, A.R. Adem, B. Muatjetjeja, Lie symmetry analysis, multiple exp-function method and conservation laws for the (2+ 1)-dimensional Boussinesq equation. Opt. Quant. Electron. 56(4), 1–16 (2024)
I. Humbu, B. Muatjetjeja, T.G. Motsumi, A.R. Adem, Multiple solitons, periodic solutions and other exact solutions of a generalized extended (2+ 1)-dimensional Kadomstev-Petviashvili equation. J. Appl. Anal. https://doi.org/10.1515/jaa-2023-0082 (2024)
E.M. Zayed, M.E. Alngar, R.M. Shohib, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, P.L. Georgescu, Dispersive optical solitons with differential group delay having multiplicative white noise by ito calculus. Electronics 12(3), 634 (2023)
A.H. Arnous, A. Biswas, A.H. Kara, Y. Yıldırım, L. Moraru, S. Moldovanu, P. L. Georgescu, A.A. Alghamdi, Dispersive optical solitons and conservation laws of Radhakrishnan-Kundu-Lakshmanan equation with dual-power law nonlinearity. Heliyon 9(3), e14036 (2023)
E.M. Zayed, M. El-Horbaty, M.E. Alngar, R.M. Shohib, A. Biswas, Y. Yıldırım, L. Moraru, C. Iticescu, D. Bibicu, P.L. Georgescu, A. Asiri, Dynamical system of optical soliton parameters by variational principle (super-Gaussian and super-sech pulses). J. Eur. Opt. Soc. Rapid Publi. 19(2), 38 (2023)
M.A. Reham Shohib, E.M. Alngar Mohamed, B. Anjan, Y. Yakup, T. Houria, M. Luminita, I. Catalina, G.P. Lucian, A. Asim, Optical solitons in magneto-optic waveguides for the concatenation model. Ukr. J. Phys. Opt. 24, 248–261 (2023)
Ahmed H. Arnous, Biswas Anjan, Yildirim Yakup, Moraru Luminita, Iticescu Catalina, Georgescu Puiu Lucian, Asiri Asim, Optical solitons and complexitons for the concatenation model in birefringent fibers. Ukr. J. Phys. Opt. 24, 04060–04086 (2023)
Elsayed M. E. Zayed, Mohamed E. M. Alngar, Reham M. A. Shohib, Anjan Biswas, Yakup Yildirim, Luminita Moraru, Puiu Lucian Georgescu, Catalina Iticescu, Asim Asiri, Highly dispersive solitons in optical couplers with metamaterials having Kerr law of nonlinear refractive index. Ukr. J. Phys. Opt. 25, 01001–01019 (2024)
A. Jawad, A. Biswas, Solutions of resonant nonlinear Schrödinger’s equation with exotic non-Kerr law nonlinearities. Al-Rafidain J. Eng. Sci. 2(1), 43–50 (2024)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Zayed, E.M.E., Shohib, R.M.A., Alngar, M.E.M. et al. Highly dispersive optical solitons with differential group delay for Sasa-Satsuma equation having multiplicative white noise. J Opt (2024). https://doi.org/10.1007/s12596-024-01801-5
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s12596-024-01801-5