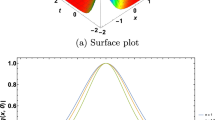
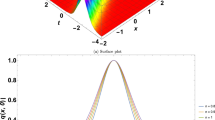
Abstract
This paper recovers optical solitons to the newly proposed dispersive concatenation model that comes with power law of self-phase modulation. The presence of white noise in the Itô sence makes the model stochastic. Two integration approaches retrieve bright and singular optical solitons. The intermediary Weierstrass’ elliptic functions are implemented for this retrieval. It has been established that the effect of white noise stays confined to the phase component of the solitons.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
The concepts of the concatenation model and dispersive concatenation model were sequentially conceived a decade ago [1,2,3,4,5]. Subsequently, extensive studies with these two models were carried out during the past couple of years. These range from the retrieval of soliton solutions and the conservation laws, studying the model with power law of self-phase modulation, addressing the Internet bottleneck effect and minimizing the slowdown of soliton transmission by introducing the spatiotemporal dispersion (STD) in addition to the pre-existing chromatic dispersion (CD). The numerical analysis of solitons for the concatenation model by the Laplace–Adomian decomposition was also conducted. Recently, the effect of white noise in soliton transmission for the concatenation model with Kerr law and power law of SPM yielded interesting results [6,7,8,9,10,11,12,13,14,15]. The current paper addresses the effect of white noise for the dispersive concatenation model that is studied in the current paper with power law of SPM. The results of the current paper follow a previously published prequel paper on dispersive optical solitons with the Kerr law of SPM, as recently reported [13].
The dispersive concatenation model is the combination of the Schrödinger–Hirota equation (SHE), Lakshmanan–Porsezian–Daniel (LPD) model and the dispersive nonlinear Schrödinger’s equation (NLSE) that carries a fifth–order dispersive effect. Therefore, the model is truly dispersive as the name bears. The current paper will address this model with power law of SPM as a sequel to the Kerr law [13]. The white noise in Itô sense is included as a source of stochasticity to the model. Two integration approaches will lead to the soliton solutions. These are the enhanced Kudryashov’s approach and the extended auxiliary equation scheme. These two algorithms would lead to the emergence of bright and singular optical solitons only. These approaches fail to recover the dark optical solitons as expected since it is well known, and experimentally proved in the past, that dark solitons are not supported by any model with power law of SPM unless the power law collapses to Kerr law. The details of the concepts and the derivation of the results are exhibited in the rest of the paper after a succinct recapitulation of the integration methodologies.
Governing model
In [1], the concatenation model with higher-order dispersion effects and power law nonlinearity was studied. In this study, we consider the dimensionless expression of this model by incorporating multiplicative white noise effect, which can be expressed as follows:
Here, q(x, t) represents a complex-valued function that describes the wave profile. In this context, x and t represent the spatial and temporal coordinates, respectively. The parameters a and b correspond to CD and SPM, respectively, of the NLSE with power law of SPM. The symbol \(i=\sqrt{-1}\) is the imaginary unit. The coefficients \(\delta _1,~\delta _2\) and \(\delta _3\) are the nonzero parameters associated with SHE, LPD model and the fifth-order NLSE, respectively. The variable \(\sigma\) is used to represent a nonzero constant value and serves as the sign for indicating the intensity of white noise. Furthermore, the standard Wiener process, represented as W(t), can be defined as the integral of the function \(\Lambda (\eta ) = dW(t)/dt\) concerning the Wiener process \(W(\eta )\), where \(\eta\) is a variable that assumes values smaller than t. In the provided context, the symbol \(\eta\) represents a stochastic variable, whereas \(\Lambda (\eta )\) is utilized to denote typical Gaussian white noise, popularly known as multiplicative white noise.
Mathematical analysis of the governing model
For investigating a model with a multiplicative white noise effect, the following wave structure is selected:
The wave variable \(\xi\) is defined as
where k and v represent nonzero constants. In Eq. (2), \(U(\xi )\) denotes a real-valued function representing the soliton solutions’ amplitude components. The variable v corresponds to the speed of the soliton, while k represents the wave width. In the provided context, the symbol \(\kappa\) represents the frequency of the solitons. The symbol \(\omega\) is used to describe the wave number. The symbol \(\sigma\) is employed to signify the noise coefficient. Lastly, the symbol \(\theta _0\) represents the phase constant. The following formulas are obtained by substituting Eq. (2) into Eq. (1) and subsequently decomposing them into their real component
and imaginary component
From the imaginary part, the soliton speed reads as
and the soliton frequency as
with the following parametric restrictions
Under the previous conditions, Eq. (1) will have the following form
Additionally, Eq. (4) will take on the following form
with
Provided that \(\sigma _3\ne 0\). Using the transformation
Equation (10) collapses to
In terms of integrability, it is observed that we possess
This assumption leads \(\sigma _5=0\). In this case, Eq. (12) reads
Balancing \(V^3 V^{\prime \prime \prime \prime }\) with \(V^5 V^{\prime \prime }\) or \(V^6\) in Eq. (14) gives \(N=1\) or \(N=2\).
An outline of the integration algorithms
We could include a governing model which has the structure of
where \(u=u(x,t)\) represents a wave profile, where t and x describe the time and space variables, respectively.
The use of the wave transformation
causes a reduction of Eq. (15) to
In that expression, k represents the wave width, \(\xi\) represents the wave variable, and \(\upsilon\) represents the wave velocity.
The enhanced Kudryashov’s method
This subsection presents a thorough overview of the basic procedures with the enhanced Kudryashov technique.
Step 1: The explicit solution for the reduced model Eq. (17) is provided as follows
along with the auxiliary equation
The constants \(\sigma _{0}\), \(\chi\), \(\sigma _{i}\), and \(\rho _{i}\) (where \(i=1,..., N\)) will be provided, with N determined by the balancing procedure in Eq. (17).
Step 2: Eq. (19) gives the soliton waves
where c is nonzero constant.
Step 3: By inserting Eq. (18) into Eq. (17), together with Eq. (19), we can derive the requisite constants for Eq. (16) and (18). In order to incorporate the identified parametric restrictions, they can be substituted into Eq. (18) together with Eq. (20). Consequently, straddled solitons are obtained, which can be further classified as bright, dark, or singular solitons.
The extended auxiliary equation scheme
This subsection presents a thorough overview of the basic procedures with the extended auxiliary equation technique.
Step 2: We assume that the solution of Eq. (17) can be expressed in the form
where \(\theta (\xi )\) satisfies
This equation gives various kinds of solutions as follows
Case 1 \({\tau _0} = {\tau _1} = {\tau _3} = 0.\) Bright and singular soliton solutions are obtained:
and
Case 2 \({\tau _0} =\frac{{\tau _2}^2}{4\tau _4},~ {\tau _1} = {\tau _3} = 0.\)
Dark and singular soliton solutions are obtained:
and
Case 3 \(\tau _1=\tau _3=0.\) A Weierstrass elliptic doubly periodic type solution is obtained:
where \({g_2} =\frac{\tau _2^2}{12}+\tau _0 \tau _4\) and \({g_3} =\frac{ \tau _2 \left( 36 \tau _0 \tau _4-\tau _2^2\right) }{216}\) are called invariants of the Weierstrass elliptic function.
Case 4 \({\tau _0} = {\tau _1} = 0,~{\tau _2,~\tau _4} > 0, ~{\tau _3} \ne \pm 2 \sqrt{{\tau _2}{\tau _4}}\) Straddled soliton solutions are obtained:
and
Step 3: Determine the positive integer number N in Eq. (8) by balancing the highest order derivatives and the nonlinear terms in Eq. (17).
Step 4: Substitute (21) into (17) along with (22). As a result of this substitution, we get a polynomial of \(\theta (\xi )\). In this polynomial we gather all terms of same powers and equating them to be zero, we get an over-determined system of algebraic equations which can be solved together to get the unknown parameters \(k,v,\alpha _0,\alpha _i\) and \(\beta _i ~(i=1,2,...)\). Consequently, we obtain the exact solutions of (15).
Application to the governing model
This section uses the two preceding techniques discussed in this work. Using these methods, we predict the emergence of bright and dark soliton solutions. This section will be divided into two subsections. At first, we will study the governing model in the case when the balance constant \(N=1\). Subsequently, we will investigate the governing model under the issue where the balance constant \(N=2\).
Case 1 \(N=1\)
The enhanced Kudryashov method
In accordance with the enhanced Kudryashov technique, the solution is expressed in the following structure
Plugging Eq. (30) together with Eq. (19) into Eq. (14), we get a system of algebraic equations. Solving these equations together yields the following result:
As a consequence, we obtain the exact solutions of Eq. (1) as follows
Set \(\chi =\pm 4 c^2\) in solution (32). Consequently, for \(w _4 n^2 \left( 6 n^2+5 n+1\right) - w _8 \left( 4 n^3+2 n^2+n+1\right)\) and \({ w _8 w _6 (n+1)}>0\), we have bright soliton with \({ w _6}>0\) and singular soliton with \({ w _6}<0\)
and
The extended auxiliary equation method
In accordance with the extended auxiliary equation technique, the solution is expressed in the following structure
Plugging Eq. (35) together with Eq. (22) into Eq. (14), we get a system of algebraic equations. Solving these equations together yields the following results:
Case 1 \(\tau _0=\tau _1=\tau _3=0.\)
For this case, the solution of (1) reads
and
These solitons with \(\tau _2>0\) are bright for \(\tau _4<0\) and singular for \(\tau _4>0\).
Case 2 \(\tau _1=0,~\tau _3=0,~\tau _0=\frac{\tau _2^2}{4 \tau _4}\)
For this case, the solution of (1) reads
The obtained soliton is singular with \(\tau _2<0\) and \(\tau _4>0\).
Case 3 \(\tau _1=0,~\tau _3=0\)
For this case, the solution of (1) reads
where
The Weierstrass elliptic doubly periodic type solution (42) with its restricted invariants (43) can be converted to a singular soliton solution
Case 4 \(\tau _0=0,~\tau _1=0\)
For this case, the obtained solitons are bright for \(\tau _2>0\) and \(\tau _4<0\) and singular for \(\tau _2>0\) and \(\tau _4>0\)
and
Case 2\(N=2\)
The enhanced Kudryashov method
In accordance with the enhanced Kudryashov technique, the solution is expressed in the following structure
Insert (48) together with (19) into Eq. (14) to get a system of algebraic equations. Solving these equations together yields the following result:
As a consequence, the solution of Eq. (1) reaches
Set \(\chi =\pm 4 c^2\) to recover bright and singular solitons for \(w _4<0\) and \(w _6<0\)
and
The extended auxiliary equation method
In accordance with the extended auxiliary equation technique, the solution is expressed in the following structure
Plugging Eq. (53) together with Eq. (22) into Eq. (14), we get a system of algebraic equations. Solving these equations together yields the following results:
Case 1 \(\tau _0=\tau _1=\tau _3=0.\)
For this case, the solution of (1) reads
and
These solutions are bright and singular solitons with \(w _4<0\) and \(w _6<0\).
Case 2 \(\tau _0=\frac{\tau _2^2}{4 \tau _4},~\tau _1=\tau _3=0.\)
For this case, the solution of (1) reads
and
Case 3 \(\tau _1=\tau _3=0.\)
For this case, the solution of (1) reads
where
The Weierstrass elliptic doubly periodic type solution (61) with its restricted invariants (62) can be converted to a singular soliton solution
Case 4 \(\tau _0=\tau _1=0.\)
For this case, the solution of (1) reads
and
These solutions are bright and singular solitons with \(w _4<0\) and \(w _6<0\).
The enhanced Kudryashov’s approach and the extended auxiliary equation technique were unsuccessful in retrieving dark solitons within the constraints of the governing model.
Conclusions
The paper studied the dispersive concatenation model with power law of SPM in presence of white noise. The bright and singular soliton solutions to the model were retrieved using a couple of integration algorithms. They are the enhanced Kudryashov’s approach and the extended auxiliary equation scheme. These methodologies failed to furnish the dark soliton solutions to the model. This is because of the fact that the model with power law of SPM can recover dark solitons only if the power law collapses to Kerr law, a fact that has been experimentally proven earlier. There is another observation that has been made in the work. The effect of white noise is confined to the phase component of the bright and singular soliton solutions and does not affect the amplitude components of such solitons.
The results of the paper are thus indeed promising. The studies with the current model can be extended further. For example, this research can be carried out with additional optoelectronic devices such as optical couplers, optical metamaterials, PCF, gap solitons and many others. Later, the studies can be extended to fibers with differential group delay as well as dispersion–flattened fibers. The numerical scheme such as variational iteration method and/or Laplace–Adomian decomposition schemes will be implemented to achieve a numerical picture to the studies. The bifurcation analysis and additional analytical aspects are yet to be covered. These results will be disseminated after they are available and aligned with the pre–existing ones [16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58]
References
A. Ankiewicz, N. Akhmediev, Higher-order integrable evolution equation and its soliton solutions. Phys. Lett. A 378, 358–361 (2014)
A. Ankiewicz, Y. Wang, S. Wabnitz, N. Akhmediev, Extended nonlinear Schrödinger equation with higher-order odd and even terms and its rogue wave solutions. Phys. Rev. E 89, 012907 (2014)
A. Chowdury, D.J. Kedziora, A. Ankiewicz, N. Akhmediev, Soliton solutions of an integrable nonlinear Schrödinger equation with quintic terms. Phys. Rev. E 91, 032922 (2014)
A. Chowdury, D.J. Kedziora, A. Ankiewicz, N. Akhmediev, Breather-to-soliton conversions described by the quintic equation of the nonlinear Schrödinger hierarchy. Phys. Rev. E 91, 032928 (2015)
A. Chowdury, D.J. Kedziora, A. Ankiewicz, N. Akhmediev, Breather solutions of the integrable quintic nonlinear Schrödinger equation and their interactions. Phys. Rev. E 91, 022919 (2015)
A. H. Arnous, A. Biswas, A. H. Kara, Y. Yıldırım & A. Asiri. Optical solitons and conservation laws for the dispersive concatenation model with power–law nonlinearity. J. Opt. (2023) https://doi.org/10.1007/s12596-023-01453-x
A.H. Arnous, A. Biswas, Y. Yildirim, A. Asiri, Quiescent optical solitons for the concatenation model having nonlinear chromatic dispersion with differential group delay. Contemp. Math. 4(4), 877–904 (2023)
A. H. Arnous, M. Mirzazadeh, A. Biswas, Y. Yildirim, H. Triki & A. Asiri. A wide spectrum of optical solitons for the dispersive concatenation model. J. Opti. https://doi.org/10.1007/s12596-023-01383-8
E. M. E. Zayed, A. H. Arnous, A. Biswas, Y. Yildirim & A. Asiri. Optical solitons for the concatenation model with multiplicative white noise. J. Opt. (2023) https://doi.org/10.1007/s12596-023-01381-w
A.H. Arnous, Optical solitons with Biswas–Milovic equation in magneto-optic waveguide having Kudryashov’s law of refractive index’’. Optik 247, 167987 (2021). https://doi.org/10.1016/j.ijleo.2021.167987
A.M. Elsherbany, A.H. Arnous, A.J.M. Jawad, A. Biswas, Y. Yildirim, L. Moraru, A.S. Alshomrani, Quiescent optical solitons for the dispersive concatenation model with Kerr law of nonlinearity having nonlinear chromatic dispersion. Ukr. J. Phys. Opt. 25(1), 01054–01064 (2024)
A. H. Arnous, A. Biswas, Y. Yildirim, A. S. Alshomrani. Stochastic perturbation of optical solitons for the concatenation model with power–law of self–phase modulation having multiplicative white noise. Contemp. Math. (2024) https://doi.org/10.37256/cm.5120244107
A. H. Arnous, A. Biswas, Y. Yildirim & A. S. Alshomrani. Optical solitons with dispersive concatenation model having multiplicative white noise by the enhanced direct algebraic method. Submitted
A. Biswas, J.M. Vega-Guzman, Y. Yildirim, S.P. Moshokoa, M. Aphane, A.A. Alghamdi, Optical solitons for the concatenation model with power-law nonlinearity: undetermined coefficients. Ukr. J. Phys. Opt. 24(3), 185–192 (2023)
O. Gonzalez-Gaxiola, A. Biswas, J.R. de Chavez, A. Asiri, Bright and dark optical solitons for the concatenation model by Laplace-Adomian decomposition scheme. Ukr. J. Phys. Opt. 24(3), 222–234 (2023)
A.J.M. Jawad, M.J.A. Al-Shaeer, Highly dispersive optical solitons with cubic law and cubic-quintic-septic law nonlinearities by two methods. Al-Rafidain J. Eng. Sci. 1(1), 1–8 (2023)
A.J.M. Jawad, A. Biswas, Solutions of resonant nonlinear Schrödinger’s equation with exotic non-Kerr law nonlinearities. Al-Rafidain J. Eng. Sci. 2(1), 43–50 (2024)
N. Jihad, M.A.A. Almuhsan, Evaluation of impairment mitigations for optical fiber communications using dispersion compensation techniques. Al-Rafidain J. Eng. Sci. 1(1), 81–92 (2023)
Z. Li & E. Zhu. Optical soliton solutions of stochastic Schrödinger-Hirota equation in birefringent fibers with spatiotemporal dispersion and parabolic law nonlinearity". J. Opt. https://doi.org/10.1007/s12596-023-01287-7
S. Nandy, V. Lakshminarayanan, Adomian decomposition of scalar and coupled nonlinear Schrödinger equations and dark and bright solitary wave solutions. J. Opt. 44, 397–404 (2015)
A. Secer, Stochastic optical solitons with multiplicative white noise via Itô calculus. Optik 268, 169831 (2022)
L. Tang. Bifurcations and optical solitons for the coupled nonlinear Schrödinger equation in optical fiber Bragg gratings. J. Opt. 52, 1388–1398. https://doi.org/10.1007/s12596-022-00963-4
L. Tang, Phase portraits and multiple optical solitons perturbation in optical fibers with the nonlinear Fokas–Lenells equation. J. Opt. 52(4), 2214–2223 (2023)
Y. Zhong, H. Triki, Q. Zhou, Analytical and numerical study of chirped optical solitons in a spatially inhomogeneous polynomial law fiber with parity-time symmetry potential. Commun. Theor. Phys. 75, 025003 (2023)
Q. Zhou, Influence of parameters of optical fibers on optical soliton interactions. Chin. Phys. Lett. 39(1), 010501 (2022)
E.M. Zayed, R.M. Shohib, A.G. Al-Nowehy, On solving the (3+ 1)-dimensional NLEQZK equation and the (3+ 1)-dimensional NLmZK equation using the extended simplest equation method. Comput. Math. Appl. 78(10), 3390–3407 (2019)
E.M. Zayed, R.M. Shohib, A.G. Al-Nowehy, Solitons and other solutions for higher-order NLS equation and quantum ZK equation using the extended simplest equation method. Comput. Math. Appl. 76(9), 2286–2303 (2018)
E.M. Zayed, M.E. Alngar, R.M. Shohib, Cubic-quartic embedded solitons with \(\chi\) (2) and \(\chi\) (3) nonlinear susceptibilities having multiplicative white noise via Itô calculus. Chaos Solitons Fract. 168, 113186 (2023)
E.M. Zayed, M.E. Alngar, R.M. Shohib, Dispersive optical solitons to stochastic resonant NLSE with both spatio-temporal and inter-modal dispersions having multiplicative white noise. Mathematics 10(17), 3197 (2022)
E.M. Zayed, R.M. Shohib, M.E. Alngar, Cubic-quartic optical solitons in Bragg gratings fibers for NLSE having parabolic non-local law nonlinearity using two integration schemes. Opt. Quant. Electron. 53(8), 452 (2021)
E.M. Zayed, K.A. Gepreel, R.M. Shohib, M.E. Alngar, Solitons in magneto-optics waveguides for the nonlinear Biswas–Milovic equation with Kudryashov’s law of refractive index using the unified auxiliary equation method. Optik 235, 166602 (2021)
E.M. Zayed, K.A. Gepreel, R.M. Shohib, M.E. Alngar, Y. Yıldırım, Optical solitons for the perturbed Biswas–Milovic equation with Kudryashov’s law of refractive index by the unified auxiliary equation method. Optik 230, 166286 (2021)
Zayed, E. M. E., & Shohib, R. M. A. (2019). Solitons and other solutions for two higher-order nonlinear wave equations of KdV type using the unified auxiliary equation method. Acta Physica Polonica A 136(1)
E.M. Zayed, R.M. Shohib, K.A. Gepreel, M.M. El-Horbaty, M.E. Alngar, Cubic-quartic optical soliton perturbation Biswas–Milovic equation with Kudryashov’s law of refractive index using two integration methods. Optik 239, 166871 (2021)
E.M. Zayed, R.M. Shohib, M.E. Alngar, A. Biswas, S. Khan, Y. Yıldırım, H. Triki, A.K. Alzahrani, M.R. Belic, Cubic-quartic optical solitons with Kudryashov’s arbitrary form of nonlinear refractive index. Optik 238, 166747 (2021)
E. Zayed, R. Shohib, M. Alngar, A. Biswas, M. Ekici, S. Khan, A.K. Alzahrani, M. Belic, Optical solitons and conservation laws associated with Kudryashov’s sextic power-law nonlinearity of refractive index. Ukr. J. Phys. Opt. 22, 38–49 (2021)
E.M. Zayed, R.M. Shohib, M.E. Alngar, Y. Yıldırım, Optical solitons in fiber Bragg gratings with Radhakrishnan–Kundu–Lakshmanan equation using two integration schemes. Optik 245, 167635 (2021)
E.M. Zayed, R.M. Shohib, M.M. El-Horbaty, A. Biswas, M. Asma, M. Ekici, A.K. Alzahrani, M.R. Belic, Solitons in magneto-optic waveguides with quadratic-cubic nonlinearity. Phys. Lett. A 384(25), 126456 (2020)
S.A. AlQahtani, R.M. Shohib, M.E. Alngar, A.M. Alawwad, High-stochastic solitons for the eighth-order NLSE through Itô calculus and STD with higher polynomial nonlinearity and multiplicative white noise. Opt. Quant. Electron. 55(14), 1227 (2023)
S.A. AlQahtani, M.S. Al-Rakhami, R.M.A. Shohib, M.E.M. Alngar, P. Pathak, Dispersive optical solitons with Schrödinger-Hirota equation using the -model expansion approach. Opt. Quant. Electron. 55, 701 (2023)
A.R. Adem, T.J. Podile, B. Muatjetjeja, A generalized (3+ 1)-dimensional nonlinear wave equation in liquid with gas bubbles: symmetry reductions; exact solutions; conservation laws. Int. J. Appl. Comput. Math. 9(5), 82 (2023)
I. Humbu, B. Muatjetjeja, T.G. Motsumi, A.R. Adem, Solitary waves solutions and local conserved vectors for extended quantum Zakharov–Kuznetsov equation. Eur. Phys. J. Plus 138(9), 873 (2023)
M.C. Sebogodi, B. Muatjetjeja, A.R. Adem, Exact solutions and conservation laws of a (2+ 1)-dimensional combined potential kadomtsev–petviashvili-b-type kadomtsev–petviashvili equation. Int. J. Theor. Phys. 62(8), 165 (2023)
I. Humbu, B. Muatjetjeja, T.G. Motsumi, A.R. Adem, Periodic solutions and symmetry reductions of a generalized Chaffee–Infante equation. Partial Diff. Equ. Appl. Math. 7, 100497 (2023)
A.R. Adem, T.S. Moretlo, B. Muatjetjeja, A generalized dispersive water waves system: Conservation laws; symmetry reduction; travelling wave solutions; symbolic computation. Partial Diff. Equ. Appl. Math. 7, 100465 (2023)
A.R. Adem, B. Muatjetjeja, T.S. Moretlo, An extended (2+ 1)-dimensional coupled burgers system in fluid mechanics: symmetry reductions; Kudryashov method; conservation laws. Int. J. Theor. Phys. 62(2), 38 (2023)
A.R. Adem, B. Muatjetjeja, Conservation laws and exact solutions for a 2D Zakharov–Kuznetsov equation. Appl. Math. Lett. 48, 109–117 (2015)
A.R. Adem, The generalized (1+ 1)-dimensional and (2+ 1)-dimensional Ito equations: multiple exp-function algorithm and multiple wave solutions. Comput. Math. Appl. 71(6), 1248–1258 (2016)
A.R. Adem, X. Lü, Travelling wave solutions of a two-dimensional generalized Sawada–Kotera equation. Nonlinear Dyn. 84, 915–922 (2016)
A.R. Adem, Solitary and periodic wave solutions of the Majda–Biello system. Mod. Phys. Lett. B 30(15), 1650237 (2016)
Adem, A. R. A (2+ 1)-dimensional Korteweg-de Vries type equation in water waves: Lie symmetry analysis; multiple exp-function method; conservation laws. Int. J. Modern Phys. B 30, 1640001 (2016)
E.M. Zayed, M.E. Alngar, R.M. Shohib, A. Biswas, Y. Yıldırım, L. Moraru, S. Moldovanu, P.L. Georgescu, Dispersive optical solitons with differential group delay having multiplicative white noise by ito calculus. Electronics 12(3), 634 (2023)
A. Biswas, J. Vega-Guzman, A.H. Kara, S. Khan, H. Triki, O. González-Gaxiola, L. Moraru, P.L. Georgescu, Optical solitons and conservation laws for the concatenation model: undetermined coefficients and multipliers approach. Universe 9(1), 15 (2022)
A. H. Arnous, A. Biswas, A. H. Kara, Y. Yıldırım, L. Moraru, S. Moldovanu, P. L. Georgescu, A. A. Alghamdi, Dispersive optical solitons and conservation laws of Radhakrishnan–Kundu–Lakshmanan equation with dual-power law nonlinearity. Heliyon (2023) https://doi.org/10.1016/j.heliyon.2023.e14036
E.M. Zayed, M. El-Horbaty, M.E. Alngar, R.M. Shohib, A. Biswas, Y. Yıldırım, L. Moraru, C. Iticescu, D. Bibicu, P.L. Georgescu, A. Asiri, Dynamical system of optical soliton parameters by variational principle (super-Gaussian and super-sech pulses). J. Eur. Opt. Soc. 19(2), 38 (2023)
R.M.A. Shohib, M.E.M. Alngar, A. Biswas, Y. Yildirim, H. Triki, L. Moraru, C. Iticescu, P.L. Georgescu, A. Asiri, Optical solitons in magneto-optic waveguides for the concatenation model. Ukr. J. Phys. Opt. 24, 248–261 (2023)
A.H. Arnous, A. Biswas, Y. Yildirim, L. Moraru, C. Iticescu, P.L. Georgescu, A. Asiri, Optical solitons and complexitons for the concatenation model in birefringent fibers. Ukr. J. Phys. Opt. 24, 04060–04086 (2023)
E.M.E. Zayed, M.E.M. Alngar, R.M.A. Shohib, A. Biswas, Y. Yildirim, L. Moraru, P.L. Georgescu, C. Iticescu, Siri, A., Highly dispersive solitons in optical couplers with metamaterials having Kerr law of nonlinear refractive index. Ukr. J. Phys. Opt. 25, 1001–1019 (2024)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Arnous, A.H., Biswas, A., Yildirim, Y. et al. Optical solitons for the dispersive concatenation model with power law of self-phase modulation and multiplicative white noise. J Opt (2024). https://doi.org/10.1007/s12596-024-01670-y
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s12596-024-01670-y