Reducing temperature distribution in solid-state lasers utilizing annular beam profile: modeling and simulation

Abstract

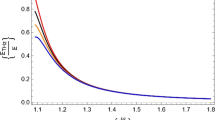
Based on Kirchhoff’s transformation a mathematical expression was derived to reduce the temperature distribution in a double-end-pumping configuration utilizing an annular intensity profile. The critical parameters affecting the laser rod temperature distribution, such as the cooling temperature, waist radii, and pump power were theoretically analyzed and simulated using the MATLAB software. The results revealed that with annular pumping; a reduction in temperature of approximately 28.58% was obtained compared to the top-hat intensity profile. In the present study, the derived expression offers an exact solution for thermal mitigation for different cavity configurations and laser parameters.

Appendix A: Calculations of temperature distribution utilizing annular-beam profile in double end-pumped lasers configuration

The steady-state heat conductance equation described by Eq. (1) can be simplified by using the assumptions of an axisymmetric pump profile and isotropic cooling in the z-plane and it could be written as:

$$\frac{1}{r}\frac{{\text{d}}}{{{\text{d}}r}}\left[ {r k\left( T \right)\frac{{{\text{d}}T\left( {r,\;z} \right)}}{{{\text{d}}r}}} \right] + Q\left( {r,\;z} \right) = 0$$

(15)

Applying Kirchhoff transformation in term of function U, Eq. (15) can be solved as follows:

$$U_{{\left( {{\text{r}},\;{\text{z}}} \right)}} = \smallint k\left( T \right){\text{d}}T$$

(16)

This leads to

$$k\left( T \right) = \frac{{{\text{d}}U}}{{{\text{d}}T}}$$

(17)

Substituting Eq. (17) into Eq. (16), and by applying differentiation chain rule, Eq. (16) transformed into the linear form:

$$\nabla^{2} U_{{\left( {{\text{r,}}\;{\text{z}}} \right)}} = \frac{1}{r}\frac{{{\text{d}}U_{{\left( {{\text{r,}}\;{\text{z}}} \right)}} }}{{{\text{d}}r}} + \frac{{{\text{d}}^{2} U_{{\left( {{\text{r,}}\;\;{\text{z}}} \right)}} }}{{{\text{d}}r^{2} }} = - Q_{{\left( {{\text{r,}}\;\;{\text{z}}} \right)}}$$

(18)

In case of annular-beam pumping temperature distribution can be classified to three regions, for the inner region, $0 \le r \le r_{1}$ Equation (18) can be written as

$$\frac{1}{r}\frac{{{\text{d}}U_{1} \left( {r,\;z} \right)}}{{{\text{d}}r}} + \frac{{{\text{d}}^{2} U_{1} \left( {r,\;z} \right)}}{{{\text{d}}r^{2} }} = 0$$

(19)

let $\frac{{{\text{d}}U_{1} \left( {r,\;z} \right)}}{{{\text{d}}r}} = w_{1} \left( r \right)$ and $\frac{{{\text{d}}^{2} U_{1} \left( {r,\;z} \right)}}{{{\text{d}}r^{2} }} = \frac{{{\text{d}}w_{1} \left( r \right)}}{{{\text{d}}r}}$. Multiplying by r and Substituting ${1} = \frac{{{\text{d}}r}}{{{\text{d}}r}}$, that gives

$$\frac{{{\text{d}}r}}{{{\text{d}}r}} w_{1} \left( r \right) + r\frac{{{\text{d}}w_{1} \left( r \right)}}{{{\text{d}}r}} = 0$$

(20)

Equation (20) can be written as

$$\frac{{\text{d}}}{{{\text{d}}r}}\left[ {r w_{1} \left( r \right)} \right] = 0$$

(21)

And by applying integration into Eq. (21), that gives

$$w_{1} \left( r \right) = \frac{{{\text{d}}U_{1} \left( {r,\;z} \right)}}{{{\text{d}}r}} = \frac{A}{r}$$

(22)

where A is an integration constant. By using another integration,

$$U_{1} \left( {r,\;z} \right) = A\;\ln \;\left( r \right) + B$$

(23)

Equations (2) and (16), gives

$$U = \frac{{k_{0} }}{{\left( {m + 1} \right) T_{0}^{m} }}T^{m + 1} + C$$

(24)

Then the temperature equation would be

$$T_{1} \left( {r,\;z} \right) = \left\{ {\left( {A\;\ln \;\left( r \right) + B} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{m + 1}}}$$

(25)

For the second region, $r_{1} < r \le r_{2}$,Eq. (18) becomes

$$\frac{1}{r}\frac{{{\text{d}}U_{2} \left( {r,\;z} \right)}}{{{\text{d}}r}} + \frac{{{\text{d}}^{2} U_{2} \left( {r,\;z} \right)}}{{{\text{d}}r^{2} }} = - Q_{L} e^{ - \alpha z} - Q_{R} e^{{ - \alpha \left( {l - z} \right)}}$$

(26)

In the same way, this equation was solved and the result was

$$U_{2} \left( {r,\;z} \right) = \frac{{ - Q_{L} e^{ - \alpha z} }}{4}r^{2} - \frac{{Q_{R} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r^{2} + D\;\ln \;\left( r \right) + E$$

(27)

And by using Eq. (24), temperature equation was obtained

$$T_{2} \left( {r,\;z} \right) = \left\{ {\left( {\frac{{ - Q_{{\text{L}}} e^{ - \alpha z} }}{4}r^{2} - \frac{{Q_{{\text{R}}} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r^{2} + D\;\ln \left( r \right) + E} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{{{\text{m}} + 1}}}}$$

(28)

For the last region,$r_{2} < r \le { }r_{0}$, Eq. (18) becomes

$$\frac{1}{r}\frac{{{\text{d}}U_{3} \left( {r,\;z} \right)}}{{{\text{d}}r}} + \frac{{{\text{d}}^{2} U_{3} \left( {r,\;z} \right)}}{{{\text{d}}r^{2} }} = 0$$

(29)

Using the same steps, this equation was solved to obtain

$$U_{3} \left( {r,\;z} \right) = G\ln \;\left( r \right) + H$$

(30)

Equation (24) utilized to obtain temperature equation

$$T_{3} = \left\{ {\left( {G\ln \left( r \right) + H} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{m + 1}}}$$

(31)

A, B, D, E, G, and H are integration constants, that can be found by using a boundary conditions.

For symmetry reasons, the heat flux in r direction must be zero at the center of the rod, which means:

$$- k\left( T \right)\left. {\frac{{{\text{d}}T_{1} }}{{{\text{d}}r}}} \right|_{r = 0} = 0$$

(32)

Substitute Eq. (17) into Eq. (32), the first boundary condition written as

$$- \left. {\frac{{{\text{d}}U_{1} }}{{{\text{d}}r}}} \right|_{r = 0} = 0$$

(33)

And by using Eq. (A-11), the first integration constant obtained

$$A = 0$$

(34)

On the other hand, the boundary condition at the rod surface $\left(r={r}_{0}\right)$ can be written as:

$$\left. { - \frac{{{\text{d}}U_{3} \left( {r,\;z} \right)}}{{{\text{d}}r}}} \right|_{{r = r_{0} }} = h\left[ {T_{3} \left( {r = r_{0} } \right) - T_{{\text{c}}} } \right]$$

(35)

Substituting Eqs. (30) and (31) into Eq. (35) that lead to

$$\frac{G}{{r_{0} h}} = T_{{\text{c}}} - \left\{ {\left( {G\ln \left( {r_{0} } \right) + H} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{m + 1}}}$$

(36)

The other boundary conditions at $r = r_{1}$ and $r = r_{2}$ where

$$T_{1} \left( {r = r_{1} } \right) = T_{2} \left( {r = r_{1} } \right)$$

(37)

That gives

$$\left\{ {\left( {A\;\ln \left( {r_{1} } \right) + B} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{m + 1}}} = \left\{ {\left( {\frac{{ - Q_{0,1} e^{ - \alpha z} }}{4}r_{1}^{2} - \frac{{Q_{0,2} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r_{1}^{2} + D\;\ln \;\left( {r_{1} } \right) + E} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{1}{m + 1}}}$$

(38)

And

$$\left. {\frac{{{\text{d}}T_{1} }}{{{\text{d}}r}}} \right|_{{r = r_{1} }} = \left. {\frac{{{\text{d}}T_{2} }}{{{\text{d}}r}}} \right|_{{r = r_{1} }}$$

(39)

Which gives

$$\begin{gathered} \frac{1}{m + 1}\left\{ {\left( {A\;\ln \;\left( {r_{1} } \right) + B} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{ - m}{{m + 1}}}} *\frac{A}{{r_{1} }}*\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }} \hfill \\ = \frac{1}{m + 1}\left\{ {\left( {\frac{{ - Q_{0,1} e^{ - \alpha z} }}{4}r_{1}^{2} - \frac{{Q_{0,2} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r_{1}^{2} + D\;\ln \;\left( {r_{1} } \right) + E} \right)\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }}} \right\}^{{\frac{ - m}{{m + 1}}}} \hfill \\ *\left( {\frac{{ - Q_{0,1} e^{ - \alpha z} }}{2}r_{1} - \frac{{Q_{0,2} e^{{ - \alpha \left( {l - z} \right)}} }}{2}r_{1} + \frac{D}{{r_{1} }}} \right)*\frac{{T_{0}^{m} \left( {m + 1} \right)}}{{k_{0} }} \hfill \\ \end{gathered}$$

(40)

And for the other condition, at $r = r_{2}$

$$T_{2} \left( {r = r_{2} } \right) = T_{3} \left( {r = r_{2} } \right)$$

(41)

$$\left. {\frac{{{\text{dT}}_{2} }}{{{\text{dr}}}}} \right|_{{{\text{r}} = {\text{r}}_{2} }} = \left. {\frac{{{\text{dT}}_{3} }}{{{\text{dr}}}}} \right|_{{{\text{r}} = {\text{r}}_{2} }}$$

(42)

That give:

$$\frac{{ - Q_{0,\;1} e^{ - \alpha z} }}{4}r_{2}^{2} - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r_{2}^{2} + D\;\ln \;\left( {r_{2} } \right) + E = G\ln \;\left( {r_{2} } \right) + H$$

(43)

$$\begin{gathered} \left( {\frac{{ - Q_{0,\;1} e^{ - \alpha z} }}{4}r_{2}^{2} - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {l - z} \right)}} }}{4}r_{2}^{2} + D\ln \left( {r_{2} } \right) + E} \right)^{{\frac{ - m}{{m + 1}}}} \left( {\frac{{ - Q_{0,\;1} e^{ - \alpha z} }}{2}r_{2} - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {l - z} \right)}} }}{2}r_{2} + \frac{D}{{r_{2} }}} \right) \hfill \\ = \frac{G}{{r_{2} }}\left( {G\ln \left( {r_{2} } \right) + H} \right)^{{\frac{ - m}{{m + 1}}}} \hfill \\ \end{gathered}$$

(44)

$B,D,E,G,\mathrm{and} H$ can be found by solving Eqs. (36), (38), (40), (43), and (44)

$$\begin{gathered} B = \frac{{Q_{0,\;1} e^{ - \alpha z} }}{4}\left( {r_{2}^{2} - r_{1}^{2} + r_{2}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{2}^{2} }}} \right) - r_{1}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{1}^{2} }}} \right)} \right) + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{4}\left( {r_{2}^{2} - r_{1}^{2} + r_{2}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{b}^{2} }}} \right) - r_{1}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{1}^{2} }}} \right)} \right) \hfill \\ + \frac{{k_{0} }}{{T_{0}^{m} \left( {m + 1} \right)}}\left[ {T_{{\text{c}}} + \frac{{Q_{0,\;1} e^{ - \alpha z} }}{{2r_{0} h}}\left( {r_{2}^{2} - r_{1}^{2} } \right) + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{{2r_{0} h}}\left( {r_{2}^{2} - r_{1}^{2} } \right)} \right]^{m + 1} \hfill \\ \end{gathered}$$

(45)

$$D = \frac{{Q_{0,\;1} e^{ - \alpha z} }}{2}r_{1}^{2} + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{2}r_{1}^{2}$$

(46)

$$\begin{gathered} E = \frac {{k}_{0}}{T_{0}^{m} (m + 1)} \left[ {T_{c} + \frac{{Q_{0,\;1} e^{ - \alpha z} }}{{2r_{0} h}}\left( {r_{2}^{2} - r_{1}^{2} } \right) + \frac{{Q_{0,2} e^{{ - \alpha \left( {L - z} \right)}} }}{{2r_{0} h}}\left( {r_{2}^{2} - r_{1}^{2} } \right)} \right]^{m + 1} + \;\frac{{Q_{0,\;1} e^{ - \alpha z} }}{4}\left( { - 2r_{2}^{2} \ln \left( {r_{2} } \right) + 2r_{2}^{2} \ln \left( {r_{0} } \right) - 2r_{1}^{2} \ln \left( {r_{0} } \right) + r_{2}^{2} } \right) \hfill \\ + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{{2r_{0} h}}\left( { - 2r_{2}^{2} \ln \left( {r_{2} } \right) + 2r_{2}^{2} \ln \left( {r_{0} } \right) - 2r_{1}^{2} \ln \left( {r_{0} } \right) + r_{1}^{2} } \right) \hfill \\ \end{gathered}$$

(47)

$$G = \frac{{ - Q_{0,\;1} e^{ - \alpha z} }}{2}r_{2}^{2} - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{2}r_{2}^{2} + \frac{{Q_{0,\;1} e^{ - \alpha z} }}{2}r_{1}^{2} + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{2}r_{1}^{2}$$

(48)

$$\begin{gathered} H = \frac{{k_{0} }}{{T_{0}^{m} \left( {m + 1} \right)}}\left[ {T_{{\text{c}}} + \frac{{Q_{0,\;1} e^{ - \alpha z} }}{{2r_{0} h}}r_{2}^{2} + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{{2r_{0} h}}r_{2}^{2} - \frac{{Q_{0,1} e^{ - \alpha z} }}{{2r_{0} h}}r_{1}^{2} - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{{2r_{0} h}}r_{1}^{2} } \right]^{m + 1} + \frac{{Q_{0} e^{ - \alpha z} }}{2} \hfill \\ r_{2}^{2} \ln \left( {r_{0} } \right) + \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{2}r_{2}^{2} \ln \left( {r_{0} } \right) - \frac{{Q_{0,\;1} e^{ - \alpha z} }}{2}r_{1}^{2} \ln \left( {r_{0} } \right) - \frac{{Q_{0,\;2} e^{{ - \alpha \left( {L - z} \right)}} }}{2}r_{1}^{2} \;{\text{In}}\;\;(r_{0} ) \hfill \\ \end{gathered}$$

(49)

Substitute Eqs. (45), (46), (47), (48), and (49) into the Eqs. (25), (28), and (31) to obtain

$$\begin{aligned} T_{1} \left( {r,\;z} \right) = & \left\{ {\frac{{\eta_{h} \alpha T_{0}^{m} \left( {m + 1} \right)}}{{4\pi k_{0} }}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)} \right.\left( {\frac{{r_{2}^{2} - r_{1}^{2} + r_{2}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{2}^{2} }}} \right) - r_{1}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{1}^{2} }}} \right)}}{{r_{2}^{2} - r_{1}^{2} }}} \right) \\ \,\left. { + \left[ {T_{{\text{c}}} + \frac{{\eta_{{\text{h}}} \alpha }}{{2\pi r_{0} h}}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)^{m + 1} } \right]} \right\}^{{\frac{1}{m + 1}}} \\ \end{aligned}$$

(50)

$$\begin{gathered} T_{2} \left( {r,z} \right) = \left\{ {\frac{{\eta_{h} \alpha T_{0}^{m} \left( {m + 1} \right)}}{{4\pi k_{0} }}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)\left( {\frac{{r_{2}^{2} - r^{2} + r_{2}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r_{2}^{2} }}} \right) - r_{1}^{2} \ln \left( {\frac{{r_{0}^{2} }}{{r^{2} }}} \right)}}{{\left( {r_{2}^{2} - r_{1}^{2} } \right)}}} \right)} \right. \hfill \\ \left. { + \left[ {T_{c} + \frac{{\eta_{h} \alpha }}{{2\pi r_{0} h}}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)} \right]^{m + 1} } \right\}^{{\frac{1}{m + 1}}} \hfill \\ \end{gathered}$$

(51)

$$\begin{aligned} T_{3} \left( {r,\;z} \right) & = \left\{ {\frac{{\eta_{{\text{h}}} \alpha T_{0}^{m} \left( {m + 1} \right)}}{{4\pi k_{0} }}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)\ln \;\left( {\frac{{r_{0}^{2} }}{{r^{2} }}} \right)} \right. \\ \left. { + \left[ {T_{{\text{c}}} + \frac{{\eta_{h} \alpha }}{{2\pi r_{0} h}}\left( {P_{{{\text{inL}}}} e^{ - \alpha z} + P_{{{\text{inR}}}} e^{{ - \alpha \left( {l - z} \right)}} } \right)} \right]^{m + 1} } \right\}^{{\frac{1}{m + 1}}} \\ \end{aligned}$$

(52)