Skip to main content
SpringerLink
Log in

Melnikov analysis of subharmonic motions for a class of bistable vibro-impact oscillators

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

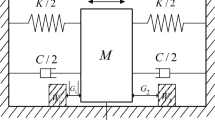
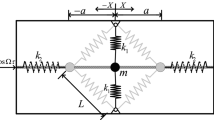
Abstract

In this paper, subharmonic motions of a bistable vibro-impact oscillator are studied by establishing the theoretical framework of the Melnikov analysis for an abstract non-smooth dynamical system. A ideal impacting map is employed to describe velocity changing during instantaneous collision with the bilateral rigid constraints. The unperturbed system without considering damping and external excitations is supposed to have a pair of homoclinic orbits connecting the origin to itself, and the inner and outer regions separated by the homoclinic orbits are assumed to be fully covered by periodic orbits, whose periods monotonically increase as they approach the homoclinic connections. Furthermore, periodic or homoclinic grazing of the unperturbed system can also occur by adjusting the position of the constraints. When a periodic perturbation is considered, the definitions of the Unilateral subharmonic orbits, the Bilateral subharmonic orbits and the Compound subharmonic orbits for this class of non-smooth systems are given by combining the impacting dynamics. The Melnikov functions for the first two types subharmonic orbits are also obtained and employed to detect the initial conditions for the existence of the corresponding subharmonic orbits. Finally, the bistable vibro-impact oscillator as an example is used to show the effectiveness of the developed Melnikov method for seeking subharmonic motions for this class of bistable vibro-impact oscillator. A numerical integration method is also introduced to overcome the difficulty of the Melnikov integration along the unperturbed periodic orbits.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

Data availability statement

The authors declare that all data generated or analyzed during this study are included in this published article.

References

  1. Brogliato, B.: Nonsmooth Mechanics. Springer, Berlin (1999)

    Book  MATH  Google Scholar 

  2. Feeny, B.F., Moon, F.C.: Empirical dry-friction modelling in a forced oscillator using chaos. Nonlinear Dyn. 47, 129–141 (2007). https://doi.org/10.1007/s11071-006-9065-5

    Article  MATH  Google Scholar 

  3. Banerjee, S., Verghese, G.: Nonlinear Phenomena in Power Electronics. IEEE Press, New York (2001)

    Book  Google Scholar 

  4. Garcia, Mariano: The simplest walking model: stability, complexity, and scaling. J. Biomech. Eng. 120(2), 281 (1998). https://doi.org/10.1115/1.2798313

    Article  Google Scholar 

  5. Filippov, A.F.: Differential Equations with Discontinuous Right-Hand Sides: Mathematics and Its Applications. Kluwer Academic, Dordrecht (1988)

    Book  Google Scholar 

  6. Aizerman, M.A., Pyatnitskii, E.S.: Foundation of a theory of discontinuous systems. Autom. Remote Control 35, 1066–1079 (1974)

    MathSciNet  MATH  Google Scholar 

  7. Feigin, M.I.: Forced Vibrations of Nonlinear Systems with Discontinuities. Nauka, Moscow (1994)

    Google Scholar 

  8. Kunze, M.: Non-Smooth Dynamical Systems. Springer, Berlin (2000)

    Book  MATH  Google Scholar 

  9. Di Bernardo, M., Budd, C.J., Champneys, A.R., Kowalczyk, P.: Piecewise-Smooth Dynamical Systems: Theory and Application. Springer, London (2008)

    MATH  Google Scholar 

  10. Melnikov, V.K.: On the stability of the center for time periodic perturbations. Moscow Math. Soc. 12, 1–57 (1963). http://mi.mathnet.ru/eng/mmo137

  11. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical System and Bifurcations of Vector Fields. Springer, New York (1983)

    Book  MATH  Google Scholar 

  12. Wiggins, S.: Global Bifurcations and Chaos-Analytical Methods. Springer, New York (1988)

    Book  MATH  Google Scholar 

  13. Kukučka, P.: Melnikov method for discontinuous planar systems. Nonlinear Anal. 66(12), 2698–2719 (2007). https://doi.org/10.1016/j.na.2006.04.001

    Article  MathSciNet  MATH  Google Scholar 

  14. Shi, L., Zou, Y., Tassilo, K.: Melnikov method and detection of chaos for non-smooth systems. Acta Math. Appl. Sin. Engl. Ser. 29, 881–896 (2013). https://doi.org/10.1007/s10255-013-0265-8

    Article  MathSciNet  MATH  Google Scholar 

  15. Battelli, F., Fečkan, M.: Homoclinic trajectories in discontinuous systems. J. Dyn. Differ. Equ. 20, 337–376 (2008). https://doi.org/10.1007/s10884-007-9087-9

    Article  MathSciNet  MATH  Google Scholar 

  16. Battelli, F., Fečkan, M.: Bifurcation and chaos near sliding homoclinics. J. Dyn. Differ. Equ. 248, 2227–2262 (2010). https://doi.org/10.1016/j.jde.2009.11.003

    Article  MathSciNet  MATH  Google Scholar 

  17. Battelli, F., Fečkan, M.: Nonsmooth homoclinic orbits, Melnikov functions and chaos in discontinuous systems. Physica D 241, 1962–1975 (2012). https://doi.org/10.1016/j.physd.2011.05.018

    Article  MathSciNet  Google Scholar 

  18. Li, S.B., Zhang, W., Hao, Y.X.: Melnikov-type method for a class of discontinuous planar systems and applications. Int. J. Bifurc. Chaos 24(2), 1450022 (2014). https://doi.org/10.1142/S0218127414500229

    Article  MathSciNet  MATH  Google Scholar 

  19. Li, S.B., Shen, C., Zhang, W., Hao, Y.X.: Homoclinic bifurcations and chaotic dynamics for a piecewise linear system under a periodic excitation and a viscous damping. Nonlinear Dyn. 79, 2395–2406 (2015). https://doi.org/10.1007/s1107-014-1820-4

    Article  MathSciNet  MATH  Google Scholar 

  20. Li, S.B., Shen, C., Zhang, W., Hao, Y.X.: The Melnikov method of heteroclinic orbits for a class of planar hybrid piecewise-smooth systems and application. Nonlinear Dyn. 85(2), 1091–1104 (2016). https://doi.org/10.1007/s11071-016-2746-9

    Article  MathSciNet  MATH  Google Scholar 

  21. Li, S.B., Gong, X.J., Zhang, W.: The Melnikov method for detecting chaotic dynamics in a planar hybrid piecewise-smooth system with a switching manifold. Nonlinear Dyn. 9, 939–953 (2017). https://doi.org/10.1007/s11071-017-3493-2

    Article  MathSciNet  MATH  Google Scholar 

  22. Awrejcewicz, J., Holicke, M.M.: Smooth and Nonsmooth High Dimensional Chaos and Melnikov-Type Method. World Scientific, Singapore (2007)

    Book  MATH  Google Scholar 

  23. Li, S.B., Zhang, W.: Melnikov Method and Its Applications of Global Dynamics for Plannar Non-Smooth Systems. Science Press, Beijing (2022)

    Google Scholar 

  24. Du, Z.D., Zhang, W.N.: Melnikov method for homoclinic bifurcation in nonlinear impact oscillators. Comput. Math. Appl. 50(3–4), 445–458 (2005). https://doi.org/10.1016/j.camwa.2005.03.007

    Article  MathSciNet  MATH  Google Scholar 

  25. Xu, W., Feng, J.Q., Rong, H.W.: Melnikov method for a general nonlinear vibro-impact oscillator. Nonlinear Anal. 71(1–2), 418–426 (2009). https://doi.org/10.1016/j.na.2008.10.120

    Article  MathSciNet  MATH  Google Scholar 

  26. Cao, Q.J., Wiercigroch, M., Pavlovskaia, E.E., Thompson, J.M.T., Grebogi, C.: Piecewise linear approach to an archetypal oscillator for smooth and discontinuous dynamics. Philos. Trans. R. Soc. 366, 635–652 (2008). https://doi.org/10.1098/rsta.2007.2115

    Article  MathSciNet  MATH  Google Scholar 

  27. Cao, Q., Wiercigroch, M., Pavlovskaia, E.E., Grebogi, C., Thompson, J.M.T.: Archetypal oscillator for smooth and discontinuous dynamics. Phys. Rev. E 74, 046218 (2006). https://doi.org/10.1103/PhysRevE.74.046218

    Article  MathSciNet  MATH  Google Scholar 

  28. Gao, J.M., Du, Z.D.: Homoclinic bifurcation in a quasiperiodically excited impact inverted pendulum. Nonlinear Dyn. 79(2), 1061–1074 (2015). https://doi.org/10.1007/s11071-014-1723-4

    Article  MathSciNet  MATH  Google Scholar 

  29. Tian, R.L., Zhou, Y.F., Zhang, B.L., Yang, X.W.: Chaotic threshold for a class of impulsive differential system. Nonlinear Dyn. 83, 2229–2240 (2016). https://doi.org/10.1007/s11071-015-2477-3

    Article  MathSciNet  MATH  Google Scholar 

  30. Li, S.B., Wu, H.L., Zhou, X.X., Wang, T.T., Zhang, W.: Theoretical and experimental studies of global dynamics for a class of bistable nonlinear impact oscillators with bilateral rigid constraints. Int. J. Non Linear Mech. 133, 103720 (2021). https://doi.org/10.1016/j.ijnonlinmec.2021.103720

    Article  Google Scholar 

  31. Zhou, B.L., Jin, Y.F.: Chaos research of coupled SD oscillator under Gaussian colored noise and harmonic excitation. J. Theor. Appl. Mech. 54(7), 2030–2040 (2022). https://doi.org/10.6052/0459-1879-22-123

    Article  Google Scholar 

  32. Georgiev, Z.D., Uzunov, I.M., Todorov, T.G.: Analysis and synthesis of oscillator systems described by a perturbed double-well Duffing equation. Nonlinear Dyn. 94, 57–85 (2018). https://doi.org/10.1007/s11071-018-4345-4

    Article  MATH  Google Scholar 

  33. Rounak, A., Gupta, S.: Bifurcations in a pre-stressed, harmonically excited, vibro-impact oscillator at subharmonic resonances. Int. J. Bifurc. Chaos 30(08), 2050111 (2020). https://doi.org/10.1142/S0218127420501114

    Article  MathSciNet  MATH  Google Scholar 

  34. Gao, M., Fan, J.J.: Analysis of dynamical behaviors of a 2-DOF friction oscillator with elastic impacts and negative feedbacks. Nonlinear Dyn. 102.1, 45–78 (2020). https://doi.org/10.1007/s11071-020-05904-z

    Article  Google Scholar 

  35. Bernardo, M.D., Budd, C.J., Champneys, A.R., Kowalczyk, P.: Piecewise-Smooth Dynamical Systems: Theory and Application. Springer, London (2008)

    MATH  Google Scholar 

  36. Li, S.B., Wang, T.T.: Global dynamics for a class of new bistable nonlinear oscillators with bilateral elastic collisions. Int. J. Dyn. Control (2020). https://doi.org/10.1007/s40435-020-00733-9

  37. Shen, J., Du, Z.D.: Double impact periodic orbits for an inverted pendulum. Int. J. Nonlinear Mech. 46(9), 1177–1190 (2011). https://doi.org/10.1016/j.ijnonlinmec.2011.05.010

    Article  Google Scholar 

  38. Du, Z., Li, Y.: Type I periodic motions for nonlinear impact oscillators. Nonlinear Anal. 67(5), 1344–1358 (2007). https://doi.org/10.1016/j.na.2006.07.021

    Article  MathSciNet  MATH  Google Scholar 

  39. Li, Y., Du, Z., Zhang, W.: Asymmetric type II periodic motions for nonlinear impact oscillators. Nonlinear Anal. 68(9), 2681–2696 (2008). https://doi.org/10.1016/j.na.2007.02.015

    Article  MathSciNet  MATH  Google Scholar 

  40. Granados, A., Hogan, S.J., Seara, T.M.: The Melnikov method and subharmonic orbits in a piecewise smooth system. SIAM J. Appl. Dyn. Syst. 11(3), 801–830 (2012). https://doi.org/10.1137/110850359

    Article  MathSciNet  MATH  Google Scholar 

  41. Li, S.B., Ma, W.S., Zhang, W., Hao, Y.X.: Melnikov method for a three-zonal planar hybrid piecewise-smooth system and application. Int. J. Bifurc. Chaos 26(1), 1650014 (2016). https://doi.org/10.1142/S0218127416500140

    Article  MathSciNet  MATH  Google Scholar 

  42. Li, S.B., Zhao, S.B.: The analytical method of studying subharmonic periodic orbits for planar piecewise-smooth systems with two switching manifolds. Int. J. Dyn. Control (2018). https://doi.org/10.1007/s40435-018-0433-z

  43. Shen, J., Li, Y.R., Du, Z.D.: Subharmonic and grazing bifurcations for a simple bilinear oscillator. Int. J. Nonlinear Mech. 60, 70–82 (2014). https://doi.org/10.1016/j.ijnonlinmec.2014.01.003

    Article  Google Scholar 

  44. Chow, S.N., Shaw, S.W.: Bifurcations of subharmonics. J. Differ. Equ. 65(3), 304–320 (1986). https://doi.org/10.1016/0022-0396(86)90022-7

  45. Granados, A., Hogan, S.J., Seara, T.M.: The scattering map in two coupled piecewise-smooth systems, with numerical application to rocking blocks. Phys. D. 269(7), 1–20 (2014). https://doi.org/10.1016/j.physd.2013.11.008

    Article  MathSciNet  MATH  Google Scholar 

  46. Zhou, B.L., Jin, Y.F., Xu, H.D.: Subharmonic resonance and chaos for a class of vibration isolation system with two pairs of oblique springs. Appl. Math. Model. 108, 427–444 (2022). https://doi.org/10.1016/j.apm.2022.03.021

    Article  MathSciNet  MATH  Google Scholar 

  47. Luo, A.C.: The mapping dynamics of periodic motions for a three-piecewise linear system under a periodic excitation. J. Sound Vib. 283(3–5), 723–748 (2005). https://doi.org/10.1016/j.jsv.2004.05.023

    Article  MathSciNet  MATH  Google Scholar 

  48. Li, S.B., Wu, H.L., Chen, J.: Global dynamics and performance of vibration reduction for a new vibro-impact bistable nonlinear energy sink. Int. J. Non Linear Mech. 139, 103891 (2022). https://doi.org/10.1016/j.ijnonlinmec.2022.103891

    Article  Google Scholar 

Download references

Acknowledgements

The authors gratefully acknowledge the support of the National Natural Science Foundation of China (NNSFC) through Grant Nos. 12172376 and 11672326, the Fundamental Research Funds for the Central Universities through Grant No. 3122022PT09.

Funding

This study was founded by the National Natural Science Foundation of China through Grant Nos. 12172376 and 11672326 and the Fundamental Research Funds for the Central Universities through Grant No. 3122022PT09.

Author information

Authors and Affiliations

  1. Research Institute of Science and Technology, Civil Aviation University of China, Tianjin, 300300, China

    Shuangbao Li

  2. College of Science, Civil Aviation University of China, Tianjin, 300300, China

    Ran Sun

Authors
  1. Shuangbao Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ran Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shuangbao Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, S., Sun, R. Melnikov analysis of subharmonic motions for a class of bistable vibro-impact oscillators. Nonlinear Dyn 111, 1047–1069 (2023). https://doi.org/10.1007/s11071-022-07902-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07902-9

Keywords

Search

Navigation