Skip to main content
SpringerLink
Log in

A natural absolute coordinate formulation for the kinematic and dynamic analysis of rigid multibody systems

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

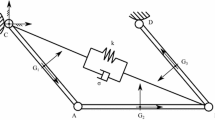
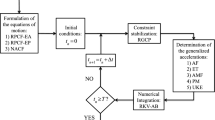
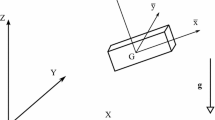
The purpose of this paper was to present the key features of a novel coordinate formulation for the analytical description of the motion of rigid multibody systems, namely the natural absolute coordinate formulation (NACF). As it is shown in this work, the kinematic and dynamic analysis of rigid multibody systems can be significantly enhanced employing the NACF. In particular, this formulation combines the main advantages of the natural coordinate formulation (NCF), such as the remarkable property of leading to a constant mass matrix and to zero centrifugal and Coriolis generalized inertia forces, with the generality and the effectiveness of the reference point coordinate formulation (RPCF), which is essentially represented by the possibility to develop and assemble the equations of motion of a multibody system together with the algebraic equations which model the joint constraints in a systematic manner. Moreover, a new computational method hereinafter referred to as the robust generalized coordinate partitioning algorithm is also introduced in this work. The robust generalized coordinate partitioning algorithm can be successfully utilized to numerically solve the index-one form of the multibody system equations of motion formulated by using the proposed NACF as well as the well-known RPCF. In particular, the computational procedure presented in this paper owes its robustness to the combination of the main ideas of the well-established generalized coordinate partitioning method, which is commonly employed to cope with the drift phenomenon of the constraint equations at the position and velocity levels when an index-one formulation of the equations of motion is considered, with the more general and advanced constraint enforcement technique at the acceleration level represented by the fundamental equations of constrained motion. In fact, the fundamental equations of constrained motion represent an effective and efficient method able to calculate analytically the generalized constraint forces relative to a multibody system subjected to a general set of redundant holonomic and/or nonholonomic constraint equations by using the Gauss principle of least constraint, thus avoiding the definition of the Lagrange multipliers. The fundamental equations of constrained motion are remarkably effective when used for modeling the dynamic behavior of rigid multibody systems mathematically represented employing the NACF, as it is shown in this paper. Four simple benchmark multibody systems are also examined in order to exemplify the application of the principal concepts developed in the paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Schiehlen, W.: Multibody Systems Handbook. Springer, Berlin (1990)

    Book  Google Scholar 

  2. Shabana, A.A.: Computational Continuum Mechanics, 2nd edn. Cambridge University Press, New York (2011)

    Book  MATH  Google Scholar 

  3. Angeles, J.: Fundamentals of Robotic Mechanical Systems: Theory, Methods, and Algorithms, 4th edn. Springer, New York (2013)

    Google Scholar 

  4. Roberson, R.E., Schwertassek, R.: Dynamics of Multibody Systems. Springer, Berlin (2012) (Reprint)

  5. Fuhrer, C., Eich-Soellner, E.: Numerical Methods in Multibody Dynamics. Springer Fachmedien Wiesbaden, Stuttgart (2013) (Reprint)

  6. Siciliano, B., Sciavicco, L., Villani, L., Oriolo, G.: Robotics: Modelling, Planning and Control, 2nd edn. Spinger, London (2010)

    Google Scholar 

  7. Cheli, F., Pennestri, E.: Cinematica e Dinamica dei Sistemi Multibody, vol. 1. Casa Editrice Ambrosiana, Milano (2006)

    Google Scholar 

  8. Cheli, F., Pennestri, E.: Cinematica e Dinamica dei Sistemi Multibody, vol. 2. Casa Editrice Ambrosiana, Milano (2006)

    Google Scholar 

  9. Jain, A.: Robot and Multibody Dynamics: Analysis and Algorithm. Springer, New York (2011) (Reprint)

  10. Amirouche, F.M.L.: Fundamentals of Multibody Dynamics. Theory And Applications. Birkhauser, Boston (2006)

    MATH  Google Scholar 

  11. Nikravesh, P.E.: Computer-Aided Analysis of Mechanical Systems. Prentice Hall, Englewood Cliffs (1988)

    Google Scholar 

  12. Bauchau, O.A.: Flexible Multibody Dynamics. Springer, New York (2011) (Reprint)

  13. Wittenburg, J.: Dynamics of Multibody Systems, 2nd edn. Springer, Berlin (2007)

    Google Scholar 

  14. Geradin, M., Cardona, A.: Flexible Multibody Dynamics: A Finite Element Approach. Wiley, Chichester (2001)

    Google Scholar 

  15. Hairer, E., Norsett, S.P., Wanner, G.: Solving Ordinary Differential Equations I: Nonstiff Problems, 3rd edn. Springer, Berlin (2009)

    Google Scholar 

  16. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II: Stiff and Differential-Algebraic Problems, 2nd edn. Springer, Berlin (2009)

    Google Scholar 

  17. Lanczos, C.: The Variational Principles of Mechanics, 4th edn. Dover, Toronto (1986) Please provide publisher name for references [17, 24, 39, 76, 79, 80]

  18. Von Schwerin, R.: MultiBody System Simulation: Numerical Methods, Algorithms, and Software. Springer, Berlin (1999) (Reprint)

  19. Garcia De Jalon, J., Bayo, E.: Kinematic and Dynamic Simulation of Multibody Systems: The Real-Time Challenge. Springer, New-York (2011) (Reprint)

  20. De Jalon, Garcia: J., Unda, J., Avello, A.: Natural coordinates for the computer analysis of multibody systems. J Comput. Methods Appl. Mech. Eng. 56(3), 309–327 (1986)

    Article  MATH  Google Scholar 

  21. De Jalon, Garcia: J., Unda, J., Avello, A., Jimenez, J.M.: Dynamic analysis of three-dimensional mechanisms in natural coordinates. J. Mech. Des. 109(4), 460–465 (1987)

    Google Scholar 

  22. Bayo, E.: A modified Lagrangian formulation for the dynamic analysis of constrained mechanical systems. J. Comput. Methods Appl. Mech. Eng. 71(2), 183–195 (1988)

    Article  MathSciNet  Google Scholar 

  23. De Jalon, Garcia: J.: Dynamic analysis of three-dimensional mechanisms in natural coordinates. J Multibody Syst. Dyn. 18(1), 15–33 (2007)

    Article  MATH  Google Scholar 

  24. Guida, D., Pappalardo, C.M.: Modelling rigid multibody systems using natural absolute coordinates. Accepted for publication, J. Mech. Eng. Ind. Des (2014)

    Google Scholar 

  25. De Jalon, Garcia: J., Serna, M.A.: Computer method for kinematic analysis of lower-pair mechanisms. Part I: velocities and accelerations. J Mech. Mach. Theory 16(5), 543–556 (1981)

    Article  MATH  Google Scholar 

  26. De Jalon, Garcia: J., Serna, M.A., Aviles, R.: Computer method for kinematic analysis of lower-pair mechanisms. Part II: position problems. J Mech. Mach. Theory 16(5), 557–566 (1981)

    Article  Google Scholar 

  27. Shabana, A.A., Yakoub, R.Y.: Three dimensional absolute nodal coordinate formulation for beam elements: theory. J. Mech. Des. 123(4), 606–613 (2000)

    Article  Google Scholar 

  28. Yakoub, R.Y., Shabana, A.A.: Three dimensional absolute nodal coordinate formulation for beam elements: implementation and applications. J. Mech. Des. 123(4), 614–621 (2000)

    Article  Google Scholar 

  29. Lan, P., Sahana, A.A.: Rational finite elements and flexible body dynamics. J. Vib. Acoust. 132(4), 1–9 (2010)

    Article  MATH  Google Scholar 

  30. Wehage, R.A., Haug, E.J.: Generalized coordinate partitioning for dimension reduction in analysis of constrained dynamic systems. J. Mech. Des. 104(1), 247–255 (1982)

    Article  Google Scholar 

  31. Kalaba, R.E., Udwadia, F.E.: Equations of motion for nonholonomic, constrained dynamical systems via Gausss principle. J. Appl. Mech. 60(3), 662–668 (1993)

    Article  MathSciNet  Google Scholar 

  32. Shabana, A.A.: Dynamics of Multibody Systems, 4th edn. Cambridge University Press, New York (2013)

    MATH  Google Scholar 

  33. Meirovitch, L.: Fundamentals of Vibrations. McGraw Hill, Boston (2010)

    Google Scholar 

  34. Goldstein, H., Poole, C.P., Safko, J.L.: Classical Mechanics, 3rd edn. Addison Wesley, San Francisco (2001)

    MATH  Google Scholar 

  35. Shabana, A.A.: Computational Dynamics, 3rd edn. Wiley, New York (2010)

    Book  MATH  Google Scholar 

  36. Golub, G.H., Van Loan, C.F.: Matrix Computations, 4th edn. Johns Hopkins University Press, Baltimore (2012)

    Google Scholar 

  37. Pfeiffer, F., Glocker, C.: Multibody Dynamics with Unilateral Contacts. Wiley, New York (1996)

    Book  MATH  Google Scholar 

  38. Haug, E.J.: Computer Aided Kinematics and Dynamics of Mechanical Systems. Volume I: Basic Methods. Allyn and Bacon, Needham (1989)

  39. Meirovitch, L.: Methods of Analytical Dynamics. Dover, New York (2010)

    Google Scholar 

  40. Shabana, A.A., Zaazaa, K.E., Sugiyama, H.: Railroad Vehicle Dynamics: A Computational Approach. CRC Press, Boca Raton (2007)

    Book  MATH  Google Scholar 

  41. Ardema, M.D.: Analytical Dynamics: Theory and Applications. Springer, New-York (2004)

    MATH  Google Scholar 

  42. Kane, T.R., Levinson, D.A.: Dynamics: Theory and Applications. McGraw-Hill, New York (1985)

    Google Scholar 

  43. Udwadia, F.E., Massa, G.D.: Sphere rolling on a moving surface: application of the fundamental equation of constrained motion. Simul. Model. Pract. Theory 19(4), 1118–1138 (2011)

    Article  Google Scholar 

  44. Flores, P., Ambrosio, J., Pimenta Claro, J.C., Lankarani, H.M.: Kinematics and Dynamics of Multibody Systems with Imperfect Joints: Models and Case Studies. Springer, Berlin (2008)

  45. Laulusa, A., Bauchau, O.A.: Review of classical approaches for constraint enforcement in multibody systems. J. Comput. Nonlinear Dyn. 3(1), 1–8 (2007)

    MATH  Google Scholar 

  46. Bauchau, O.A., Laulusa, A.: Review of contemporary approaches for constraint enforcement in multibody systems. J. Comput. Nonlinear Dyn. 3(1), 1–8 (2007)

    MATH  Google Scholar 

  47. Yakoub, R.Y., Shabana, A.A.: Use of Cholesky coordinates and the absolute nodal coordinate formulation in the computer simulation of flexible multibody systems. J. Nonlinear Dyn. 20(3), 267–282 (1999)

    Article  Google Scholar 

  48. Higham, N.J.: Accuracy and Stability of Numerical Algorithm. Siam, Philadelphia (2002)

    Book  Google Scholar 

  49. Sugiyama, H., Escalona, J.L., Shabana, A.A.: Formulation of three-dimensional joint constraints using the absolute nodal coordinates. J. Nonlinear Dyn. 31(2), 167–195 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  50. Guida, D., Pappalardo, C.M.: Forward and inverse dynamics of nonholonomic mechanical systems. Meccanica 49(7), 1547–1559 (2014)

    Article  MathSciNet  Google Scholar 

  51. Guida, D., Pappalardo, C.M.: Development of a closed-chain multibody model of a high-speed railway pantograph for hybrid motion/force control of the pantograph/catenary interaction. J. Mech. Eng. Ind. Des. 3(5), 45–85 (2013)

    Google Scholar 

  52. Negrut, D., Serban, R., Porta, F.A.: A topology-based approach to exploiting sparsity in multibody dynamics: joint formulation. J. Mech. Struct. Mach. 25(2), 221–241 (1997)

    Article  Google Scholar 

  53. Udwadia, F.E., Schutte, A.D.: Equations of motion for general constrained systems in Lagrangian mechanics. Acta Mech. 213(1–2), 111–129 (2010)

    Article  Google Scholar 

  54. Udwadia, F.E., Kalaba, R.E.: On the foundations of analytical dynamics. Int. J. Non Linear Mech. 37(6), 1079–1090 (2002)

    Article  MathSciNet  Google Scholar 

  55. Udwadia, F.E., Kalaba, R.E.: On motion. J. Franklin Inst. 330(3), 571–577 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  56. Udwadia, F.E., Wanichanon, T.: On general nonlinear constrained mechanical systems. Numer. Algebra Control Optim. 3(3), 425–443 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  57. De Falco, D., Pennestri, E., Vita, L.: Investigation of the influence of pseudoinverse matrix calculations on multibody dynamics simulations by means of The Udwadia-Kalaba formulation. J. Aerosp. Eng. 22(4), 365–372 (2009)

    Article  MATH  Google Scholar 

  58. Udwadia, F.E., Phohomsiri, P.: Explicit equations of motion for constrained mechanical systems with singular mass matrices and applications to multi-body dynamics. Proc. R. Soc. Lond. Ser. A 462, 2097–2117 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  59. Udwadia, F.E., Kalaba, R.E., Phohomsiri, P.: Mechanical systems with nonideal constraints: explicit equations without the use of generalized inverses. J. Appl. Mech. 71(5), 618–621 (2004)

    Article  MathSciNet  Google Scholar 

  60. Udwadia, F.E., Wanichanon, T.: On general nonlinear constrained mechanical systems. J. Numer. Algebra Control Optim. 3(3), 425–443 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  61. Hogben, L.: Handbook of Linear Algebra (Discrete Mathematics and Its Applications), 2nd edn. Chapman and Hall/CRC Press, Boca Raton (2013)

    Google Scholar 

  62. Schutte, A.D., Udwadia, F.E.: New approach to the modeling of complex multibody dynamical systems. J. Appl. Mech. 78(2), 1–11 (2010)

    Google Scholar 

  63. Udwadia, F.E., Kalaba, R.E.: Analytical Dynamics: A New Approach. Cambridge University Press, Cambridge (2007) (Reprint)

  64. Pappalardo, C.M.: Dynamics. Identification and Control of Multibody Systems. University of Salerno, Salerno (2012)

    Google Scholar 

  65. Deriglazov, A.: Classical Mechanics: Hamiltonian and Lagrangian Formalism. Springer, Berlin (2014) (Reprint)

  66. Blajer, W., Schiehlen, W., Schirm, W.: A projective criterion to the coordinate partitioning method for multibody dynamics. Arch. Appl. Mech. 64(2), 86–98 (1994)

    Google Scholar 

  67. Udwadia, F.E.: Equations of motion for constrained multibody systems and their control. J. Optim. Theory Appl. 127(3), 627–638 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  68. Ider, S.K., Amirouche, F.M.L.: Coordinate reduction in the dynamics of constrained multibody systems–a new approach. J. Appl. Mech. 55(4), 899–904 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  69. Serna, M.A., Aviles, R.: Garcia De Jalon, J.: Dynamic analysis of plane mechanisms with lower pairs in basic coordinates. J Mech. Mach. Theory 17(6), 397–403 (1982)

    Article  Google Scholar 

  70. Mani, N.K., Haug, E.J., Atkinson, K.E.: Application of singular value decomposition for analysis of mechanical system dynamics. J. Appl. Mech. 107(1), 82–87 (1985)

    Google Scholar 

  71. Hussein, B.A., Shabana, A.A.: Sparse matrix implicit numerical integration of the stiff differential/algebraic equations: implementation. J. Nonlinear Dyn. 65(4), 369–382 (2011)

    Article  MathSciNet  Google Scholar 

  72. Mariti, L., Belfiore, N., Pennestri, E., Valentini, P.: Comparison of solution strategies for multibody dynamics equations. Int. J. Numer. Meth. Eng. 88(7), 637–656 (2011)

    Article  MathSciNet  Google Scholar 

  73. De Jalon, Garcia: J., Gutierrez-Lopez, M.D.: Multibody dynamics with redundant constraints and singular mass matrix: existence, uniqueness, and determination of solutions for accelerations and constraint forces. J Multibody Syst. Dyn. 30(3), 311–341 (2013)

    Article  MATH  Google Scholar 

  74. Wojtyra, M.: Joint reaction forces in multibody systems with redundant constraints. J. Multibody Syst. Dyn. 14(1), 23–46 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  75. Guida, D., Pappalardo, C.M.: Swing-up and stabilization of an inverted pendulum with dry friction. J. Mech. Eng. Ind. Des. 2(5), 40–56 (2013)

    Google Scholar 

  76. Pappalardo, C.M., Patel, M.D., Tinsley, B., Shabana, A.A.: Contact force control in multibody pantograph/catenary systems. ASME J. Comput. Nonlinear Dyn, Submitted (2015)

    Google Scholar 

  77. Guida, D., Pappalardo, C.M.: Sommerfeld and mass parameter identification of lubricated journal bearing. WSEAS Trans. Appl. Theor. Mech. 4(4), 205–214 (2009)

    MATH  Google Scholar 

  78. Nilvetti, F., Pappalardo, C.M., Guida, D.: Mass, stiffness and damping identification of a two-story building model. J. Mech. Eng. Ind. Des. 1(2), 19–35 (2012)

    MATH  Google Scholar 

  79. Guida, D., Pappalardo, C.M.: Feedforward control design by adjoint-based control optimization method for hybrid motion-force control of nonlinear mechanical systems. Accepted for publication, J. Mech. Eng. Ind. Des (2014)

  80. Guida, D., Pappalardo, C.M.: Feedback control design by adjoint-based parameter optimization method for hybrid motion-force control of nonlinear mechanical systems. Accepted for publication, J. Mech. Eng. Ind. Des (2014)

    Google Scholar 

  81. Kraus, C., Winckler, M., Bock, H.: Modeling mechanical DAE using natural coordinates. Math. Comput. Model. Dyn. Syst. 7(2), 145–158 (2001)

    Article  Google Scholar 

  82. Shabana, A.A.: ANCF reference node for multibody system analysis. Proc. Inst. Mech. Eng. Part K J. Multibody Dyn. 229(1), 109–112 (2015)

Download references

Author information

Authors and Affiliations

  1. Department of Industrial Engineering, University of Salerno, Via Giovanni Paolo II, 132, 84084, Fisciano, Salerno, Italy

    Carmine M. Pappalardo

Authors
  1. Carmine M. Pappalardo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Carmine M. Pappalardo.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Pappalardo, C.M. A natural absolute coordinate formulation for the kinematic and dynamic analysis of rigid multibody systems. Nonlinear Dyn 81, 1841–1869 (2015). https://doi.org/10.1007/s11071-015-2111-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-015-2111-4

Keywords

Search

Navigation