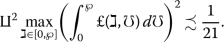A tripled fixed point technique for solving a tripled-system of integral equations and Markov process in CCbMS

Abstract

We prove the existence of tripled fixed points (TFPs) of a new generalized nonlinear contraction mapping in complete cone b-metric spaces (CCbMSs). Also, we present some exciting consequences as corollaries and three nontrivial examples. Finally, we find a solution for a tripled-system of integral equations (TSIE) and discussed a unique stationary distribution for the Markov process (SDMP).

1 Introduction and elementary discussion

Not knowing mathematics in a mathematically-driven world is like walking around a museum without looking at its walls. Learning and appreciating mathematics can help you appreciate certain things you would not otherwise focus on in your surrounding world. Mathematics is everywhere in nature. A typical example is the celebrated Fibonacci sequence of numbers, which is present in reproduction of species in nature. Mathematics is also useful to formulate epidemic models via differential or difference systems of equations that describe the couplings of the dynamics among subpopulations like susceptible, exposed, infectious, or recovered with immunity.

One of important branches of mathematics is functional analysis. The development of this field progressed in parallel to the development of modern theoretical physics. The formal framework of functional analysis adjusts closely the laws of both quantum mechanics and quantum field theory. At the same time, these physics theoretical frameworks have very relevant influence and links substantiating the body of problems and solution methodology of functional analysis.

A drop was taken from this branch and was called fixed point theory. Fixed-point technologies offer a focal concept with many diverse utilizations. It has been and still is an important theoretical tool in many fields and various disciplines such as topology, game theory, optimal control, artificial intelligence, logic programming, dynamical systems (and chaos), functional analysis, differential equations, and economics. For example, the fixed-point technique is applied to finding the solution of the equilibrium troubles in economics and game theory. In nonlinear integral equations, it used to find analytical and numerical solutions to Fredholm integral equations [1–5], and so on.

The idea of a coupled fixed point was initiated in [6]. Under this idea, some main results in partially ordered metric spaces were obtained by the same authors. For relevant properties in coupled fixed point consequences and related topics in abstract spaces, the reader can shed light from [7–21].

Pivotal results related to a TFP (established in 2011 by Berinde and Borcut [22]) were presented in partially ordered metric spaces. For more topics of this notion, we refer to [23–30].

As a generalization of ordinary metric space (OMC), a b-metric space (bMS) was presented by Bakhtin [31]. Under certain contraction conditions, he showed some important sequences in b-metric spaces. After his paper, a lot of authors discussed fixed point theory or various principles for operators (single- or multivalued) in the mentioned space as an extension of Banach’s principle in OMC; see [32–34].

Another extension of OMC, a cone metric space (CMS), was introduced by Huang and Zhang [35]. They discussed some fixed point theorems, which expanded certain results of this type to CMS.

Great papers incorporate bMS with CMS clarified by Hussain and Shah [36] under the name a cone b-metric space (CbMS), where some topological properties in such spaces and recent results about KKM mappings in a CbMS were established.

As an extension of this work, this paper was written to give some new TFPs under generalized nonlinear contraction mapping in CCbMS, We also give some important examples and corollaries to corroborate our theoretical results. Finally, we discuss contributions of TFPs to finding a solution of TSIE and a unique SDMP.

Now we present important notions of CbMS used in our paper.

Definition 1.1

([35])

Let B be a real Banach space, and let Q be a subset B. By ϑ we denote the zero element of B. The subset Q is called a cone if the following stipulations are fulfilled:

• (i) $Q\neq \varnothing$ is closed, and $Q\neq \{\vartheta \}$;

• (ii) $\omega ,\hslash \in \mathbb{R}$, $\omega ,\hslash \geq 0$, and $p,q\in Q\Rightarrow \omega p+\hslash q\in Q$;

• (iii) $Q\cap (-Q)=\{\vartheta \}$.

For a given cone $Q\subset B$, we define a partial ordering ≾ with respect to Q by $p\precsim q$ iff $q-p\in Q$. We write $p\prec q$ to indicate that $p\precsim q$ but $p\neq q$, whereas $p\ll q$ stands for $p-q\in Q^{\circ }$, where $Q^{\circ }$ is the interior of Q.

Let $\Vert \cdot \Vert$ be a norm on B. The cone Q is called normal if there is a number $\Pi >0$ such that for all $p,q\in B$, $\vartheta \leq p\leq q\Rightarrow \Vert p \Vert \leq \Pi \Vert q \Vert$. The least positive number satisfying this inequality is called the normal constant of Q.

Definition 1.2

([35])

Let $\chi \neq \varnothing$, and let $\xi :\chi \times \chi \rightarrow B$ be a mapping such that for all $p,q,r\in \chi$,

$(c_{1})$:

$\vartheta \leq \xi (p,q)$, and $\xi (p,q)=\vartheta \Leftrightarrow p=q$;

$(c_{2})$:

$\xi (p,q)=\xi (q,p)$;

$(c_{3})$:

$\xi (p,q)\leq \xi (p,r)+\xi (r,q)$.

The pair $(\chi ,\xi )$ is called a CMS.

Definition 1.3

([36])

Let $\chi \neq \varnothing$, and let $s\geq 1$. A mapping $\xi :\chi \times \chi \rightarrow B$ is called a CbM for all $p,q,r\in \chi$ iff the following conditions are fulfilled:

$(cb_{1})$:

$\vartheta \leq \xi (p,q)$, and $\xi (p,q)=\vartheta \Leftrightarrow p=q$;

$(cb_{2})$:

$\xi (p,q)=\xi (q,p)$;

$(cb_{3})$:

$\xi (p,q)\leq s ( \xi (p,r)+\xi (r,q) )$.

The pair $(\chi ,\xi )$ is called a CbMS.

From these two definitions we have that any CMS is a CbMS. So the class CbMSs is larger than the class of CMSs. Here we can confirm that CbMS generalizes bMS and CMS.

We strongly refer to paper [37], which gives some important examples that show that a CbMS $(\chi ,\xi )$ is not necessarily a CMS.

Definition 1.4

([36])

Let $(\chi ,\xi )$ be a CbMS, and let $\{p_{n}\}$ be a sequence in χ. Then for all $p\in \chi$,

• (1) $\{p_{n}\}$ converges to p if for every ε in B with $\vartheta \ll \varepsilon$, there is $\mathbb{N}$ such that for all $n>\mathbb{N}$, $\xi (p_{n},p)\ll \varepsilon$, and we write $\lim_{n\rightarrow \infty }p_{n}=p$ or $p_{n}\rightarrow p$ as $n\rightarrow \infty$;

• (2) $\{p_{n}\}$ is a Cauchy sequence if for every ε in B with $\vartheta \ll \varepsilon$, there is $\mathbb{N}$ such that $\xi (p_{n},p_{m})\ll \varepsilon$ for all $n,m>\mathbb{N}$;

• (3) the pair $(\chi ,\xi )$ is a CCbMS if every Cauchy sequence is convergent.

The following lemma is very important, especially when dealing with CMS.

Lemma 1.5

([38])

If $\hbar \in \operatorname{int}(Y)$ and $\vartheta \precsim p_{n} \overset{n\rightarrow \infty }{\rightarrow }\vartheta$, where Y is a cone, and $\{p_{n}\}$ is a sequence in B, then for an arbitrary fixed N and all $n\in N$, $p_{n}\ll \hbar$.

Lemma 1.6

([38])

If $p\precsim q$ and $q\ll r$ for all $p,q,r\in B$, then $p\ll r$.

Lemma 1.7

([39])

Let Q be a cone. If $\nu \in Q$ and $\nu \leq \tau \nu$ for some $\tau \in {}[ 0,1)$, then $\nu =\vartheta$.

Definition 1.8

([40])

A triple $(\wp ,\hbar ,\eth )\in \chi ^{3}$ is a TFP of a self-mapping $\Re :\chi ^{3}\rightarrow \chi$ if $\wp =\Re (\wp ,\hbar ,\eth )$, $\hbar =\Re (\hbar ,\wp ,\hbar )$, and $\eth =\Re (\eth ,\hbar ,\wp )$.

Definition 1.9

([41])

Let $(\chi ,\xi )$ be a CMS, Q be a solid cone and $\Im :\chi \rightarrow \chi$. Then

• (1) ℑ is said to be continuous if $\lim_{n\rightarrow \infty }p_{n}=p$ implies that $\lim_{n\rightarrow \infty }fx_{n}=fx$ for all $\{x_{n}\}$ in X;

• (2) ℑ is said to be sequentially convergent if for every sequence $\{x_{n}\}$ such that $\{\Im x_{n}\}$ is convergent, $\{x_{n}\}$ is also convergent.

• (3) ℑ is said to be subsequentially convergent if for every sequence $\{x_{n}\}$ such that $\{\Im x_{n}\}$ is convergent, $\{x_{n}\}$ has a convergent subsequence.

2 Main results

We begin this section with a new definition and an example that supports it.

Definition 2.1

Let $(\chi ,\xi )$ be a CbMS with the coefficient $s\geq 1$, let $Q\cup (-Q)=B$ (i.e., Q is a total ordering cone), and let $\Im :\chi \rightarrow \chi$. We will say that a mapping $\Re :\chi ^{3}\rightarrow \chi$ is a $\Im _{\theta }$-contraction if there is $\theta \in {}[ 0,1)$ such that

$$ \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) ,\xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r, \Im r^{\ast } \bigr) \bigr\} $$

(1)

for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$.

Example 2.2

Let $B=\mathbb{R} ^{2}$ and $Q=\{(p,q)\in B: p,q\geq 0\}\subset B$. Define the distance $\xi :\chi ^{2}\rightarrow B$ by $\xi (a,b)= ( \vert a-b \vert ^{i},\gamma \vert a-b \vert ^{i} )$, where $\gamma \geq 0$ and $i>1$ are constants. Define the mappings $\Im :\chi \rightarrow \chi$ and $\Re :\chi ^{3}\rightarrow \chi$ by

$$ \Im (p)=\frac{1}{2}p\quad \text{and}\quad \Re (p,q,r)= \textstyle\begin{cases} \frac{p-q-r}{4} & \text{if }p>q+r, \\ 0 & \text{otherwise}.\end{cases}$$

It is clear that the pair $(B,\xi )$ is a CbMS. If $p\leq q+r$ for all $p,q,r\in \chi$, then ℜ is an $\Im _{\theta }$-contraction.

On the other hand, take $p>q+r$, $q>q^{\ast }$, $r>r^{\ast }$, and $\theta =\frac{1}{4^{i}}<1$. Then for all $p,q,r\in \chi$, we get

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad = \bigl( \bigl\vert \Im \Re (p,q,r)-\Re \Im \bigl(p^{\ast },q^{\ast },r^{ \ast } \bigr) \bigr\vert ^{i},\gamma \bigl\vert \Im \Re (p,q,r)-\Im \Re \bigl(p^{ \ast },q^{\ast },r^{\ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad \precsim \biggl( \biggl\vert \frac{p-q-r}{8}- \frac{p^{\ast }-q^{\ast }-r^{\ast }}{8} \biggr\vert ^{i},\gamma \biggl\vert \frac{p-q-r}{8}- \frac{p^{\ast }-q^{\ast }-r^{\ast }}{8} \biggr\vert ^{i} \biggr) \\& \quad = \frac{1}{8^{i}} \bigl( \bigl\vert \bigl( p-p^{\ast } \bigr) - \bigl( q-q^{\ast } \bigr) - \bigl(r-r^{\ast } \bigr) \bigr\vert ^{i},\gamma \bigl\vert \bigl( p-p^{\ast } \bigr) - \bigl( q-q^{\ast } \bigr) - \bigl(r-r^{ \ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad \precsim \frac{1}{8^{i}} \bigl( \bigl\vert \bigl( p-p^{\ast } \bigr) \bigr\vert ^{i},\gamma \bigl\vert \bigl( p-p^{\ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad = \frac{3}{4^{i}} \biggl( \biggl\vert \frac{ ( p-p^{\ast } ) }{2} \biggr\vert ^{i},\gamma \biggl\vert \frac{ ( p-p^{\ast } ) }{2} \biggr\vert ^{i} \biggr) \\& \quad = \frac{1}{4^{i}}\xi \bigl( \Im p,\Im p^{\ast } \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) , \xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r, \Im r^{\ast } \bigr) \bigr\} . \end{aligned}$$

Hence the mapping ℜ is an $\Im _{\theta }$-contraction, and $(0,0,0)$ is a TFP of ℜ.

The following theorem is the first main result of this paper.

Theorem 2.3

Let the mapping ℜ be an $\Im _{\theta }$-contraction (1) defined on CCbMS $(\chi ,\xi )$ with the coefficient $s\geq 1$ such that B is a solid cone with $Q\cup (-Q)=B$ and $\Im :\chi \rightarrow \chi$ is a continuous and one-to-one. Then for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$,

• (a) there exist $\upsilon _{p_{\circ }},\upsilon _{q_{\circ }},\upsilon _{r_{\circ }} \in \chi$ and iterative sequences $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })=p_{n}$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })=q_{n}$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })=r_{n}$ such that

  $$\begin{aligned}& \lim_{n\rightarrow \infty }\Im \Re ^{n}(p_{\circ },q_{\circ },r_{ \circ })= \upsilon _{p_{\circ }}, \qquad \lim_{n\rightarrow \infty } \Im \Re ^{n}(q_{\circ },p_{\circ },q_{\circ })=\upsilon _{q_{\circ }}, \quad \textit{and} \\& \lim_{n\rightarrow \infty }\Im \Re ^{n}(r_{\circ },q_{\circ },p_{ \circ })= \upsilon _{r_{\circ }}; \end{aligned}$$

• (b) $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })$ have a convergent subsequence whenever ℑ is subsequentially convergent;

• (c) there are unique $\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }}\in \chi$ such that $\Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }})= \sigma _{p_{\circ }}$, $\Re (\sigma _{q_{\circ }},\sigma _{p_{\circ }},\sigma _{q_{\circ }})= \sigma _{q_{\circ }}$, and $\Re (\sigma _{r_{\circ }},\sigma _{q_{\circ }},\sigma _{p_{\circ }})= \sigma _{r_{\circ }}$;

• (d) for all $p_{\circ },q_{\circ },r_{\circ }\in \chi$, the sequences $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })$ converge to $\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }}\in \chi$, respectively, provided that ℑ is subsequentially convergent.

Proof

For arbitrary $p_{\circ },q_{\circ },r_{\circ }\in \chi$, we define three sequences as follows:

$$ \textstyle\begin{cases} p_{n+1}=\Re ( p_{n},q_{n},r_{n} ) =\Re ^{n+1}(p_{\circ },q_{ \circ },r_{\circ }), \\ q_{n+1}=\Re ( q_{n},p_{n},q_{n} ) =\Re ^{n+1} ( q_{ \circ },p_{\circ },q_{\circ } ) , \\ r_{n+1}=\Re ( r_{n},q_{n},p_{n} ) =\Re ^{n+1} ( r_{ \circ },q_{\circ },p_{\circ } ) ,\end{cases}\displaystyle \quad \text{for all }n\geq 0. $$

Applying condition (1), we get

$$\begin{aligned}& \xi ( \Im p_{n},\Im p_{n+1} ) \\& \quad = \xi \bigl( \Im \Re ( p_{n-1},q_{n-1},r_{n-1} ) ,\Im \Re ( p_{n},q_{n},r_{n} ) \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im p_{n-1},\Im p_{n} ) ,\xi ( \Im q_{n-1},\Im q_{n} ) ,\xi ( \Im r_{n-1}, \Im r_{n} ) \bigr\} , \end{aligned}$$

(2)

$$\begin{aligned}& \xi ( \Im q_{n},\Im q_{n+1} ) \\& \quad = \xi \bigl( \Im \Re ( q_{n-1},p_{n-1},q_{n-1} ) ,\Im \Re ( q_{n},p_{n},q_{n} ) \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im q_{n-1},\Im q_{n} ) ,\xi ( \Im p_{n-1},\Im p_{n} ) \bigr\} \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im q_{n-1},\Im q_{n} ) ,\xi ( \Im p_{n-1},\Im p_{n} ) ,\xi ( \Im r_{n-1}, \Im r_{n} ) \bigr\} , \end{aligned}$$

(3)

and

$$\begin{aligned}& \xi ( \Im r_{n},\Im r_{n+1} ) \\& \quad = \xi \bigl( \Im \Re ( r_{n-1},q_{n-1},p_{n-1} ) ,\Im \Re ( r_{n},q_{n},p_{n} ) \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im r_{n-1},\Im r_{n} ) ,\xi ( \Im q_{n-1},\Im q_{n} ) ,\xi ( \Im p_{n-1}, \Im p_{n} ) \bigr\} . \end{aligned}$$

(4)

Set $\eta _{n}=\max \{ \xi ( \Im p_{n},\Im p_{n+1} ) , \xi ( \Im q_{n},\Im q_{n+1} ) ,\xi ( \Im r_{n},\Im r_{n+1} ) \}$. By (2)–(4) we have

$$ \eta _{n}\precsim \theta \max \bigl\{ \xi ( \Im p_{n-1}, \Im p_{n} ) ,\xi ( \Im q_{n-1},\Im q_{n} ) ,\xi ( \Im r_{n-1}, \Im r_{n} ) \bigr\} =\theta \eta _{n-1}w, $$

where $\theta \in {}[ 0,1)$. Continuing this technique, we can write

$$ \vartheta \leq \eta _{n}\precsim \theta \eta _{n-1} \precsim \cdots \precsim \theta ^{n}\eta _{\circ }. $$

If we appoint $\eta _{\circ }=\vartheta$, then the triple $(p_{\circ },q_{\circ },r_{\circ })$ is a tripled fixed point of ℜ. Assuming that $\eta _{\circ }>\vartheta$, for all $m\geq 1$ and $i\geq 1$, we get

$$\begin{aligned}& \xi ( \Im p_{m+i},\Im p_{m} ) \\& \quad \precsim s \bigl[ \xi ( \Im p_{m+i},\Im p_{m+i-1} ) + \xi ( \Im p_{m+i-1},\Im p_{m} ) \bigr] \\& \quad = s\xi ( \Im p_{m+i},\Im p_{m+i-1} ) +s\xi ( \Im p_{m+i-1}, \Im p_{m} ) \\& \quad \precsim s\xi ( \Im p_{m+i},\Im p_{m+i-1} ) +s^{2} \bigl[ \xi ( \Im p_{m+i-1},\Im p_{m+i-2} ) + \xi ( \Im p_{m+i-2},\Im p_{m} ) \bigr] \\& \quad = s\xi ( \Im p_{m+i},\Im p_{m+i-1} ) +s^{2}\xi ( \Im p_{m+i-1},\Im p_{m+i-2} ) +s^{2}\xi ( \Im p_{m+i-2}, \Im p_{m} ) \\& \quad \precsim s\xi ( \Im p_{m+i},\Im p_{m+i-1} ) +s^{2}\xi ( \Im p_{m+i-1},\Im p_{m+i-2} ) +s^{3}\xi ( \Im p_{m+i-2}, \Im p_{m+i-3} ) +\cdots \\& \qquad {} +s^{i-1}\xi ( \Im p_{m+2},\Im p_{m+1} ) +s^{i-1}\xi ( \Im p_{m+1},\Im p_{m} ) . \end{aligned}$$

(5)

In a similar way, we get

$$\begin{aligned} \xi ( \Im q_{m+i},\Im q_{m} ) \precsim& s\xi ( \Im q_{m+i},\Im q_{m+i-1} ) +s^{2}\xi ( \Im q_{m+i-1}, \Im q_{m+i-2} ) \\ &{}+s^{3}\xi ( \Im q_{m+i-2},\Im q_{m+i-3} ) + \cdots+s^{i-1} \xi ( \Im q_{m+2},\Im q_{m+1} ) \\ &{}+s^{i-1}\xi ( \Im q_{m+1},\Im q_{m} ) \end{aligned}$$

(6)

and

$$\begin{aligned} \xi ( \Im r_{m+i},\Im r_{m} ) \precsim &s\xi ( \Im r_{m+i},\Im r_{m+i-1} ) +s^{2}\xi ( \Im r_{m+i-1}, \Im r_{m+i-2} ) \\ &{}+s^{3}\xi ( \Im r_{m+i-2},\Im r_{m+i-3} ) + \cdots \\ &{}+s^{i-1}\xi ( \Im r_{m+2},\Im r_{m+1} ) +s^{i-1}\xi ( \Im r_{m+1},\Im r_{m} ) . \end{aligned}$$

(7)

It follows from (5)–(7) that

$$\begin{aligned}& \max \bigl\{ \xi ( \Im p_{m+i},\Im p_{m} ) ,\xi ( \Im q_{m+i},\Im q_{m} ) ,\xi ( \Im r_{m+i},\Im r_{m} ) \bigr\} \\& \quad \precsim s\max \bigl\{ \xi ( \Im p_{m+i},\Im p_{m+i-1} ) , \xi ( \Im q_{m+i},\Im q_{m+i-1} ) ,\xi ( \Im r_{m+i},\Im r_{m+i-1} ) \bigr\} \\& \qquad {} +s^{2}\max \bigl\{ \xi ( \Im p_{m+i-1},\Im p_{m+i-2} ) , \xi ( \Im q_{m+i-1},\Im q_{m+i-2} ) ,\xi ( \Im r_{m+i-1}, \Im r_{m+i-2} ) \bigr\} +\cdots \\& \qquad {} +s^{i-1}\max \bigl\{ \xi ( \Im p_{m+2},\Im p_{m+1} ) , \xi ( \Im q_{m+2},\Im q_{m+1} ) ,\xi ( \Im r_{m+2}, \Im r_{m+1} ) \bigr\} \\& \qquad {} +s^{i-1}\max \bigl\{ \xi ( \Im p_{m+1},\Im p_{m} ) , \xi ( \Im q_{m+1},\Im q_{m} ) ,\xi ( \Im r_{m+1}, \Im r_{m} ) \bigr\} \\& \quad = s\eta _{m+i-1}+s^{2}\eta _{m+i-2}+s^{3} \eta _{m+i-3}+...s^{i-1} \eta _{m+1}+s^{i-1} \eta _{m} \\& \quad \precsim \bigl( s\theta ^{m+i-1}+s^{2}\theta ^{m+i-2}+ s^{3} \theta ^{m+i-3}..s^{i-1} \theta ^{m+1} \bigr) \eta _{\circ }+s^{i-1} \theta ^{m}\eta _{\circ } \\& \quad = \frac{s\theta ^{m+i} [ ( s\theta ^{-1} ) ^{i-1}-1 ] }{s-\theta }\eta _{\circ }+s^{i-1}\theta ^{m}\eta _{\circ } \\& \quad \precsim \frac{s^{i}\theta ^{m+i}}{s-\theta }\eta _{\circ }+s^{i-1} \theta ^{m}\eta _{\circ }. \end{aligned}$$

Suppose that $\vartheta \ll \hbar$ is given. Observe that $\frac{s^{i}\theta ^{m+1}}{s-\theta }\eta _{\circ }+s^{i-1}\theta ^{m}\eta _{ \circ }\rightarrow \vartheta$ as $m\rightarrow \infty$. By Lemma 1.5 there is $m_{\circ }\in N$ such that

$$ \frac{s^{i}\theta ^{m+1}}{s-\theta }\eta _{\circ }+s^{i-1}\theta ^{m} \eta _{\circ }\ll \hbar $$

for each $m>m_{\circ }$. Hence

$$ \max \bigl\{ \xi ( \Im p_{m+i},\Im p_{m} ) ,\xi ( \Im q_{m+i},\Im q_{m} ) ,\xi ( \Im r_{m+i},\Im r_{m} ) \bigr\} \precsim \frac{s^{i}\theta ^{m_{\circ }+1}}{s-\theta }\eta _{\circ }+s^{i-1} \theta ^{m_{\circ }}\eta _{\circ } \ll \hbar $$

for all $m>m_{\circ }$ and i. Hence Lemma 1.6 implies that $\{\Im p_{n}\}$, $\{\Im q_{n}\}$, and $\{\Im r_{n}\}$ are Cauchy sequences in χ. Since $(\chi ,\xi )$ is complete, there exist $\upsilon _{p_{\circ }},\upsilon _{q_{\circ }},\upsilon _{r_{\circ }} \in \chi$ such that

$$ \begin{aligned} &\lim_{n\rightarrow \infty }\Im \Re ^{n}(p_{\circ },q_{\circ },r_{ \circ })= \upsilon _{p_{\circ }},\qquad \lim_{n\rightarrow \infty } \Im \Re ^{n}(q_{\circ },p_{\circ },q_{\circ })=\upsilon _{q_{\circ }},\quad \text{and} \\ &\lim_{n\rightarrow \infty }\Im \Re ^{n}(r_{\circ },q_{\circ },p_{ \circ })= \upsilon _{r_{\circ }}. \end{aligned} $$

(8)

Since $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })$ have convergent subsequences when ℑ is subsequentially convergent, there are $\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }}\in \chi$ and sequences $\{p_{n_{w}}\}$, $\{q_{n_{w}}\}$, and $\{r_{n_{w}}\}$ such that

$$ \begin{aligned} &\Re ^{n_{w}}(p_{\circ },q_{\circ },r_{\circ }) \rightarrow \sigma _{p_{ \circ }},\qquad \Re ^{n_{w}}(q_{\circ },p_{\circ },q_{\circ }) \rightarrow \sigma _{q_{\circ }},\quad \text{and} \\ &\Re ^{n_{w}} \rightarrow \sigma _{r_{\circ }}\quad \text{as }w\rightarrow \infty . \end{aligned} $$

(9)

Since ℑ is continuous, we have

$$ \begin{aligned} &\lim_{w\rightarrow \infty }\Im \Re ^{n_{w}}(p_{\circ },q_{\circ },r_{ \circ })= \Im \sigma _{p_{\circ }},\qquad \lim_{w\rightarrow \infty } \Im \Re ^{n_{w}}(q_{\circ },p_{\circ },q_{\circ })=\Im \sigma _{q_{ \circ }},\quad \text{and} \\ &\lim_{w\rightarrow \infty }\Im \Re ^{n_{w}}(r_{ \circ },q_{\circ },p_{\circ })=\Im \sigma _{r_{\circ }}. \end{aligned} $$

(10)

Equations (8) and (10) yield

$$ \Im \sigma _{p_{\circ }}=\upsilon _{p_{\circ }},\qquad \Im \sigma _{q_{ \circ }}=\upsilon _{q_{\circ }},\quad \text{and}\quad \Im \sigma _{r_{\circ }}= \upsilon _{r_{\circ }}. $$

(11)

From another trend, using (10) and (11), we can write

$$ \xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im (\sigma _{p_{\circ }}) \bigr) \precsim s \left( \begin{aligned} &\xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im \Re (p_{n_{w}},q_{n_{w}},r_{n_{w}}) \bigr) \\ &\quad {}+\xi \bigl( \Im \Re (p_{n_{w}},q_{n_{w}},r_{n_{w}}), \Im (\sigma _{p_{ \circ }}) \bigr) \end{aligned}\right) . $$

(12)

Letting $w\rightarrow \infty$ in (12) and using (10), we have

$$\begin{aligned} \xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im \sigma _{p_{\circ }} \bigr) \precsim &s \bigl[ \xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im (\sigma _{p_{\circ }}) \bigr) +\xi \bigl( \Im (\sigma _{p_{\circ }}),\Im (\sigma _{p_{\circ }}) \bigr) \bigr] \\ =&s\xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im (\sigma _{p_{\circ }}) \bigr) , \end{aligned}$$

which implies that $(1-s)\xi ( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im \sigma _{p_{\circ }} ) \precsim \vartheta$. Since $1-s\neq \vartheta$, we have $\xi ( \Im \Re (\sigma _{p_{\circ }}, \sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im \sigma _{p_{\circ }} ) =\vartheta$, that is, $\Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{ \circ }})=\Im \sigma _{p_{\circ }}$. Similarly, we obtain $\Im \Re (\sigma _{q_{\circ }},\sigma _{p_{\circ }},\sigma _{q_{ \circ }})=\Im \sigma _{q_{\circ }}$ and $\Im \Re (\sigma _{r_{\circ }},\sigma _{q_{\circ }},\sigma _{p_{ \circ }})=\Im \sigma _{r_{\circ }}$. Since ℑ is one-to-one, we have $\Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }})= \sigma _{p_{\circ }}$, $\Re (\sigma _{q_{\circ }},\sigma _{p_{\circ }},\sigma _{q_{\circ }})= \sigma _{q_{\circ }}$, and $\Re (\sigma _{r_{\circ }},\sigma _{q_{\circ }},\sigma _{p_{\circ }})= \sigma _{r_{\circ }}$. Therefore there is a tripled fixed point $(\sigma _{p_{\circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }})$ of ℜ.

Let us assume that there is another TFP of ℜ of the form $(\eth _{p_{\circ }},\eth _{q_{\circ }},\eth _{r_{\circ }})$. Then

$$\begin{aligned}& \xi ( \Im \sigma _{p_{\circ }},\Im \eth _{p_{\circ }} ) \\& \quad = \xi \bigl( \Im \Re (\sigma _{p_{\circ }},\sigma _{q_{\circ }}, \sigma _{r_{\circ }}),\Im \Re (\eth _{p_{\circ }},\eth _{q_{\circ }}, \eth _{r_{\circ }}) \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im \sigma _{p_{\circ }}, \Im \eth _{p_{\circ }} ) ,\xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} ) ,\xi ( \Im \sigma _{r_{\circ }},\Im \eth _{r_{\circ }} ) \bigr\} , \end{aligned}$$

(13)

$$\begin{aligned}& \xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} ) = \xi \bigl( \Im \Re (\sigma _{q_{\circ }},\sigma _{p_{\circ }}, \sigma _{q_{\circ }}),\Im \Re (\eth _{q_{\circ }},\eth _{p_{\circ }}, \eth _{q_{\circ }}) \bigr) \\& \hphantom{\xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} )}\precsim \theta \max \bigl\{ \xi ( \Im \sigma _{q_{\circ }}, \Im \eth _{q_{\circ }} ) ,\xi ( \Im \sigma _{p_{\circ }},\Im \eth _{p_{\circ }} ) \bigr\} , \end{aligned}$$

(14)

and

$$\begin{aligned}& \xi ( \Im \sigma _{r_{\circ }},\Im \eth _{r_{\circ }} ) \\& \quad = \xi \bigl( \Im \Re (\sigma _{r_{\circ }},\sigma _{q_{\circ }}, \sigma _{p_{\circ }}),\Im \Re (\eth _{r_{\circ }},\eth _{q_{\circ }}, \eth _{p_{\circ }}) \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im \sigma _{r_{\circ }}, \Im \eth _{r_{\circ }} ) ,\xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} ) ,\xi ( \Im \sigma _{p_{\circ }},\Im \eth _{p_{\circ }} ) \bigr\} . \end{aligned}$$

(15)

It follows by (13)–(15) that

$$\begin{aligned}& \max \bigl\{ \xi ( \Im \sigma _{p_{\circ }},\Im \eth _{p_{ \circ }} ) , \xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{ \circ }} ) ,\xi ( \Im \sigma _{r_{\circ }},\Im \eth _{r_{ \circ }} ) \bigr\} \\& \quad \precsim \theta \max \bigl\{ \xi ( \Im \sigma _{p_{\circ }}, \Im \eth _{p_{\circ }} ) ,\xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} ) ,\xi ( \Im \sigma _{r_{\circ }},\Im \eth _{r_{\circ }} ) \bigr\} . \end{aligned}$$

By Lemma 1.7 we get

$$ \xi ( \Im \sigma _{p_{\circ }},\Im \eth _{p_{\circ }} ) = \xi ( \Im \sigma _{q_{\circ }},\Im \eth _{q_{\circ }} ) = \xi ( \Im \sigma _{r_{\circ }},\Im \eth _{r_{\circ }} ) = \vartheta . $$

Hence $\Im \sigma _{p_{\circ }}=\Im \eth _{p_{\circ }}$, $\Im \sigma _{q_{\circ }}=\Im \eth _{q_{\circ }}$, and $\Im \sigma _{r_{\circ }}=\Im \eth _{r_{\circ }}$. Since ℑ is one-to-one, we can write $(\eth _{p_{\circ }},\eth _{q_{\circ }},\eth _{r_{\circ }})=(\sigma _{p_{ \circ }},\sigma _{q_{\circ }},\sigma _{r_{\circ }})$. Finally, if ℑ is subsequentially convergent, then substituting $n_{w}$ with n into (9), we can get

$$ \Re ^{n}(p_{\circ },q_{\circ },r_{\circ }) \rightarrow \sigma _{p_{ \circ }},\qquad \Re ^{n}(q_{\circ },p_{\circ },q_{\circ }) \rightarrow \sigma _{q_{ \circ }},\quad \text{and}\quad \Re ^{n} \rightarrow \sigma _{r_{\circ }} \quad \text{as }n\rightarrow \infty . $$

This ends the proof. □

Example 2.4

Let $\chi =[0,1]$, $B=\mathbb{R} ^{2}$, and $i>1$. Specify $Q=\{(p,q)\in B: p,q\geq 0\}$. Realize the function $\xi :\chi ^{2}\rightarrow B$ as

$$ \xi (\wp ,\partial )= \bigl( \vert \wp -\partial \vert ^{i}, \vert \wp -\partial \vert ^{i} \bigr) \quad \text{for } \wp , \partial \in {}[ 0,1]. $$

Assuming that $\Im :\chi \rightarrow \chi$ and $\Re :\chi ^{3}\rightarrow \chi$ are defined as

$$ \Im (p)=\frac{1}{6}p\quad \text{and}\quad \Re (p,q,r)=\frac{p}{8}- \frac{q+r}{16}\quad \text{for } p,q,r\in \chi . $$

It is obvious that $(B,\xi )$ is a CCbMS and ℑ is continuous and one-to-one. To fulfill (11), we take $\theta =\frac{1}{8^{i}}$, $i>1$. Then for all $p,q,r\in \chi$, we have

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad = \bigl( \bigl\vert \Im \Re (p,q,r)-\Im \Re \bigl(p^{\ast },q^{ \ast },r^{\ast } \bigr) \bigr\vert ^{i}, \bigl\vert \Im \Re (p,q,r)-\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad = \frac{1}{6^{i}}\left(\begin{aligned}& \biggl\vert \biggl( \frac{p}{8}-\frac{q+r}{16} \biggr) - \biggl( \frac{p^{\ast }}{8}-\frac{q^{\ast }+r^{\ast }}{16} \biggr) \biggr\vert ^{i}, \\ & \biggl\vert \biggl( \frac{p}{8}-\frac{q+r}{16} \biggr) - \biggl( \frac{p^{\ast }}{8}-\frac{q^{\ast }+r^{\ast }}{16} \biggr) \biggr\vert ^{i}\end{aligned}\right) \\& \quad \precsim \frac{1}{ ( 48 ) ^{i}} \bigl( \bigl\vert p-p^{ \ast } \bigr\vert ^{i}, \bigl\vert p-p^{\ast } \bigr\vert ^{i} \bigr) \\& \quad = \frac{1}{8^{i}} \biggl( \biggl\vert \frac{p-p^{\ast }}{6} \biggr\vert ^{i}, \biggl\vert \frac{p-p^{\ast }}{6} \biggr\vert ^{i} \biggr) \\& \quad = \frac{1}{8^{i}}\xi \bigl( \Im p,\Im p^{\ast } \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) , \xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r, \Im r^{\ast } \bigr) \bigr\} . \end{aligned}$$

Therefore ℜ is an $\Im _{\theta }$-contraction. Now we verify the remaining conditions as follows:

• (a) let $(\frac{1}{6},\frac{1}{6},\frac{1}{6})\in \chi$, and consider the iterative sequences $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })=\{\frac{1}{n}\}_{n\in N}=p_{n}$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })=\{\frac{n^{2}}{n+7}\}_{n\in N}=q_{n}$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })=\{\frac{1}{e^{n}}\}_{n\in N}=r_{n}$. Then

  $$\begin{aligned}& \lim_{n\rightarrow \infty }\Im \Re ^{n}(p_{\circ },q_{\circ },r_{ \circ }) = \Im \Bigl( \lim_{n\rightarrow \infty }\Re ^{n}(p_{ \circ },q_{\circ },r_{\circ }) \Bigr) =\Im \biggl( \lim_{n \rightarrow \infty }\frac{1}{n} \biggr) =\Im ( 0 ) =\frac{1}{6},\text{ } \\& \lim_{n\rightarrow \infty }\Im \Re ^{n}(q_{\circ },p_{\circ },q_{ \circ }) = \Im \biggl( \lim_{n\rightarrow \infty }\frac{n^{2}}{n+7} \biggr) =\Im ( 0 ) =\frac{1}{6}, \\& \lim_{n\rightarrow \infty }\Im \Re ^{n}(r_{\circ },q_{\circ },p_{ \circ }) = \Im \biggl( \lim_{n\rightarrow \infty }\frac{1}{e^{n}} \biggr) =\Im ( 0 ) =\frac{1}{6}; \end{aligned}$$

• (b) intuitively achieved;

• (c) there is a unique point $(0,0,0)\in \chi$, as required;

• (d) if ℑ is subsequentially convergent, then the sequences $\Re ^{n}(p_{\circ },q_{\circ },r_{\circ })$, $\Re ^{n}(q_{\circ },p_{\circ },q_{\circ })$, and $\Re ^{n}(r_{\circ },q_{\circ },p_{\circ })$ converge to $(0,0,0)\in \chi$.

Hence all the conditions of Theorem 2.3 are fulfilled, and $(0,0,0)$ is a unique TFP of the mapping ℜ.

Theorem 2.5

Suppose that $(\chi ,\xi )$ is a CCbMS with coefficient $s\geq 1$, B is a solid cone with $Q\cup (-Q)=B$, $\Im :\chi \rightarrow \chi$ is a continuous one-to-one mapping, and $\Re :\chi ^{3}\rightarrow \chi$ is a mapping such that

$$ \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \precsim \theta \max \bigl\{ \xi \bigl( \Im \Re (p,q,r), \Im p \bigr) ,\xi \bigl( \Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr), \Im p^{\ast } \bigr) \bigr\} $$

for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$, where $\theta \in {}[ 0,1)$. Then the conclusion of Theorem 2.3is achieved.

Proof

The proof immediately follows from Theorem 2.3. □

Theorem 2.6

Suppose that $(\chi ,\xi )$ is a CCbMS with coefficient $s\geq 1$, B is a solid cone with $Q\cup (-Q)=B$, $\Im :\chi \rightarrow \chi$ is a continuous one-to-one mapping, and $\Re :\chi ^{3}\rightarrow \chi$ is a mapping such that

$$ \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \precsim \theta \max \bigl\{ \xi \bigl( \Im \Re (p,q,r), \Im p^{\ast } \bigr) ,\xi \bigl( \Im \Re \bigl(p^{\ast },q^{\ast },r^{ \ast } \bigr),\Im p \bigr) \bigr\} $$

for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$, where $\theta \in {}[ 0,1)$. Then the conclusion of Theorem 2.3is achieved.

Proof

The proof immediately follows from Theorem 2.3. □

The following corollary contains some contractive terms inspired by Theorem 2.3.

Corollary 2.7

Suppose that $(\chi ,\xi )$ is a CCbMS with coefficient $s\geq 1$, B is a solid cone with $Q\cup (-Q)=B$, $\Im :\chi \rightarrow \chi$ is a continuous one-to-one mapping, and $\Re :\chi ^{3}\rightarrow \chi$ is a mapping such that one of the following conditions holds:

$(\spadesuit _{1})$:

$$ \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \precsim \theta \xi \bigl( \Im p,\Im p^{\ast } \bigr), $$

$(\spadesuit _{2})$:

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad \precsim \theta \max \left\{ \begin{aligned} &\xi \bigl( \Im p,\Im p^{\ast } \bigr) ,\xi \bigl( \Im q,\Im q^{ \ast } \bigr) ,\xi \bigl( \Im r,\Im r^{\ast } \bigr) , \\ &\frac{\xi ( \Im p,\Im p^{\ast } ) +\xi ( \Im q,\Im q^{\ast } ) +\xi ( \Im r,\Im r^{\ast } ) }{3}\end{aligned} \right\} , \end{aligned}$$

$(\spadesuit _{3})$:

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad \precsim \theta \max \left\{ \begin{aligned} &\xi \bigl( \Im \Re (p,q,r),\Im p \bigr) ,\xi \bigl( \Im \Re \bigl(p^{ \ast },q^{\ast },r^{\ast } \bigr),\Im p \bigr) , \\ & \frac{\xi ( \Im \Re (p,q,r),\Im p^{\ast } ) +\xi ( \Im \Re (p^{\ast },q^{\ast },r^{\ast }),\Im p ) }{2}\end{aligned} \right\} , \end{aligned}$$

$(\spadesuit _{4})$:

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad \precsim \theta \max \left\{ \begin{aligned} &\xi \bigl( \Im \Re (p,q,r),\Im p^{\ast } \bigr) ,\xi \bigl( \Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr),\Im p \bigr) , \\ & \frac{\xi ( \Im \Re (p,q,r),\Im p^{\ast } ) +\xi ( \Im \Re (p^{\ast },q^{\ast },r^{\ast }),\Im p ) }{2}\end{aligned} \right\} \end{aligned}$$

for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$, where $\theta \in {}[ 0,1)$. Then the conclusion of Theorem 2.3is achieved.

Now we can discuss another generalization of our results.

Lemma 2.8

Let $(\chi ,\xi )$ be a CCbMS with coefficient $s\geq 1$. Then we have the following two properties:

$(\heartsuit _{1})$:

$(\chi \times \chi \times \chi ,\xi _{1})$ is a CCbMS equipped with

$$ \xi _{1} \bigl((p,q,r), \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr)=\max \bigl\{ \xi \bigl(p,p^{ \ast } \bigr),\xi \bigl(q,q^{\ast } \bigr),\xi \bigl(r,r^{\ast } \bigr) \bigr\} ; $$

$(\heartsuit _{2})$:

If the mapping $\Gamma _{\Re }:\chi ^{3}\rightarrow \chi ^{3}$ defined by the relation $\Gamma _{\Re }(p,q,r)= ( \Re (p,q,r), \Re (q,p,q),\Re (r,q,p) )$ has a fixed point in $\chi ^{3}$, then the mapping $\Re :\chi ^{3}\rightarrow \chi$ has a tripled fixed point, and the opposite is generally true.

Proof

$(\heartsuit _{1})$ Hypotheses $(cb_{1})$ and $(cb_{1})$ of Definition 1.3 are fulfilled. Just check the triangle inequality. Since $(\chi ,\xi )$ is a CbMS, we have

$$\begin{aligned}& \xi _{1} \bigl((p,q,r), \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad = \max \bigl\{ \xi \bigl(p,p^{\ast } \bigr),\xi \bigl(q,q^{\ast } \bigr),\xi \bigl(r,r^{\ast } \bigr) \bigr\} \\& \quad \precsim \max \bigl\{ s \bigl( \xi (p,\wp )+\xi \bigl(\wp ,p^{\ast } \bigr) \bigr) ,s \bigl( \xi (q,\partial )+\xi \bigl(\partial ,q^{\ast } \bigr) \bigr) ,s \bigl( \xi (r,\hbar )+\xi \bigl(\hbar ,r^{\ast } \bigr) \bigr) \bigr\} \\& \quad \precsim s \bigl( \max \bigl\{ \xi (p,\wp ),\xi (q,\partial ),\xi (r, \hbar ) \bigr\} +\max \bigl\{ \xi \bigl(\wp ,p^{\ast } \bigr),\xi \bigl(\partial ,q^{ \ast } \bigr),\xi \bigl(\hbar ,r^{\ast } \bigr) \bigr\} \bigr) \\& \quad = s \bigl( \xi _{1} \bigl((p,q,r),(\wp ,\partial ,\hbar ) \bigr)+ \xi _{1} \bigl((\wp , \partial ,\hbar ), \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \bigr) \end{aligned}$$

for all $(p,q,r),(p^{\ast },q^{\ast },r^{\ast }),(\wp ,\partial ,\hbar )\in \chi ^{3}$. Again, the completeness of $(\chi ,\xi )$ leads to the completeness of $(\chi ^{3},\xi _{1})$. Therefore $(\chi ^{3},\xi _{1})$ is a CCbMS.

$(\heartsuit _{2})$ Let there is a tripled fixed point $(p,q,r)$ of ℜ, that is, $\Re (p,q,r)=p$, $\Re (q,p,q)=q$, and $\Re (r,q,p)=r$. Hence

$$ \Gamma _{\Re }(p,q,r)= \bigl( \Re (p,q,r),\Re (q,p,q),\Re (r,q,p) \bigr) =(p,q,r), $$

that is, a triple $(p,q,r)$ is a fixed point of $\Gamma _{\Re }$. Vice versa, Let $(p,q,r)\in \chi ^{3}$ be a fixed point of the mapping $\Gamma _{\Re }$. Then $\Gamma _{\Re }(p,q,r)=(p,q,r)$, which yields, $\Re (p,q,r)=p$, $\Re (q,p,q)=q$, and $\Re (r,q,p)=r$. □

Theorem 2.9

Assume that $(\chi ,\xi )$ is a CCbMS with coefficient $s\geq 1$, B is a total ordering solid cone, $\Im :\chi \rightarrow \chi$ is a continuous one-to-one mapping, and $\Re :\chi ^{3}\rightarrow \chi$ is a mapping such that the following hypothesis are satisfied:

$$\begin{aligned}& \max \left\{ \begin{aligned} &\xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) , \\ &\xi \bigl( \Im \Re (q,p,q),\Im \Re \bigl(q^{\ast },p^{\ast },q^{\ast } \bigr) \bigr) , \\ &\xi \bigl( \Im \Re (r,q,p),\Im \Re \bigl(r^{\ast },q^{\ast },p^{\ast } \bigr) \bigr)\end{aligned} \right\} \\& \quad \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{ \ast } \bigr) ,\xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r,\Im r^{\ast } \bigr) \bigr\} \end{aligned}$$

(16)

for all $p,q,r,p^{\ast },q^{\ast },r^{\ast }\in \chi$, where $\theta \in {}[ 0,1)$. Then the conclusion of Theorem 2.3is achieved.

Proof

We begin by defining the mapping as . Note that is one-to-one and continuous. Allocate $\aleph =(p,q,r)$ and $\gimel =(p^{\ast },q^{\ast },r^{\ast })\in \chi ^{3}$ and apply the hypotheses of Lemma 2.8. Then condition (16) reduces to

So, the proof is finished by condition $(\spadesuit _{1})$ of Corollary 2.7. □

Example 2.10

Let $\chi =[0,1]$, and let $B=C_{\mathbb{R} }^{1}[0,1]$ be a family of real-valued functions on χ with continuous derivatives on χ. Define the distance $\xi (p,q)= \vert p-q \vert ^{i}e^{\kappa }$, where $i\geq 1$ is a constant, and $e^{\kappa }\in B$ on $Q= \{ \psi \in B:\psi \geq 0 \}$. Hence $(\chi ,\xi )$ is a CCbMS with coefficient $s=2^{i}$. Define two mappings $\Im :\chi \rightarrow \chi$ and $\Re :\chi ^{3}\rightarrow \chi$ by

$$ \Im (p)=\frac{1}{3}p\quad \text{and}\quad \Re (p,q,r)=\frac{p+q-r}{7},\quad \forall p,q,r\in \chi . $$

It is easy to write

$$\begin{aligned}& \xi \bigl( \Im \Re (p,q,r),\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\& \quad = \bigl\vert \Im \Re (p,q,r)-\Im \Re \bigl(p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr\vert ^{i}e^{\kappa } \\& \quad = \biggl\vert \frac{p+q-r}{21}- \frac{p^{\ast }+q^{\ast }-r^{\ast }}{21} \biggr\vert ^{i}e^{\kappa } \\& \quad = \frac{e^{\kappa }}{(21)^{i}} \bigl( \bigl\vert \bigl(p-p^{\ast } \bigr)+ \bigl(q-q^{ \ast } \bigr)- \bigl(r-r^{\ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad \precsim \frac{e^{\kappa }}{(21)^{i}} \bigl\{ \bigl\vert p-p^{ \ast } \bigr\vert ^{i}+ \bigl\vert q-q^{\ast } \bigr\vert ^{i}+ \bigl\vert \bigl(r-r^{\ast } \bigr) \bigr\vert ^{i} \bigr\} \\& \quad \precsim \frac{3}{7^{i}}\max \biggl\{ \biggl\vert \frac{p-p^{\ast }}{3} \biggr\vert ^{i}e^{\kappa }, \biggl\vert \frac{q-q^{\ast }}{3} \biggr\vert ^{i}e^{\kappa }, \biggl\vert \frac{r-r^{\ast }}{3} \biggr\vert ^{i}e^{ \kappa } \biggr\} \\& \quad = \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) ,\xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r,\Im r^{\ast } \bigr) \bigr\} , \end{aligned}$$

(17)

$$\begin{aligned}& \xi \bigl( \Im \Re (q,p,q),\Im \Re \bigl(q^{\ast },p^{\ast },q^{\ast } \bigr) \bigr) \\& \quad = \biggl\vert \frac{q+p-q}{21}- \frac{q^{\ast }+p^{\ast }-q^{\ast }}{21} \biggr\vert ^{i}e^{\kappa } \\& \quad = \frac{e^{\kappa }}{(21)^{i}} \bigl( \bigl\vert \bigl(p-p^{\ast } \bigr) \bigr\vert ^{i} \bigr) \\& \quad \precsim \frac{1}{(7)^{i}} \biggl\{ \biggl\vert \frac{p-p^{\ast }}{3} \biggr\vert ^{i}e^{\kappa } \biggr\} \\& \quad \precsim \frac{3}{7^{i}}\xi \bigl( \Im p,\Im p^{\ast } \bigr) \\& \quad \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) , \xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r, \Im r^{\ast } \bigr) \bigr\} . \end{aligned}$$

(18)

Similarly, we have

$$ \xi \bigl( \Im \Re (r,q,p),\Im \Re \bigl(r^{\ast },q^{\ast },p^{\ast } \bigr) \bigr) \precsim \theta \max \bigl\{ \xi \bigl( \Im p,\Im p^{\ast } \bigr) ,\xi \bigl( \Im q,\Im q^{\ast } \bigr) ,\xi \bigl( \Im r, \Im r^{\ast } \bigr) \bigr\} . $$

(19)

Therefore relations (17)–(19) demonstrate verification of stipulation (16) for all $p,q,r, p^{\ast },q^{\ast },r^{\ast }\in \chi$ and $\theta =\frac{3}{7^{i}}$. As in Example 2.4, we find that the remaining conditions of Theorem 2.3 are fulfilled. Thus a triple $(0,0,0)$ is a unique tripled fixed point of ℜ.

3 Solve a tripled system of integral equations

In this part, we use the theoretical results obtained in the previous section to clarify the existence and uniqueness of the solution for the following system:

(20)

for all $\gimel \in {}[ 0,\wp ]$. Postulate the following assumptions:

$\blacktriangledown _{1}$:

$\aleph :[0,\wp ]\rightarrow \mathbb{R}$ and are continuous;

$\blacktriangledown _{2}$:

$\Game _{l}:[0,\wp ]\times \mathbb{R} \rightarrow \mathbb{R}$ ($l=1,2,3$) are continuous;

$\blacktriangledown _{3}$:

there is a constant $\amalg >0$ such that for all $p,q\in \mathbb{R}$,

$$\begin{aligned}& 0 \precsim \Game _{1}(\mho ,p)-\Game _{1}(\mho ,q) \precsim \amalg (p-q), \\& 0 \precsim \Game _{2}(\mho ,p)-\Game _{2}(\mho ,q) \precsim \amalg (p-q), \\& 0 \precsim \Game _{3}(\mho ,p)-\Game _{3}(\mho ,q) \precsim \amalg (p-q); \end{aligned}$$

$\blacktriangledown _{4}$:

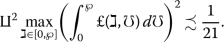
Let $\chi =C([0,\wp ],\mathbb{R} )$ be the set of all continuous real-valued functions on $[0,\wp ]$ taking values in $\mathbb{R}$, and let $Q=\{\pi \in B:\pi \geq 0\}$. Set $\xi :\chi \times \chi \rightarrow B$ as $\xi (o,O)=e^{\gimel }\max_{\gimel \in {}[ 0,\wp ]} \vert o( \gimel )-O(\gimel ) \vert ^{2}$. It is obvious that $(\chi ,\xi )$ is a CCbMS.

Our main theorem is the following:

Theorem 3.1

Under hypotheses $(\blacktriangledown _{1})$–$(\blacktriangledown _{4})$, problem (20) has a solution in $\chi ^{3}$, where $\chi =C([0,\wp ],\mathbb{R} )$.

Proof

Define the operators $\Re :\chi ^{3}\rightarrow \chi$ and $\Im :\chi \rightarrow \chi$ by

and $\Im (\zeta )=\zeta$ for all $\gimel \in {}[ 0,\wp ]$ and $\zeta _{1},\zeta _{2},\zeta _{3}\in \chi$. The triple $(\zeta _{1},\zeta _{2},\zeta _{3})$ is a solution of system (20) if and only if $(\zeta _{1},\zeta _{2},\zeta _{3})$ is a tripled fixed point of ℜ. The existence of this triple follows from Theorem 2.3, since ℑ is the identity mapping. Therefore it is necessary to fulfill the remaining conditions of Theorem 2.3. For all $\zeta _{1},\zeta _{2},\zeta _{3}\in \chi$ and $\gimel \in {}[ 0,\wp ]$, setting $\theta =\frac{3}{7}$, we get

This means that condition (1) of Theorem 2.3 is fulfilled. Thus ℜ has a tripled fixed point $(\zeta _{1},\zeta _{2},\zeta _{3})\in C([0,\wp ],\mathbb{R} )\times C([0,\wp ],\mathbb{R} )\times C([0,\wp ],\mathbb{R} )$, which, at the same time, is a solution to problem (20). □

4 A stationary distribution of the Markov process

Suppose that $\mathbb{R} _{+}^{n}=\{(\delta _{1},\delta _{2},\ldots,\delta _{n})= \delta :\delta _{\ell }\geq 0, \ell \geq 1\}$ and $\Omega _{n-1}^{3}=\{\sigma =(p,q,r)\in \mathbb{R} _{+}^{n}\times \mathbb{R} _{+}^{n}\times \mathbb{R} _{+}^{n}:\sum_{\ell =1}^{n}\sigma _{\ell }=\sum_{\ell =1}^{n} ( p_{\ell }+q_{\ell }+r_{\ell } ) =1 \}$ refer to the $3(n-1)$-dimensional unit simplex and $\sigma \in \Omega _{n-1}^{3}$ is considered as a possibility over 3n possible statuses. Here the Markov process is a stochastic process such that 3n statuses are realized in each period $\gimel =1,2,\ldots$ with probability contingent on the current achieved status. Assume that $a_{\ell \kappa }$ refer to the probability contingent that status ℓ is achieved in the subsequent period beginning in status κ. Hence in periods ℷ and $\gimel +1$ the prior probability vector $\sigma ^{\gimel }$ and the posterior probability $\sigma ^{\gimel +1}$ are given by $\sigma _{\ell }^{\gimel +1}=\sum_{\ell }a_{\ell \kappa } \sigma _{\kappa }^{\gimel }$ for each $\ell \geq 1$. To write this in matrix form, we consider $\sigma ^{\gimel }$ as a column vector, and then $\sigma ^{\gimel +1}=\Theta \sigma ^{\gimel }$. Note that $a_{\ell \kappa }\geq 0$ and $\sum_{\ell =1}^{n}a_{\ell \kappa }=1$, which is required for conditional probability. The vector $\sigma ^{\gimel }$ is called a stationary distribution of the Markov process at any period if $\sigma ^{\gimel }=\sigma ^{\gimel +1}$. This mean that the problem of finding a stationary distribution is equivalent to the fixed point problem $\Theta \sigma ^{\gimel }=\sigma ^{\gimel }$.

Suppose that $\delta _{\ell }=\min_{\kappa }a_{\ell \kappa }$ and define $\delta =\sum_{\ell =1}^{n}\delta _{\ell }$.

Before presenting our main theorem of this section, we need the following inequality.

Minkowski’s Inequality

(Theorem 6, [42], p. 25)

For $p_{i},q_{i}\geq 0$ and $\alpha >1$, we have

$$ \Biggl[ \sum_{i=1}^{n} ( p_{i}+q_{i} ) ^{\alpha } \Biggr] ^{\frac{1}{\alpha }} \leq \Biggl[ \sum_{i=1}^{n}p_{i}^{\alpha } \Biggr] ^{\frac{1}{\alpha }}+ \Biggl[ \sum_{i=1}^{n}q_{i}^{\alpha } \Biggr] ^{\frac{1}{\alpha }}. $$

Theorem 4.1

There exists a unique stationary distribution for the Markov process whenever $a_{\ell \kappa }\geq 0$.

Proof

Let $\xi :\Omega _{n-1}^{3}\times \Omega _{n-1}^{3}\rightarrow \mathbb{R} ^{2}$ be defined by

$$\begin{aligned} \xi ( \Phi ,\Psi ) =&\xi \bigl( ( p,q,r ) , \bigl( p^{\ast },q^{\ast },r^{\ast } \bigr) \bigr) \\ =& \left( \begin{aligned} &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert p_{ \ell }-p_{\ell }^{\ast } \bigr\vert + \bigl\vert q_{\ell }-q_{\ell }^{ \ast } \bigr\vert + \bigl\vert r_{\ell }-r_{\ell }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum _{\ell =1}^{n} \bigl( \bigl\vert p_{\ell }-p_{\ell }^{ \ast } \bigr\vert + \bigl\vert q_{\ell }-q_{\ell }^{\ast } \bigr\vert + \bigl\vert r_{\ell }-r_{\ell }^{\ast } \bigr\vert \bigr) ^{ \alpha } \Biggr\} ^{\frac{1}{\alpha }} \end{aligned} \right) \end{aligned}$$

for all $\Phi ,\Psi \in \Omega _{n-1}^{3}$, with $\alpha \geq 1$.

It is obvious that $\xi ( \Phi ,\Psi ) \geq (0,0)$ for all $\Phi ,\Psi \in \Omega _{n-1}^{3}$, and if $\xi ( \Phi ,\Psi ) =(0,0)$, then this leads to $( \{ \sum_{\ell =1}^{n} ( \vert p_{ \ell }-p_{\ell }^{\ast } \vert + \vert q_{\ell }-q_{\ell }^{ \ast } \vert + \vert r_{\ell }-r_{\ell }^{\ast } \vert ) ^{\alpha } \} ^{\frac{1}{\alpha }}, \{ \sum_{\ell =1}^{n} ( \vert p_{\ell }-p_{\ell }^{ \ast } \vert + \vert q_{\ell }-q_{\ell }^{\ast } \vert + \vert r_{\ell }-r_{\ell }^{\ast } \vert ) ^{ \alpha } \} ^{\frac{1}{\alpha }} ) =(0,0)$, and thus $\vert p_{\ell }-p_{\ell }^{\ast } \vert + \vert q_{ \ell }-q_{\ell }^{\ast } \vert + \vert r_{\ell }-r_{\ell }^{ \ast } \vert =0$ for all ℓ, which leads to $p=p^{\ast }$, $q=q^{\ast }$, and $r=r^{\ast }$, that is, $\Phi =\Psi$. On the other hand, if $\Phi =\Psi$, then $p_{\ell }=p_{\ell }^{\ast }$, $q_{\ell }=q_{\ell }^{\ast }$, and $r_{\ell }=r_{\ell }^{\ast }$ for all ℓ, and thus $\vert p_{\ell }-p_{\ell }^{\ast } \vert = \vert q_{ \ell }-q_{\ell }^{\ast } \vert = \vert r_{\ell }-r_{\ell }^{ \ast } \vert =0$, which yields $( \{ \sum_{\ell =1}^{n} ( \vert p_{ \ell }-p_{\ell }^{\ast } \vert + \vert q_{\ell }-q_{\ell }^{ \ast } \vert + \vert r_{\ell }-r_{\ell }^{\ast } \vert ) ^{\alpha } \} ^{\frac{1}{\alpha }}, \{ \sum_{\ell =1}^{n} ( \vert p_{\ell }-p_{\ell }^{ \ast } \vert + \vert q_{\ell }-q_{\ell }^{\ast } \vert + \vert r_{\ell }-r_{\ell }^{\ast } \vert ) ^{ \alpha } \} ^{\frac{1}{\alpha }} ) =0\Rightarrow \xi ( \Phi ,\Psi ) =0$.

We also have

$$\begin{aligned} \xi ( \Phi ,\Psi ) =& \left( \begin{aligned}&\Biggl\{ \sum_{ \ell =1}^{n} \bigl( \bigl\vert p_{\ell }-p_{\ell }^{\ast } \bigr\vert + \bigl\vert q_{\ell }-q_{\ell }^{\ast } \bigr\vert + \bigl\vert r_{\ell }-r_{\ell }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum _{\ell =1}^{n} \bigl( \bigl\vert p_{\ell }-p_{\ell }^{\ast } \bigr\vert + \bigl\vert q_{\ell }-q_{\ell }^{\ast } \bigr\vert + \bigl\vert r_{\ell }-r_{ \ell }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{ \frac{1}{\alpha }} \end{aligned} \right) \\ =& \left( \begin{aligned} &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert p_{ \ell }^{\ast }-p_{\ell } \bigr\vert + \bigl\vert q_{\ell }^{\ast }-q_{ \ell } \bigr\vert + \bigl\vert r_{\ell }^{\ast }-r_{\ell } \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum _{\ell =1}^{n} \bigl( \bigl\vert p_{\ell }^{\ast }-p_{ \ell } \bigr\vert + \bigl\vert q_{\ell }^{\ast }-q_{\ell } \bigr\vert + \bigl\vert r_{\ell }^{\ast }-r_{\ell } \bigr\vert \bigr) ^{ \alpha } \Biggr\} ^{\frac{1}{\alpha }} \end{aligned} \right) \\ =&\xi ( \Psi ,\Phi ) . \end{aligned}$$

Now we can write

$$\begin{aligned}& \xi ( \Phi ,\Xi ) \\& \quad = \left( \begin{aligned} &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \vert p_{ \ell }-\tau _{\ell } \vert + \vert q_{\ell }-\eta _{\ell } \vert + \vert r_{\ell }- \omega _{\ell } \vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \vert p_{\ell }-\tau _{\ell } \vert + \vert q_{\ell }-\eta _{\ell } \vert + \vert r_{\ell }- \omega _{\ell } \vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }} \end{aligned} \right) \\& \quad = \left(\begin{aligned} &\left\{ \sum_{\ell =1}^{n} \left( \begin{aligned} &\bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) + \bigl( p_{\ell }^{\ast }-\tau _{ \ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }-q_{\ell }^{ \ast } \bigr) + \bigl( q_{\ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert \\ &\quad {}+ \bigl\vert \bigl( r_{\ell }-r_{\ell }^{\ast } \bigr)+ \bigl( r_{\ell }^{\ast }-\omega _{\ell } \bigr) \bigr\vert \end{aligned} \right) ^{\alpha } \right\} ^{\frac{1}{\alpha }}, \\ &\left\{ \sum_{\ell =1}^{n} \left( \begin{aligned} &\bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) + \bigl( p_{\ell }^{\ast }-\tau _{ \ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }-q_{\ell }^{ \ast } \bigr) + \bigl( q_{\ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert \\ &\quad {}+ \bigl\vert \bigl( r_{\ell }-r_{\ell }^{\ast } \bigr) + \bigl( r_{\ell }^{\ast }-\omega _{\ell } \bigr) \bigr\vert \end{aligned} \right) ^{\alpha } \right\} ^{\frac{1}{\alpha }}\end{aligned}\right) \\& \quad \precsim \left(\begin{aligned} &\left\{ \begin{aligned} &\sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert \\ &\quad {}+ \bigl\vert \bigl( r_{\ell }-r_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }^{\ast }- \omega _{\ell } \bigr) \bigr\vert \bigr) ^{ \alpha } \end{aligned} \right\} ^{\frac{1}{\alpha }}, \\ &\left\{ \begin{aligned} &\sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert \\ &\quad {}+ \bigl\vert \bigl( r_{\ell }-r_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }^{\ast }- \omega _{\ell } \bigr) \bigr\vert \bigr) ^{ \alpha } \end{aligned} \right\} ^{\frac{1}{\alpha }}\end{aligned}\right) \\& \quad \precsim 2^{\alpha }\left(\begin{aligned} &\left(\begin{aligned} &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }-r_{\ell }^{\ast } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }} \\ &\quad{} + \Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( r_{\ell }^{\ast }- \omega _{\ell } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}\end{aligned}\right) , \\ &\left(\begin{aligned}& \Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }-r_{\ell }^{\ast } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }} \\ &\quad {}+ \Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( r_{\ell }^{\ast }- \omega _{\ell } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}\end{aligned}\right) \end{aligned}\right) \\& \quad = 2^{\alpha }\left\{ \left(\begin{aligned} &\Biggl\{ \sum _{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }-r_{\ell }^{\ast } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }-p_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( q_{ \ell }-q_{\ell }^{\ast } \bigr) \bigr\vert + \bigl\vert \bigl( r_{ \ell }-r_{\ell }^{\ast } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}\end{aligned}\right)\right. \\& \qquad {}\left. + \left(\begin{aligned} &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }^{\ast }- \eta _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( r_{\ell }^{\ast }-\omega _{\ell } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}, \\ &\Biggl\{ \sum_{\ell =1}^{n} \bigl( \bigl\vert \bigl( p_{ \ell }^{\ast }-\tau _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( q_{\ell }^{\ast }-\eta _{\ell } \bigr) \bigr\vert + \bigl\vert \bigl( r_{\ell }^{\ast }- \omega _{\ell } \bigr) \bigr\vert \bigr) ^{\alpha } \Biggr\} ^{\frac{1}{\alpha }}\end{aligned}\right) \right\} \\& \quad = s \bigl( \xi ( \Phi ,\Psi ) +\xi ( \Psi ,\Xi ) \bigr) \end{aligned}$$

for $\Xi = ( \tau ,\eta ,\omega ) \in \Omega _{n-1}^{3}$. Thus $(\Omega _{n-1}^{3},\xi )$ is a CbMS with $s=2^{\alpha }$ and $Q=\{(\delta _{1},\delta _{2},\ldots,\delta _{n}):\delta _{\ell }\geq 0, \ell \geq 1\}$.

Let $\Psi =\Theta \sigma$ for all $\Psi \in \Omega _{n-1}^{3}$. Therefore each $\rho _{\ell }=\sum_{\kappa =1}^{n}a_{\ell \kappa }\sigma _{ \kappa }\geq 0$. Moreover, since each $\sum_{\kappa =1}^{n}a_{\ell \kappa }=1$, we get

$$ \sum_{\ell =1}^{n}\rho _{\ell }= \sum_{\ell =1}^{n} \sum _{\kappa =1}^{n}a_{\ell \kappa }\sigma _{\kappa }=\sum_{\kappa =1}^{n}a_{\ell \kappa } \sum_{\kappa =1}^{n}(p_{ \kappa }+q_{\kappa }+r_{\kappa })= \sum_{\kappa =1}^{n} ( (p_{\kappa }+q_{\kappa }+r_{\kappa } ) =1, $$

which shows that $\Psi \in \Omega _{n-1}^{3}$. Thus we can write $\Theta :\Omega _{n-1}^{3}\rightarrow \Omega _{n-1}^{3}$. Now we will prove that Θ is a contraction.

Let $\Theta _{\ell }$ refer to the ℓth row of Θ. So for any $( p,q,r ) ,(p^{\ast },q^{\ast },r^{\ast })\in \Omega _{n-1}^{3}$ and all $\alpha \geq 1$, we get (note that $\sum_{\kappa =1}^{n} ( p_{\kappa }+q_{\kappa }+r_{ \kappa } ) =1$)

$$\begin{aligned}& \xi \bigl( \Theta ( p,q,r ) ,\Theta \bigl(p^{\ast },q^{ \ast },r^{\ast } \bigr) \bigr) \\& \quad = \left( \begin{aligned} &\sum_{\ell =1}^{n} \Biggl( \Biggl\vert \sum_{\kappa =1}^{n}a_{\ell \kappa }(p_{\kappa }+q_{\kappa }+r_{ \kappa })-a_{\ell \kappa } \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{\ast }+r_{ \kappa }^{\ast } \bigr) \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }} , \\ & \sum_{\ell =1}^{n} \Biggl( \Biggl\vert \sum_{\kappa =1}^{n}a_{\ell \kappa }(p_{\kappa }+q_{\kappa }+r_{ \kappa })-a_{\ell \kappa } \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{\ast }+r_{ \kappa }^{\ast } \bigr) \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }}\end{aligned} \right) \\& \quad = \left( \begin{aligned} &\sum_{\ell =1}^{n} \left( \left \vert \begin{aligned} &\sum_{\kappa =1}^{n}(a_{\ell \kappa }- \delta _{\ell }) \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{ \ast }+r_{\kappa }^{\ast } \bigr) \bigr] \\ &\quad {}+\delta _{\ell } \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{ \kappa }^{\ast }+q_{\kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \end{aligned} \right \vert ^{\alpha } \right) ^{\frac{1}{\alpha }} , \\ &\sum_{\ell =1}^{n} \left( \left \vert \begin{aligned} &\sum_{\kappa =1}^{n}(a_{\ell \kappa }- \delta _{\ell }) \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{ \ast }+r_{\kappa }^{\ast } \bigr) \bigr] \\ &\quad {}+\delta _{\ell } \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{ \kappa }^{\ast }+q_{\kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \end{aligned} \right \vert ^{\alpha } \right) ^{\frac{1}{\alpha }} \end{aligned} \right) \\& \quad \precsim \left( \begin{aligned} &\begin{aligned} &\sum _{\ell =1}^{n} \Biggl( \Biggl\vert \sum _{\kappa =1}^{n}(a_{ \ell \kappa }-\delta _{\ell }) \bigl[ (p_{\kappa }+q_{\kappa }+r_{ \kappa })- \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }} \\ &\quad {}+ \Biggl( \Biggl\vert \sum_{\kappa =1}^{n} \delta _{\ell } \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{\kappa }^{\ast }+q_{ \kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }},\end{aligned} \\ &\begin{aligned} &\sum_{\ell =1}^{n} \Biggl( \Biggl\vert \sum_{\kappa =1}^{n}(a_{ \ell \kappa }- \delta _{\ell }) \bigl[ (p_{\kappa }+q_{\kappa }+r_{ \kappa })- \bigl(p_{\kappa }^{\ast }+q_{\kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }} \\ &\quad {}+ \Biggl( \Biggl\vert \sum_{\kappa =1}^{n} \delta _{\ell } \bigl[ (p_{\kappa }+q_{\kappa }+r_{\kappa })- \bigl(p_{\kappa }^{\ast }+q_{ \kappa }^{\ast }+r_{\kappa }^{\ast } \bigr) \bigr] \Biggr\vert ^{\alpha } \Biggr) ^{\frac{1}{\alpha }}\end{aligned}\end{aligned} \right) \\& \quad \precsim \left(\begin{aligned} &\Biggl( \sum_{\ell =1}^{n} \sum_{\kappa =1}^{n}\bigl( \vert a_{\ell \kappa }-\delta _{\ell } \vert \bigr)^{\alpha } \bigl( \bigl\vert p_{\kappa }-p_{\kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{\ast } \bigr\vert + \bigl\vert r_{ \kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr) ^{ \frac{1}{\alpha }}, \\ &\Biggl( \sum_{\ell =1}^{n}\sum _{\kappa =1}^{n}\bigl( \vert a_{\ell \kappa }-\delta _{\ell } \vert \bigr)^{\alpha } \bigl( \bigl\vert p_{\kappa }-p_{\kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{\ast } \bigr\vert + \bigl\vert r_{ \kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr) ^{ \frac{1}{\alpha }}\end{aligned}\right) \\& \quad = \left(\begin{aligned} &\Biggl( \sum_{\kappa =1}^{n} \bigl( \bigl\vert p_{\kappa }-p_{ \kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{ \ast } \bigr\vert + \bigl\vert r_{\kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha }\sum_{\ell =1}^{n} \bigl( \vert a_{\ell \kappa }-\delta _{\ell } \vert \bigr)^{\alpha } \Biggr) ^{\frac{1}{\alpha }}, \\ &\Biggl( \sum_{\kappa =1}^{n} \bigl( \bigl\vert p_{\kappa }-p_{ \kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{ \ast } \bigr\vert + \bigl\vert r_{\kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha }\sum_{\ell =1}^{n}\bigl( \vert a_{\ell \kappa }-\delta _{\ell } \vert \bigr)^{\alpha } \Biggr) ^{\frac{1}{\alpha }}\end{aligned}\right) \\& \quad = (1-\delta ) \left(\begin{aligned} &\Biggl( \sum_{\kappa =1}^{n} \bigl( \bigl\vert p_{\kappa }-p_{ \kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{ \ast } \bigr\vert + \bigl\vert r_{\kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr) ^{\frac{1}{\alpha }}, \\ &\Biggl( \sum_{\kappa =1}^{n} \bigl( \bigl\vert p_{\kappa }-p_{ \kappa }^{\ast } \bigr\vert + \bigl\vert q_{\kappa }-q_{\kappa }^{ \ast } \bigr\vert + \bigl\vert r_{\kappa }-r_{\kappa }^{\ast } \bigr\vert \bigr) ^{\alpha } \Biggr) ^{\frac{1}{\alpha }}\end{aligned}\right) \\& \quad = (1-\delta )\xi \bigl( ( p,q,r ) , \bigl( p^{\ast },q^{ \ast },r^{\ast } \bigr) \bigr) , \end{aligned}$$

which proves that Θ is a contraction. Hence if the mapping $\Im =I_{\chi }$ is the identity mapping in Theorem 2.3, then the Markov process has a unique stationary distribution, and the sequence $\{ \Theta ^{n}\sigma ^{\dagger } \}$ converges to this distribution for any $\sigma ^{\dagger }\in \Omega _{n-1}^{3}$. □