Abstract
We introduce the concept of interval harmonically convex functions. By using two different classes of convexity, we get some further refinements for interval fractional Hermite–Hadamard type inequalities. Also, some examples are presented.
Similar content being viewed by others
1 Introduction
It is well known that convex function and convexity are very important in mathematical economy, probability theory, optimal control theory, and other fields of mathematics. Over the years, classical convexity has been extended and generalized to harmonically convex, h-convex, p-convex, among others. In fact, the concepts of convex function and convexity are founded on inequality, and the important role of inequalities cannot be undermined. Recently, the following Hermite–Hadamard inequality, one of the most important classical inequalities, has gained plenty of attention. Let interval \(J^{\diamond }\subseteq \mathbb{R}\), and \(a,b \in J^{\diamond }\) with \(a< b\). If \(f:J^{\diamond }\rightarrow \mathbb{R}\) is a convex function, then
The following inequality as the weighted generalization of (1.1) was established by Fejér in [1].
Theorem 1.1
Let f be a convex function and \(\psi (a+b-x)=\psi (x)\geq 0\) holds for all \(x\in J^{\diamond }\), then
Due to the difference among the concepts of convexity, integral inequality (1.1) and (1.2) in various forms have also been extensively studied in [2,3,4,5,6,7,8]. With the increasing importance of fractional integrals, several authors extend their research by combining Hermite–Hadamard type inequalities with fractional integrals. In this way, some fractional Hermite–Hadamard type inequalities have been established, see [9,10,11,12,13,14,15] and the references therein.
On the other hand, interval analysis was firstly introduced as a significant tool to handle interval uncertainty by Moore in [16]. It has been widely used in various fields [17,18,19,20]. Especially, several classical inequalities have been studied with interval-valued functions by Chalco-Cano et al. [21, 22], Costa and Román-Flores. [23], Zhao et al. [24, 25], An et al. [26], and so on. As a further extension, Budak et al. [27] proved the fractional Hermite–Hadamard inequality for interval convex function. Motivated by [9,10,11,12, 24, 25, 27], we establish some further refinements for interval fractional Hermite–Hadamard type inequalities. Our results generalize some previous inequalities. In addition, perhaps the results can be recognized as the significant methods to investigate the research of interval-valued differential equations, interval optimization, interval vector spaces, among others.
We give some preliminaries in Sect. 2. In Sect. 3, we introduce the concept of interval harmonically convex functions and prove some interval fractional Hermite–Hadamard type inequalities. Finally, in Sect. 4, some examples are presented.
2 Preliminaries
We begin by using \(\mathcal{K}\) denote the space of all intervals of \(\mathbb{R}\). Let \(D\in \mathcal{K}\),
When \(\underline{d}=\overline{d}\), the interval D is said to be degenerate. We call D positive if \(\underline{d}>0\) or negative if \(\overline{d}<0\). We use \(\mathcal{K}^{+}\) and \(\mathcal{K}^{-}\) to represent the sets of all positive intervals and negative intervals. Let \(\lambda \in \mathbb{R}\), then
For \(D_{1},D_{2}\in \mathcal{K}\), the addition and Minkowski difference are defined by
and
respectively.
The inclusion “⊆” is defined by
For more basic notations with interval analysis, see [24, 25]. Furthermore, we recall the following results in [20].
Let \(\mathcal{F}(x)=[\underline{f}(x),\overline{f}(x)]\), \(x\in J^{ \diamond }\). We call \(\mathcal{F}(x)\) is Lebesgue integrable if \(\underline{f}(x)\) and \(\overline{f}(x)\) are measurable and Lebesgue integrable in \(J^{\diamond }\). Moreover, we define \(\int _{a}^{b}\mathcal{F}(x)\,dx\) as follows:
Let \(\mathscr{IL}_{([a,b])}\) be the collections of all Lebesgue integrable interval-valued functions on \([a,b]\). If \(\mathcal{F} \in \mathscr{IL}_{([a,b])}\), the interval left-sided Riemann–Liouville fractional integral of \(\mathcal{F}(x)\) is defined by
where \(\varGamma (\alpha )\) is the Gamma function defined by \(\varGamma ( \alpha )= \int _{0}^{\infty }e^{-x}x^{\alpha -1}\,dx\) with \(\alpha >0\).
In [27], the interval right-sided Riemann–Liouville fractional integral of \(\mathcal{F}(x)\) is defined by
where \(\varGamma (\alpha )\) is an Euler Gamma function.
It is obvious that \(\mathfrak{J}^{\alpha }_{a^{+}}\mathcal{F}(x)= [ {J}^{\alpha }_{a^{+}}\underline{f}(x),{J}^{\alpha }_{a^{+}} \overline{f}(x)],\mathfrak{J}^{\alpha }_{b^{-}}\mathcal{F}(x)= [{J} ^{\alpha }_{b^{-}}\underline{f}(x),{J}^{\alpha }_{b^{-}}\overline{f}(x)]\), for all \(x\in J^{\diamond }\).
Definition 2.1
([6])
\(f:J^{\diamond }\subseteq \mathbb{R}\backslash \{0\} \rightarrow \mathbb{R}\) is called a harmonically convex function if
holds for any \(x,y\in J^{\diamond } \) and \(\mathfrak{t} \in [0,1]\).
Definition 2.2
([28])
\(\mathcal{F}:J^{\diamond }\rightarrow \mathcal{K}^{+}\) is called an interval convex function if
holds for any \(x,y\in J^{\diamond } \) and \(\mathfrak{t} \in [0,1]\).
3 Main result
First, we give definition of interval harmonically convex functions as follows.
Definition 3.1
\(\mathcal{F}:J^{\diamond }\subseteq \mathbb{R}\backslash \{0\}\rightarrow \mathcal{K}^{+}\) is called an interval harmonically convex function if
holds for all \(x,y\in J^{\diamond } \) and \(\mathfrak{t} \in [0,1]\).
Let \(\mathcal{FC}{(J^{\diamond },\mathcal{K}^{+})}\) and \(\mathcal{FHC}{(J^{\diamond },\mathcal{K}^{+})}\) denote the family of interval convex and harmonically convex functions in \(J^{\diamond }\), respectively.
In [27], Budak et al. give the following Hermite–Hadamard inequality for the interval convex function.
Theorem 3.2
Let \(\mathcal{F}\in \mathscr{IL}_{([a,b])}\) and \(a,b \in J^{\diamond }\) with \(0\leq a< b\). If \(\mathcal{F}\in \mathcal{FC}{(J^{\diamond }, \mathcal{K}^{+})}\), then
Remark 3.3
In Theorem 3.2, if \(\underline{f}=\overline{f}\), then we get ([12], Theorem 2).
Next, we give some further refinements for interval fractional Hermite–Hadamard type inequalities.
Theorem 3.4
Let \(\mathcal{F}\in \mathscr{IL}_{([a,b])}\), and \(a,b \in J^{\diamond }\) with \(0\leq a< b\). If \(\mathcal{F}\in \mathcal{FC}(J^{\diamond }, \mathcal{K}^{+})\) and \(\psi (a+b-x)=\psi (x)\geq 0 \) holds for all \(x\in J^{\diamond }\), then
Proof
Since \(\mathcal{F}\in \mathcal{FC}( J^{\diamond },\mathcal{K}^{+})\), we have
with \(\nu =1-\mu \), \(\mu \in [0,1]\).
Multiplying both sides of (3.3) by \(2\mu ^{\alpha -1}\psi (\mu b+ \nu a)\), then
Consequently,
Setting \(\omega =\mu b+\nu a\), then
Therefore
Since \(\mathcal{F}\in \mathcal{FC}([a,b],\mathcal{K}^{+})\), we have
and
with \(\nu =1-\mu \), \(\mu \in [0,1]\). Then
By multiplying both sides (3.5) with \(\mu ^{\alpha -1}\psi (\mu b+ \nu a)\), and integrating the resulting inequality, we get
and the result follows. □
Remark 3.5
In Theorem 3.4, if \(\psi (x)=1\), inequality (3.2) becomes inequality (3.1) in Theorem 3.2.
If \(\underline{f}=\overline{f}\), then we get ([9], Theorem 4).
Theorem 3.6
Let \(\mathcal{F}\in \mathscr{IL}_{([a,b])}\), and \(a,b \in J^{\diamond }\) with \(0\leq a< b\). If \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond }, \mathcal{K}^{+})\), and \(g(x)=\frac{1}{x},x\in [\frac{1}{b}, \frac{1}{a}]\) then
Proof
Since \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond },\mathcal{K}^{+})\), we have
Let \(\nu =1-\mu \), \(\mu \in [0,1]\), setting
By multiplying both sides with \(\mu ^{\alpha -1}\), and integrating the resulting inequality, we get
Let \(\nu =1-\mu \), \(\mu \in [0,1]\), then thanks to \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond },\mathcal{K}^{+})\)
and
This implies
Then
Therefore
with \(g(x)=\frac{1}{x}\).
This gives the result. □
Remark 3.7
The function \(\mathcal{F}(x)\in \mathcal{FHC}(J^{\diamond }, \mathcal{K}^{+})\) if and only if \(\mathcal{H}(x)=\mathcal{F}( \frac{ab}{x})\in \mathcal{FC}(J^{\diamond },\mathcal{K}^{+})\). By using inequality (3.1) for \(\mathcal{H}(x)\), we obtain inequality (3.7).
Remark 3.8
In Theorem 3.6, if \(\underline{f}=\overline{f}\), then we get ([10], Theorem 4).
Theorem 3.9
Let \(\mathcal{F}\in \mathscr{IL}_{([a,b])}\), and \(a,b \in J^{\diamond }\) with \(0\leq a< b\). If \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond }, \mathcal{K}^{+})\) and \(\psi (\frac{1}{\frac{1}{a}+\frac{1}{b}- \frac{1}{x}} )=\psi (x)\geq 0\) holds for all \(x\in J^{\diamond }\), then
with \(g(x)=\frac{1}{x},x\in [\frac{1}{b},\frac{1}{a}]\).
Proof
Since \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond },\mathcal{K}^{+})\), we have
with \(\nu =1-\mu , \mu \in [0,1]\).
Multiplying both sides of (3.10) by \(2\mu ^{\alpha -1}\psi (\frac{ab}{ \mu b+\nu a})\), we get
Let \(\omega =\frac{\mu b+\nu a}{ab}\), then \(d\mu =\frac{ab}{b-a}\,d \omega \). One has
This implies that
Similarly, \(\mathcal{F}\in \mathcal{FHC}(J^{\diamond },\mathcal{K} ^{+})\), then
Multiplying both sides of (3.12) by \(\mu ^{\alpha -1}\psi (\frac{ab}{ \mu b+\nu a})\), one has
Hence,
and the result follows. □
Remark 3.10
If \(\underline{f}=\overline{f}\), then we get ([11], Theorem 5).
If \(\psi (x)=1\), inequality (3.9) reduces to inequality (3.7) in Theorem 3.6.
4 Examples
Example 4.1
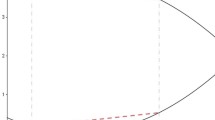
Let \(\mathcal{F}(x)= [-\sqrt{x}+2,\sqrt{x}+2 ]\), \(x \in [0,2]\), and \(\alpha =\frac{1}{2}\). Then \(\mathcal{F}\in \mathcal{FC}([0,2],\mathcal{K}^{+})\), and we have
On the other hand,
and
Thus,
Consequently, Theorem 3.2 is verified.
Example 4.2
Let \(\mathcal{F}:[0,2]\rightarrow \mathcal{K}\) is defined as the above example, and
then \(\psi (2-x)=\psi (x)\geq 0\) for all \(x\in [0,2]\). Let \(\alpha = \frac{1}{2}\), we obtain
Furthermore, by Example 4.1, we have
Consequently, Theorem 3.4 is verified.
5 Conclusions
In this research, we get a new extension of interval harmonically convex functions and some further refinements for interval fractional Hermite–Hadamard type inequalities. The results obtained in this work are the promotions of those given in previous research. Moreover, our results can be recognized as significant methods in the fields of mathematics. At a further research direction, we will investigate the integral inequalities with a new class of fractional integral.
References
Fejér, L.: Uberdie Fourierreihen, II. Math. Naturwise. Anz Ungar. Akad. Wiss. 24, 369–390 (1906)
Bombardelli, M., Varošanec, S.: Properties of h-convex functions related to the Hermite–Hadamard–Fejér inequalities. Comput. Math. Appl. 58, 1869–1877 (2009)
İşcan, İ.: Some new Hermite–Hadamard type inequalities for s-geometrically convex functions and their applications. Contemp. Anal. Appl. Math. 2, 230–241 (2014)
Noor, M.A., Noor, K.I., Awan, M.U., Li, J.: On Hermite–Hadamard inequalities for h-preinvex functions. Filomat 24, 1463–1474 (2014)
Latif, M.A., Alomari, M.: On Hadmard-type inequalities for h-convex functions on the co-ordinates. Int. J. Math. Anal. 3, 1645–1656 (2009)
İşcan, İ.: Hermite–Hadamard type inequalities for harmonically convex functions. Hacet. J. Math. Stat. 43, 935–942 (2014)
Tseng, K.L., Yang, G.S., Hsu, K.C.: Some inequalities for differentiable mappings and applications to Fejér inequality and weighted trapezoidal formula. Taiwan. J. Math. 15, 1737–1747 (2011)
Dragomir, S.S.: Inequalities of Hermite–Hadamard type for h-convex functions on linear spaces. Proyecciones 34, 323–341 (2015)
İşcan, İ.: Hermite–Hadamard–Fejér type inequalities for convex functions via fractional integrals. Stud. Univ. Babeş–Bolyai, Math. 60, 355–366 (2015)
İşcan, İ., Wu, S.: Hermite–Hadamard type inequalities for harmonically convex functions via fractional integrals. Appl. Math. Comput. 238, 237–244 (2014)
İşcan, İ., Kunt, M., Yazici, N.: Hermite–Hadamard–Fejér type inequalities for harmonically convex functions via fractional integrals. New Trends Math. Sci. 4, 239–253 (2016)
Sarikaya, M.Z., Set, E., Yaldiz, H., Başak, N.: Hermite–Hadamard’s inequalities for fractional integrals and related fractional inequalities. Math. Comput. Model. 57, 2403–2407 (2013)
İşcan, İ.: Generalization of different type integral inequalities for s-convex functions via fractional integrals. Appl. Anal. 93, 1846–1862 (2014)
Noor, M.A., Cristescu, G., Awan, M.U.: Generalized fractional Hermite–Hadamard inequalities for twice differentiable s-convex functions. Filomat 29, 807–815 (2015)
Wang, J.R., Li, X.Z., Fečkan, M., Zhou, Y.: Hermite–Hadamard-type inequalities for Riemann–Liouville fractional integrals via two kinds of convexity. Appl. Anal. 92, 2241–2253 (2013)
Moore, R.E.: Interval Analysis. Prentice-Hall, Englewood Cliffs (1966)
Chalco-Cano, Y., Rufián-Lizana, A., Román-Flores, H., Jiménez-Gamero, M.D.: Calculus for interval-valued functions using generalized Hukuhara derivative and applications. Fuzzy Sets Syst. 219, 49–67 (2013)
Costa, T.M., Chalco-Cano, Y., Lodwick, W.A., Silva, G.N.: Generalized interval vector spaces and interval optimization. Inf. Sci. 311, 74–85 (2015)
Osuna-Gómez, R., Chalco-Cano, Y., Hernández-Jiménez, B., Ruiz-Garzón, G.: Optimality conditions for generalized differentiable interval-valued functions. Inf. Sci. 321, 136–146 (2015)
Lupulescu, V.: Fractional calculus for interval-valued functions. Fuzzy Sets Syst. 265, 63–85 (2015)
Chalco-Cano, Y., Flores-Franulič, A., Román-Flores, H.: Ostrowski type inequalities for interval-valued functions using generalized Hukuhara derivative. Comput. Appl. Math. 31, 457–472 (2012)
Chalco-Cano, Y., Lodwick, W.A., Condori-Equice, W.: Ostrowski type inequalities and applications in numerical integration for interval-valued functions. Soft Comput. 19, 3293–3300 (2015)
Costa, T.M., Román-Flores, H.: Some integral inequalities for fuzzy-interval-valued functions. Inf. Sci. 420, 110–125 (2017)
Zhao, D.F., An, T.Q., Ye, G.J., Liu, W.: New Jensen and Hermite–Hadamard type inequalities for h-convex interval-valued functions. J. Inequal. Appl. 2018, 302 (2018)
Zhao, D.F., Ye, G.J., Liu, W., Torres, M.: Some inequalities for interval-valued functions on time scales. Soft Comput. (2018). https://doi.org/10.1007/s00500-018-3538-6
An, Y.R., Ye, G.J., Zhao, D.F., Liu, W.: Hermite–Hadamard type inequalities for interval \((h_{1},h_{2})\)-convex functions (2019). https://doi.org/10.3390/math7050436
Budak, H., Tunç, T., Sarikaya, M.Z.: Fractional Hermite–Hadamard type inequalities for interval-valued functions. Proc. Am. Math. Soc. (2019). https://doi.org/10.1090/proc/14741
Costa, T.M.: Jensen’s inequality type integral for fuzzy-interval-valued functions. Fuzzy Sets Syst. 327, 31–47 (2017)
Acknowledgements
The authors are very grateful to the anonymous referees, for several valuable and helpful comments, suggestions and questions, which helped them to improve the paper into present form.
Availability of data and materials
Not applicable.
Funding
The work is supported by the Fundamental Research Funds for the Central Universities (2017B19714, 2017B07414 and 2019B44914), Special Soft Science Research Projects of Technological Innovation in Hubei Province (2019ADC146), Natural Science Foundation of Jiangsu Province (BK20180500) and the National Key Research and Development Program of China (2018YFC1508106).
Author information
Authors and Affiliations
Contributions
Each of the authors contributed to each part of this study equally, all authors read and approved the final manuscript.
Corresponding author
Ethics declarations
Competing interests
The authors declare that they have no competing interests.
Additional information
Abbreviations
Not applicable.
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Liu, X., Ye, G., Zhao, D. et al. Fractional Hermite–Hadamard type inequalities for interval-valued functions. J Inequal Appl 2019, 266 (2019). https://doi.org/10.1186/s13660-019-2217-1
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13660-019-2217-1