The Effect of Movement Behavior on Population Density in Patchy Landscapes

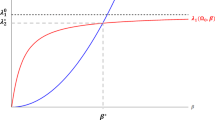
Abstract

Many biological populations reside in increasingly fragmented landscapes, where habitat quality may change abruptly in space. Individuals adjust their movement behavior to local habitat quality and show preferences for some habitat types over others. Several recent publications explore how such individual movement behavior affects population-level dynamics in a framework of reaction–diffusion systems that are coupled through discontinuous boundary conditions. While most of those works are based on linear analysis, we study positive steady states of the nonlinear equations. We prove existence, uniqueness and global stability, and we classify their qualitative shape depending on movement behavior. We apply our results to study the question why and under which conditions the total population abundance at steady state may exceed the total carrying capacity of the landscape.

Appendix

In this appendix, we provide the Proof of Lemma 5 and a discussion for why we cannot expect a similar result to hold when we choose the ecological (forward Kolmogorov) diffusion term. The proof is a slight adaptation from Lou (2006). We assume that u(x) is a positive solution of the steady-state equation with Fickian diffusion term and no-flux boundary condition:

$$\begin{aligned} {\left\{ \begin{array}{ll} \dfrac{\mathrm {d}}{\mathrm {d} x}\left( D(x) \dfrac{\mathrm {d} u(x)}{\mathrm {d} x}\right) +u(x)\left( K(x)-u(x)\right) =0,\,&{} x\in [0,L]; \\ \\ D(0)\dfrac{\mathrm {d} u(0)}{\mathrm {d} x}=0,\quad D(L)\dfrac{\mathrm {d} u(L)}{\mathrm {d} x}=0. \end{array}\right. } \end{aligned}$$

We assume that all functions are sufficiently smooth and positive.

First, we divide both sides of the equation by u(x) and integrate by parts over the interval [0, L]. After applying the boundary conditions, we obtain:

$$\begin{aligned} \int _{0}^{L} \left( u(x)-K(x)\right) \mathrm {d}x= & {} \int _{0}^{L} \dfrac{1}{u(x)} \left( \dfrac{\mathrm {d}}{\mathrm {d} x}\left( D(x) \dfrac{\mathrm {d} u(x)}{\mathrm {d} x}\right) \right) \,\mathrm {d}x\\= & {} \int _{0}^{L} D(x) \left( \dfrac{1}{u(x)}\,\dfrac{\mathrm {d} u(x)}{\mathrm {d}x} \right) ^{2}\mathrm {d}x. \end{aligned}$$

Thus, the integral difference is positive, as claimed in the lemma.

Now, we consider the same equation, but with the Fickian diffusion term replaced by the ecological diffusion (or forward Kolmogorov) term (Turchin 1998):

$$\begin{aligned} {\left\{ \begin{array}{ll} \dfrac{\mathrm {d^2}}{\mathrm {d} x^2}\left( D(x)u(x)\right) +u(x)\left( K(x)-u(x)\right) =0,\,&{} x\in [0,L]; \\ \\ \dfrac{\mathrm {d} }{\mathrm {d} x}(D(x)u(x))|_{x=0}=0, \quad \dfrac{\mathrm {d} }{\mathrm {d} x}(D(x)u(x))|_{x=L}=0.\\ \end{array}\right. } \end{aligned}$$

We follow the same steps as above to find

$$\begin{aligned}&\int _{0}^{L} \left( u(x)-K(x)\right) \mathrm {d}x = \int _{0}^{L} \dfrac{1}{u(x)}\dfrac{\mathrm {d^2}}{\mathrm {d} x^2}\left( D(x)u(x)\right) {\mathrm {d}}x\\&\quad =\int _{0}^{L} \dfrac{\mathrm {d}}{\mathrm {d} x}D(x) \left( \dfrac{\mathrm {d}}{\mathrm {d} x}\ln (u(x))\right) \,\mathrm {d}x + \int _{0}^{L} D(x) \left( \dfrac{1}{u(x)}\,\dfrac{\mathrm {d} u(x)}{\mathrm {d}x} \right) ^{2}\mathrm {d}x. \end{aligned}$$

We see that the right-hand side is not necessarily positive. Instead, some conditions on slope or curvature need to be satisfied for positivity. Since the shape of u depends on the shape of K and D, it is not obvious what these conditions are.