Analytical Solution and Exposure Analysis of a Pharmacokinetic Model with Simultaneous Elimination Pathways and Endogenous Production: The Case of Multiple Dosing Administration

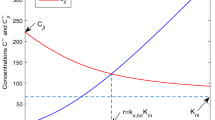
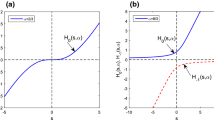
Abstract

In this paper, a typical pharmacokinetic (PK) model is studied for the case of multiple intravenous bolus-dose administration. This model, of one-compartment structure, not only exhibits simultaneous first-order and Michaelis–Menten elimination, but also involves a constant endogenous production. For the PK characterization of the model, we have established the closed-form solution of concentrations over time, the existence and local stability of the steady state. Using analytical approaches and the concept of corrected concentration, we have shown that the area under the curve ($\hbox {AUC}^{corr}_{ss,\tau }$) at steady state is higher compared to that at the single dose ($\hbox {AUC}^{corr}_{0-\infty }$). Moreover, by splitting the dose and dosing interval into halves, we have revealed that it can result in a significant decrease in the steady-state average concentration. These model-based findings, which contrast with the current knowledge for linear PK, confirm the necessity to revisit drugs exhibiting nonlinear PK and to suggest a rational way of using mathematical analysis for the dosing regimen design.

Appendices

Appendix A: Introduction of X Function and Unique Real Branch in the First Quadrant in $R^2$

The aim of introduction of X function is to express the closed-form solution of a one-compartment PK open model exhibiting simultaneous first-order and Michaelis–Menten elimination (Wu et al. 2015). Specifically, the model is described as

$$\begin{aligned} \left\{ \begin{array}{ll} \frac{\mathrm{{d}}C(t)}{\mathrm{{d}}t}&{}=-k_\mathrm{el}C(t)-\frac{V_\mathrm{max}C(t)}{V_\mathrm{{d}}(K_\mathrm{{m}}+C(t))},\,\,t>0\\ C(0^+)&{}=D/V_\mathrm{{d}}, \end{array} \right. \end{aligned}$$

(A.1)

where D is an intravenous bolus dose, $k_\mathrm{el}$ is the rate constant of the first-order elimination, $V_\mathrm{max}$ and $K_\mathrm{{m}}$ are the corresponding parameters of Michaelis–Menten elimination representing the maximum velocity of its kinetics and the concentration value at which 50% of $V_\mathrm{max}$ is reached, and $V_\mathrm{{d}}$ is the apparent volume of distribution.

Integrating Eq. A.1 gives rise to the following algebraic equation

$$\begin{aligned} \left( \frac{C(t)}{C_{\beta }}\right) ^{p_1}\left( \frac{C(t)}{C_{\beta }}+1\right) ^{p_2}=\left( \frac{C_0}{C_{\beta }}\right) ^{p_1}\left( \frac{C_0}{C_{\beta }}+1\right) ^{p_2} \mathrm{{e}}^{-t} \end{aligned}$$

(A.2)

where

$$\begin{aligned} C_{\beta }=K_\mathrm{{m}}+\frac{V_\mathrm{max}}{k_\mathrm{el}V_\mathrm{{d}}}>K_\mathrm{{m}},\,\,p_1=\frac{1}{k_\mathrm{el}}\frac{K_\mathrm{{m}}}{C_{\beta }}>0,\,\,p_2=\frac{1}{k_\mathrm{el}}\frac{C_{\beta }-K_\mathrm{{m}}}{C_{\beta }}>0. \end{aligned}$$

Using the definition of X function (see Definition 1), we are able to express C(t) as

$$\begin{aligned} C(t)=C_{\beta }\cdot X\left( \left( \frac{D/V_\mathrm{{d}}}{C_{\beta }}\right) ^{p_1} \left( \frac{D/V_\mathrm{{d}}}{C_{\beta }}+1\right) ^{p_2}\mathrm{{e}}^{-t},p_1,p_2\right) , \end{aligned}$$

(A.3)

For more details, refer to Wu et al. (2015).

Next, we show the proof of unique real branch of X function in the first quadrant in ${\mathbb {R}}^2$, where $p>0$ and $q>0$.

From the definition of X function, we denote

$$\begin{aligned} F(z, X)=(X(z,p,q))^p(X(z,p,q)+1)^q-z. \end{aligned}$$

(A.4)

In the scope of real number in the first quadrant in ${\mathbb {R}}^2$, namely for each $z, X>0$, we can have

$$\begin{aligned} F_z(z,X)=-1,\quad \quad F_X(z,X)=z\left( \frac{p}{X}+\frac{q}{X+1}\right) \ne 0. \end{aligned}$$

By implicit function theorem, there is a unique real solution $X(z,p,q)>0$ such that the equation $X(z,p,q)^p(X(z,p,q)+1)^q=z$ is valid. Moreover, the derivative of X(z, p, q) with respect to z is given by

$$\begin{aligned} \frac{\mathrm{{d}}X(z,p,q)}{\mathrm{{d}}z}=-\frac{F_z(x,X)}{F_X(z,X)}=\frac{1}{z}\left( \frac{p}{X}+\frac{q}{X+1}\right) ^{-1}>0, \end{aligned}$$

which implies the function X(z, p, q) is increasing with the variable z in the first quadrant.

Appendix B: Proof of the Relationship of Eq. 35

To prove Eq. 35, we define a continuous function as

$$\begin{aligned}&h(x){\mathop {=}\limits ^{def}}\left( 1+\frac{\tfrac{1}{2}D/V_\mathrm{{d}}}{C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-x}\right) ^2 \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x}\right) ^{-1},\nonumber \\&\quad x\in \left[ 0, C_{\beta }^{en}+C_\mathrm{hs}\right] , \end{aligned}$$

(C.1)

where $C_{\beta }^{en}$ and $C_\mathrm{hs}$ are concentrations defined in Sect. 2.3, D is dose amount, $V_\mathrm{{d}}$ is the apparent volume of distribution, $C^{tr}_{ss}(D,\tau )$ and $C^{tr}_{ss}(\tfrac{D}{2},\tfrac{\tau }{2})$ are respective steady- state trough concentrations which are greater than $C_\mathrm{hs}$.

Taking derivative of h(x) with respect to the variable x yields

$$\begin{aligned} h'(x)=\frac{D}{V_\mathrm{{d}}} \left( 1+\frac{\tfrac{1}{2}D/V_\mathrm{{d}}}{C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-x}\right) \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x}\right) ^{-2}\cdot g,\nonumber \\ \end{aligned}$$

(C.2)

where $g=g_1-g_2$ with

$$\begin{aligned} g_1=\frac{1}{\big (C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-x\big )^2} \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x}\right) \end{aligned}$$

and

$$\begin{aligned} g_2=\frac{1}{\big (C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x\big )^2} \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-x}\right) . \end{aligned}$$

Considering the right hand of $h'(x)$, the left portion before g is positive; thus, the sign of $h'(x)$ depends on that of g alone. Furthermore, g can be rewritten as

$$\begin{aligned} g=\frac{1}{\big (C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-x\big )^2(C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x)^2}(w_1-w_2) \end{aligned}$$

with

$$\begin{aligned} w_1=\left( \tfrac{D}{V_\mathrm{{d}}}+C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x\right) \left( C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-x\right) \end{aligned}$$

and

$$\begin{aligned} w_2=\left( \tfrac{D}{V_\mathrm{{d}}}+C^{tr}_{ss}(\tfrac{D}{2},\tfrac{\tau }{2})+C_{\beta }^{en}-x\right) \left( C^{tr}_{ss}(\tfrac{D}{2},\tfrac{\tau }{2})+C_{\beta }^{en}-x\right) . \end{aligned}$$

Since $C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})>C^{tr}_{ss}(D,\tau )>C_\mathrm{hs}$ and $x\in [0,C_{\beta }^{en}+C_\mathrm{hs}]$, we have $0<w_1<w_2$, and thus, g is negative. Therefore, h(x) is a decreasing function with respect to its variable $x\in [0,C_{\beta }^{en}+C_\mathrm{hs}]$. Subsequently, we have

$$\begin{aligned} \begin{aligned} h(C_{\beta }^{en}+C_\mathrm{hs})&=\left( 1+\frac{\tfrac{1}{2}D/V_\mathrm{{d}}}{C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})+C_{\beta }^{en}-(C_{\beta }^{en}+C_\mathrm{hs})}\right) ^2\\&\quad \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(D,\tau )+C_{\beta }^{en}-(C_{\beta }^{en}+C_\mathrm{hs})}\right) ^{-1}\\&=\left( 1+\frac{\tfrac{1}{2}D/V_\mathrm{{d}}}{C^{tr}_{ss}(\frac{D}{2},\frac{\tau }{2})-C_\mathrm{hs}}\right) ^2 \left( 1+\frac{D/V_\mathrm{{d}}}{C^{tr}_{ss}(D,\tau )-C_\mathrm{hs}}\right) ^{-1}\\&<h(0)=\left( 1+\tfrac{1}{2}A\right) ^2 \left( 1+B\right) ^{-1}. \end{aligned} \end{aligned}$$