Skip to main content
SpringerLink
Log in

The time-fractional diffusion inverse problem subject to an extra measurement by a local discontinuous Galerkin method

  • Published:
BIT Numerical Mathematics Aims and scope Submit manuscript

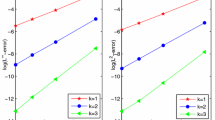
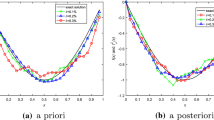
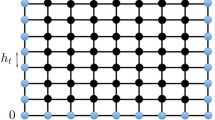
Abstract

This paper deals with an inverse problem of identifying a space dependent coefficient in a time-fractional diffusion equation on a finite domain with final observation. The existence and uniqueness of this inverse problem are proved. A numerical scheme is proposed to solve the problem. The main idea of the proposed scheme is approximating the time fractional derivative by Diethelm’s quadrature formula and use the local discontinuous Galerkin method in space variable. Also, an error estimate for this problem is presented. Finally, two numerical example is studied to demonstrate the accuracy and efficiency of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Al-Refai, M., Luchko, Y.: Maximum principles for the fractional diffusion equations with the Riemann–Liouville fractional derivative and their applications. Fract. Calc. Appl. Anal. 17, 483–498 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  2. Arnold, D.N., Brezzi, F., Cockburn, B., Marini, D.: Discontinuous Galerkin methods for elliptic problems. In: Discontinuous Galerkin Methods: Theory, Computation and Applications, vol. 11, pp. 89–101 (2000)

  3. Asadzadeh, M., Schatz, A., Wendland, W.: Asymptotic error expansions for the finite element method for second order elliptic problems in \({ R} _N, N>=2\), I: local interior expansions. SIAM J. Numer. Anal. 48, 2000–2017 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  4. Asadzadeh, M., Beilina, L.: A posteriori error analysis in a globally convergent numerical method for a hyperbolic coefficient inverse. Inverse Probl. 26, 115–127 (2010)

    Article  MATH  Google Scholar 

  5. Bagley, R., Torvik, P.: A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 27, 201–210 (1983)

    Article  MATH  Google Scholar 

  6. Bassi, F., Rebay, S.: A high-order accurate discontinuous finite element method for the numerical solution of the compressible Navier–Stokes equations. J. Comput. Phys. 131, 267–279 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  7. Brunner, H., Han, H., Yin, D.: The maximum principle for time-fractional diffusion equations and its application. Numer. Func. Anal. Optim. 36, 1307–1321 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  8. Cannon, J.R., Yin, H.-M.: A class of nonlinear non-classical parabolic equations. J. Differ. Equ. 79, 266–288 (1989)

    Article  MATH  Google Scholar 

  9. Cannon, J.R., Yin, H.-M.: Numerical solutions of some parabolic inverse problems. Numer. Methods Partial Differ. Equ. 6, 177–191 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  10. Castillo, P.: An optimal estimate for the local discontinuous Galerkin method. In: Discontinuous Galerkin Methods: Theory, Computation and Applications. Lecture Notes in Computational Science and Engineering, vol. 11, pp. 285–290 (2000)

  11. Chen, Q., Liu, J.J.: Solving an inverse parabolic problem by optimization from final measurement data. J. Comput. Appl. Math. 193, 183–203 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  12. Cheng, J., Nakagawa, J., Yamamoto, M., Yamazaki, T.: Uniqueness in an inverse problem for a one-dimensional fractional diffusion equation. Inverse Probl. 25(11), 551–562 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  13. Cockburn, B., Shu, C.W.: The local discontinuous Galerkin method for time dependent converction-diffusion systems. SIAM J. Numer. Anal. 35, 2440–246 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  14. Cockburn, B., Kanschat, G., Perugia, I., Schötzau, D.: Superconvergence of the local discontinuous Galerkin method for elliptic problems on cartesian grids. SIAM J. Numer. Anal. 39(1), 264–285 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  15. Deng, W.H., Hesthaven, J.S.: Discontinuous Galerkin methods for fractional diffusion equations. ESAIM 47, 1845–1864 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  16. Diethelm, K.: Numerical Approximation for Finite-Part Integrals with Generalized Compound Quadrature Formulae. Hildesheimer Informatikberichte (1995)

  17. Diethelm, K.: An algorithm for the numerical solution of differential equations of fractional order. Electron. Trans. Numer. Anal. 5, 1–6 (1997)

    MathSciNet  MATH  Google Scholar 

  18. Douglas, J., Dupont, J.T.: Interior penalty procedures for elliptic and parabolic Galerkin methods. Lecture Notes in Physics, vol. 58. Springer, Berlin (1976)

  19. Furati, KhM, Iyiola, O., Kirane, M.: An inverse problem for a generalized fractional diffusion. Appl. Math. Comput. 249, 24–31 (2014)

    MathSciNet  MATH  Google Scholar 

  20. Georgoulis, E.H.: hp-version interior penalty discontinuous Galerkin finite element methods on anisotropic meshes. Int. J. Numer. Anal. Model. 3, 52–79 (2006)

    MathSciNet  MATH  Google Scholar 

  21. Guo, B., Pu, X., Huang, F.: Fractional Partial Differential Equations and Their Numerical Solutions. World Scientific, Singapore (2015)

    Book  MATH  Google Scholar 

  22. Hesthaven, J.S., Warburton, T.: Nodal Discontinuous Galerkin Methods Algorithms, Analysis, and Applications. Springer, Berlin (2007). https://doi.org/10.1007/978-0-387-72067-8

    MATH  Google Scholar 

  23. Ismailov, M.I., Çiçek, M.: Inverse Source Problem for a time-fractional diffusion equation with nonlocal boundary conditions. Appl. Math. Model. 40, 4891–4899 (2016)

    Article  MathSciNet  Google Scholar 

  24. Jin, B., Rundell, W.: An inverse problem for a one-dimensional time-fractional diffusion problem. Inverse Probl. 28(7), 234–245 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  25. Larsson, S., Racheva, M., Saedpanah, F.: Discontinuous Galerkin method for an integro-differential equation modeling dynamic fractional order viscoelasticity. Comput. Methods Appl. Mech. Eng. 283, 196–209 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  26. Li, X.J., Xu, C.J.: Existence and uniqeness of the weak solution of the space–time fractional diffusion equation and a spectral method approximation. Commun. Comput. Phys. 8, 1016–1051 (2010)

    MathSciNet  MATH  Google Scholar 

  27. Liu, L.L., Yamamoto, M.: A backward problem for the time-fractional diffusion equation. Appl. Anal. 89, 1769–1788 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  28. Liu, L.L., Yamamoto, M., Yan, L.: On the uniqueness and reconstruction for an inverse problem of the fractional diffusion process. Appl. Numer. Math. 87, 1–19 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Luchko, Y.: Maximum principle for the generalized time-fractional diffusion equation. J. Math. Anal. Appl. 351, 218–223 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  30. Metzler, R., Klafter, J.: The restaurant at the end of the random walk: recent developments in the description of anomalous transport by fractional dynamics. J. Phys. A Math. Gen. 37, 161–208 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  31. Metzler, R., Klafter, J.: The random walks guide to an omalous diffusion: a fractional dynamics approach. Phys. Rep. 339, 1–77 (2000)

    Article  MATH  Google Scholar 

  32. Murio, D.A.: Implicit finite difference approximation for time fractional diffusion equations. Comput. Math. Appl. 56, 1138–1145 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  33. Murio, D.A.: Time fractional IHCP with Caputo fractional derivatives. Comput. Math. Appl. 56, 2371–2381 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  34. Oldham, K.B., Spanier, J.: The Fractional Calculus: Theory and Application of Differentiation and Integration to Arbitrary Order. Academic Press, New York (1974)

    MATH  Google Scholar 

  35. Prilepko, A.I., Orlovsky, D.G., Vasian, I.A.: Methods for Solving Inverse Problems in Mathematical Physics. Marcel Dekker Inc., New York (2000)

    Google Scholar 

  36. Podlubny, I.: Fractiorlal Differential Eqnations. Academic Press, New York (1999)

    Google Scholar 

  37. Rostamy, D., Abdollahi, N.: Gegenbauer cardinal functions for the inverse source parabolic problem with a time-fractional diffusion equation. J. Comput. Theor. Transp. 46, 307–329 (2017)

    Article  MathSciNet  Google Scholar 

  38. Rostamy, D.: The new streamline diffusion for the 3D coupled Schrodinger equations with a cross-phase modulation. ANZIAM J. 55, 51–78 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  39. Rostamy, D., Qasemi, S.: Discontinuous Petrov-Galerkin and Bernstein-Legendre polynomials method for solving fractional damped heat- and wave-like equations. Transp. Theory Stat. Phys. 44, 1–23 (2015)

    MathSciNet  Google Scholar 

  40. Scalas, E., Gorenflo, R., Mainardi, F.: Fractional calculus and continuous-time finance. Phys. A Stat. Mech. Appl. 284, 376–84 (2000)

    Article  MathSciNet  Google Scholar 

  41. Shao, L., Feng, X., He, Y.: The local discontinuous Galerkin finite element method for Burgers equation. Math. Comput. Model. 54, 2943–2954 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  42. Tarasov, V.E.: Fractional vector calculus and fractional Maxwells equations. Ann. Phys. 323, 2756–2778 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  43. Tatar, S., Ulusoy, S.: An inverse source problem for a one-dimensional space-time fractional diffusion equation. Appl. Anal. 94, 2233–2244 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  44. Tuan, V.K.: Inverse problem for fractional diffusion equation. Fract. Calc. Appl. Anal. 14, 31–55 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  45. Wei, T., Zhang, Z.Q.: Reconstruction of a time- dependent source term in a time-fractional diffusion equation. Eng. Anal. Bound. Elem. 37, 23–31 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  46. Wei, T., Wang, J.: A modified quasi-boundary value method for an inverse source problem of the time-fractional diffusion equation. Appl. Numer. Math. 78, 95–111 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  47. Wei, T., Li, X.L., Li, Y.S.: An inverse time-dependent source problem for a time-fractional diffusion equation. Ineverse Probl. 32, 085003 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  48. Wei, T., Zhang, Z.Q.: Robin coefficient identification for a time-fractional diffusion equation. Inverse Probl. Sci. Eng. 24, 647–666 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  49. Wu, B., Wu, S.: Existence and uniqueness of an inverse source problem for a fractional integrodifferential equation. Comput. Math. Appl. 68, 1123–1136 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  50. Xu, Y., Shu, C.W.: Local discontinuous Galerkin method for the Camassa–Holm equation. SIAM J. Numer. Anal. 46, 1998–2021 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  51. Yeganeh, S., Mokhtari, R., Hesthaven, J.S.: Space-dependent source determination in a time-fractional diffusion equation using a local discontinuous Galerkin method. BIT Numer. Math. 57, 685–707 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  52. Zhang, Y., Meerschaert, M.M., Baeumer, B.: Particle tracking for time-fractional diffusion. Phys. Rev. E. 78, 1–7 (2008)

    MATH  Google Scholar 

  53. Zhang, Y., Xu, X.: Inverse source problem for a fractional diffusion equation. Inverse Probl. 27, 035010 (2011)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Imam Khomeini International University, Qazvin, Iran

    Samaneh Qasemi, Davood Rostamy & Nazdar Abdollahi

Authors
  1. Samaneh Qasemi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Davood Rostamy
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Nazdar Abdollahi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Samaneh Qasemi.

Additional information

Communicated by Jan Hesthaven.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Qasemi, S., Rostamy, D. & Abdollahi, N. The time-fractional diffusion inverse problem subject to an extra measurement by a local discontinuous Galerkin method. Bit Numer Math 59, 183–212 (2019). https://doi.org/10.1007/s10543-018-0731-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10543-018-0731-z

Keywords

Mathematics Subject Classification

Search

Navigation