Space-dependent source determination in a time-fractional diffusion equation using a local discontinuous Galerkin method

Abstract

This paper is devoted to determining a space-dependent source term in an inverse problem of the time-fractional diffusion equation. We use a method based on a finite difference scheme in time and a local discontinuous Galerkin method in space and investigate the numerical stability and convergence of the proposed method. Finally, various numerical examples are used illustrate the effectiveness and accuracy of the method.

Appendix

Table 6 $\parallel A_M^{-1}\parallel _2$ for different values of $\alpha$

Table 7 Norm 2 of some matrices for Example 4.2 for different $\alpha$ with $\varepsilon =0.01$ (top) and for different $\varepsilon$ with $\alpha =0.6$ (bottom)

We seek to explain why our proposed method need not regularization method. Consider the time-fractional diffusion equation (1.1), with $a(x)=1$ and $c(x)=0$ (as we consider in Examples 4.1 and 4.3–4.5). Let us decompose the domain of the problem into cells of equal length h and choose for local basis functions of ${\mathbb {P}}^1(I_j)$ the monomial basis functions [20]. Solving (3.4), we have

$$\begin{aligned} K_{11}= & {} K_{22}={\mathrm{diag}}\left( h,\frac{h}{3},\ldots ,h,\frac{h}{3}\right) ,\qquad {\bar{K}}_{12}={\bar{K}}_{21}={\mathrm{diag}}(Z,\ldots ,Z),\qquad \\ Z= & {} \left[ \begin{array}{cc}0&{}\quad 0\\ 2&{}\quad 0\end{array}\right] , \end{aligned}$$

therefore ${\bar{K}}_{12}K_{22}^{-1}{\bar{K}}_{21}K_{22}^{-1}=(0)_{2N\times 2N}$, $K_m=I$ and

$$\begin{aligned} A_M F=G-\beta G_1+\beta {\bar{K}}_{12} K_{22}^{-1} G_2. \end{aligned}$$

(5.1)

$A_M$ has a complex structure and we are not able to find a closed form for it. In Table 6, we report $\parallel A_M^{-1}\parallel _2$ for different values of $\alpha$. Obviously $\parallel A_M^{-1}\parallel _2$ has a reasonable size and therefore in Examples 4.1 and 4.3–4.5 numerical solutions are not sensitive with respect to the perturbation in the initial data.

Fig. 6

Picard plot for Example 4.2 ($\alpha =0.1$)

Fig. 7

Picard plot for Example 4.2 ($\alpha =0.6$)

Fig. 8

Picard plot for Example 4.2 ($\alpha =0.95$)

In Table 7, we show $L^2$-norm of matrices G, $A_M^{-1}G$ and $A_M^{-1}$ for Example 4.2. Obviously, $\parallel A_M^{-1} G^\delta \parallel _2$ is small and $\parallel A_M^{-1} \parallel _2$ has a reasonable size. Therefore numerical solutions are not sensitive with respect to the perturbation in the initial data.

Therefore, we need not any regularization method.

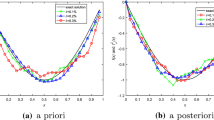
The sequel of this appendix is devoted to investigating the discrete Picard condition [10] in order to show that our proposed method need not any regularization. Here we just investigate Example 4.2. Similar results obtain for Examples 4.3–4.5. We use the MATLAB codes developed by Hansen [11] to prepare Picard plots for both the unperturbed and the perturbed data with various noise levels $\varepsilon =0.1, 1, 10\%$ which presented in Figs. 6, 7 and 8 for $\alpha =0.1, 0.6, 0.95$, respectively. In all cases, the Fourier coefficients $V_i^TR$ decay to zero faster than the $\sigma _i$. Here, $(\sigma _i,V_i)$’s are the pair of singular values and corresponding (left) singular vectors of matrix $A_M$ and R is the right-hand side of the linear system which will be solved, i.e. $A_MF=R:=K_M G-\beta G_1+\beta {\bar{K}}_{12} K_{22}^{-1} G_2$. Therefore, according to the discrete Picard condition, our method need not any regularization.