A Theoretical Approach to Understanding Population Dynamics with Seasonal Developmental Durations

Abstract

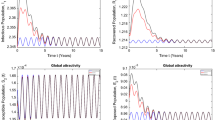
There is a growing body of biological investigations to understand impacts of seasonally changing environmental conditions on population dynamics in various research fields such as single population growth and disease transmission. On the other side, understanding the population dynamics subject to seasonally changing weather conditions plays a fundamental role in predicting the trends of population patterns and disease transmission risks under the scenarios of climate change. With the host–macroparasite interaction as a motivating example, we propose a synthesized approach for investigating the population dynamics subject to seasonal environmental variations from theoretical point of view, where the model development, basic reproduction ratio formulation and computation, and rigorous mathematical analysis are involved. The resultant model with periodic delay presents a novel term related to the rate of change of the developmental duration, bringing new challenges to dynamics analysis. By investigating a periodic semiflow on a suitably chosen phase space, the global dynamics of a threshold type is established: all solutions either go to zero when basic reproduction ratio is less than one, or stabilize at a positive periodic state when the reproduction ratio is greater than one. The synthesized approach developed here is applicable to broader contexts of investigating biological systems with seasonal developmental durations.

Appendix

To numerically compute the basic reproduction ratio, we are going to rewrite the linear operator L into the form of Eq. (3) in Posny and Wang (2014), where an algorithm is proposed for the $R_0$ computation of periodic ordinary differential systems. We should also note that other algorithms have been proposed, such as Bacaër (2007), for periodic growth models with time delay. However, here the delay is periodic and therefore, we first fit our computation into a former algorithm. Since

$$\begin{aligned} F(t-s)\left( \begin{array}{c} \phi _1 \\ \phi _2\end{array}\right) =\left( \begin{array}{c}b_L(t-s) \phi _2(-\tau _L(t-s))\\ b_P(t-s) \phi _1(-\tau _P(t-s))\end{array}\right) , \end{aligned}$$

we have

$$\begin{aligned}{}[Lv](t)= & {} \int _0^{\infty }Z(t,t-s)F(t-s)v(t-s+\cdot )ds \\= & {} \int _0^{\infty }\left( \begin{array}{llll}e^{-\int _{t-s}^t d_L(\xi )d\xi } &{} 0 \\ 0 &{} e^{-\int _{t-s}^t d_P(\xi )d\xi } \end{array}\right) \left( \begin{array}{c}b_L(t-s) v_2(t-s-\tau _L(t-s))\\ b_P(t-s) v_1(t-s-\tau _P(t-s))\end{array}\right) ds \\= & {} \left( \begin{array}{c}\int _0^{\infty }e^{-\int _{t-s}^t d_L(\xi )d\xi }b_L(t-s) v_2(t-s-\tau _L(t-s)) ds \\ \int _0^{\infty }e^{-\int _{t-s}^t d_P(\xi )d\xi }b_P(t-s) v_1(t-s-\tau _P(t-s)) ds \end{array}\right) . \end{aligned}$$

Let $t-s-\tau _L(t-s)=t-s_1$. Since the function $y=x-\tau _L(x)$ is strictly increasing, the inverse function exists and we can solve $x=h_L(y)$. Hence, we obtain $t-s=h_L(t-s_1)$, that is, $s=t-h_L(t-s_1)$, $ds_1=d(s+\tau _L(t-s))=(1-\tau _L'(t-s))ds$ and $ds=\frac{1}{1-\tau _L'(h_L(t-s_1))}ds_1$. Therefore,

$$\begin{aligned}&\int _0^{\infty }e^{-\int _{t-s}^t d_L(\xi )d\xi }b_L(t-s) v_2(t-s-\tau _L(t-s)) ds \\= & {} \int _{\tau _L(t)}^{\infty }\frac{e^{-\int _{h_L(t-s_1)}^t d_L(\xi )d\xi }b_L(h_L(t-s_1)) }{1-\tau _L'(h_L(t-s_1))}v_2(t-s_1)ds_1 \\= & {} \int _{\tau _L(t)}^{\infty }\frac{e^{-\int _{h_L(t-s)}^t d_L(\xi )d\xi }b_L(h_L(t-s)) }{1-\tau _L'(h_L(t-s))}v_2(t-s)ds. \end{aligned}$$

Similarly, let $t-s-\tau _P(t-s)=t-s_2$. Assume the inverse function of $y=x-\tau _P(x)$ is $y=h_P(x)$. Solving $t-s=h_P(t-s_2)$, we get

$$\begin{aligned} s=t-h_P(t-s_2), ds_2=(1-\tau _P'(t-s))ds \text { and } ds=\frac{1}{1-\tau _P'(h_P(t-s_2))}ds_2. \end{aligned}$$

Therefore,

$$\begin{aligned}&\int _0^{\infty }e^{-\int _{t-s}^t d_P(\xi )d\xi }b_P(t-s) v_1(t-s-\tau _P(t-s)) ds \\= & {} \int _{\tau _P(t)}^{\infty }\frac{e^{-\int _{h_P(t-s_2)}^t d_P(\xi )d\xi }b_P(h_P(t-s_2)) }{1-\tau _P'(h_P(t-s_2))}v_1(t-s_2)ds_2 \\= & {} \int _{\tau _P(t)}^{\infty }\frac{e^{-\int _{h_P(t-s)}^t d_P(\xi )d\xi }b_P(h_P(t-s)) }{1-\tau _P'(h_P(t-s))}v_1(t-s)ds. \end{aligned}$$

Define

$$\begin{aligned} K_{12}(t,s)=\left\{ \begin{array}{lrl} 0, &{} s<\tau _L(t) \\ \frac{e^{-\int _{h_L(t-s)}^t d_L(\xi )d\xi }b_L(h_L(t-s)) }{1-\tau _L'(h_L(t-s))}, &{} s\ge \tau _L(t) \\ \end{array}\right. \end{aligned}$$

and

$$\begin{aligned} K_{21}(t,s)=\left\{ \begin{array}{lrl} 0, &{} s<\tau _P(t) \\ \frac{e^{-\int _{h_P(t-s)}^t d_P(\xi )d\xi }b_P(h_P(t-s)) }{1-\tau _P'(h_P(t-s))}, &{} s\ge \tau _P(t) \\ \end{array}\right. \end{aligned}$$

while $K_{11}(t,s)=K_{22}(t,s)=0$. Then we can rewrite

$$\begin{aligned} \begin{array}{lrl} [Lv](t) &{}=&{} \int _0^{\infty }K(t,s)v(t-s)ds \\ &{}=&{} \sum \limits _{j=0}^{\infty }\int _{j\omega }^{(j+1)\omega }K(t,s)v(t-s)ds \\ &{}=&{} \sum \limits _{j=0}^{\infty }\int _{0}^{\omega }K(t,j\omega +s)v(t-s-j\omega )ds \\ &{}=&{} \int _{0}^{\omega }G(t,s)v(t-s)ds \\ \end{array} \end{aligned}$$

with

$$\begin{aligned} G(t,s)=\sum \limits _{j=0}^{\infty }K(t,j\omega +s), \end{aligned}$$

which is of the integral form in Posny and Wang (2014). Thus, the numerical algorithm in Posny and Wang (2014) can be used to compute the basic reproduction ratio for our model system.