Generalized Prolate Spheroidal Wave Functions: Spectral Analysis and Approximation of Almost Band-Limited Functions

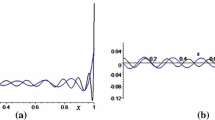
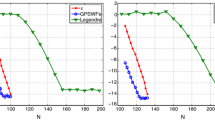
Abstract

In this work, we first give various explicit and local estimates of the eigenfunctions of a perturbed Jacobi differential operator. These eigenfunctions generalize the famous classical prolate spheroidal wave functions (PSWFs), founded in 1960s by Slepian and his co-authors and corresponding to the case $\alpha =\beta =0.$ They also generalize the new PSWFs introduced and studied recently in Wang and Zhang (Appl Comput Harmon Anal 29:303–329, 2010), denoted by GPSWFs and corresponding to the case $\alpha =\beta .$ The main content of this work is devoted to the previous interesting special case $\alpha =\beta >- 1.$ In particular, we give further computational improvements, as well as some useful explicit and local estimates of the GPSWFs. More importantly, by using the concept of a restricted Paley–Wiener space, we relate the GPSWFs to the solutions of a generalized energy maximisation problem. As a consequence, many desirable spectral properties of the self-adjoint compact integral operator associated with the GPSWFs are deduced from the rich literature of the PSWFs. In particular, we show that the GPSWFs are well adapted for the spectral approximation of the classical c-band-limited as well as almost c-band-limited functions. Finally, we provide the reader with some numerical examples that illustrate the different results of this work.

Appendix: Proof of Theorem 1

The proof is divided into three steps. To alleviate notations of this proof, we will simply denote $\psi _{n,c}^{(\alpha )}$ and $\chi _n(c)$ by $\psi _{n,c}$ and $\chi _n,$ respectively.

First step: We prove that for for any positive integer j with $j(j+2\alpha +1)\le \chi _n$, all moments $\int _{-1}^1 y^j \psi _{n,c}(y)\, dy$ are non negative and

$$\begin{aligned} 0\le \int _{-1}^1 y^{j} \psi _{n,c}(y)\, \omega _{\alpha }(y)\, dy \le \sqrt{1+\alpha }\left( \frac{1}{q}\right) ^{j} |\mu _n^{(\alpha )}(c)|. \end{aligned}$$

(73)

To this end, we first check that for any integer $k\ge 0$ satisfying $k(k+2\alpha +1)\le \chi _n,$ we have

$$\begin{aligned} \left| \psi _{n,c}^{(k)}(0)\right| \le (\sqrt{\chi _n})^k \sqrt{1+\alpha }. \end{aligned}$$

(74)

It suffices to prove that $m_k=\frac{|\psi _{n,c}^{(k)}(0)|}{\sqrt{\chi _n}^k} \le \sqrt{1+\alpha }.$ From the parity of $\psi _{n,c},$ we need only to consider derivatives of even or odd order. We assume that $n=2l$ is even. The case where n is odd is done in a similar manner. Note that for a fixed n,  $\psi _{n,c}^{(2l)}(0)$ has alternating signs, that is $\psi _{n,c}^{(k)}(0) \psi _{n,c}^{(k-2)}(0)<0$ In fact, for $k=0,$ we have $\psi _{n,c}(0)\psi _{n,c}^{(2)}(0)=-\chi _n\psi _{n,c}(0)^2<0.$ By induction, we assume that $\psi _{n,c}^{(k)}(0) \psi _{n,c}^{(k-2)}<0 .$ As it is done in [6], we have

$$\begin{aligned} \psi _{n,c}^{(k+2)}(0) \psi _{n,c}^{(k)}(0)=\Big ( k(k+1+2\alpha )-\chi _n \Big ) \psi _{n,c}^{(k)}(0)^2+k(k-1)c^2 \psi _{n,c}^{(k-2)}(0)\psi _{n,c}^{(k)}(0). \end{aligned}$$

(75)

By using the induction hypothesis as well as the fact that $k(k+1+\alpha +\beta ) \le \chi _n,$ one concludes that the induction assumption holds for the order k. Consequently, we have

$$\begin{aligned} |\psi _{n,c}^{(k+2)}(0)|=\Big (\chi _n-k(k+1+2\alpha )\Big ) |\psi _{n,c}^{(k)}(0)|+k(k-1)c^2| \psi _{n,c}^{(k-2)}(0)|. \end{aligned}$$

(76)

The previous equality implies that

$$\begin{aligned} m_{k+2}=\Big (1-\frac{k(k+1+2\alpha )}{\chi _n}\Big )m_k +k(k-1)\frac{q}{\chi _n}m_{k-2}. \end{aligned}$$

(77)

Hence, for any positive and even integer k with $k(k+2\alpha +1)\le \chi _n,$ we have $m_k\le m_0\le \sqrt{1+\alpha }.$ This last inequality follows from (32) with $t=0.$ This proves the inequality (74). Moreover, by taking the jth derivative at zero on both sides of ${\int _{-1}^1 e^{icxy} \psi _{n,c}(y)\omega _{\alpha }(y) dy =\mu _n^{(\alpha )}(c) \psi _{n,c}(x),}$ one gets

$$\begin{aligned} \int _{-1}^1 y^j \psi _{n,c}(y)\,\omega _{\alpha }(y) dy= (-i)^j c^{-j} \mu _n^{(\alpha )}(c)\psi _{n}^{(j)}(0). \end{aligned}$$

(78)

Since $\psi _{n}^{(j)}(0)$ and $\psi _{n}^{(j+2)}(0)$ have opposite signs, then the previous equation implies that all moments with even order j with $j(j+2\alpha +1)\le \chi _n$ have the same sign. The inequality (73) follows from (74).

Second step: We show that for all positive integers k, n with $k(k+2\alpha +1)+C_{\alpha }\, c^2\le \chi _n(c)$, we have $\beta _k^n\ge 0.$ Here $C_{\alpha }$ is as given by (53). The positivity of $\beta _0^n$ (when n is even) and $\beta _1^n$ (when n is odd) follow from the fact that

$$\begin{aligned}&\beta _0^n=\sqrt{\frac{\Gamma (\alpha +3/2)}{\sqrt{\pi }\Gamma (\alpha +1)}} |\mu _n^{(\alpha )}(c)| |\psi _{n,c}(0)|,\nonumber \\&\beta _1^n=\sqrt{\frac{\Gamma (\alpha +3/2)}{\sqrt{\pi }\Gamma (\alpha +1)}}\sqrt{2\alpha +3} |\mu _n^{(\alpha )}(c)| \left| \frac{\psi _n'(0)}{c}\right| . \end{aligned}$$

(79)

Since the $\beta _k^n$ are given by (24), then by using the hypothesis of the theorem, we have

$$\begin{aligned}&\beta _2^{n} =\frac{2\alpha +3}{c}\sqrt{\frac{2(2\alpha +5)}{2\alpha +2}}\left( \chi _n-\frac{c^2}{2\alpha +3}\right) \beta _0^{n} \ge \beta _0^n,\\&\beta _3^{n} =\frac{2\alpha +5}{2c^2}\sqrt{\frac{3(\alpha +1)}{2\alpha +7}}\Big ( (2\alpha +2)+\frac{3c^2}{2\alpha +5}\Big )\beta _1^{n}\ge 0. \end{aligned}$$

For $j\ge 2$ and by rearranging the system (24) and using the induction hypothesis $\beta _j^n \ge \beta _{j-2}^n \ge 0,$ one gets

$$\begin{aligned} M_{\alpha } c^2 (\beta _{j+2}^n +\beta _{j-2}^n)\ge ( \chi _n(c)-j(j+2\alpha +1)-N_{\alpha } c^2) \beta _j^n, \end{aligned}$$

(80)

where $M_{\alpha }$ and $N_{\alpha }$ are as given by (53). If we suppose that $\beta _{j+2} \le \beta _j^n,$ then from (80), one gets

$$\begin{aligned} 2M_{\alpha } c^2 \beta _{j}^n \ge ( \chi _n(c)-j(j+2\alpha +1)-N_{\alpha } c^2) \beta _j^n \end{aligned}$$

(81)

which contradicts the choice of $C_{\alpha }$ and the fact that $k(k+2\alpha +1)+C_{\alpha }\, c^2\le \chi _n(c).$ Hence, the induction hypothesis holds for $\beta _{j+2}^n.$

Third step: We prove (52). The first inequality follows from (79) and (74). To prove the second inequality, we recall that the moments $M_{j,k}$ of the normalized Jacobi polynomials ${\widetilde{P}}_k^{(\alpha ,\alpha )}$ are given by (17) and they are non-negative. Moreover, since ${\displaystyle x^j=\sum \nolimits _{k=0}^j M_{jk} {\widetilde{P}}_k^{(\alpha ,\alpha )}(x),}$ then the moments of the $\psi _{n,c}$ are related to the GPSWFs series expansion coefficients by the following relation

$$\begin{aligned} \int _{-1}^1 x^j \psi _{n,c}(x)\,\omega _{\alpha }(x)\, dx = \sum _{k=0}^j M_{j,k} \beta _k^n. \end{aligned}$$

Since from the previous step, we have $\beta _k^n \ge 0,$ for any $0\le k\le j$ and since the $a_{jk}$ are non negative, then the previous equality implies that

$$\begin{aligned} \beta _j^n \le \frac{1}{M_{j,j}} \int _{-1}^1 x^j \psi _{n,c}(x)\,\omega _{\alpha }(x)\, dx \le \frac{1}{M_{j,j}} \sqrt{1+\alpha }\left( \frac{1}{q}\right) ^{j}|\mu _n^{(\alpha )}(c)|. \end{aligned}$$

(82)

The last inequality follows from the result of the first step. Moreover, by using the explicit expression of $M_{j,j},$ given by (17), together with (10), (51), the decay of the function $\varphi ,$ given by (13), as well as some straightforward computations, one obtains

$$\begin{aligned} \frac{1}{M_{j,j}}\le 2^j \frac{2^{\alpha }}{e^{2\alpha +3/2}}\frac{(3/2)^{3/4}(3/2+2\alpha )^{3/4+\alpha }}{\sqrt{3/2+\alpha }}. \end{aligned}$$

(83)

Finally, by combining (82) and (83), one gets the second inequality of (52). $\square$