1 Introduction

Recently, the global exponential stability of positive periodic solutions and almost-periodic solutions for the famous Nicholson’s blowflies equation with multiple time-varying delays:

$$\begin{aligned} x'(t)=-\beta (t)x(t)+\sum_{j=1}^{m} \alpha _{j}(t)x\bigl(t-\sigma _{j}(t)\bigr)e ^{-\gamma _{j}(t)x(t-\sigma _{j}(t))},\quad t \geq t_{0}, \end{aligned}$$
(1.1)

has been intensively studied in [1–3]. Here, \(\beta (t), \alpha _{j}(t), \sigma _{j}(t)\), and \(\gamma _{j}(t)\) are all continuous and nonnegative functions, \(\beta (t) \) and \(\gamma _{j}(t)\) are bounded below by positive constants, \(\beta (t) x(t)\) is the death rate of the population which depends on time t and the current population level \(x (t)\), \(\alpha _{j}(t) x(t-\sigma _{j} (t) )e^{- \gamma _{j}(t) x(t-\sigma _{j}(t))}\) is the time-dependent birth function which involves maturation delay \(\sigma _{ j}(t)\), and reproduces at its maximum rate \(\frac{1}{\gamma _{ j}(t)}\), and \(j\in \varPi:=\{1,2, \dots, m\}\).

It should be mentioned that, by restricting the existence of the periodic and almost-periodic solutions for (1.1) in a small interval \([\kappa, \widetilde{\kappa }]\approx [0.7215355, 1.342276]\), all results in [1–3] were obtained under the crucial assumption:

$$\begin{aligned} \gamma _{j}(t)\geq 1, \quad\text{for all } t\in \mathbb{R}, j\in \varPi, \end{aligned}$$
(1.2)

where \(\kappa \in (0, 1)\) and \(\tilde{\kappa }\in (1,+\infty )\) satisfy

$$\begin{aligned} \frac{1-\kappa }{e^{\kappa }}=\frac{1}{e^{2}}, \qquad \sup_{x\geq \kappa } \biggl\vert \frac{1-x}{e^{x}} \biggr\vert =\frac{1}{e^{2}}, \qquad \kappa e^{-\kappa }=\widetilde{\kappa } e^{-\widetilde{\kappa }}. \end{aligned}$$
(1.3)

Most recently, the authors in [4–6] pointed out that it is interesting that when the maximum reproduction rate is not limited (i.e., \(\frac{1}{\gamma _{j}(t)}\) maybe sufficiently large), the stability of a class of delayed nonlinear density-dependent mortality Nicholson’s blowflies models without the assumption (1.2) can be established. However, there are no research works on the global exponential stability of periodic solutions for Nicholson’s blowflies equation (1.1) without assumption (1.2) and \([\kappa, \widetilde{\kappa }]\) as the existence interval for the periodic solutions.

Based on the above discussions, in this paper, avoiding assumption (1.2) and without adopting \([\kappa, \widetilde{\kappa }]\) as the existence interval of periodic solutions, we establish the global exponential stability of periodic solutions for system (1.1). The proposed criterion improves and complements some existing results in the recent publications [1–3], and its effectiveness is demonstrated by a numerical example.

From now on, we suppose that \(\beta, \gamma _{j}:[ t_{0}, +\infty ) \rightarrow (0, +\infty ) \) and \(\sigma _{j}, \alpha _{j}:[ t_{0}, + \infty )\rightarrow [0, +\infty )\) are continuous T-periodic functions with \(T>0\) and \(j\in \varPi \). Let

$$\begin{aligned} 1\geq \gamma ^{+}=\max_{j\in \varPi }\Bigl\{ \sup _{t\in [t_{0}, +\infty )}\gamma _{j}(t)\Bigr\} , \qquad \gamma ^{-}=\min_{j\in \varPi }\Bigl\{ \inf_{t\in [t_{0}, +\infty )} \gamma _{j}(t)\Bigr\} , \end{aligned}$$

and

$$\begin{aligned} \sigma =\max_{ j\in \varPi }\Bigl\{ \sup_{t\in [t_{0}, +\infty )} \sigma _{j}(t)\Bigr\} ,\qquad C_{+}= C\bigl([- \sigma, 0], [0, +\infty)\bigr). \end{aligned}$$

Furthermore, consider the following initial value conditions:

$$\begin{aligned} x(t_{0}+\theta ) =\varphi (\theta ), \quad\theta \in [-\sigma, 0], \varphi \in C_{+} \text{ and } \varphi (0)>0. \end{aligned}$$
(1.4)

We let \(x(t; t_{0}, \varphi ) \) denote a solution of the initial value problem (1.1) and (1.4), and the maximal right-interval of existence of \(x(t; t_{0}, \varphi )\) is marked by \([t_{0}, \eta (\varphi ))\). Then, the existence and uniqueness of \(x(t; t_{0}, \varphi )\) is straightforwardly established in [1].

2 Preliminary results

In this section, we give three lemmas which will play important roles in the next section.

Lemma 2.1

LetAandδbe constants such that \(A>1, e<\frac{1}{\delta }\leq e^{2}\), and \(\delta =Ae^{-A}\). Then, \(\delta A> \frac{1}{e}\).

Proof

Evidently,

$$\begin{aligned} \delta A=A^{2}e^{-A} \quad\text{and} \quad e< \frac{e^{A}}{A}\leq e^{2}. \end{aligned}$$
(2.1)

Define \(f(u)=\frac{e^{u}}{u}\) for \(u\in (0, +\infty )\) and \(f(x_{0})=e^{2}\) with \(x_{0}>1\). Then, \(f'(u)= \frac{e^{u}(u-1)}{u^{2}}>0 (u>1)\), and \(f(u)\) is monotonously increasing on \((1,+\infty )\). Clearly, \(f(1)=e, f(e)=e^{e-1}< e^{2}\), which, together with (2.1), suggests that \(A\in (1,x_{0} ]\), and \(x_{0}>e\).

Now, letting \(G(u)=u^{2}e^{-u}\) on \((0, +\infty )\), it suffices to show that \(G(A)>\frac{1}{e}\). In fact, \(G'(u)= e^{-u}u(2-u)\), \(G(u)\) increases on \((1, 2)\) and decreases on \((2, x_{0})\), \(G(1)=e^{-1}=\frac{1}{e}\), as well as

$$\begin{aligned} G(x_{0})=x_{0}^{2}e^{-x_{0}}=\frac{x_{0}}{f(x_{0})}>\frac{e}{e^{2}}=\frac{1}{e}. \end{aligned}$$

Consequently, \(G(A)>\min \{G(1), G(x_{0})\}=\frac{1}{e}\). The proof is complete. □

Lemma 2.2

\(x (t; t_{0},\varphi )\)is positive and bounded on \([t_{0}, \eta ( \varphi )) \), and \(\eta (\varphi )=+\infty \).

Proof

First, it follows from Theorem 5.2.1 in [7], p. 81, that \(x_{t}(t_{0}, \varphi )\in C_{+}\) for all \(t \in [t_{0}, \eta (\varphi ))\). Let \(x (t )= x (t; t_{0},\varphi )\). Noting that \(x(t_{0})=\varphi (0)>0\), we gain

$$\begin{aligned} x(t)={}&e^{-\int _{t_{0}}^{t}\beta (v)\,dv}x(t_{0}) \\ &{} + \int _{t_{0}}^{t}e^{-\int _{s}^{t} \beta (v)\,dv}\sum _{j=1}^{m} \alpha _{j}(s)x \bigl(s- \sigma _{j}(s)\bigr)e^{- \gamma _{j}(s)x(s- \sigma _{j}(s))}\,ds \\ >{}&0, \quad\text{for all } t\in [t_{0}, \eta (\varphi )). \end{aligned}$$

Second, due to the positivity and periodicity of coefficient functions, we can choose a positive constant M such that

$$\begin{aligned} \sum_{j=1}^{m} \frac{\alpha _{j} (t)}{\beta (t)\gamma _{j} (t)} \leq M\quad \text{for all } t\in [t_{0}, +\infty ). \end{aligned}$$

Therefore, in view of the fact that \(\sup_{u\geq 0} ue^{-u}=\frac{1}{ e}\), we obtain

$$\begin{aligned} x(t)={}&e^{-\int _{t_{0}}^{t}\beta (v)\,dv}x(t_{0}) \\ & {}+ \int _{t_{0}}^{t} e^{ -\int _{s}^{t} \beta (v)\,dv}\sum _{j=1}^{m} \frac{\alpha _{j} (s)}{\gamma _{j} (s)}\gamma _{j} (s)x \bigl(s- \sigma _{j}(s)\bigr)e^{-\gamma _{j} (s)x(s-\sigma _{j} (s))} \,ds \\ \leq{} &e^{-\int _{t_{0}}^{t} \beta (v) \,dv}x(t_{0}) + \int_{t_{0}}^{t} e ^{ -\int _{s}^{t} \beta (v) \,dv} \sum _{j=1}^{m} \frac{\alpha _{j} (s)}{\gamma _{j} (s)} \frac{1}{e}\,ds \\ \leq{}& e^{-\int _{t_{0}}^{t} \beta (v) \,dv}x (t_{0}) +\frac{M}{e} \int _{t_{0}}^{t} e^{ -\int _{s}^{t} \beta (v) \,dv} \beta (s)\,ds \\ &=e^{-\int _{t_{0}}^{t}\beta (v)\,dv}x(t_{0})+\frac{M}{e} \bigl[1-e^{-\int _{t_{0}}^{t} \beta (v) \,dv}\bigr] \\ :={}&\varLambda (t), \quad\text{for all } t\in [t_{0}, \eta (\varphi )). \end{aligned}$$

Finally, we have from Theorem 2.3.1 in [8] and the boundedness of \(\varLambda (t)\) that \(\eta (\varphi )=+\infty \), and \(x (t )\) is positive and bounded on \([t_{0}, +\infty )\). □

Lemma 2.3

Let

$$ \textstyle\begin{cases} \inf_{t\in [t_{0}, +\infty )}(\frac{\sum_{j=1}^{m} \alpha _{j} (t)}{\beta (t)})>1, \\ e< \inf_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{ \alpha _{j} (t)}{\beta (t)\gamma _{j}(t)}\leq \sup_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{\alpha _{j} (t)}{\beta (t)\gamma _{j} (t)}< e^{2} \end{cases} $$
(2.2)

and

$$\begin{aligned} \inf_{t\in [t_{0}, +\infty )}\ln \biggl( \frac{\sum_{j=1} ^{m} \alpha _{j}(t)}{\beta (t)}\biggr)> \frac{\kappa }{\gamma ^{-}},\qquad \frac{ \inf_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{ \alpha _{j} (t)}{\beta (t) }}{\sup_{t\in [t_{0}, +\infty )} \sum_{j=1}^{m} \frac{\alpha _{j} (t)}{\beta (t)\gamma _{j} (t)}}>\frac{ \kappa }{\gamma ^{-}}. \end{aligned}$$
(2.3)

Then,

$$\begin{aligned} \frac{\kappa }{\gamma ^{-}}< l:= \liminf_{t\rightarrow +\infty }x(t; t_{0}, \varphi ) \leq L:= \limsup_{t\rightarrow +\infty }x(t; t_{0}, \varphi ) < A, \end{aligned}$$
(2.4)

where

$$\begin{aligned} \delta = \frac{1}{\sup_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{\alpha _{j}(t)}{\beta (t)\gamma _{j} (t)}},\qquad A>1 \quad\textit{and}\quad \delta =Ae^{-A}. \end{aligned}$$
(2.5)

Proof

From (2.2), (2.5) and Lemma 2.1, one can see that

$$\begin{aligned} \sup_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{ \alpha _{j} (t)}{\beta (t)\gamma _{j}(t)}\frac{1}{e}< A. \end{aligned}$$
(2.6)

We claim that \(\liminf_{t\to +\infty } x(t)=l>0\). Otherwise, \(l=0\). For any \(t\geq t_{0} \), we set

$$\begin{aligned} \nu (t)=\max \Bigl\{ \varrho \in [t_{0}, t]|x(\varrho )=\min _{t_{0} \leq s\leq t} x (s) \Bigr\} . \end{aligned}$$

We conclude from \(l=0\) that \(\nu (t) \to +\infty \) as \(t\to +\infty \) and \(\lim_{t\to +\infty }x(\nu (t))=0\). On the other hand, \(x(\nu (t))=\min_{t_{0} \leq s\leq t}x(s)\), and \(x'(\nu (t)) \leq 0 \text{ for all } \nu (t)>t_{0}\). Then, (1.1) leads to

$$\begin{aligned} 0 \geq x'\bigl(\nu (t)\bigr)= {}&{-}\beta \bigl(\nu (t)\bigr)x\bigl( \nu (t)\bigr) \\ &{}+\sum_{j=1}^{m}\alpha _{j}\bigl(\nu (t)\bigr)x\bigl(\nu (t) -\sigma _{j} \bigl( \nu (t)\bigr)\bigr)e^{-\gamma _{j}(\nu (t))x(\nu (t)-\sigma _{j}(\nu (t)))} \end{aligned}$$
(2.7)

and

$$\begin{aligned} \beta \bigl(\nu (t)\bigr) x \bigl(\nu (t)\bigr) \geq \alpha _{j} \bigl(\nu (t)\bigr) x \bigl(\nu (t)- \sigma _{j} \bigl(\nu (t)\bigr) \bigr)e^{- \gamma _{j} (\nu (t)) x (\nu (t)-\sigma _{j} ( \nu (t)))}, \end{aligned}$$

where \(\nu (t)>t_{0}, j\in \varPi \), which, together with the fact that \(\lim_{t\to +\infty }x (\nu (t))=0 \), suggests that

$$\begin{aligned} \lim_{t\rightarrow +\infty }x \bigl(\nu (t)- \sigma _{j} \bigl( \nu (t)\bigr)\bigr)=0,\quad j\in \varPi. \end{aligned}$$
(2.8)

From (2.2), (2.7), (2.8) and the fact that

$$\begin{aligned} 1 &\geq \frac{1}{\beta (\nu (t))} \sum_{j=1}^{m} \alpha _{j} \bigl( \nu (t)\bigr) \frac{x (\nu (t)-\sigma _{j} (\nu (t)))}{x (\nu (t))}e^{- \gamma _{j} (\nu (t)) x (\nu (t)-\sigma _{j} (\nu (t)))} \\ &\geq \frac{1}{\beta (\nu (t))} \sum_{j=1}^{m} \alpha _{j} \bigl( \nu (t)\bigr)e^{- \gamma _{j} (\nu (t))x(\nu (t)-\sigma _{j}(\nu (t)))}, \end{aligned}$$

letting \(t\rightarrow +\infty \) results in

$$\begin{aligned} 1\geq \limsup_{t\rightarrow +\infty }\biggl( \frac{\sum_{j=1} ^{m}\alpha _{j} (\nu (t))}{\beta (\nu (t))}\biggr)\geq \inf_{t\in [t_{0}, +\infty )}\biggl(\frac{\sum_{j=1}^{m}\alpha _{j} (t)}{\beta (t)}\biggr)>1, \end{aligned}$$

which is a contradiction. Hence, \(l>0\).

Now, by the fluctuation lemma [9], Lemma A.1., there are two sequences \(\{q_{k}^{*}\}_{k=1}^{+\infty }\) and \(\{q_{k}^{**}\}_{k=1}^{+\infty }\) obeying

$$\begin{aligned} \lim_{k\rightarrow +\infty }q_{k}^{*}\rightarrow + \infty,\qquad \lim_{k\rightarrow +\infty }x \bigl(q_{k}^{*} \bigr)=L\quad \text{and}\quad \lim_{k\rightarrow +\infty }x' \bigl(q_{k}^{*} \bigr) = 0, \end{aligned}$$
(2.9)

and

$$\begin{aligned} \lim_{k\rightarrow +\infty }q_{k}^{**}\rightarrow + \infty,\qquad \lim_{k\rightarrow +\infty }x \bigl(q_{k}^{**} \bigr) =l \quad\text{and} \quad\lim_{k\rightarrow +\infty } x' \bigl(q_{k}^{**} \bigr) = 0, \end{aligned}$$
(2.10)

respectively. Without loss of generality, regarding the periodicity of delays and coefficients, we can assume that \(\lim_{k \rightarrow + \infty } \beta (q_{k}^{*}), \lim_{k \rightarrow + \infty } \alpha _{j} (q_{k}^{*}), \lim_{k \rightarrow + \infty } \gamma _{j}(q_{k}^{*}), \lim_{k \rightarrow + \infty } x(q_{k}^{*}-\sigma _{ j} (q_{k}^{*})), \lim_{k \rightarrow + \infty } \beta (q_{k}^{**}), \lim_{k \rightarrow + \infty }\alpha _{j}(q_{k}^{**}), \lim_{k \rightarrow + \infty } \gamma _{j}(q_{k}^{**}) \) and \(\lim_{k \rightarrow + \infty }x(q_{k}^{**}-\sigma _{ j} (q _{k}^{**})) \) exist for all \(j\in \varPi \).

Likewise, (1.1), (2.6) and (2.9) yield

$$\begin{aligned} 0={}&\lim_{k \rightarrow + \infty }x' \bigl(q_{k}^{*} \bigr) \\ ={}&{-}\lim_{k \rightarrow + \infty }\beta \bigl(q_{k}^{*} \bigr) \lim_{k \rightarrow + \infty }x\bigl(q_{k}^{*} \bigr) \\ &{} +\sum_{j=1}^{m}\lim _{k \rightarrow + \infty }\frac{ \alpha _{j}(q_{k}^{*})}{\gamma _{j}(q_{k}^{*})} \lim_{k \rightarrow + \infty } \bigl[\gamma _{j}\bigl(q_{k}^{*}\bigr)x \bigl(q_{k}^{*}- \sigma _{j} \bigl(q_{k}^{*}\bigr) \bigr)e^{- \gamma _{j}(q_{k}^{*})x(q_{k}^{*}-\sigma _{j}(q_{k}^{*}))}\bigr] \\ \leq{}& {-}\lim_{k \rightarrow +\infty }\beta \bigl(q_{k}^{*} \bigr)L + \sum_{j=1}^{m}\lim _{k \rightarrow + \infty }\frac{\alpha _{j}(q_{k}^{*})}{\gamma _{j}(q_{k}^{*})}\frac{1}{e}, \end{aligned}$$

and

$$\begin{aligned} L\leq \lim_{k \rightarrow + \infty } \Biggl[\sum_{j=1}^{m} \frac{ \alpha _{j}(q_{k}^{*})}{\beta (q_{k}^{*})\gamma _{j}(q_{k}^{*})}\Biggr] \frac{1}{e}< A. \end{aligned}$$
(2.11)

Consequently, let \(j_{0}\in \varPi \) such that

$$\begin{aligned} \lim_{k \rightarrow + \infty } x \bigl(q_{k}^{**}- \sigma _{j_{0}} \bigl(q _{k}^{**}\bigr) \bigr)=l_{0}\in [l, L], \end{aligned}$$

and

$$\begin{aligned} &\lim_{k \rightarrow +\infty }\bigl[x \bigl(q_{k}^{**}- \sigma _{j_{0}} \bigl(q _{k}^{**}\bigr) \bigr)e^{-x(q_{k}^{**}-\sigma _{j_{0}}(q_{k}^{**}))}\bigr] \\ &\quad =l_{0}e^{-l_{0}}=\min_{j\in \varPi }\lim _{k \rightarrow + \infty }\bigl[x\bigl(q_{k}^{**}-\sigma _{j} \bigl(q_{k}^{**}\bigr) \bigr)e^{-x(q_{k}^{**}-\sigma _{j}(q_{k}^{**}))}\bigr]. \end{aligned}$$

It follows from (1.1), (2.10) and the fact that \(\min_{[a, b]\subseteq [0, +\infty )}ue^{-u}=\min \{ae^{-a}, be ^{-b}\}\) that

$$\begin{aligned} 0={}&\lim_{k \rightarrow + \infty }x' \bigl(q_{k}^{**} \bigr) \\ ={}&{-}\lim_{k \rightarrow + \infty }\beta \bigl(q_{k}^{**} \bigr)l+\sum_{j=1}^{m}\lim _{k \rightarrow + \infty }\alpha _{j}\bigl(q _{k}^{**} \bigr)\lim_{k \rightarrow + \infty }\bigl[x \bigl(q_{k}^{**}- \sigma _{j}\bigl(q_{k}^{**}\bigr)\bigr) \\ &{} \times e^{- \gamma _{j}(q_{k}^{**})x(q_{k}^{**}-\sigma _{j}(q_{k}^{**}))}\bigr] \\ \geq{} &{-}\lim_{k \rightarrow + \infty }\beta \bigl(q_{k}^{**} \bigr)l+ \sum_{j=1}^{m}\lim _{k \rightarrow + \infty }\alpha _{j}\bigl(q _{k}^{**} \bigr)\lim_{k \rightarrow + \infty }\bigl[x \bigl(q_{k}^{**}- \sigma _{j}\bigl(q_{k}^{**}\bigr)\bigr) \\ & {}\times e^{-x(q_{k}^{**}-\sigma _{j}(q_{k}^{**}))}\bigr] \\ \geq{}& {-}\lim_{k \rightarrow + \infty }\beta \bigl(q_{k}^{**} \bigr)l+l _{0}e^{-l_{0}}\sum_{j=1}^{m} \lim_{k \rightarrow +\infty }\alpha _{j}\bigl(q_{k}^{**} \bigr) \\ \geq{} &{-}\lim_{k \rightarrow + \infty }\beta \bigl(q_{k}^{**} \bigr)l+ \min \bigl\{ le^{-l}, Le^{-L}\bigr\} \sum _{j=1}^{m}\lim_{k \rightarrow +\infty }\alpha _{j}\bigl(q_{k}^{**}\bigr). \end{aligned}$$
(2.12)

If \(le^{-l}=\min \{le^{-l}, Le^{-L}\}\), (2.3) and (2.12) yield

$$\begin{aligned} l\geq \ln \biggl(\lim_{k \rightarrow +\infty }\frac{\sum_{j=1} ^{m}\alpha _{j}(q_{k}^{**})}{\beta (q_{k}^{**})}\biggr)\geq \inf_{t\in \mathbb{R}}\ln \biggl( \frac{\sum_{j=1}^{m}\alpha _{j}(t)}{ \beta (t)}\biggr)> \frac{\kappa }{\gamma ^{-}}. \end{aligned}$$
(2.13)

If \(Le^{-L}=\min \{le^{-l}, Le^{-L}\}< le^{-l}\), then (2.11) implies

$$\begin{aligned} 1< L\leq A,\qquad Le^{-L}\geq Ae^{-A}, \end{aligned}$$

which, together with (2.3) and (2.12), entails that

$$\begin{aligned} l\geq \frac{Ae^{-A}}{\lim_{k \rightarrow +\infty }\frac{\beta (q_{k}^{**})}{\sum_{j=1}^{m}\alpha _{j}(q_{k}^{**})} }\geq \frac{ \inf_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{ \alpha _{j} (t)}{\beta (t) }}{\sup_{t\in [t_{0}, +\infty )} \sum_{j=1}^{m} \frac{\alpha _{j} (t)}{\beta (t)\gamma _{j} (t)}}>\frac{ \kappa }{\gamma ^{-}}, \end{aligned}$$
(2.14)

which, together with (2.11) and (2.13), establishes (2.4). This finishes the proof of Lemma 2.3. □

3 Main results

The main results in this paper will now be presented as the subsequent proposition and theorem.

Proposition 3.1

Suppose that all assumptions in Lemma 2.3are satisfied. Then, for \(\varphi,\psi \in C_{+}\)with \(\varphi (0)>0\)and \(\psi (0)>0\), there exist constants \(Q_{\varphi, \psi }>t_{0}\), \(K _{\varphi, \psi }>0\)and \(\lambda >0\)such that

$$\begin{aligned} \bigl\vert x(t; t_{0}, \varphi )-x(t; t_{0}, \psi ) \bigr\vert < K _{\varphi, \psi } e ^{-\lambda t} \quad\textit{for all } t>Q_{\varphi, \psi }>t_{0}. \end{aligned}$$
(3.1)

Proof

Denote \(x^{\varphi }(t)= x(t; t_{0}, \varphi )\) and \(x^{\psi }(t)= x(t; t_{0}, \psi )\). By Lemma 2.3 and the fact that \(\gamma ^{+}\leq 1\), there exists \(Q_{\varphi, \psi }>t_{0} \) such that

$$\begin{aligned} \frac{\kappa }{\gamma ^{-}} < x^{\varphi }(t),\qquad x^{\psi }(t)< A, \quad \kappa < \gamma _{j}(t)x^{\varphi }\bigl(t -\sigma _{j}(t)\bigr), \gamma _{j}(t)x ^{\psi }\bigl(t -\sigma _{j}(t)\bigr) \end{aligned}$$
(3.2)

for all \(t\in [Q_{\varphi, \psi }-\sigma, +\infty ) \) and \(j\in \varPi \). According to (2.2), we can take \(\lambda >0\) such that

$$\begin{aligned} \max_{t\in [0, T]} \Biggl\{ -\bigl[\beta (t)-\lambda \bigr] +\sum ^{m} _{j=1}\frac{\alpha _{ j}(t)}{\gamma _{ j}(t)} \frac{1}{e^{2}} e^{\lambda \sigma } \Biggr\} < 0. \end{aligned}$$
(3.3)

Define \(z(t)= x^{\varphi }(t)-x^{\psi }(t)\) and \(M (t) = |z(t)|e^{ \lambda t}\) for all \(t\in [t_{0}-\sigma, +\infty )\). Consequently,

$$\begin{aligned} z'(t)={}&{-}\beta (t)z(t)+\sum^{m}_{j=1} \alpha _{j}(t)\bigl[x^{\varphi }\bigl(t- \sigma _{j}(t)\bigr)e^{-\gamma _{ j}(t)x^{\varphi }(t-\sigma _{j}(t))} \\ &{} -x^{\psi }\bigl(t-\sigma _{j}(t)\bigr)e^{-\gamma _{j}(t)x^{\psi } (t-\sigma _{ j}(t))} \bigr] \end{aligned}$$

and

$$\begin{aligned} D^{-}\bigl(M (t)\bigr)\leq{}& {-}\beta (t) \bigl\vert z (t ) \bigr\vert e^{\lambda t} \\ &{} +\sum^{m}_{j=1}\alpha _{ j}(t) \bigl\vert x^{\varphi }\bigl(t-\sigma _{j}(t)\bigr)e ^{-\gamma _{ j}(t)x^{\varphi }(t-\sigma _{j}(t))}-x^{\psi }\bigl(t-\sigma _{j}(t)\bigr) \\ & {}\times e^{-\gamma _{ j}(t)x^{\psi }(t-\sigma _{ j}(t))} \bigr\vert e^{\lambda t}+ \lambda \bigl\vert z (t) \bigr\vert e^{\lambda t},\quad\text{for all }t>Q_{\varphi, \psi }. \end{aligned}$$
(3.4)

Now, we show that

$$\begin{aligned} M(t) &< e^{\lambda Q_{\varphi,\psi }}\Bigl(\max_{t\in [t_{0}-\sigma, Q_{\varphi, \psi }]} \bigl\vert x^{\varphi }(t)-x ^{\psi } (t) \bigr\vert +1\Bigr) \\ &:= K_{\varphi, \psi } \quad\text{for all }t>Q_{\varphi, \psi }. \end{aligned}$$
(3.5)

Suppose on the contrary and pick \(Q_{*}>Q_{\varphi, \psi }\) such that

$$\begin{aligned} M(Q_{*})=K_{\varphi, \psi } \quad\text{and}\quad M (t)< K _{\varphi, \psi }\quad \text{for all } t\in [t_{0}-\sigma, Q_{*}). \end{aligned}$$
(3.6)

From the definition of κ, we have

$$\begin{aligned} \bigl\vert ae^{-a}-be^{-b} \bigr\vert \leq \frac{1}{e^{2}} \vert a -b \vert \quad\text{for all } a,b\in [\kappa, + \infty ), \end{aligned}$$
(3.7)

which, together with (3.2), (3.3), (3.4), (3.6), and (3.7), results in

$$\begin{aligned} 0\leq{}& D^{-}\bigl(M (Q_{*})\bigr) \\ \leq{}& {-}\beta (Q_{*}) \bigl\vert z (Q_{*} ) \bigr\vert e^{\lambda Q_{*}}+\sum^{m}_{j=1} \frac{ \alpha _{ j}(Q_{*})}{\gamma _{ j}(Q_{*})} \bigl\vert \gamma _{ j}(Q_{*})x^{\varphi } \bigl(Q_{*}-\sigma _{j}(Q_{*})\bigr) \\ &{} \times e^{-\gamma _{ j}(Q_{*})x^{\varphi }(Q_{*}-\sigma _{ j}(Q_{*}))} \\ &{} -\gamma _{j}(Q_{*})x^{\psi } \bigl(Q_{*}-\sigma _{j}(Q_{*}) \bigr)e^{-\gamma _{j}(Q _{*})x^{\psi } (Q_{*}-\sigma _{ j}(Q_{*}))} \bigr\vert e^{\lambda Q_{*}}+\lambda \bigl\vert z (Q_{*}) \bigr\vert e^{\lambda Q_{*}} \\ \leq{}& {-}\bigl[\beta (Q_{*})-\lambda \bigr] \bigl\vert z (Q_{*} ) \bigr\vert e^{\lambda Q_{*}} \\ &{} +\sum^{m}_{j=1}\frac{\alpha _{ j}(Q_{*})}{\gamma _{ j}(Q_{*})} \frac{1}{e ^{2}} \bigl\vert z \bigl(Q_{*}-\sigma _{ j}(Q_{*})\bigr) \bigr\vert e^{\lambda (Q_{*}-\sigma _{ j}(Q _{*}))}e^{\lambda \sigma _{ j}(Q_{*}) } \\ \leq {}&\Biggl\{ -\bigl[\beta (Q_{*})-\lambda \bigr] +\sum ^{m}_{j=1}\frac{\alpha _{ j}(Q _{*})}{\gamma _{ j}(Q_{*})} \frac{1}{e^{2}} e^{\lambda \sigma }\Biggr\} K_{ \varphi, \psi } \end{aligned}$$

and

$$\begin{aligned} 0 \leq -\bigl[\beta (Q_{*})-\lambda \bigr] +\sum ^{m}_{j=1}\frac{\alpha _{ j}(Q _{*})}{\gamma _{ j}(Q_{*})} \frac{1}{e^{2}} e^{\lambda \sigma }< 0, \end{aligned}$$

which is a contradiction, validating (3.5). This implies that (3.1) holds, and the proof of the Proposition 3.1 is now finished. □

Theorem 3.1

Under the assumptions of Proposition 3.1, system (1.1) has exactly one globally exponentially stable positiveT-periodic solution \(x^{*}(t)\in [\frac{\kappa }{\gamma ^{-}}, A]\).

Proof

According to Proposition 3.1 and Lemma 2.3, one can follow the argument of Theorem 3.1 in [1] to demonstrate that \(x(t+qT)=x(t+qT;t _{ 0},\varphi )\) is not only convergent on every compact interval as \(q\to +\infty \), but also converges uniformly to a continuous function \(x^{*}(t)\), where \(x^{*}\) is a T-periodic solution of (1.1), and such that

$$\begin{aligned} 0< \frac{\kappa }{\gamma ^{-}} \leq x^{*}(t) \leq A , \quad\text{for all } t \in \mathbb{R}. \end{aligned}$$

Furthermore, by applying a similar argument as in Lemma 2.3, we can validate the global exponential stability of \(x^{*}(t)\). This completes the proof of Theorem 3.1. □

By applying Theorem 3.1, we can obtain the following result.

Corollary 3.1

Assume that \(\beta \in (0, +\infty )\)and \(\sigma _{j}, \alpha _{j}\in [0, +\infty )\)are constants, and

$$\begin{aligned} e< \sum_{j=1}^{m} \frac{\alpha _{j} }{\beta } < e^{2}. \end{aligned}$$
(3.8)

Then, the classical autonomous Nicholson’s blowflies equation,

$$\begin{aligned} x'(t)=-\beta x(t) +\sum_{j=1}^{m} \alpha _{j}x\bigl(t-\sigma _{j} (t) \bigr)e ^{- x(t-\sigma _{j}(t))}, \end{aligned}$$

has a globally exponentially stable positive equilibrium point \(x^{*}\in [ \kappa, A]\), where

$$\begin{aligned} \delta = \frac{1}{ \sum_{j=1}^{m} \frac{\alpha _{j} }{\beta }},\quad A>1,\quad \textit{and}\quad \delta =Ae^{-A}. \end{aligned}$$
(3.9)

Remark 3.1

Under the conditions (3.8), the stability of the classical autonomous Nicholson’s blowflies equation in the main results of [10, 11] can be concluded from the above Corollary 3.1. In addition, in [10, 11], the exponential stability and existence range of a positive equilibrium point have not been considered for the classical autonomous Nicholson’s blowflies equation with the conditions (3.8), which implies that the obtained results of this present paper improve and complement some existing ones.

4 Example

In this section, we present a numerical example to verify the theoretical results derived in the previous section.

Example 4.1

Consider the delayed Nicholson’s blowflies equations described by

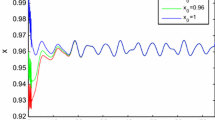
$$\begin{aligned} x'(t) ={}&{-}\bigl(10\sin ^{2}t+2\bigr)x(t) \\ &{} +2\bigl(10\sin ^{2}t+2\bigr) (1.1+0.01\cos t)x\bigl(t- 100\sin ^{2}t \bigr) \\ &{} \times e^{ -(0.9+0.01\sin t)x(t-100\sin ^{2}t )} \\ &{} + \bigl(10\sin ^{2}t+2\bigr) (1.1+0.01\cos 20t)x\bigl(t- 100\cos ^{2}t \bigr) \\ &{} \times e^{ -(0.9+0.01\cos t)x(t-100\cos ^{2}t )}. \end{aligned}$$
(4.1)

Obviously, it is observed that (4.1) satisfies (2.2) and (2.3). Therefore, from Theorem 3.1, one can see that (4.1) has exactly one globally exponentially stable positive 2π-periodic solution. This fact is also supported by the numerical simulations in Fig. 1 (numerical solutions of (4.1) for different initial values).

Figure 1
figure 1

Numerical solutions on the state trajectories of state variables x of system (4.1) for differential initial values: \(\varphi (s) =0.9, 1, 1.2, s\in [-100,0]\)

Full size image

Remark 4.1

It should be pointed out that in (4.1),

$$\begin{aligned} \gamma _{1}(t)=0.9+0.01\sin t< 1 \quad\text{and} \quad\gamma _{2}(t)=0.9+0.01 \cos t< 1 \end{aligned}$$

do not satisfy assumption (1.2) mentioned in Sect. 1. In addition, the results concerning on population dynamics in [12–16] give no clue about the problem of periodic solutions of Nicholson’s blowflies model without assumption (1.2). This implies that all the results in [1–6, 10–57] cannot be used to show the global exponential stability on the positive periodic solution of system (4.1).

5 Conclusions

In this paper, we combine the Lyapunov function method with the differential inequality method to establish some new criteria ensuring the existence and exponential stability of positive periodic solutions for a class of Nicholson’s blowflies equation with multiple time-varying delays. Avoiding the assumption that the maximum reproduction rate is less than 1, these criteria are obtained without assuming that \([\kappa, \widetilde{\kappa }] \approx [0.7215355, 1.342276] \) is the existence region of periodic solutions, and the analogous results in the recently published literature are summarized and refined. The approach presented in this article can be used as a possible way to study other population models involving multiple time-varying delays, for example, neoclassical growth model, Mackey–Glass model, epidemical system or age-structured population model, and so on.