1 Introduction

Just as pointed out by Berezansky and Braverman [1], in the study of mathematical biology, many models of population dynamics can be characterized by the following delayed differential equation:

$$ x '(t)=\sum^{m}_{j=1}F_{j} \bigl(t, x\bigl(t-\tau _{1}(t)\bigr),\ldots ,x\bigl(t-\tau _{l}(t)\bigr)\bigr)-G\bigl(t, x(t)\bigr),\quad t \geq t_{0}, $$
(1.1)

where m and l are positive integers, \(F_{j}\) and G are nonnegative continuous functions. Here the functions \(F_{j}\) describe productions incorporating delay, and G corresponds to the instantaneous mortality. Clearly, (1.1) includes the modified Nicholson’s blowflies model with a nonlinear density-dependent mortality term

$$ x' (t)= -\frac{a(t)x(t)}{b(t)+x(t)} +\sum _{j=1}^{m}\beta _{j}(t) x \bigl(t-h_{j} (t) \bigr)e^{-\gamma _{j}(t) x(t-g_{j}(t))},\quad t \geq t_{0}, $$
(1.2)

which in the case \(h_{j}\equiv g_{j}\) coincides with the classical models [2–6]; \(\frac{a(t)x(t)}{b(t)+x(t)}\) is the death rate of the population which depends on time t and the current population level \(x (t)\), \(\beta _{j}(t) x (t-h_{j} (t) )e^{-\gamma _{j}(t) x(t-g_{j}(t))}\) is the time-dependent birth function which involves maturation delay \(h_{ j}(t)\) and incubation delay \(g_{ j}(t)\), and reproduces at its maximum rate \(\frac{1}{\gamma _{ j}(t)}\); \(a(t)\), \(b(t)\), \(\beta _{j}(t)\), \(g _{j}(t)\), \(h _{j}(t)\), and \(\gamma _{j}(t) \) are all nonnegative, continuous and bounded functions; \(a(t)\), \(b(t) \) and \(\gamma _{j}(t) \) are bounded below by positive constants, and \(j\in I:=\{1,2,\ldots , m \}\).

For the past decade or so, for the special case of (1.1) with \(h_{j}\equiv g_{j} (j\in I)\), the existence of positive solutions, permanence, oscillation, periodicity, and stability of such equations and similar models have been studied extensively [7–14]. In particular, the authors in [1] illustrated that two or more delays involved in the same nonlinear function \(F_{j}\) can lead to chaotic oscillations, and they also gave some examples to show that having two delays instead of one can produce sustainable oscillations. In fact, if two or more delays occur, the time delay feedback function \(F_{j}\) should be considered as a function of several variables. This will add difficulty when studying the dynamics of (1.1) and (1.2). So far, results of global stability analysis for models (1.1) and (1.2) involving two or more delays are very few, we only find that the global stability results of Mackey–Glass equation with two different delays

$$ x '(t)=r(t)\biggl[\frac{ax(t-h(t))}{1+x^{\nu }(t-g(t))}-x(t)\biggr],\quad a>1, \nu > 0, t \geq t_{0}, $$
(1.3)

are established under the additional technical conditions on the delay terms [1].

Most recently, Győri et al. [15] established the permanence in the following two constant-delay differential equation:

$$ x '(t) = \alpha (t)H\bigl(x(t-\sigma ), x(t - \tau )\bigr)-\beta (t)f\bigl( x(t)\bigr). $$
(1.4)

On the other hand, El-Morshedy and Ruiz-Herrera [16] used the classical approach of “decomposing + embedding” to derive some criteria to guarantee the global attraction to a positive equilibrium for the autonomous equation

$$ x '(t) = -\beta x(t) + \beta F\bigl(x(t-\sigma ), x(t - \tau ) \bigr), $$
(1.5)

where \(\beta , \sigma , \tau \in (0, +\infty )\), and \(\sigma \leq \tau \). However, as in [1–14], the above two works shed no light on the global stability on the modified Nicholson’s blowflies model (1.2).

For convenience, given a bounded continuous function g defined on \(\mathbb{R}\), let \(g^{+}\) and \(g^{-}\) be defined as

$$ g^{+}=\sup_{t\in [t_{0}, +\infty )}g(t),\qquad g^{-}=\inf _{t\in [t_{0}, +\infty )}g(t). $$

It should be mentioned that some delay-independent criteria ensuring the global asymptotic stability of for the Nicholson’s blowflies model (1.2) with \(h_{j}\equiv g_{j} (j\in I)\) have been established in [17]. More precisely, the author in [17] obtained the main result as follows.

Theorem 1.1

Suppose that

$$ \textstyle\begin{cases} \max_{ j \in I}\gamma _{j}^{+} \leq 1 , \\ \sup_{t\in R} \sum_{j=1}^{m} \frac{\beta _{j} (t )}{ \gamma _{j} (t ) } < \frac{a ^{-}}{\max \{1, b^{+} \}}, \\ \varlimsup_{t\rightarrow +\infty }\sum_{j=1}^{m}\frac{ \beta _{j}(t )}{\gamma _{j} (t )} \frac{1}{e}< \frac{a^{-} }{b^{+ }+1}. \end{cases} $$
(1.6)

Then 0 is a globally asymptotically stable equilibrium point on \(C([-\tau , 0], (0, +\infty )) \), where \(\tau :=\max \{\max_{1\leq j \leq m} g_{j}^{+}, \max_{1\leq j \leq m} h _{j}^{+} \} >0 \).

Unfortunately, there are some mistakes in the proof of main results in [17]. In fact, in lines 3–4 of page 856 in [17], letting \(t\rightarrow \eta (\varphi )\) cannot lead to \(\varlimsup_{t\rightarrow +\infty } \sum_{j=1} ^{m} \frac{\beta _{j }(t)}{\gamma _{j}(t)a(t)} \frac{1}{e} \geq 1 \) since \(\eta (\varphi )=+\infty \) has not been proved. We find that the conclusion of Theorem 1.1 is correct, and the above mistake can be corrected. This has been done in the first half of the proof of Lemma 2.1; please see Sect. 2.

Inspired by the above discussions, in this paper, we consider the nonlinear density-dependent mortality Nicholson’s blowflies model with multiple pairs of time-varying delays described in (1.2). Here, we develop an approach based on differential inequality techniques coupled with an application of the Fluctuation Lemma to establish a delay-independent criterion to ensure the global asymptotic stability of (1.2) in the important, yet difficult case where the two delays are asymptotically apart, i.e., \(h_{j}\not \equiv g_{j}\) (\(j\in I\)). The obtained results have not been investigated till now. Moreover, the proposed results extend and improve all known ones in [17], and the error mentioned above has been corrected. In particular, our analysis can also be applied to the nonautonomous Mackey–Glass equation, and our work partially solves an open problem posed for the Mackey–Glass equation in [1].

2 Preliminary results

We first recall some notions. Let \(C= C([-\tau , 0], \mathbb{R})\) be the Banach space of all continuous functions from \([-\tau , 0]\) to \(\mathbb{R}\) equipped with the supremum norm \(\|\cdot \|\) and \(C_{+}= C([-\tau , 0], [0, +\infty ))\). Let \(t_{0}\in \mathbb{R}\). Then for a continuous function \(x:[t_{0}-\tau ,t_{0}+\sigma )\to \mathbb{R}\) with \(\sigma >0\) and \(t\in [t_{0},t_{0}+\sigma )\), \(x_{t}\in C\) is defined by \(x_{t}(\theta )=x(t+\theta )\) for \(\theta \in [-\tau , 0]\). Denote by \(x_{t}( t_{0}, \varphi )\) (\(x(t; t _{0}, \varphi )\)) a solution of (1.2) with the initial condition

$$ x_{t_{0}}=\varphi ,\quad \varphi \in C_{+}. $$
(2.1)

In addition, let \([t_{0},\eta (\varphi ))\) be the maximal right-interval of the existence of \(x_{t}(t_{0}, \varphi )\).

Lemma 2.1

Assume that

$$ \varlimsup_{t\rightarrow +\infty } \sum_{j=1}^{m} \frac{ \beta _{j }(t)}{\gamma _{j}(t)a(t)} \frac{1}{e} < 1, $$
(2.2)

as well as

$$ g_{j}(t)\geq h_{j}(t), \quad \textit{and}\quad \lim _{t\rightarrow +\infty }\bigl( g_{j}(t)-h_{j}(t)\bigr) e^{\int _{t_{0}}^{t}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}=0, $$
(2.3)

where \(t\in [t_{0},+\infty )\), \(j\in I\), hold. Then, the solution \(x (t)=x(t; t_{0}, \varphi )\geq 0\)for all \(t\in [t_{0}, \eta (\varphi ))\), the set of \(\{x_{t}(t_{0},\varphi ): t\in [t_{0}, \eta (\varphi ))\}\)is bounded, and \(\eta (\varphi )=+\infty \).

Proof

From Theorem 5.2.1 in [18], we obtain \(x (t)=x(t; t _{0}, \varphi )\geq 0\) for all \(t \in [t_{0}, \eta (\varphi ))\). Now, we show that \(\eta (\varphi )=+\infty \). For all \(t\in [t_{0}, \eta (\varphi ))\), defining \(y(t)=\max_{t_{0}-\tau \leq s \leq t} x (s)\), we gain

$$ x' (t)\leq \sum_{j=1}^{m} \beta _{j}(t) x \bigl(t-h_{j} (t) \bigr) \leq \sum _{j=1}^{m}\beta _{j} (t) y (t), $$

and

$$ x (t)\leq x (t_{0}) + \int _{t_{0}}^{t} \Biggl[ \sum _{j=1}^{m}\beta _{j} (v) y (v) \Biggr]\,dv \leq \Vert \varphi \Vert + \int _{t_{0}}^{t}\Biggl(\sum _{j=1} ^{m}\beta _{j} (v)\Biggr)y (v) \,dv, $$

which suggests that

$$ y (t)\leq \Vert \varphi \Vert + \int _{t_{0}}^{t}\Biggl(\sum _{j=1}^{m}\beta _{j} (v)\Biggr) y (v) \,dv,\quad \forall t\in \bigl[t_{0}, \eta (\varphi )\bigr). $$

Hence, by the Gronwall–Bellman inequality, we obtain

$$ x (t)\leq y (t)\leq \Vert \varphi \Vert e^{\int _{t_{0}}^{t}(\sum _{j=1} ^{m}\beta _{j} (v)) \,dv} ,\quad \forall t\in \bigl[t_{0}, \eta (\varphi )\bigr). $$

This follows from Theorem 2.3.1 in [19] that \(\eta ( \varphi )=+\infty \), and then

$$ x (t)\leq y (t)\leq \Vert \varphi \Vert e^{\int _{t_{0}}^{t}(\sum _{j=1} ^{m}\beta _{j} (v)) \,dv} , \quad \forall t\in [t_{0}, +\infty ). $$
(2.4)

Furthermore, for each \(t\in [t_{0}-\tau , +\infty ) \), we define

$$ M(t)=\max \Bigl\{ \xi :\xi \leq t, x (\xi )=\max_{t_{0}-\tau \leq s\leq t} x (s) \Bigr\} . $$

Next, we show that \(x (t)\) is bounded on \([t_{0}, \eta (\varphi ))\). Assume on the contrary that

$$ \lim_{t\rightarrow +\infty }x \bigl(M(t)\bigr)=+\infty \quad \mbox{and}\quad \lim _{t\rightarrow +\infty }M(t)=+\infty . $$
(2.5)

Let \(T^{*}\ge t_{0}\) be such that \(M(t)\ge t_{0}+\tau \) for \(t\ge T^{*}\). Note that for \(t\ge t_{0}\), it follows from (1.2) that

$$ x'(s) \le \sum_{j=1}^{m} \beta _{j}(s)x\bigl(M(t)\bigr)= \sum_{j=1} ^{m}\beta _{j}(s)y \bigl(M(t)\bigr), $$
(2.6)

for all \(s\in [t_{0}, t]\) and \(t\in [t_{0}, + \infty )\).

This, combined with (1.2), (2.3), (2.4), and the fact that \(\sup_{w\ge 0} we^{-w}=\frac{1}{e}\), gives us

$$\begin{aligned} 0 &\leq x'\bigl(M(t)\bigr) \\ &=-\frac{a(M(t))x(M(t))}{b(M(t))+x(M(t))} \\ &\quad {} +\sum_{j=1}^{m}\beta _{j}\bigl(M(t)\bigr)x\bigl(M(t)-g_{j}\bigl(M(t)\bigr) \bigr)e^{-\gamma _{j}(M(t))x(M(t)-g_{j}(M(T)))} \\ &\quad {} +\sum_{j=1}^{m}\beta _{j}\bigl(M(t)\bigr) \int _{M(t)-g_{j} (M(t))}^{M(t)-h _{j} (M(t))}x '(s)\,ds e^{-\gamma _{j}(M(t)) x(M(t)-g_{j}(M(t)))} \\ &\leq a\bigl(M(t)\bigr)\Biggl\{ \Biggl[-\frac{ x (M(t))}{b(M(t))+x (M(t))} \\ &\quad {} +\sum_{j=1}^{m} \frac{\beta _{j }(M(t))}{\gamma _{j}(M(t))a(M(t))} \gamma _{j}\bigl(M(t)\bigr) x \bigl(M(t)-g _{j} \bigl(M(t)\bigr)\bigr)e^{-\gamma _{j}(M(t)) x(M(t)-g_{j}(M(t)))}\Biggr] \\ &\quad {} +\frac{1}{a(M(t))}\sum_{j=1}^{m} \beta _{j }\bigl(M(t)\bigr) \int _{M(t)-g_{j} (M(t))}^{M(t)-h_{j} (M(t))}\sum_{j=1}^{m} \beta _{j}(s)y \bigl(M(t)\bigr)\,ds\Biggr\} \\ &\leq a\bigl(M(t)\bigr)\Biggl\{ \Biggl[-\frac{x(M(t))}{b(M(t))+x(M(t))}+\sum _{j=1}^{m}\frac{ \beta _{j}(M(t))}{\gamma _{j}(M(t))a(M(t))} \frac{1}{e}\Biggr] \\ &\quad {} +\frac{1}{a(M(t))}\sum_{j=1}^{m} \beta _{j}\bigl(M(t)\bigr)\bigl[\bigl(g_{j}\bigl(M(t) \bigr)-h _{j}\bigl(M(t)\bigr)\bigr)e^{\int _{t_{0}}^{M(t)}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \\ &\quad {} \times \Biggl[\Biggl(\sum_{j=1}^{m} \beta _{j}^{+}\Biggr) \Vert \varphi \Vert \Biggr] \Biggr\} \end{aligned}$$

and

$$\begin{aligned} 0 &\leq \Biggl[-\frac{x(M(t))}{b(M(t))+x(M(t))}+\sum_{j=1}^{m} \frac{\beta _{j}(M(t))}{\gamma _{j}(M(t))a(M(t))}\frac{1}{e}\Biggr] \\ &\quad {} +\frac{1}{a(M(t))}\sum_{j=1}^{m} \beta _{j}\bigl(M(t)\bigr)\bigl[\bigl(g_{j}\bigl(M(t) \bigr)-h _{j}\bigl(M(t)\bigr)\bigr)e^{\int _{t_{0}}^{M(t)}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \\ &\quad {} \times \Biggl[\Biggl(\sum_{j=1}^{m} \beta _{j}^{+}\Biggr) \Vert \varphi \Vert \Biggr], \end{aligned}$$
(2.7)

where \(M(t)>2\tau +t_{0}\).

Letting \(t\rightarrow +\infty \), due to the facts

$$ \lim_{t\rightarrow +\infty }\bigl( g_{j}(t)-h_{j}(t) \bigr) e^{\int _{t _{0}}^{t}(\sum _{j=1}^{m}\beta _{j} (v)) \,dv}=0 \quad \mbox{and}\quad \lim_{t\rightarrow +\infty }M(t)=+\infty , $$

inequality (2.7) yields

$$ 0 \leq -1 +\varlimsup_{t\rightarrow +\infty }\sum _{j=1}^{m} \frac{\beta _{j }(t)}{\gamma _{j}(t)a(t)} \frac{1}{e}, $$

which contradicts assumption (2.2). This implies that \(x (t)\) is bounded on \([t_{0}, +\infty )\), and ends the proof of Lemma 2.1. □

3 Main result

Theorem 3.1

Assume that (2.3) and

$$ \textstyle\begin{cases} \max_{1\leq j \leq m}\varlimsup_{t\rightarrow + \infty }\gamma _{j}(t) \leq 1,\qquad \sup_{t\in [t_{0}, +\infty )} \sum_{j=1}^{m} \frac{\beta _{j }(t)\max \{1, b(t ) \}}{ a(t)} < 1, \\ \varlimsup_{t\rightarrow +\infty } \sum_{j=1}^{m} \frac{\beta _{j} (t )(1+ b (t ))}{ a (t )\gamma _{j} (t ) } \frac{1}{e}< 1,\qquad \varlimsup_{t\rightarrow +\infty } \sum_{j=1}^{m} \frac{ \beta _{j} (t )\max \{1, b (t )\}}{ a (t )\gamma _{j} (t ) } < 1 . \end{cases} $$
(3.1)

are satisfied. Then 0 is a globally asymptotically stable equilibrium point on \(C_{+} \).

Proof

Let \(x (t)=x(t; t_{0}, \varphi )\). From Lemma 2.1, one can see that the set \(\{x_{t}(t_{0}, \varphi ): t\in [t_{0}, +\infty )\}\) is bounded, and \(0\leq u = \limsup_{t\rightarrow +\infty } x (t ) <+\infty \).

We now prove that 0 is a stable equilibrium point. Without loss of generality, let \(0<\epsilon <1\) satisfy

$$ \sup_{t\in [t_{0}, +\infty )}\sum_{j=1}^{m} \frac{ \beta _{j }(t)\max \{1, b(t ) \}}{ a(t)} < e^{-\epsilon } . $$
(3.2)

Choosing \(0<\delta <\epsilon \), we claim that, for \(\|\varphi \|< \delta \),

$$ x(t)=x(t; t_{0}, \varphi )< \epsilon \quad \mbox{for all } t\in [t _{0}, +\infty ). $$
(3.3)

We can pick \(t_{*}\in (t_{0}, +\infty )\) such that

$$ x(t_{*})=\epsilon , x(t)< \epsilon\quad \mbox{for all } t\in [t_{0}-\tau , t_{*}) . $$
(3.4)

Noting that

$$ b(t)+x \leq \max \bigl\{ 1, b(t) \bigr\} e^{ x}\quad \mbox{for all } (t, x) \in [t_{0}, +\infty )\times [0, +\infty ) , $$
(3.5)

(1.2), (3.2) and (3.4) result in

$$\begin{aligned} 0 &\leq x'(t_{*}) \\ &= -\frac{a(t_{*})x(t_{*})}{b(t_{*})+x(t_{*})} +\sum_{j=1} ^{m} \beta _{j} (t_{*}) x \bigl(t_{*}-h_{j}(t_{*}) \bigr)e^{- \gamma _{j} (t_{*}) x (t_{*}-g_{j}(t_{*}))} \\ &\leq a(t_{*}) \Biggl\{ - \frac{1}{\max \{1, b(t_{*}) \}} x(t_{*})e ^{-x(t_{*})} +\sum_{j=1}^{m} \frac{\beta _{j} (t_{*})}{a(t_{*})} \Biggr\} \\ &= a(t_{*}) \Biggl\{ - \frac{1}{\max \{1, b(t_{*}) \}} \epsilon e^{- \epsilon } +\sum_{j=1}^{m} \frac{\beta _{j} (t_{*})}{a(t_{*}) } \epsilon \Biggr\} \\ &= a(t_{*})\frac{1}{\max \{1, b(t_{*}) \}} \Biggl\{ - e^{- \epsilon } + \sum_{j=1}^{m} \frac{\beta _{j }(t_{*})\max \{1, b(t_{*}) \}}{ a(t _{*})} \Biggr\} \epsilon \\ &< 0, \end{aligned}$$

which is a contradiction, so that (3.3) holds. Thus, 0 is a stable equilibrium point.

Hereafter, it is sufficient to show that \(u =\limsup_{t\rightarrow +\infty } x (t ) =0\). By the Fluctuation Lemma [20, Lemma A.1], there exists a sequence \(\{t_{k} \} _{k\geq 1}\) such that

$$ t_{k}\to +\infty ,\quad x (t_{k})\to u, \qquad x '(t_{k})\to 0\quad \mbox{as }k\to +\infty . $$

Moreover, from (3.1) and the boundedness of the coefficients and delay functions in (1.2), without loss of generality, we can assume that

$$\begin{aligned} &\lim_{k\rightarrow + \infty }a(t_{k})=a^{*}\in \bigl[a^{-},a^{+}\bigr],\qquad \lim_{k\rightarrow + \infty }b(t_{k})=b^{*} \in \bigl[b^{-},b^{+}\bigr], \\ &\lim_{k\rightarrow + \infty }\beta _{j}(t_{k})=\beta _{j}^{*} \in \bigl[\beta _{j}^{-}, \beta _{j}^{+}\bigr],\qquad \lim_{k\rightarrow + \infty }\gamma _{j}(t_{k})=\gamma _{j}^{*} \in \bigl[\gamma _{j}^{-},\gamma _{j}^{+} \bigr],\quad j \in I, \\ &\lim_{k\rightarrow + \infty }g_{j}(t_{k})=g_{j}^{*} \in \bigl[g _{j}^{-},g_{j}^{+} \bigr],\qquad \lim_{k\rightarrow + \infty }h_{j}(t_{k})=h _{j}^{*}\in \bigl[h_{j}^{-},h_{j}^{+} \bigr],\quad j\in I, \\ &\lim_{k \rightarrow + \infty }\gamma _{j}(t_{k}) x \bigl(t_{k}-g _{j} (t_{k})\bigr)= \mu _{j}^{*} \in [ 0, u ], \quad j\in I, \\ &\lim_{k \rightarrow + \infty } \sum_{j=1}^{m} \frac{ \beta _{j }(t_{k})(1+b(t_{k})) }{\gamma _{j}(t_{k})a(t_{k})} \frac{1}{e}=\sum_{j=1}^{m} \frac{\beta ^{*} _{j}( b ^{*}+1 )}{ \gamma ^{*} _{j} a^{*}}\frac{1}{e}\leq \varlimsup_{t\rightarrow +\infty } \sum_{j=1}^{m} \frac{\beta _{j} (t )(1+ b (t ))}{ a (t )\gamma _{j} (t ) } \frac{1}{e}< 1, \end{aligned}$$

and

$$ \lim_{k \rightarrow + \infty } \sum_{j=1}^{m} \frac{\beta _{j}(t _{k})\max \{1, b (t_{k}) \}}{\gamma _{j}(t_{k})a(t_{k})}=\sum_{j=1}^{m} \frac{\beta ^{*} _{j}\max \{1, b ^{*} \}}{a^{*}\gamma ^{*} _{j}} \leq \varlimsup_{t\rightarrow +\infty } \sum _{j=1}^{m} \frac{\beta _{j} (t )\max \{1, b (t )\}}{ a (t ) \gamma _{j} (t ) } < 1. $$

Furthermore, from (1.2), (2.3), (2.4), we get

$$\begin{aligned} x '(t_{k}) &= -\frac{a(t_{k})x(t_{k})}{b(t_{k})+x(t_{k})} \\ &\quad {} +\sum_{j=1}^{m} \beta _{j }(t_{k}) x \bigl(t_{k}-g_{j} (t _{k})\bigr)e^{-\gamma _{j}(t_{k}) x(t_{k}-g_{j}(t_{k}))} \\ &\quad {} +\sum_{j=1}^{m} \beta _{j }(t_{k}) \int _{t_{k}-g_{j} (t_{k})}^{t_{k}-h_{j} (t_{k})}x '(s)\,ds e^{-\gamma _{j}(t_{k}) x(t_{k}-g_{j}(t_{k}))} \\ &\leq a(t_{k})\Biggl[-\frac{x(t_{k})}{b(t_{k})+x(t_{k})} \\ &\quad {} +\sum_{j=1}^{m} \frac{\beta _{j }(t_{k})}{\gamma _{j}(t _{k})a(t_{k})} \gamma _{j}(t_{k}) x \bigl(t_{k}-g_{j} (t_{k})\bigr)e^{-\gamma _{j}(t _{k}) x(t_{k}-g_{j}(t_{k}))}\Biggr] \\ &\quad {} +\sum_{j=1}^{m} \beta _{j }(t_{k}) \int _{t_{k}-g_{j} (t_{k})}^{t_{k}-h_{j} (t_{k})}\sum_{j=1}^{m} \beta _{j}(s)y (t_{k})\,ds \\ &\leq a(t_{k})\Biggl[-\frac{x(t_{k})}{b(t_{k})+x(t_{k})} \\ &\quad {} +\sum_{j=1}^{m} \frac{\beta _{j }(t_{k})}{\gamma _{j}(t _{k})a(t_{k})} \gamma _{j}(t_{k}) x \bigl(t_{k}-g_{j} (t_{k})\bigr)e^{-\gamma _{j}(t_{k}) x(t_{k}-g_{j}(t_{k}))}\Biggr] \\ &\quad {}+\sum_{j=1}^{m} \beta _{j }(t_{k}) \bigl[\bigl(g_{j}(t_{k})-h_{j}(t_{k}) \bigr) e^{\int _{t_{0}}^{t_{k}}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \Biggl[\Biggl( \sum_{j=1}^{m} \beta _{j} ^{+} \Biggr) \Vert \varphi \Vert \Biggr], \end{aligned}$$

and

$$\begin{aligned} \frac{1}{a(t_{k})}x '(t_{k}) \leq{} &\Biggl[- \frac{x(t_{k})}{b(t_{k})+x(t_{k})} \\ &{}+\sum_{j=1}^{m} \frac{\beta _{j }(t_{k}) }{\gamma _{j}(t_{k})a(t _{k})} \gamma _{j}(t_{k}) x \bigl(t_{k}-g_{j} (t_{k})\bigr)e^{-\gamma _{j}(t_{k}) x(t_{k}-g_{j}(t_{k}))}\Biggr] \\ &{}+\frac{1}{a(t_{k})}\sum_{j=1}^{m} \beta _{j }(t_{k}) \bigl[\bigl(g_{j}(t _{k})-h_{j}(t_{k})\bigr) e^{\int _{t_{0}}^{t_{k}}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \\ &{} \times \Biggl[\Biggl(\sum_{j=1}^{m} \beta _{j} ^{+} \Biggr) \Vert \varphi \Vert \Biggr], \end{aligned}$$
(3.6)

where \(t_{k}>2\tau +t_{0}\).

If \(u\geq 1\), from (2.3), (3.1), (3.6) and the facts that \(\frac{u}{b ^{*}+u}\geq \frac{1}{b ^{*}+1}\) and \(\sup_{u\geq 0} ue^{- u}=\frac{1}{ e}\), letting \(k\rightarrow +\infty \) leads to

$$ 0 \leq -\frac{1}{b ^{*}+1} +\sum_{j=1}^{m} \frac{\beta ^{*} _{j}}{\gamma ^{*} _{j} a^{*}} \frac{1}{e} = \frac{1}{b ^{*}+1} \Biggl\{ -1 + \sum_{j=1}^{m}\frac{\beta ^{*} _{j}( b ^{*}+1 )}{\gamma ^{*} _{j} a^{*}} \frac{1}{e} \Biggr\} < 0, $$

which a contradiction, so that \(0\leq u<1\).

If \(0< u< 1\), from (2.3), (3.1), (3.5), (3.6), and the fact that \(xe^{-x}\) is monotone increasing on \([0,1]\), we have

$$\begin{aligned} & \frac{1}{a(t_{k})}x '(t_{k}) \\ &\quad \leq \Biggl[- \frac{1}{\max \{1, b(t_{k} ) \}}x(t_{k})e^{-x(t_{k})} \\ &\qquad {} +\sum_{j=1}^{m} \frac{\beta _{j }(t_{k})}{\gamma _{j}(t_{k})a(t _{k})} \gamma _{j}(t_{k}) x \bigl(t_{k}-g_{j} (t_{k})\bigr)e^{-\gamma _{j}(t_{k}) x(t_{k}-g_{j}(t_{k}))}\Biggr] \\ &\qquad {} +\frac{1}{a(t_{k})}\sum_{j=1}^{m} \beta _{j }(t_{k}) \bigl[ \bigl(g_{j}(t _{k})-h_{j}(t_{k})\bigr) e^{\int _{t_{0}}^{t_{k}}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \Biggl[ \Biggl(\sum_{j=1}^{m} \beta _{j} ^{+} \Biggr) \Vert \varphi \Vert \Biggr] \\ &\quad = \frac{1}{\max \{1, b(t_{k} ) \}} [- x(t_{k})e^{-x(t_{k})} \\ &\qquad {} +\frac{1}{a(t_{k})}\sum_{j=1}^{m} \beta _{j }(t_{k}) \bigl[ \bigl(g_{j}(t _{k})-h_{j}(t_{k})\bigr) e^{\int _{t_{0}}^{t_{k}}(\sum _{j=1}^{m}\beta _{j}(v))\,dv}\bigr] \Biggl[ \Biggl(\sum_{j=1}^{m} \beta _{j} ^{+} \Biggr) \Vert \varphi \Vert \Biggr] , \end{aligned}$$

where \(t_{k}>2\tau +t_{0}\), and

$$\begin{aligned} 0 &\leq \frac{1}{\max \{1, b ^{*} \}} \Biggl[ -ue^{-u} +\sum _{j=1} ^{m}\frac{\beta ^{*}_{j}\max \{1,b ^{*}\}}{a^{*}\gamma ^{*} _{j}} \mu ^{*}_{j} e^{-\mu ^{*}_{ j}} \Biggr] \\ & \leq \frac{1}{\max \{1, b ^{*} \}} \Biggl[ -u e^{-u} +\sum _{j=1} ^{m}\frac{\beta ^{*} _{j}\max \{1, b ^{*} \}}{a^{*}\gamma ^{*} _{j}} ue ^{-u} \Biggr] \\ &= \frac{1}{\max \{1, b ^{*} \}} \Biggl[ -1 +\sum_{j=1}^{m} \frac{ \beta ^{*} _{j}\max \{1, b ^{*} \}}{a^{*}\gamma ^{*} _{j}} \Biggr]ue^{-u} \\ &\leq 0, \end{aligned}$$

which is also a contradiction, proving that \(u=0\). This completes the proof of Theorem 3.1. □

By applying Theorem 3.1, we can obtain the following result.

Corollary 3.1

Suppose that (3.1) holds, and \(g_{j}(t)\equiv h_{j}(t)\), for all \(t\in [t_{0}, +\infty )\), \(j\in I\). Then 0 is a globally asymptotically stable equilibrium point on \(C_{+} \).

Remark 3.1

It is obvious that all results in Theorem 1.1 are special cases of Corollary 3.1 since the assumptions adopted are weaker than those ones in Theorem 1.1.

4 A numerical example

This section presents an example with graphical illustration to show the applicability of the analytical results derived in this article.

Example 4.1

Consider the following nonautonomous Nicholson’s blowflies equation with two pairs of different time-varying delays:

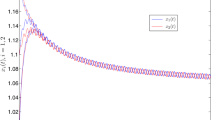
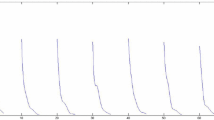
$$\begin{aligned} x' (t) = {}&{-}\frac{(3 + \vert \cos \sqrt{2} t \vert ) x (t)}{(5.1 + \frac{t^{4}}{t ^{4}+1} ) +x (t)} \\ &{}+\frac{1}{200}\bigl(1 + \sin ^{2} t \bigr) x \bigl(t-2e^{ \vert \arctan t \vert }\bigr) \\ &{} \times e^{-\frac{1+23e^{-t}}{12}(1+ \vert \arctan t \vert )x (t-2e^{ \vert \arctan t \vert }-100e^{-2t})} \\ &{}+\frac{1}{200}\bigl(1 + \sin ^{2} 2t \bigr) x \bigl(t-2e^{ \vert \arctan t \vert }\bigr) \\ &{} \times e^{-\frac{1+25e^{-t}}{13}(1+ \vert \arctan t \vert )x (t-2e^{ \vert \arctan t \vert }-150e^{-2t})}, \quad t\geq t_{0}=0. \end{aligned}$$
(4.1)

Obviously, it is easy to check that assumptions (2.3) and (3.1) are satisfied in (4.1). Hence, from Theorem 3.1, we have that the zero equilibrium point for the model (3.1) is globally asymptotically stable on \(C_{+} = C([-(2e^{ \frac{\pi }{2}}+150), 0], [0, +\infty ) )\). Figure 1 supports this result with the numerical solutions involving different initial values.

Figure 1
figure 1

Trajectories of system (4.1) involving differential initial values

Full size image

Remark 4.1

It should be mentioned that the global asymptotic stability on the Nicholson’s blowflies model involving nonlinear density-dependent mortality terms and multiple pairs of time-varying delays has not been touched in the previous literature. As for [1–17, 21–51], the authors still give no clues on the global asymptotic stability of the Nicholson’s blowflies model involving multiple pairs of time-varying delays. One can see that all the results in the above mentioned references cannot be applied to prove that all the solutions of model (4.1) converge to the zero equilibrium.

5 Conclusions

In this article, the global asymptotic stability of the zero equilibrium for a Nicholson’s blowflies model involving nonlinear density-dependent mortality terms and multiple pairs of time-varying delays is established. To the best of our knowledge, this is the first paper to study the global dynamics for the nonlinear density-dependent mortality Nicholson’s blowflies model involving multiple pairs of time-varying delays and the obtained results are new. Some sufficient conditions set up here are easily verified and these conditions are independent of the multiple pairs of time-varying delays in the addressed model, which improves and complements some existing results mentioned in the Introduction. Moreover, the method used in this paper provides a possible approach for studying the global asymptotic stability of other population dynamic models involving multiple pairs of time-varying delays.