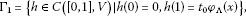Existence of positive solutions of elliptic mixed boundary value problem

Abstract

In this paper, we use variational methods to prove two existence of positive solutions of the following mixed boundary value problem:

$$\{\begin{array}{cc}-\mathrm{\Delta }u=f(x,u),\hfill & x\in \mathrm{\Omega },\hfill \\ u=0,\hfill & x\in \sigma ,\hfill \\ \frac{\partial u}{\partial \nu }=g(x,u),\hfill & x\in \mathrm{\Gamma }.\hfill \end{array}$$

One deals with the asymptotic behaviors of $f(x,u)$ near zero and infinity and the other deals with superlinear of $f(x,u)$ at infinity.

MSC:35M12, 35D30.

1 Introduction and preliminaries

This paper is concerned with the existence of positive solutions of the following elliptic mixed boundary value problem:

$$\{\begin{array}{cc}-\mathrm{\Delta }u=f(x,u),\hfill & x\in \mathrm{\Omega },\hfill \\ u=0,\hfill & x\in \sigma ,\hfill \\ \frac{\partial u}{\partial \nu }=g(x,u),\hfill & x\in \mathrm{\Gamma },\hfill \end{array}$$

(1)

where Ω is a bounded domain in ${\mathbb{R}}^n$ with Lipschitz boundary ∂ Ω, $\sigma \cup \mathrm{\Gamma }=\partial \mathrm{\Omega }$, $\sigma \cap \mathrm{\Gamma }=\mathrm{Ø}$, Γ is a sufficiently smooth $(n-1)$-dimensional manifold, and ν is the outward normal vector on ∂ Ω. We assume $f:\mathrm{\Omega }\times \mathbb{R}\to \mathbb{R}$, $g:\mathrm{\Gamma }\times \mathbb{R}\to \mathbb{R}$ are continuous and satisfy

(S1) $f(x,t)\ge 0$, $\mathrm{\forall }t\ge 0$, $x\in \mathrm{\Omega }$, $f(x,0)=0$. $f(x,t)\equiv 0$, $\mathrm{\forall }t<0$, $x\in \mathrm{\Omega }$.

(S2) For almost every $x\in \mathrm{\Omega }$, $\frac{f(x,t)}{t}$ is nondecreasing with respect to $t>0$.

(S3) ${lim}_{t\to 0}\frac{f(x,t)}{t}=p(x)$, ${lim}_{t\to +\mathrm{\infty }}\frac{f(x,t)}{t}=q(x)\not\equiv 0$ uniformly in a.e. $x\in \mathrm{\Omega }$, where ${\parallel p(x)\parallel }_{\mathrm{\infty }}<{\lambda }_1$, ${\lambda }_1$ is the first eigenvalue of (2), $0\le p(x)$, $q(x)\in L^{\mathrm{\infty }}(\mathrm{\Omega })$.

(S4) There exists $c_1,c_2>0$ such that $|f(x,t)|\le c_1+c_2{|t|}^{p-1}$ for some $p\in (2,\frac{2n}{n-2})$ as $n\ge 3$ and $p\in (2,+\mathrm{\infty })$ as $n=1,2$.

The eigenvalue problem of (1) is studied by Liu and Su in [1]

$$\{\begin{array}{cc}-\mathrm{\Delta }u=\lambda u\hfill & \text{in }\mathrm{\Omega },\hfill \\ u=0\hfill & \text{on }\sigma ,\hfill \\ \frac{\partial u}{\partial \nu }=\lambda u\hfill & \text{on }\mathrm{\Gamma }.\hfill \end{array}$$

(2)

There exists a set of eigenvalues $\{{\lambda }_k\}$ and corresponding eigenfunctions $\{u_k\}$ which solve problem (2), where $0\le {\lambda }_1\le {\lambda }_2\le \cdots \le {\lambda }_k\le \cdots$, ${\lambda }_k\to \mathrm{\infty }$ as $k\to \mathrm{\infty }$, ${\lambda }_1={inf}_{0\ne u\in V}\frac{{\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx}{{\int }_{\mathrm{\Omega }}{|u|}^{2}dx+{\int }_{\mathrm{\Gamma }}{|u|}^{2}ds}$.

There have been many papers concerned with similar problems at resonance under the boundary condition; see [2–10]. Moreover, some multiplicity theorems are obtained by the topological degree technique and variational methods; interested readers can see [11–17]. Problem (1) is different from the classical ones, such as those with Dirichlet, Neuman, Robin, No-flux, or Steklov boundary conditions.

In this paper, we assume $V:=\{v\in H^1(\mathrm{\Omega }):v{|}_{\sigma }=0\}$ is a closed subspace of $H^1(\mathrm{\Omega })$. We define the norm in V as ${\parallel u\parallel }^2={\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx+{\int }_{\mathrm{\Gamma }}{|\gamma u|}^{2}ds$, ${\parallel \cdot \parallel }_{L^p(\mathrm{\Omega })}$ is the $L^p(\mathrm{\Omega })$ norm, ${\parallel \cdot \parallel }_{L^p(\mathrm{\Gamma })}$ is the $L^p(\mathrm{\Gamma })$ norm, $\gamma :V\to L^2(\mathrm{\Gamma })$ is the trace operator with $\gamma u=u_{\mathrm{\Gamma }}$ for all $u\in H^1(\mathrm{\Omega })$, that is continuous and compact (see [18]). Furthermore, we define $g=\gamma f$, $0\le g(x,t)\le |\gamma f(x,t)|$ for $t>0$ (see [1]). Then, by (S3), we obtain

$$\underset{t\to +\mathrm{\infty }}{lim}\frac{g(x,t)}{t}\le \underset{t\to +\mathrm{\infty }}{lim}\frac{|\gamma f(x,t)|}{t}=q(x)\not\equiv 0,\text{a.e. }x\in \overline{\mathrm{\Omega }}.$$

(3)

Let Ω be a bounded domain with a Lipschitz boundary; there is a continuous embedding $V\hookrightarrow L^y(\mathrm{\Omega })$ for $y\in [2,\frac{2n}{n-2}]$ when $n\ge 3$, and $y\in [2,+\mathrm{\infty })$ when $n=1,2$. Then there exists ${\gamma }_y>0$, such that

$${\parallel u\parallel }_{L^y(\mathrm{\Omega })}\le {\gamma }_y\parallel u\parallel ,\mathrm{\forall }u\in V.$$

(4)

Moreover, there is a continuous boundary trace embedding $V\hookrightarrow L^z(\mathrm{\Gamma })$ for $z\in [2,\frac{2(n-1)}{n-2}]$ when $n\ge 3$, and $z\in [2,+\mathrm{\infty })$ when $n=1,2$. Then there exists $k_z>0$, such that

$${\parallel u\parallel }_{L^z(\mathrm{\Gamma })}\le k_z\parallel u\parallel ,\mathrm{\forall }u\in V.$$

(5)

It is well known that to seek a nontrivial weak solution of problem (1) is equivalent to finding a nonzero critical value of the $C^1$ functional

$$J(u)=\frac{1}{2}{\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx-{\int }_{\mathrm{\Omega }}F(x,u)dx-{\int }_{\mathrm{\Gamma }}G(s,u)ds,$$

(6)

where $u\in V$, $F(x,u)={\int }_0^{u}f(x,t)dt$, $G(x,u)={\int }_0^{u}g(x,t)dt$. Moreover, by (S1) and the Strong maximum principle, a nonzero critical point of J is in fact a positive solution of (1). In order to find critical points of the functional (6), one often requires the technique condition, that is, for some $\mu >2$, $\mathrm{\forall }|u|\ge M>0$, $x\in \mathrm{\Omega }$,

$$0<\mu F(x,u)\le uf(x,u),F(x,u)={\int }_0^{u}f(x,t)dt.$$

(AR)

It is easy to see that the condition (AR) implies that ${lim}_{u\to +\mathrm{\infty }}\frac{F(x,u)}{u^2}=+\mathrm{\infty }$, that is, $f(x,u)$ must be superlinear with respect to u at infinity. In the present paper, motivated by [19] and [20], we study the existence and nonexistence of positive solutions for problem (1) with the asymptotic behavior assumptions (S3) of f at zero and infinity. Moreover, we also study superlinear of f at infinity with $q(x)\equiv +\mathrm{\infty }$ in (S3), which is weaker than the (AR) condition, that is the (AR) condition does not hold.

In order to get our conclusion, we define the minimization problem

$$\mathrm{\Lambda }=inf\{{\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx:u\in V,{\int }_{\mathrm{\Omega }}q(x)u^{2}dx+{\int }_{\mathrm{\Gamma }}q(s)u^{2}ds=1\},$$

(7)

then $\mathrm{\Lambda }>0$, which is achieved by some ${\phi }_{\mathrm{\Lambda }}\in V$ with ${\phi }_{\mathrm{\Lambda }}(x)>0$ a.e. in Ω; see Lemma 1.

We denote by c, $c_1$, $c_2$ universal constants unless specified otherwise. Our main results are as follows.

Theorem 1 Let conditions (S 1) to (S 3) hold, then:

• (i) If $\mathrm{\Lambda }>1$, then the problem (1) has no any positive solution in V.

• (ii) If $\mathrm{\Lambda }<1$, then the problem (1) has at least one positive solution in V.

• (iii) If $\mathrm{\Lambda }=1$, then the problem (1) has one positive solution $u(x)\in V$ if and only if there exists a constant $c>0$ such that $u(x)=c{\phi }_{\mathrm{\Lambda }}(x)$ and $f(x,u)=q(x)u(x)$, $g(x,u)=q(x)u(x)$ a.e. $x\in \mathrm{\Omega }$, where ${\phi }_{\mathrm{\Lambda }}(x)>0$ is the function which achieves Λ.

Corollary 2 Let conditions (S 1) to (S 3) with $q(x)\equiv l>0$ hold, then:

• (i) If $l<{\lambda }_1$, then the problem (1) has no any positive solution in V.

• (ii) If ${\lambda }_1<l<+\mathrm{\infty }$, then the problem (1) has at least one positive solution in V.

• (iii) If $l={\lambda }_1$, then the problem (1) has one positive solution $u(x)\in V$ if and only if there exists a constant $c>0$ such that $u(x)=c{\phi }_1(x)$ and $f(x,u)={\lambda }_{1}u(x)$, $g(x,u)={\lambda }_{1}u(x)$ a.e. $x\in \mathrm{\Omega }$, where ${\phi }_1(x)>0$ is the eigenfunction of the ${\lambda }_1$.

Theorem 3 Let conditions (S 1) to (S 4) with $q(x)\equiv +\mathrm{\infty }$ hold, then the problem (1) has at least one positive solution in V.

2 Some lemmas

We need the following lemmas.

Lemma 1 If $q(x)\in L^{\mathrm{\infty }}(\mathrm{\Omega })$, $q(x)\ge 0$, $q(x)\not\equiv 0$, then $\mathrm{\Lambda }>0$ and there exists ${\phi }_{\mathrm{\Lambda }}(x)\in V$ such that $\mathrm{\Lambda }={\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }{\phi }_{\mathrm{\Lambda }}|}^{2}dx$ and ${\int }_{\mathrm{\Omega }}q(x){\phi }_{\mathrm{\Lambda }}^{2}dx+{\int }_{\mathrm{\Gamma }}q(s){\phi }_{\mathrm{\Lambda }}^{2}ds=1$. Moreover, ${\phi }_{\mathrm{\Lambda }}(x)>0$ a.e. in V.

Proof By the Sobolev embedding function $V\hookrightarrow L^2(\mathrm{\Omega })$ and Fatou’s lemma, it is easy to know that $\mathrm{\Lambda }>0$ and there exists ${\phi }_{\mathrm{\Lambda }}(x)\in V$, which satisfies Λ, that is, ${\int }_{\mathrm{\Omega }}q(x){\phi }_{\mathrm{\Lambda }}^{2}dx+{\int }_{\mathrm{\Gamma }}q(s){\phi }_{\mathrm{\Lambda }}^{2}ds=1$. Furthermore, we assume ${\phi }_{\mathrm{\Lambda }}(x)\ge 0$, then ${\phi }_{\mathrm{\Lambda }}(x)$ could replace by $|{\phi }_{\mathrm{\Lambda }}(x)|$. By the Strong maximum principle, we know ${\phi }_{\mathrm{\Lambda }}(x)>0$ a.e. in V. □

Lemma 2 If conditions (S 1) to (S 3) hold, then there exists $\beta ,\rho >0$ such that $J{|}_{\partial B_{\rho }(0)}\ge \beta$, $\mathrm{\forall }u\in V$, $\parallel u\parallel =\rho$.

Proof By condition (S3), there exists $\delta >0$, $\epsilon >0$ such that $\frac{f(x,u)}{u}\le {\lambda }_1-\epsilon$, $\frac{g(x,u)}{u}\le \frac{\gamma f(x,u)}{u}\le {\lambda }_1-\epsilon$ as $0<|u|\le \delta$. Which implies that $F(x,u)\le \frac{1}{2}({\lambda }_1-\epsilon )u^2+c{|u|}^y$, $G(x,u)\le \frac{1}{2}({\lambda }_1-\epsilon )u^2+c{|u|}^z$.

By (4) and (5), we obtain

$$\begin{array}{rcl}J(u)& =& \frac{1}{2}{\parallel \mathrm{\nabla }u\parallel }_{L^2(\mathrm{\Omega })}^2-{\int }_{\mathrm{\Omega }}F(x,u)dx-{\int }_{\mathrm{\Gamma }}G(s,u)ds\\ \ge & \frac{1}{2}{\parallel \mathrm{\nabla }u\parallel }_{L^2(\mathrm{\Omega })}^2+\frac{1}{2}{\parallel \gamma u\parallel }_{L^2(\mathrm{\Gamma })}^2-\frac{1}{2}{\parallel \gamma u\parallel }_{L^2(\mathrm{\Gamma })}^2-\frac{1}{2}({\lambda }_1-\epsilon ){\parallel u\parallel }_{L^2(\mathrm{\Omega })}^2\\ -c{\parallel u\parallel }_{L^y(\mathrm{\Omega })}^y-\frac{1}{2}({\lambda }_1-\epsilon ){\parallel u\parallel }_{L^2(\mathrm{\Gamma })}^2-c{\parallel u\parallel }_{L^z(\mathrm{\Gamma })}^z\\ \ge & \frac{1}{2}{\parallel u\parallel }^2-\frac{1}{2}({\lambda }_1-\epsilon )\frac{1}{{\lambda }_1}{\parallel u\parallel }^2-c{\gamma }_y^y{\parallel u\parallel }^y-\frac{1}{2}({\lambda }_1-\epsilon +1)\frac{1}{{\lambda }_1+1}{\parallel u\parallel }^2-c{k}_z^z{\parallel u\parallel }^z\\ =& [\frac{\epsilon (2{\lambda }_1+1)}{2{\lambda }_1({\lambda }_1+1)}-\frac{1}{2}]{\parallel u\parallel }^2-c{\gamma }_y^y{\parallel u\parallel }^y-c{k}_z^z{\parallel u\parallel }^z.\end{array}$$

Hence, $y,z>2$; we take ε which satisfies $\frac{\epsilon (2{\lambda }_1+1)}{2{\lambda }_1({\lambda }_1+1)}-\frac{1}{2}>0$, that is, $\epsilon >\frac{{\lambda }_1({\lambda }_1+1)}{2{\lambda }_1+1}$. Then we take a positive constant β such that $J{|}_{\partial B_{\rho }(0)}\ge \beta$ as $\parallel u\parallel =\rho$, and is small enough. □

Lemma 3 If conditions (S 1) to (S 3) hold, $\mathrm{\Lambda }<1$, ${\phi }_{\mathrm{\Lambda }}(x)>0$ is defined by Lemma 1, then $J(t{\phi }_{\mathrm{\Lambda }}(x))\to -\mathrm{\infty }$ as $t\to +\mathrm{\infty }$.

Proof If $\mathrm{\Lambda }<1$, ${\phi }_{\mathrm{\Lambda }}(x)>0$ is defined by Lemma 1, by Fatou’s lemma, and (S3), we have

So, $J(t{\phi }_{\mathrm{\Lambda }}(x))\to -\mathrm{\infty }$ as $t\to +\mathrm{\infty }$. □

Lemma 4 Let conditions (S 1) and (S 2) hold. If a sequence $\{u_n\}\subset V$ satisfies $〈J^{\prime }(u_n),u_n〉\to 0$ as $n\to +\mathrm{\infty }$, then there exists a subsequence of $\{u_n\}$, still denoted by $\{u_n\}$ such that $J(t{u}_n)\le \frac{1+t^2}{2n}+J(u_n)$ for all $t>0$, $n\ge 1$.

Proof Since $〈J^{\prime }(u_n),u_n〉\to 0$ as $n\to +\mathrm{\infty }$, for a subsequence, we may assume that

$$-\frac{1}{n}<〈J^{\prime }(u_n),u_n〉={\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2-{\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx-{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds<\frac{1}{n},\mathrm{\forall }n\ge 1.$$

(8)

For any fixed $x\in \mathrm{\Omega }$ and $n\ge 1$, set

$${\psi }_1(t)=\frac{t^2}{2}f(x,u_n)u_n-F(x,t{u}_n),{\psi }_2(t)=\frac{t^2}{2}g(s,u_n)u_n-G(s,t{u}_n).$$

Then (S2) implies that

$$\begin{array}{rcl}{\psi }_1^{\prime }(t)& =& tf(x,u_n)u_n-f(x,t{u}_n)u_n\\ =& t{u}_n[f(x,u_n)-\frac{f(x,t{u}_n)}{t}]\\ =& \{\begin{array}{cc}\ge 0,\hfill & 0<t\le 1;\hfill \\ \le 0,\hfill & t>1.\hfill \end{array}\end{array}$$

It implies that ${\psi }_1(t)\le {\psi }_1(1)$, $\mathrm{\forall }t>0$. Following the same procedures, we obtain ${\psi }_2(t)\le {\psi }_2(1)$, $\mathrm{\forall }t>0$.

For all $t>0$ and positive integer n, by (8), we have

$$\begin{array}{rcl}J(t{u}_n)& =& \frac{t^2}{2}{\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2-{\int }_{\mathrm{\Omega }}F(x,t{u}_n)dx-{\int }_{\mathrm{\Gamma }}G(s,t{u}_n)ds\\ \le & \frac{t^2}{2}[\frac{1}{n}+{\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx+{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds]\\ -{\int }_{\mathrm{\Omega }}F(x,t{u}_n)dx-{\int }_{\mathrm{\Gamma }}G(s,t{u}_n)ds\\ \le & \frac{t^2}{2n}+{\int }_{\mathrm{\Omega }}[\frac{1}{2}f(x,u_n)u_n-F(x,u_n)]dx+{\int }_{\mathrm{\Gamma }}[\frac{1}{2}g(s,u_n)u_n-G(s,u_n)]ds.\end{array}$$

(9)

On the other hand, by (8), one has

$$\begin{array}{rcl}J(u_n)& =& \frac{1}{2}{\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2-{\int }_{\mathrm{\Omega }}F(x,u_n)dx-{\int }_{\mathrm{\Gamma }}G(s,u_n)ds\\ \ge & \frac{1}{2}[-\frac{1}{n}+{\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx+{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds]-{\int }_{\mathrm{\Omega }}F(x,u_n)dx-{\int }_{\mathrm{\Gamma }}G(s,u_n)ds\\ =& -\frac{1}{2n}+{\int }_{\mathrm{\Omega }}[\frac{1}{2}f(x,u_n)u_n-F(x,u_n)]dx+{\int }_{\mathrm{\Gamma }}[\frac{1}{2}g(s,u_n)u_n-G(s,u_n)]ds.\end{array}$$

One has

$${\int }_{\mathrm{\Omega }}[\frac{1}{2}f(x,u_n)u_n-F(x,u_n)]dx+{\int }_{\mathrm{\Gamma }}[\frac{1}{2}g(s,u_n)u_n-G(s,u_n)]ds\le J(u_n)+\frac{1}{2n}.$$

(10)

Combining (9) and (10), we have $J(t{u}_n)\le \frac{1+t^2}{2n}+J(u_n)$. □

Lemma 5 (see [21])

Suppose E is a real Banach space, $J\in C^1(E,\mathbb{R})$ satisfies the following geometrical conditions:

• (i) $J(0)=0$; there exists $\rho >0$ such that $J{|}_{\partial B_{\rho }(0)}\ge r>0$;

• (ii) There exists $e\in E\mathrm{\setminus }\overline{B_{\rho }(0)}$ such that $J(e)\le 0$. Let ${\mathrm{\Gamma }}_1$ be the set of all continuous paths joining 0 and e:

  $${\mathrm{\Gamma }}_1=\{h\in C([0,1],E)|h(0)=0,h(1)=e\},$$

and

$$c=\underset{h\in {\mathrm{\Gamma }}_1}{inf}\underset{t\in [0,1]}{max}J(h(t)).$$

Then there exists a sequence $\{u_n\}\subset E$ such that $J(u_n)\to c\ge \beta$ and $(1+\parallel u_n\parallel )\times {\parallel J^{\prime }(u_n)\parallel }_{E^{\ast }}\to 0$.

3 Proofs of main results

Proof of Theorem 1 (i) If $u\in V$ is one positive solution of problem (1), by (3), one has

$$0=〈J^{\prime }(u),u〉={\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx-{\int }_{\mathrm{\Omega }}f(x,u)udx-{\int }_{\mathrm{\Gamma }}g(s,u)uds.$$

That is,

$$\begin{array}{rcl}{\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }u|}^{2}dx& =& {\int }_{\mathrm{\Omega }}f(x,u)udx+{\int }_{\mathrm{\Gamma }}g(s,u)uds\\ \le & {\int }_{\mathrm{\Omega }}q(x)u^{2}dx+{\int }_{\mathrm{\Gamma }}q(s)u^{2}ds=1.\end{array}$$

It implies that $\mathrm{\Lambda }\le 1$. This completes the proof of Theorem 1(i).

• (ii) By Lemma 2, there exists $\beta ,\rho >0$ such that $J{|}_{\partial B_{\rho }(0)}\ge \beta$ with $\parallel u\parallel =\rho$. By Lemma 3, we obtain $J(t_0{\phi }_{\mathrm{\Lambda }}(x))<0$ as $t_0\to +\mathrm{\infty }$. Define

  

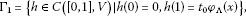  (11)

(12)

where ${\phi }_{\mathrm{\Lambda }}(x)>0$ is given by Lemma 1. Then $c\ge \beta >0$ and by Lemma 3, there exists $\{u_n\}\subset V$ such that

(13)

(14)

• (14) implies that

  $$〈J^{\prime }(u_n),u_n〉={\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2-{\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx-{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds=o(1).$$

  (15)

Here, in what follows, we use $o(1)$ to denote any quantity which tends to zero as $n\to +\mathrm{\infty }$.

If $\{u_n\}$ is bounded in V, when Ω is bounded and $f(x,u)$, $g(x,u)$ are subcritical, we can get $\{u_n\}$ has a subsequence strong convergence to a critical value of J, and our proof is complete. So, to prove the theorem, we only need show that $\{u_n\}$ is bounded in V. Supposing that $\{u_n\}$ is unbounded, that is, $\parallel u_n\parallel \to +\mathrm{\infty }$ as $n\to +\mathrm{\infty }$. We order

$$t_n=\frac{2\sqrt{c}}{\parallel u_n\parallel },w_n=t_n{u}_n=\frac{2\sqrt{c}{u}_n}{\parallel u_n\parallel }.$$

(16)

Then $\{w_n\}$ is bounded in V. By extracting a subsequence, we suppose $w_n\to w$ is a strong convergence in $L^2(\mathrm{\Omega })$, $w_n\to w$ is a convergence a.e. $x\in \mathrm{\Omega }$, $w_n\rightharpoonup w$ is a weak convergence in V.

We claim that $w\ne 0$. In fact, by (S1) and (S3), we know $\mathrm{\forall }x\in \mathrm{\Omega }$, $u_n\ge 0$, and there exists $M_1,M_2>0$ such that $|\frac{f(x,u_n)}{u_n}|\le M_1$, $|\frac{g(x,u_n)}{u_n}|\le M_2$. If $w=0$, $w_n\to 0$ is a strong convergence in $L^2(\mathrm{\Omega })$, and by (15) and (16) we know

$$\begin{array}{rcl}4c& =& t_n^2{\parallel u_n\parallel }^2=t_n^2({\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2+{\parallel \gamma u_n\parallel }_{L^2(\mathrm{\Gamma })}^2)\\ =& t_n^2{\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx+t_n^2{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds+t_n^2{\parallel \gamma u_n\parallel }_{L^2(\mathrm{\Gamma })}^2+o(1)\\ =& {\int }_{\mathrm{\Omega }}\frac{f(x,u_n)}{u_n}{w}_n^{2}dx+{\int }_{\mathrm{\Gamma }}\frac{g(s,u_n)}{u_n}{w}_n^{2}ds+t_n^2{\parallel u_n\parallel }_{L^2(\mathrm{\Gamma })}^2+o(1)\\ \le & M_1{\int }_{\mathrm{\Omega }}{w}_n^{2}dx+M_2{\int }_{\mathrm{\Gamma }}{w}_n^{2}ds+{\parallel w_n\parallel }_{L^2(\mathrm{\Gamma })}^2+o(1)\\ \to & 0.\end{array}$$

It is contradiction with $c>0$, so $w\ne 0$.

As follows, we prove $w\ne 0$ satisfies

$${\int }_{\mathrm{\Omega }}\mathrm{\nabla }\phi (x)\mathrm{\nabla }w(x)dx-{\int }_{\mathrm{\Omega }}{q}_1(x)\phi (x)w(x)dx-{\int }_{\mathrm{\Gamma }}{q}_2(s)\phi (s)w(s)ds=0.$$

We order

By (S1) and (S3), there exists $M_3>0$ such that $0\le p_n(x)\le M_3$, $0\le q_n(x)\le M_3$, $\mathrm{\forall }x\in \overline{\mathrm{\Omega }}$. We select a suitable subsequence and there exists $h_1(x)\in L^2(\mathrm{\Omega })$, $h_2(x)\in L^2(\mathrm{\Gamma })$ such that $p_n(x)\to h_1(x)$ is a strong convergence in $L^2(\mathrm{\Omega })$, $q_n(x)\to h_2(x)$ is a strong convergence in $L^2(\mathrm{\Gamma })$, and $0\le h_1(x)\le M_3$, $0\le h_2(x)\le M_3$, $\mathrm{\forall }x\in \overline{\mathrm{\Omega }}$.

It follows from $w_n\to w$ is a strong convergence in $L^2(\mathrm{\Omega })$ that

Hence, $\{p_n(x)w_n(x)\}$ is bounded in $L^2(\mathrm{\Omega })$, $p_n(x)w_n(x)\rightharpoonup h_1(x)w^{+}(x)$ in $L^2(\mathrm{\Omega })$; $\{q_n(x)w_n(x)\}$ is bounded in $L^2(\mathrm{\Gamma })$, $q_n(x)w_n(x)\rightharpoonup h_2(x)w^{+}(x)$ in $L^2(\mathrm{\Gamma })$.

By (16), we have

Since $w_n\rightharpoonup w$ is a weak convergence in V, we obtain

$${\int }_{\mathrm{\Omega }}\mathrm{\nabla }\phi (x)\mathrm{\nabla }w(x)dx-{\int }_{\mathrm{\Omega }}{h}_1(x)\phi (x)w^{+}(x)dx-{\int }_{\mathrm{\Gamma }}{h}_2(s)\phi (s)w^{+}(s)ds=0,\phi \in V.$$

We order $\phi =w^{-}$; this yields ${\parallel w^{-}\parallel }^2=0$, so $w=w^{+}\ge 0$. By the Strong maximum principle, we know $w>0$ a.e. in Ω, so $u_n\to \mathrm{\infty }$ a.e. in Ω. Combining (S3) and (3), we obtain

$${\int }_{\mathrm{\Omega }}\mathrm{\nabla }\phi (x)\mathrm{\nabla }w(x)dx-{\int }_{\mathrm{\Omega }}q(x)\phi (x)w(x)dx-{\int }_{\mathrm{\Gamma }}q(s)\phi (s)w(s)ds=0,\mathrm{\forall }\phi \in V.$$

This is a contradiction with $\mathrm{\Lambda }<1$. This completes the proof of Theorem 1(ii).

• (iii) If $\mathrm{\Lambda }=1$, by Lemma 1, there exists some ${\phi }_{\mathrm{\Lambda }}(x)>0$, such that

  $${\int }_{\mathrm{\Omega }}\mathrm{\nabla }v(x)\mathrm{\nabla }{\phi }_{\mathrm{\Lambda }}(x)dx={\int }_{\mathrm{\Omega }}q(x)v(x){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}q(s)v(s){\phi }_{\mathrm{\Lambda }}(s)ds.$$

  (17)

If u is a positive solution of (1), for the above ${\phi }_{\mathrm{\Lambda }}(x)$, we have

$${\int }_{\mathrm{\Omega }}\mathrm{\nabla }u(x)\mathrm{\nabla }{\phi }_{\mathrm{\Lambda }}(x)dx={\int }_{\mathrm{\Omega }}f(x,u(x)){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}g(s,u(s)){\phi }_{\mathrm{\Lambda }}(s)ds.$$

(18)

We order $v=u$ in (17), and it follows from (18) that

$$\begin{array}{rcl}{\int }_{\mathrm{\Omega }}\mathrm{\nabla }u(x)\mathrm{\nabla }{\phi }_{\mathrm{\Lambda }}(x)dx& =& {\int }_{\mathrm{\Omega }}q(x)u(x){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}q(s)u(s){\phi }_{\mathrm{\Lambda }}(s)ds\\ =& {\int }_{\mathrm{\Omega }}f(x,u(x)){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}g(s,u(s)){\phi }_{\mathrm{\Lambda }}(s)ds\\ \le & {\int }_{\mathrm{\Omega }}q(x)u(x){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}q(s)u(s){\phi }_{\mathrm{\Lambda }}(s)ds,\end{array}$$

which implies that ${\int }_{\mathrm{\Omega }}(f(x,u)-q(x)u(x)){\phi }_{\mathrm{\Lambda }}(x)dx+{\int }_{\mathrm{\Gamma }}(g(s,u)-q(s)u(s)){\phi }_{\mathrm{\Lambda }}(s)ds=0$.

When ${\phi }_{\mathrm{\Lambda }}(x)>0$ a.e. in Ω, combining (S2), (S3), and (3), we obtain

$$f(x,u)\le q(x)u(x),g(x,u)\le q(x)u(x).$$

Then we must have $f(x,u)=q(x)u(x)$, $g(x,u)=q(x)u(x)$ a.e. in Ω, $u(x)>0$ also achieves Λ (=1). When $u=c{\phi }_{\mathrm{\Lambda }}$, $c>0$, we have ${\int }_{\mathrm{\Omega }}{|\mathrm{\nabla }{\phi }_{\mathrm{\Lambda }}|}^{2}dx={\int }_{\mathrm{\Omega }}q(x){\phi }_{\mathrm{\Lambda }}^{2}dx+{\int }_{\mathrm{\Gamma }}q(s){\phi }_{\mathrm{\Lambda }}^{2}ds$, which achieves Λ.

On the other hand, if for some $c>0$, $u(x)=c{\phi }_{\mathrm{\Lambda }}(x)$ and $f(x,c{\phi }_{\mathrm{\Lambda }}(x))=cq(x){\phi }_{\mathrm{\Lambda }}(x)$, $g(x,u)=cq(x){\phi }_{\mathrm{\Lambda }}(x)$ a.e. $x\in \mathrm{\Omega }$, since $c{\phi }_{\mathrm{\Lambda }}(x)$ also achieves Λ. This means $u(x)=c{\phi }_{\mathrm{\Lambda }}(x)$ is a solution of problem (1) as $\mathrm{\Lambda }=1$. This completes the proof of Theorem 1(iii). □

Proof of Corollary 2 Note that when $q(x)\equiv l$, then $\mathrm{\Lambda }=\frac{{\lambda }_1}{l}$. The conclusion follows from Theorem 1. □

Proof of Theorem 3 When $q(x)\equiv +\mathrm{\infty }$, we can replace ${\phi }_{\mathrm{\Lambda }}$ by ${\phi }_1$ in (11) and define c as in (12), then following the same procedures as in the proof of Theorem 1(ii), we need to show only that $\{u_n\}$ is bounded in V. For this purpose, let $\{w_n\}$ be defined as in (16). If $\{w_n\}$ is bounded in V, we know $w_n\to w$ is a strong convergence in $L^2(\mathrm{\Omega })$, $w_n\to w$ is convergence a.e. $x\in \mathrm{\Omega }$, $w_n\rightharpoonup w$ is a weak convergence in V, and $w\in V$.

If $\parallel u_n\parallel \to +\mathrm{\infty }$, then $t_n\to 0$ and $w(x)\equiv 0$. We set ${\mathrm{\Omega }}_1=\{x\in \mathrm{\Omega }:w(x)=0\}$, ${\mathrm{\Omega }}_2=\{x\in \mathrm{\Omega }:w(x)\ne 0\}$. Obviously, by (16), $|u_n|\to +\mathrm{\infty }$ a.e. in ${\mathrm{\Omega }}_2$. When $q(x)\equiv +\mathrm{\infty }$ in (S3), there exists $K_1,K_2>0$ and n large enough we have $|\frac{f(x,u_n)}{u_n}|\ge K_1$, $|\frac{g(x,u_n)}{u_n}|\ge K_2$ uniformly in $x\in {\mathrm{\Omega }}_2$. Hence, by (15) and (16), we obtain

$$\begin{array}{rcl}4c& =& \underset{n\to +\mathrm{\infty }}{lim}{t}_n^2{\parallel u_n\parallel }^2\\ =& \underset{n\to +\mathrm{\infty }}{lim}{t}_n^2({\parallel \mathrm{\nabla }{u}_n\parallel }_{L^2(\mathrm{\Omega })}^2+{\parallel \gamma u_n\parallel }_{L^2(\mathrm{\Gamma })}^2)\\ =& \underset{n\to +\mathrm{\infty }}{lim}{t}_n^2({\int }_{\mathrm{\Omega }}f(x,u_n)u_{n}dx+{\int }_{\mathrm{\Gamma }}g(s,u_n)u_{n}ds+{\parallel \gamma u_n\parallel }_{L^2(\mathrm{\Gamma })}^2)\\ =& \underset{n\to +\mathrm{\infty }}{lim}({\int }_{\mathrm{\Omega }}\frac{f(x,u_n)}{u_n}{w}_n^{2}dx+{\int }_{\mathrm{\Gamma }}\frac{g(s,u_n)}{u_n}{w}_n^{2}ds+t_n^2{\parallel \gamma u_n\parallel }_{L^2(\mathrm{\Gamma })}^2)\\ \ge & K_1{\int }_{\mathrm{\Omega }}{w}^{2}dx+K_2{\int }_{\mathrm{\Gamma }}{w}^{2}ds+{\parallel w\parallel }_{L^2(\mathrm{\Gamma })}^2.\end{array}$$

Noticing that $w(x)\ne 0$ in ${\mathrm{\Omega }}_2$ and $K_1$, $K_2$ can be chosen large enough, so $m{\mathrm{\Omega }}_2\equiv 0$ and then $w(x)\equiv 0$ in Ω.

Then we know ${lim}_{n\to +\mathrm{\infty }}{\int }_{\mathrm{\Omega }}F(x,w_n)dx+{lim}_{n\to +\mathrm{\infty }}{\int }_{\mathrm{\Gamma }}G(s,w_n)ds=0$, and consequently,

$$\begin{array}{rcl}J(w_n)& =& \frac{1}{2}{\parallel \mathrm{\nabla }{w}_n\parallel }_{L^2(\mathrm{\Omega })}^2+o(1)\\ =& \frac{1}{2}{\parallel w_n\parallel }^2-\frac{1}{2}{\parallel w_n\parallel }_{L^2(\mathrm{\Gamma })}^2+o(1)\\ \ge & \frac{1}{2}(1-\frac{1}{{\lambda }_1+1}){\parallel w_n\parallel }^2+o(1)\\ =& 2c(1-\frac{1}{{\lambda }_1+1})+o(1).\end{array}$$

(19)

By $\parallel u_n\parallel \to +\mathrm{\infty }$, $t_n\to 0$ as $n\to +\mathrm{\infty }$, then it follows Lemma 4 and (13), we obtain

$$J(w_n)=J(t_n{u}_n)\le \frac{1+t_n^2}{2n}\le c.$$

(20)

Obviously, (19) and (20) are contradictory. So $\{u_n\}$ is bounded in V. This completes the proof of Theorem 3. □

4 Example

In this section, we give two examples on $f(x,u)$: One satisfies (S1) to (S3) with $q(x)\equiv +\mathrm{\infty }$, but does not satisfy the (AR) condition; the other illustrates how the assumptions on the boundary are not trivial and compatible with the inner assumptions in Ω.

Example 1 Set:

$$f(x,t)=\{\begin{array}{cc}0,\hfill & t\le 0;\hfill \\ tln(1+t),\hfill & t>0.\hfill \end{array}$$

Then it is easy to verify that $f(x,t)$ satisfies (S1) to (S3) with $p(x)=0$ as $t\to 0$ and $q(x)=+\mathrm{\infty }$ as $t\to +\mathrm{\infty }$. In addition,

$$F(x,t)=\frac{1}{2}{t}^{2}ln(1+t)-\frac{1}{4}{t}^2+\frac{1}{2}t-\frac{1}{2}ln(1+t).$$

So, for some $\mu >2$, $\mu F(x,t)=t^{2}ln(1+t)(\frac{\mu }{2}-\frac{\mu }{4ln(1+t)}+\frac{\mu }{2tln(1+t)}-\frac{\mu }{2t^2})>t^{2}ln(1+t)$, for all t large.

This means $f(x,t)$ does not satisfy the (AR) condition.

Example 2 Consider the following problem:

$$\{\begin{array}{cc}-u^{″}(x)=\alpha u(x),\hfill & 0<x<l,\hfill \\ u(0)=0,\hfill \\ u^{\prime }(l)=\alpha u(l),\hfill \end{array}$$

(21)

where $\alpha >0$ is a constant. It is obvious that $g=\gamma f$ as $f(x,u)=\alpha u(x)$. Problem (21) is a case of (1); we can obtain the nontrivial solution: $u(x)=\tilde{C}sin\sqrt{\alpha }x$, $\tilde{C}\ne 0$.

Author’s contributions

Li G carried out all studies in this article.